Coupling vibration analysis of single-magnet suspension vehicle-bridge for maglev train
Article Text (Baidu Translation)
-
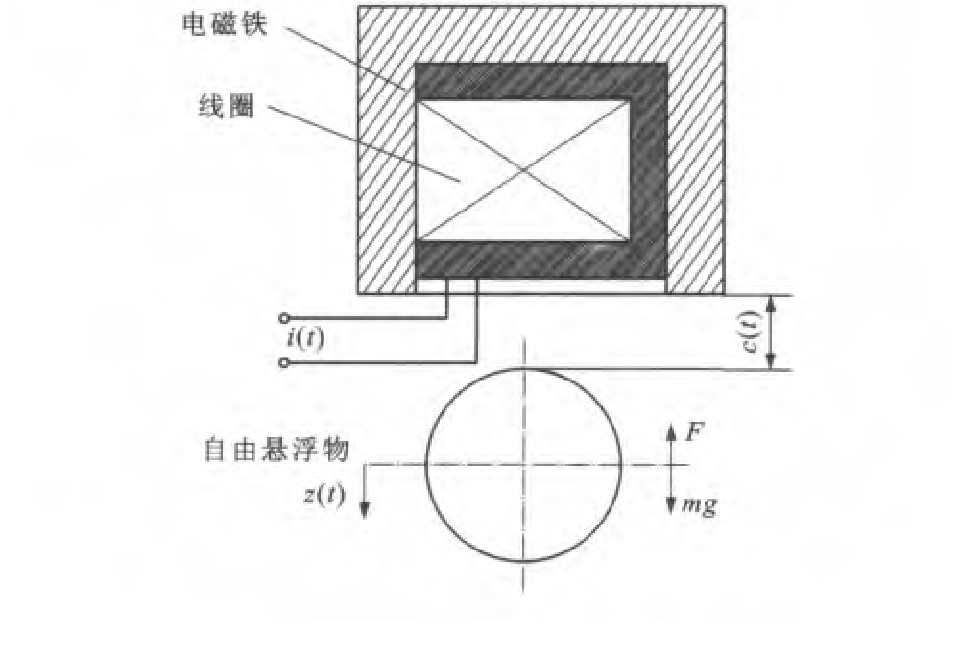
摘要: 为研究单铁悬浮车桥耦合振动, 将悬浮控制系统、车辆结构、弹性轨道梁及桥梁安装系统作为整体系统, 建立整体系统的磁浮列车的悬浮控制-弹性桥梁-机械结构垂向耦合振动模型, 以不同频率的外力激扰模拟磁浮列车不同的速度下对桥梁的作用, 分析了不同梁型在整体系统耦合条件下的跨中挠度与振动加速度的变化。研究结果表明: 单铁悬浮稳定后, 简支梁跨中挠度约为两跨连续梁悬浮处挠度的2.5倍; 以200km·h-1车速通过桥梁时其挠度略小于400km·h-1车速通过工况, 但前者再次达到稳定状态所需时间约为后者的1/3;车辆以相同速度通过桥梁时, 连续梁悬浮处跨中挠度约为简支梁的40%, 且前者振动加速度小于后者; 仿真过程中桥梁安装临界刚度范围为(5.5~6.5)×107 N·m-1; 两跨连续梁动力学性能较简支梁更为优秀。Abstract: In order to study the coupling vibration of single-magnet suspension vehicle-bridge, suspension control system, vehicle structure, elastic track beam and bridge installation system were taken as a whole system, and maglev train suspension control-elastic bridge-mechanical structure vertical coupling vibration model of whole system was established.The effects of maglev train at different speeds on birdge were simulated by using external loads with different frequencies.The changes of mid-span deflection and vibration acceleration for different beam types were analyzed under the coupling condition of whole system.Study result shows that the mid-span deflection of simple-supported beam is about 2.5 times as the suspension position deflection of two-span-continuous beam when single-magnet suspension process achieves stable state.When train passes bridge at the speed of 200 km·h-1, the deflection is slightly smaller than that at the case of 400 km·h-1, the time that requires to achieve stable state for former case is about 1/3 of latter case.The mid-span deflection at suspension position for continuous beams is about 40% of the deflexion for simple-supported beam when train passes bridge at an identical speed, and the vibration acceleration of former case is smaller than that of latter case.The range of bridge critical mounting stiffness in simulation process is from 5.5×107 N·m-1 to 6.5×107 N·m-1, and the dynamics performance of two-span-continuous beam is better than that of simple-supported beam.
-
图 8 简支梁跨中的振动加速度变化
Figure 8. Changes of vibration accelerations at simple-supported beam mid-span
图 10 第1跨跨中振动加速度变化
Figure 10. Changes of vibration accelerations at middle of first span
图 12 第2跨跨中振动加速度变化
Figure 12. Changes of vibration accelerations at middle of second span
-
[1] 姜卫利, 高芒芒. 轨道梁参数对磁浮车-高架桥垂向耦合动力响应的影响研究[J]. 中国铁道科学, 2004, 25(3): 71-75. doi: 10.3321/j.issn:1001-4632.2004.03.015JIANG Wei-li, GAO Mang-mang. Study of the effect of track beam parameters on vertical coupled dynamic response of maglev vehicle-viaduct[J]. China Railway Science, 2004, 25(3): 71-75. (in Chinese). doi: 10.3321/j.issn:1001-4632.2004.03.015 [2] 时瑾, 魏庆朝, 吴范玉. 高速磁浮铁路轨道梁振动分析及控制研究[J]. 中国安全科学学报, 2003, 13(10): 76-80. doi: 10.3969/j.issn.1003-3033.2003.10.022SHI Jin, WEI Qing-chao, WU Fan-yu. Study on vibration of the beam of magnetic levitation express railway and its con-trol[J]. China Safety Science Journal, 2003, 13(10): 76-80. (in Chinese). doi: 10.3969/j.issn.1003-3033.2003.10.022 [3] 邓亚士, 魏庆朝, 时瑾. 高速磁浮桥上轨道梁振动特性初步研究[J]. 振动工程学报, 2008, 21(3): 248-254. doi: 10.3969/j.issn.1004-4523.2008.03.006DENG Ya-shi, WEI Qing-chao, SHI Jin. Vibration charac-teristics of guideway specific for bridge of maglev under irregu-larities[J]. Journal of Vibration Engineering, 2008, 21(3): 248-254. (in Chinese). doi: 10.3969/j.issn.1004-4523.2008.03.006 [4] 翟婉明, 赵春发. 磁浮车辆/轨道系统动力学(Ⅰ)——磁/轨相互作用及稳定性[J]. 机械工程学报, 2005, 41(7): 1-10. doi: 10.3321/j.issn:0577-6686.2005.07.001ZHAI Wan-ming, ZHAO Chun-fa. Dynamics of maglev vehicle/guideway systems(Ⅰ)—magnet/rail interaction and system stability[J]. Chinese Journal of Mechanical Engineering, 2005, 41(7): 1-10. (in Chinese). doi: 10.3321/j.issn:0577-6686.2005.07.001 [5] 赵春发, 翟婉明. 磁浮车辆/轨道系统动力学(Ⅱ)——建模与仿真[J]. 机械工程学报, 2005, 41(8): 163-175. doi: 10.3321/j.issn:0577-6686.2005.08.029ZHAO Chun-fa, ZHAI Wan-ming. Dynamics of maglev vehicle/guideway systems(Ⅱ)—modeling and simulation[J]. Chinese Journal of Mechanical Engineering, 2005, 41(8): 163-175. (in Chinese). doi: 10.3321/j.issn:0577-6686.2005.08.029 [6] LEE J S, KWON S D, KIM M Y, et al. A parametric study on the dynamics of urban transit maglev vehicle running on flexible guideway bridges[J]. Jounal of Sound and Vibration, 2009, 328(3): 301-317. doi: 10.1016/j.jsv.2009.08.010 [7] ROTE D M, CAI Y. Review of dynamic stability of repul-sive-force maglev suspension systems[J]. IEEE Transactions on Magnetics, 2002, 38(2): 1383-1390. doi: 10.1109/20.996030 [8] 李莉, 孟光. 电磁型磁悬浮列车动力学研究综述[J]. 铁道学报, 2003, 25(4): 110-114. https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200304022.htmLI Li, MENG Guang. Summary of the dynamic research on EMS-maglev trains[J]. Journal of the China Railway Society, 2003, 25(4): 110-114. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200304022.htm [9] 鲍佳. 磁浮列车悬浮控制与动力学仿真[D]. 成都: 西南交通大学, 2003.BAO Jia. Suspension control and dynamic simulation of maglev[D]. Chengdu: Southwest Jiaotong University, 2003. (in Chinese). [10] ZHENG Xiao-jing, WU Jian-jun, ZHOU You-he. Numerical analyses on dynamic control of five-degree-of-freedom maglev vehicle moving on flexible guideways[J]. Journal of Sound and Vibration, 2000, 235(1): 43-61. [11] CAI Y, CHEN S S, ROTE D M, et al. Vehicle/guideway dynamic interaction in maglev systems[J]. Journal of Dynamic Systems, Measurement, and Control, 1996, 118(3): 526-530. doi: 10.1115/1.2801176 [12] YAU J D. Aerodynamic vibrations of a maglev vehicle run-ning on flexible guideways under oncoming wind actions[J]. Journal of Sound and Vibration, 2010, 329(10): 1743-1759. [13] JU S H, LIN H T. Resonance characteristics of high-speed trains passing simply supported bridges[J]. Journal of Sound and Vibration, 2003, 267(5): 1127-1141. doi: 10.1016/S0022-460X(02)01463-3 -
下载:
点击查看大图
图(12) / 表(1)
计量
- 文章访问数: 875
- HTML全文浏览量: 153
- PDF下载量: 1015
- 被引次数: 0
