Traffic signal coordinated optimization of urban arterial road based on Petri net
Article Text (Baidu Translation)
-
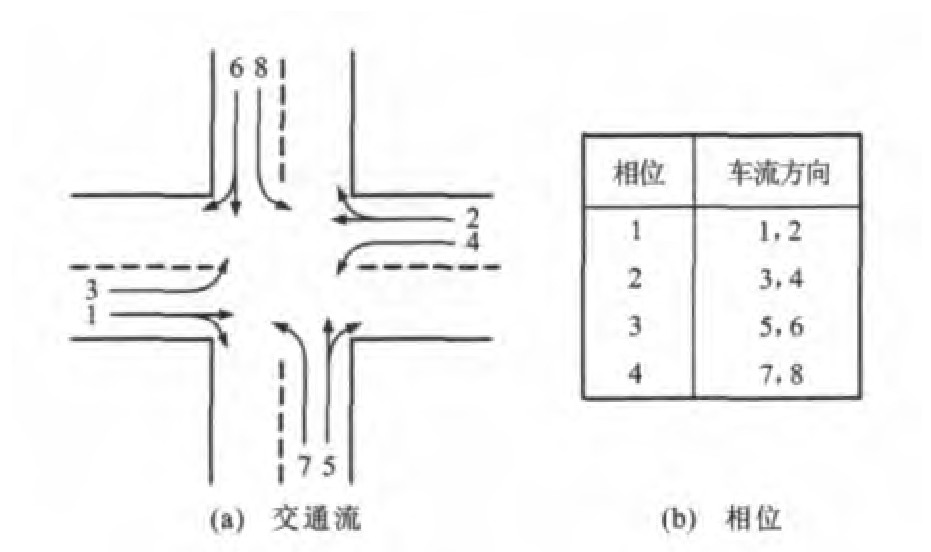
摘要: 为研究城市主干道交通信号协调优化问题, 建立了包括交叉口交通信号显示模块与信号相位转换模块的时延Petri网模型与基于变速度连续Petri网的交通流模型, 设计了由监控、判别和通行相位选择3个子系统构成的交通信号控制系统, 并给出了具体的控制步骤。根据连续Petri网中各参数间的关系, 以车辆排队长度、上游路段车流速度和下游路段畅通度为输入变量, 以相位优先指数为输出变量, 确定下一通行相位, 采用模糊Petri网确定当前相位的最佳绿灯时间, 并进行了仿真计算。仿真结果表明: 采用Petri网与模糊控制相结合的方法后, 由西向东与由东向西方向车流的行程时间分别缩短了7.1%、7.6%, 交叉口排队长度的改进率分别为11.9%、11.2%, 4个相位的交叉口平均延误分别由9.7、10.3、11.8、13.2 s下降到8.2、9.1、11.4、11.4 s。可见, 主干道信号协调优化方法可以较好地实现干线信号协调控制。Abstract: To study the traffic signal coordinated optimization of urban arterial road, a timed Petri net model with a traffic signal display module and a signal phase transition module, and a traffic flow model based on continuous Petri net with variable speeds were established.A traffic signal control system composed of 3 subsystems for monitoring, discriminating and current phase selecting was designed, and concrete control steps were presented. Considering the relationship of parameters in continuous Petri net, next green phase was determined by taking vehicle queue length, traffic flow velocity at upstream section and open degree at downstream section as input variables, and phase priority indexes as output variables.The superior green time of current phase was determined by using fuzzy Petri net, and a simulation calculation was carried out. Simulation result indicates that by combining Petri net with fuzzy control, travel times respectively shorten by 7.1% and 7.6% for west-to-east and east-to-west traffic flow, and the improvement rates of queue length at intersection are 11.9% and 11.2% respectively. The average delays of four phases at intersection decrease from 9.7, 10.3, 11.8, 13.2 s to 8.2, 9.1, 11.4, 11.4 s respectively. So, traffic signal coordinated control on urban arterial road is better realized by using traffic signal coordinated optimization method.
-
Key words:
- traffic signal control /
- coordinated optimization /
- fuzzy control /
- Petri net /
- priority index
-
图 2 交叉口交通流连续Petri网模型
Figure 2. Continuous Petri net model of traffic flow at intersection
图 3 子区段交通流连续Petri网模型
Figure 3. Continuous Petri net model of traffic flow at road section
-
[1] TIAN Z, URBANIK T. System partition technique to improve signal coordination and traffic progression[J]. Journal of Transportation Engineering, 2007, 133(2): 119-128. doi: 10.1061/(ASCE)0733-947X(2007)133:2(119) [2] LIU Y, CHANG G L. An arterial signal optimization model for intersections experiencing queue spillback and lane blockage[J]. Transportation Research Part C: Emerging Technologies, 2011, 19(1): 130-144. doi: 10.1016/j.trc.2010.04.005 [3] LI Z C. Modeling arterial signal optimization with enhanced cell transmission formulations[J]. Journal of Transportation Engineering, 2011, 137(7): 445-454. doi: 10.1061/(ASCE)TE.1943-5436.0000232 [4] 王正武, 罗大庸, 黄中祥, 等. 线控系统协调优化模型及其改进粒子群算法研究[J]. 系统工程理论与实践, 2007, 27(10): 165-171. doi: 10.3321/j.issn:1000-6788.2007.10.023WANG Zheng-wu, LUO Da-yong, HUANG Zhong-xiang, et al. Optimal coordination of artery system based on modified particle swarm algorithm[J]. Systems Engineering—Theory and Practice, 2007, 27(10): 165-171. (in Chinese). doi: 10.3321/j.issn:1000-6788.2007.10.023 [5] GARTNER N H, STAMATIADIS C. Arterial-based control of traffic flow in urban grid networks[J]. Mathematical and Computer Modelling, 2002, 35(5/6): 657-671. [6] 万绪军, 陆化普. 线控系统中相位差优化模型的研究[J]. 中国公路学报, 2001, 14(2): 99-102. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200102025.htmWAN Xu-jun, LU Hua-pu. An optimal offset model for artery traffic signal control system[J]. China Journal of Highway and Transport, 2001, 14(2): 99-102. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200102025.htm [7] FEBBRARO A D, SACCO N. On modelling urban transportation networks via hybrid Petri nets[J]. Control Engineering Practice, 2004, 12(10): 1225-1239. doi: 10.1016/j.conengprac.2004.04.008 [8] TOLBA C, LEFEBVRE D, THOMAS P, et al. Continuous and timed Petri nets for the macroscopic and microscopic traffic flow modelling[J]. Simulation Modelling Practice and Theory, 2005, 13(5): 407-436. doi: 10.1016/j.simpat.2005.01.001 [9] DOTOLI M, FANTI M P. An urban traffic network model via coloured timed Petri nets[J]. Control Engineering Practice, 2006, 14(10): 1213-1229. doi: 10.1016/j.conengprac.2006.02.005 [10] LIST G F, CETIN M. Modeling traffic signal control using Petri nets[J]. IEEE Transactions on Intelligent Transportation Systems, 2004, 5(3): 177-187. doi: 10.1109/TITS.2004.833763 [11] BARZEGAR S, DAVOUDPOUR M, MEYBODI M R, et al. Formalized learning automata with adaptive fuzzy coloured Petri net: an application specific to managing traffic signals[J]. Scientia Iranica D, 2011, 18(3): 554-565. doi: 10.1016/j.scient.2011.04.007 [12] JULVEZ J, BOEL R K. A continuous Petri net approach for model predictive control of traffic systems[J]. IEEE Transactions on Systems, Man, and Cybernetics—Part A: Systems and Human, 2010, 40(4): 686-697. doi: 10.1109/TSMCA.2010.2041448 [13] RECALDE L, HADDAD S, SILVA M. Continuous Petri nets: expressive power and decidability issues[J]. Automated Technology for Verification and Analysis, 2007(4762): 362-377. [14] 梁超, 范炳全, 韩印. 区域交通流协调控制方法[J]. 交通运输工程学报, 2011, 11(3): 112-117. http://transport.chd.edu.cn/article/id/201103019LIANG Chao, FAN Bing-quan, HAN Yin. Coordination control method of regional traffic flow[J]. Journal of Traffic and Transportation Engineering, 2011, 11(3): 112-117. (in Chinese). http://transport.chd.edu.cn/article/id/201103019 [15] 杨劲松, 凌培亮. 一种模糊Petri网的逆向知识推理方法设计实现[J]. 计算机科学, 2009, 36(12): 158-160. https://www.cnki.com.cn/Article/CJFDTOTAL-JSJA200912040.htmYANG Jin-song, LING Pei-liang. Design and implement on a backward reasoning algorithm based on fuzzy Petri net[J]. Computer Science, 2009, 36(12): 158-160. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JSJA200912040.htm -
下载:
点击查看大图
图(8) / 表(4)
计量
- 文章访问数: 924
- HTML全文浏览量: 134
- PDF下载量: 1014
- 被引次数: 0
