Influence of connecting cable chain's elastic modulus on energy conversion of flexible floating collision-prevention system
Article Text (Baidu Translation)
-
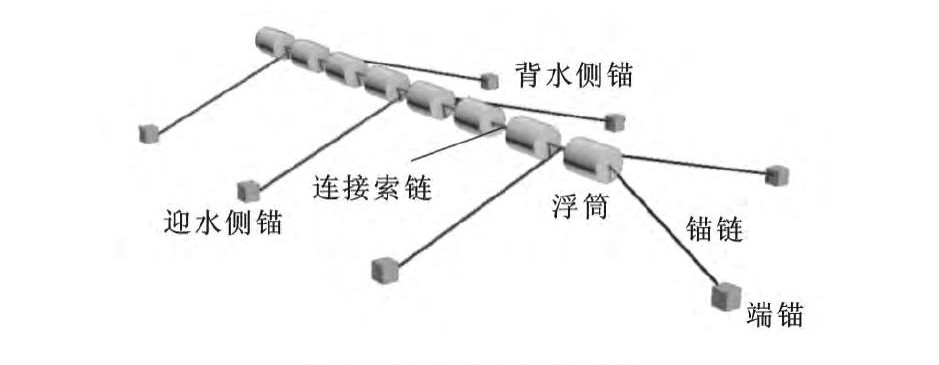
摘要: 为了研究船舶与柔性浮式防撞系统碰撞过程中能量的转换关系, 分析了连接索链弹性模量对系统消能作用的影响。在对数值计算模拟条件做出合理假设的前提下, 基于能量守恒原理, 模拟了船舶撞击柔性浮式防撞系统的运动过程, 分析了不同连接索链弹性模量条件下重力锚位移的变化规律。基于位移相似与能量等量原则, 建立了2种数学模型, 比较了数值计算结果与试验结果的差异。分析结果表明: 连接索链弹性模量衡量了其变形程度, 是影响撞击过程中船舶动能转化为弹性势能的重要因素; 在相同条件下, 连接索链的弹性模量越大, 连接索链越不易发生形变, 船舶撞击系统过程中转化为弹性势能的动能就越小, 转化为重力锚摩擦内能的动能就会越大, 各重力锚的锚位移就会越大; 通过数值计算结果与模型试验结果的比较, 模型试验中连接索链的弹性模量为260 GPa比较合适; 在保证连接索链不发生断裂的前提下, 索链的选取直接影响系统走锚的位移, 从而影响柔性浮式防撞系统的拦阻效果。Abstract: In order to study the energy conversion relations in the process of collision between ship and the flexible floating collision-prevention system (FFCPS), the influence of elastic modulus of connecting cable chain on energy dissipation was analyzed.Based on the basic principle of energy conservation, reasonable assumptions were made on the simulation conditions of numerical calculation, and the movement process of flexible floating collision-prevention system was simulated when the system was hit by ship.Under different elastic moduli of connecting cable chain, the changing rules of moving distances of gravity anchors were analyzed.2 kinds of mathematical models were established based on the principle of similarity in displacement and equality in energy, and the numerical calculation results were compared with the experiment results.Analysis result indicates that the elastic moduli of connecting cable chain measure the deformation degree of cable chain, and it is an important influencing factor in the conversion between ship kinetic energy and elastic potential energy.Under the same conditions, the bigger the elastic modulus of connecting cable chain is, the harder the deformation of connecting cable chain is, the less the energy that ship kinetic energy converts into elastic potential energy is, themore the energy that ship kinetic energy converts into friction energy is, and the longer the moving distance of gravity anchor is.Through the comparison between numerical calculation results and experiment results, the suitable value of elastic modulus of connecting cable chain in model test is 260 GPa.To be sure that the connecting cable chain cannot be broken, the selection of cable chain directly affectes the moving distance of system anchor, thus affects the arresting effect of flexible floating collision-prevention system.
-
图 2 柔性浮式防撞系统模型试验
Figure 2. Model test of flexible floating collision-prevention system
图 5 数值计算与试验得到的锚位移比较
Figure 5. Comparison of moving distances of anchors between numerical calculation and experiment
图 8 数学模型与试验得到的锚位移比较
Figure 8. Comparison of moving distances of anchors between mathematical models and experiment
-
[1] 王汉伟. 桥梁受船舶撞击分析[D]. 重庆: 重庆交通大学, 2014.WANG Han-wei. Analysis of the ship-bridge collision[D]. Chongqing: Chongqing Jiaotong University, 2014. (in Chinese). [2] WANG Li-li, YANG Li-ming, TANG Chang-gang, et al. On the impact force and energy transformation in ship-bridge collisions[J]. International Journal of Protective Structures, 2012, 3(1): 105-120. doi: 10.1260/2041-4196.3.1.105 [3] 郝二通, 柳英洲, 柳春光. 海上风机单桩基础受船舶撞击的数值研究[J]. 振动与冲击, 2015, 34(3): 7-13. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201503003.htmHAO Er-tong, LIU Ying-zhou, LIU Chun-guang. Numerical simulation of monopile foundation of an offshore wind turbine subjected to ship impact[J]. Journal of Vibration and Shock, 2015, 34(3): 7-13. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201503003.htm [4] YUN H, NAYERI R, TASBIHGOO F, et al. Monitoring the collision of a cargo ship with the Vincent Thomas Bridge[J]. Structural Control and Health Monitoring, 2008, 15(2): 183-206. doi: 10.1002/stc.213 [5] FAN Wei, YUAN Wan-cheng. Ship bow force-deformation curves for ship-impact demand of bridges considering effect of pile-cap depth[J]. Shock and Vibration, 2014, 2014(2): 1-19. [6] LEI Zheng-bao, CHEN Zhu, LEI Mu-xi, et al. Research on safety technology for initiative anti-collision of bridge[J]. Applied Mechanics and Materials, 2012, 204-208: 2196-2199. doi: 10.4028/www.scientific.net/AMM.204-208.2196 [7] ZHU Bin, CHEN Ren-peng, CHEN Yun-min, et al. Impact model tests and simplified analysis for flexible pile-supported protective structures withstanding vessel collisions[J]. Journal of Waterway, Port, Coastal and Ocean Engineering, 2012, 138(2): 86-96. doi: 10.1061/(ASCE)WW.1943-5460.0000110 [8] CHANG Liu-hong, JIANG Chang-bao, LIAO Man-jun, et al. Nonlinear dynamic response of buoys under the collision load with ships[J]. Applied Mechanics and Materials, 2012, 204-208: 4455-4459. doi: 10.4028/www.scientific.net/AMM.204-208.4455 [9] 吴广怀, 于群力, 陈徐均. 非通航孔桥的大距离走锚消能式防撞系统[J]. 公路, 2009(1): 213-218. https://www.cnki.com.cn/Article/CJFDTOTAL-GLGL200901047.htmWU Guang-huai, YU Qun-li, CHEN Xu-jun. An energy consumed collision-prevention system of long distance anchor moving for non-navigational bridge[J]. Highway, 2009(1): 213-218. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GLGL200901047.htm [10] 祝世华. 海上大型桥梁非通航孔防撞问题研究[J]. 港工技术, 2011, 48(6): 12-14, 49. doi: 10.3969/j.issn.1004-9592.2011.06.004ZHU Shi-hua. Anti-collision study on non-navigable openings of large sea bridge[J]. Port Engineering Technology, 2011, 48(6): 12-14, 49. (in Chinese). doi: 10.3969/j.issn.1004-9592.2011.06.004 [11] 陈国虞, 倪步友, 张澄, 等. 跨海湾(河湾)桥梁非通航孔柔性拦船防撞装置[J]. 广东造船, 2011, 30(1): 38-41. doi: 10.3969/j.issn.2095-6622.2011.01.009CHEN Guo-yu, NI Bu-you, ZHANG Cheng, et al. Flexible ship-bridge collision protection for the piers of un-navigable spans of bay bridge[J]. Guangdong Shipbuilding, 2011, 30(1): 38-41. (in Chinese). doi: 10.3969/j.issn.2095-6622.2011.01.009 [12] 陈云鹤, 朱应欣, 宋刚, 等. 运河桥梁浮式防船撞设施的模拟计算[J]. 解放军理工大学学报: 自然科学版, 2009, 10(6): 559-564. https://www.cnki.com.cn/Article/CJFDTOTAL-JFJL200906008.htmCHEN Yun-he, ZHU Ying-xin, SONG Gang, et al. Simulation calculation of floating-facility for anti-collision between ship and bridge over canal[J]. Journal of PLA University of Science and Technology: Natural Science Edition, 2009, 10(6): 559-564. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JFJL200906008.htm [13] 陈徐均, 黄光远, 吴广怀. 船舶撞击锚泊防撞系统的能量平衡关系分析[J]. 解放军理工大学学报: 自然科学版, 2009, 10(1): 71-76. https://www.cnki.com.cn/Article/CJFDTOTAL-JFJL200901012.htmCHEN Xu-jun, HUANG Guang-yuan, WU Guang-huai, et al. Energy balance relationship in collision between ship and moored collision-prevention system[J]. Journal of PLA University of Science and Technology: Natural Science Edition, 2009, 10(1): 71-76. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JFJL200901012.htm [14] 陈徐均, 黄光远, 吴广怀, 等. 柔性浮式系统受撞后运动的算法及其收敛性[J]. 解放军理工大学学报: 自然科学版, 2011, 12(5): 501-506. https://www.cnki.com.cn/Article/CJFDTOTAL-JFJL201105019.htmCHEN Xu-jun, HUANG Guang-yuan, WU Guang-huai, et al. New numerical method for flexible floating collision-prevention system and its convergency discussion[J]. Journal of PLA University of Science and Technology: Natural Science Edition, 2011, 12(5): 501-506. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JFJL201105019.htm [15] CHEN Xu-jun, HUANG Guang-yuan, WU Guang-huai, et al. Numerical simulation for the motion of the flexible floating collision-prevention system[J]. Journal of Offshore Mechanics and Arctic Engineering, 2013, 135(1): 1-9. [16] KOTERAYAMA W. Motions of moored floating body and dynamic tension of mooring lines in regular waves[R]. Hakon: Kyushu University, 1978. [17] KOTERAYAMA W, NAKAMURA M. Hydrodynamic forces acting on a vertical circular cylinder oscillating with a very low frequency in waves[J]. Ocean Engineering, 1988, 15(3): 271-287. doi: 10.1016/0029-8018(88)90045-5 [18] KOTERAYAMA W, NAKAMURA M. Drag and inertia force coefficients derived from field tests[J]. International Journal of Offshore and Polar Engineering, 1992, 2(3): 162-167. [19] TOSHIO N. A study of the dynamics of various types by lumped mass method[D]. Tokyo: University of Tokyo, 1991. [20] BLIEK A. Dynamic analysis of single span cables[D]. Boston: Massachusetts Institute of Technology, 1994. [21] NAKAMURA M, KOTERAYAMA W, KYOZUKA Y. Slow drift damping due to drag forces acting on mooring lines[J]. Ocean Engineering, 1991, 18(4): 283-296. doi: 10.1016/0029-8018(91)90015-I [22] SHASHIKALA A P, SUNDARAYADIVELU R, GANAPATHY C. Dynamics of a moored barge under regular and random waves[J]. Ocean Engineering, 1997, 24(5): 401-430. doi: 10.1016/S0029-8018(96)00019-4 [23] FREIRE A M S, NEGRAO J H O, LOPES A V. Geometrical nonlinearities on the static analysis of highly flexible steel cable-stayed bridges[J]. Computers and Structures, 2006, 84(31/32): 2128-2140. [24] DANIELL W E, MACDONALD J H G. Improved finite element modelling of a cable-stayed bridge through systematic manual tuning[J]. Engineering Structures, 2007, 29(3): 358-371. doi: 10.1016/j.engstruct.2006.05.003 [25] 罗伟铭, 石少卿, 田镇华, 等. 钢丝增强复合条带抗弹性能数值分析[J]. 后勤工程学院学报, 2014, 30(5): 6-9, 46. doi: 10.3969/j.issn.1672-7843.2014.05.002LUO Wei-ming, SHI Shao-qing, TIAN Zhen-hua, et al. Numerical analysis of anti-bullet performance of steel-reinforced composite strip[J]. Journal of Logistical Engineering University, 2014, 30(5): 6-9, 46. (in Chinese). doi: 10.3969/j.issn.1672-7843.2014.05.002 [26] 李斌, 韦成龙, 陈积光, 等. 能量法计算线弹性结构位移[J]. 湖南理工学院学报: 自然科学版, 2014, 27(4): 43-45, 92. doi: 10.3969/j.issn.1672-5298.2014.04.010LI Bin, WEI Cheng-long, CHEN Ji-guang, et al. Calculation of displacement of linear elastic structure with energy method[J]. Journal of Hunan Institute of Science and Technology: Natural Sciences, 2014, 27(4): 43-45, 92. (in Chinese). doi: 10.3969/j.issn.1672-5298.2014.04.010 [27] 李啟定, 李克天. 微位移工作台柔性铰链参数分析和优化[J]. 机电工程技术, 2015, 44(1): 72-75. https://www.cnki.com.cn/Article/CJFDTOTAL-JXKF201501020.htmLI Qi-ding, LI Ke-tian. Optimized and parameters analysis of flexible hinge micro-displacement[J]. Mechanical and Electrical Engineering Technology, 2015, 44(1): 72-75. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JXKF201501020.htm -
下载:
点击查看大图
图(8) / 表(4)
计量
- 文章访问数: 842
- HTML全文浏览量: 161
- PDF下载量: 490
- 被引次数: 0
