Dynamics modelling of positioning rubber joint of a bogie based on physical parameters
Article Text (Baidu Translation)
-
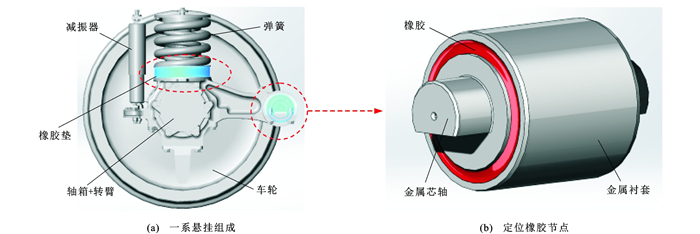
摘要: 针对高速列车转向架悬挂系统中的弹性橡胶件, 开展了基于物理参数的橡胶件非线性动力学建模方法研究; 为准确模拟其非线性刚度与阻尼的硬度相关性、结构尺寸相关性、激励频率相关性和激励位移幅值相关性, 采用有限元软件ABAQUS中的Mooney-Rivlin橡胶本构模型表征橡胶件的刚度与其结构尺寸和胶料硬度之间的相关性, 采用包括分数导数阻尼力元、摩擦力元和弹簧力元的动力学模型表征橡胶件刚度和阻尼的频变、幅变特性, 采用最小二乘法实现基于台架试验的模型参数识别; 对橡胶垫和定位橡胶节点的非线性特性进行仿真和台架试验, 验证了动力学模型的有效性; 在SIMPACK软件中定义自编力元, 进行车辆动力学性能分析, 有限元模型为动力学模型提供了基础的模型参数。分析结果表明: 橡胶垫和定位橡胶节点的刚度与胶料邵氏硬度基本呈正比关系, 硬度80 HA对应的刚度约为60 HA时的2倍; 载荷作用方向的胶料越少其对应方向的刚度越大; 橡胶垫的轴向和径向刚度解耦, 分别受高度和内外径尺寸影响, 橡胶垫轴向刚度随高度的下降率为0.2~0.6 MN·m-1·mm-1; 定位橡胶节点的芯轴尺寸改变引起其轴向和径向刚度同时变化, 定位橡胶节点径向刚度随内径的增长率为3.1~5.2 MN·m-1·mm-1; 采用非线性橡胶件动力学模型的车辆动力学仿真结果与传统等效力元模型结果差异为20%, 说明橡胶垫和定位橡胶节点动态参数的非线性对车辆动力学性能有显著影响。Abstract: Aiming at the elastic rubber components in the suspension system of a high-speed train bogie, the nonlinear dynamics modelling method for the rubber component was studied based on the physical parameters. In order to accurately simulate the correlations between the nonlinear stiffness and the damping hardness, structural dimensions, excitation frequency and excitation displacement amplitude, the Mooney-Rivlin rubber constitutive model in the software ABAQUS was used to characterize the correlation between the rubber component's stiffness and the structural dimensions and rubber hardness. A dynamics model, including a fractional derivative damping force element, a friction force element, and a spring force element, was used to characterize the frequency-dependency and amplitude-dependency of stiffness and damping of a rubber component. The least squares method was used to identify the model parameters based on the lab tests. The nonlinear characteristics of rubber bearing and positioning rubber joint were numerically simulated and tested in the lab to validate the proposed model. The vehicle dynamics performance analysis was performed by using an user-coded force element defined in the software SIMPACK, and the finite element model was used to provide the basic model parameters for the dynamics model. Analysis result shows that the stiffnesses of rubber bearing and positioning rubber joint are basically proportional to the Shore hardness of rubber material, and the stiffness corresponding to the hardness of 80 HA is about twice that at 60 HA. The less the rubber material in the direction of load action, the greater the stiffness in the corresponding direction. The axial and radial stiffnesses of rubber bearing are decoupled, which is affected by the height and the size of inner and outer diameters, respectively, and the decrease rate of the axial stiffness of rubber bearing with the increase of height is 0.2-0.6 MN·m-1·mm-1. Both the axial and radial stiffness of positioning rubber joint varies with the change of mandrel size, and the increase rate of radial stiffness with the increase of inner diameter is 3.1-5.2 MN·m-1·mm-1. The vehicle dynamics simulation results by using the nonlinear rubber component dynamics model are 20% different from the results by using a conventional equivalent force element model, which means the nonlinearities of dynamics parameters of the rubber bearing and positioning rubber joint have severe influence on the vehicle dynamics.
-
Key words:
- vehicle engineering /
- vehicle dynamics /
- bogie /
- rubber component /
- dynamic characteristic /
- nonlinear /
- dynamics simulation
-
图 2 基于物理参数的橡胶件动力学建模方法
Figure 2. Modelling method of rubber component's dynamics based on physical parameters
图 3 橡胶垫和定位橡胶节点的有限元模型
Figure 3. Finite element models of rubber bearing and positioning rubber joint
图 4 橡胶垫轴向刚度与胶料硬度的相关性
Figure 4. Correlation between axial stiffness of rubber bearing and rubber material hardness
图 5 橡胶垫轴向刚度与结构高度的相关性
Figure 5. Correlation between axial stiffness of rubber bearing and structural height
图 6 定位橡胶节点径向刚度与胶料硬度的相关性
Figure 6. Correlation between radial stiffness of positioning rubber joint and rubber material hardness
图 7 定位橡胶节点径向刚度与内径的相关性
Figure 7. Correlation between radial stiffness of positioning rubber joint and inner diameter
图 8 橡胶件动力学模型输出力组成
Figure 8. Output force components of rubber component's dynamics model
图 10 正弦信号sin(2πt)及其不同阶数的分数导数
Figure 10. Sinewave sin(2πt) and its different orders' fractional derivative
图 13 定位橡胶节点动态参数的仿真与试验结果对比(频率6 Hz)
Figure 13. Comparison of dynamic parameters of positioning rubber joint between simulation and experimental results (at frequency 6 Hz)
图 14 定位橡胶节点动态参数的仿真与试验结果对比(频率12 Hz)
Figure 14. Comparison of dynamic parameters of positioning rubber joint between simulation and experimental results (at frequency 12 Hz)
表 1 橡胶件非线性力元的输入参数
Table 1. Input parameters for nonlinear force element of rubber component
参数 单位 构架上的力元标记点 转臂上的力元标记点 初始载荷(纵向、横向和垂向) N 线性弹簧刚度(纵向、横向和垂向) N·m-1 分数导数阶数(纵向、横向和垂向) 迭代步数 等效阻尼系数(纵向、横向和垂向) N·s·m-1 最大摩擦力(纵向、横向和垂向) N 最大摩擦力之半位移(纵向、横向和垂向) mm 
下载: 导出CSV
表 2 橡胶件非线性力元的输出参数
Table 2. Output parameters for nonlinear force element of rubber component
参数 单位 标记点相对位移(纵向、横向和垂向) m 标记点相对速度(纵向、横向和垂向) m·s-1 弹簧力(纵向、横向和垂向) N 阻尼力(纵向、横向和垂向) N 摩擦力(纵向、横向和垂向) N 总输出力(纵向、横向和垂向) N
下载: 导出CSV
-
[1] SJÖBERG M, KARI L. Non-linear behavior of a rubber isolator system using fractional derivatives[J]. Vehicle System Dynamics, 2002, 37(3): 217-236. doi: 10.1076/vesd.37.3.217.3532 [2] BERG M. A non-linear rubber spring model for rail vehicle dynamics analysis[J]. Vehicle System Dynamics, 1998, 30(3/4): 197-212. [3] 吴杰, 上官文斌. 采用黏弹性分数导数模型的橡胶隔振器动态特性的建模及应用[J]. 工程力学, 2008, 25(1): 161-166. https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX200801028.htmWU Jie, SHANGGUAN Wen-bin. Modeling and applications of dynamic characteristics for rubber isolators using viscoelastic fractional derivative model[J]. Engineering Mechanics, 2008, 25(1): 161-166. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX200801028.htm [4] 吴杰, 上官文斌, 潘孝勇. 采用超弹性-黏弹性-弹塑性本构模型的橡胶隔振器动态特性计算方法[J]. 机械工程学报, 2010, 46(14): 109-114. https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201014020.htmWU Jie, SHANGGUAN Wen-bin, PAN Xiao-yong. Computational method for dynamic properties of rubber isolators using hyperelastic-viscoelastic-plastoelastic constitutive model[J]. Journal of Mechanical Engineering, 2010, 46(14): 109-114. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201014020.htm [5] SINGH M P, CHANG T S. Seismic analysis of structures with viscoelastic dampers[J]. Journal of Engineering Mechanics, 2009, 135(6): 571-580. doi: 10.1061/(ASCE)0733-9399(2009)135:6(571) [6] YAJIMA T, NAGAHAMA H. Differential geometry of viscoelastic models with fractional-order derivatives[J]. Journal of Physics A: Mathematical and Theoretical, 2010, 43(38): 1-9. [7] MIEHE C, KECK J. Superimposed finite elastic-viscoelastic-plastoelastic stress response with damage in filled rubbery polymers, experiments, modeling and algorithmic implementation[J]. Journal of the Mechanics and Physics of Solids, 2000, 48(2): 323-365. doi: 10.1016/S0022-5096(99)00017-4 [8] CHANG T S, SINGH M P. Mechanical model parameters for viscoelastic dampers[J]. Journal of Engineering Mechanics, 2009, 135(6): 581-584. doi: 10.1061/(ASCE)0733-9399(2009)135:6(581) [9] 柯维, 郑建华. 基于橡胶硬度的隔振器性能判定[J]. 船海工程, 2008, 37(4): 142-144. doi: 10.3963/j.issn.1671-7953.2008.04.044KE Wei, ZHENG Jian-hua. Judge mount's performance of vibration isolation based on the rubber's stiffness[J]. Ship and Ocean Engineering, 2008, 37(4): 142-144. (in Chinese). doi: 10.3963/j.issn.1671-7953.2008.04.044 [10] 王锐, 李世其, 宋少云. 隔振橡胶本构建模研究[J]. 振动与冲击, 2007, 26(1): 77-79, 83. doi: 10.3969/j.issn.1000-3835.2007.01.021WANG Rui, LI Shi-qi, SONG Shao-yun. Study on constitutive modelling of vibration isolation rubber[J]. Journal of Vibration and Shock, 2007, 26(1): 77-79, 83. (in Chinese). doi: 10.3969/j.issn.1000-3835.2007.01.021 [11] 赵广, 刘健, 刘占生. 橡胶隔振器非线性动力学模型理论与实验研究[J]. 振动与冲击, 2010, 29(1): 173-177. doi: 10.3969/j.issn.1000-3835.2010.01.038ZHAO Guang, LIU Jian, LIU Zhan-sheng. Theoretical and experimental study on nonlinear dynamic model of a rubber isolator[J]. Journal of Vibration and Shock, 2010, 29(1): 173-177. (in Chinese). doi: 10.3969/j.issn.1000-3835.2010.01.038 [12] 陈莲, 周海亭. 计算橡胶隔振器静态特性的数值分析方法[J]. 振动与冲击, 2005, 24(3): 120-123. doi: 10.3969/j.issn.1000-3835.2005.03.035CHEN Lian, ZHOU Hai-ting. Numerical methods for analyzing static characteristics of rubber isolator[J]. Journal of Vibration and Shock, 2005, 24(3): 120-123. (in Chinese). doi: 10.3969/j.issn.1000-3835.2005.03.035 [13] 王晓侠, 刘德立, 周海亭. 橡胶隔振器参数计算与分析[J]. 噪声与振动控制, 2008, 28(4): 9-12. doi: 10.3969/j.issn.1006-1355.2008.04.004WANG Xiao-xia, LIU De-li, ZHOU Hai-ting. Calculation and analysis of the parameter of rubber isolator[J]. Noise and Vibration Control, 2008, 28(4): 9-12. (in Chinese). doi: 10.3969/j.issn.1006-1355.2008.04.004 [14] 姚利锋, 周海亭, 陶杰. 有限元在大载荷橡胶隔振器设计中的应用研究[J]. 噪声与振动控制, 2006, 26(6): 16-18. doi: 10.3969/j.issn.1006-1355.2006.06.005YAO Li-feng, ZHOU Hai-ting, TAO Jie. Application of finite element in design of large load rubber isolator[J]. Noise and Vibration Control, 2006, 26(6): 16-18. (in Chinese). doi: 10.3969/j.issn.1006-1355.2006.06.005 [15] 韩德宝, 宋希庚. 橡胶隔振器刚度和阻尼本构关系的试验研究[J]. 振动与冲击, 2009, 28(1): 156-160. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200901036.htmHAN De-bao, SONG Xi-geng. Experimental study on constitutive model for damping and stiffness of a rubber isolator[J]. Journal of Vibration and Shock, 2009, 28(1): 156-160. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200901036.htm [16] 刘文玺, 周其斗. 基于非线性有限元法的橡胶隔振器构型优化[J]. 船舶力学, 2014, 18(4): 434-440. doi: 10.3969/j.issn.1007-7294.2014.04.011LIU Wen-xi, ZHOU Qi-dou. Study on configuration optimization of rubber isolator by nonlinear FEM[J]. Journal of Ship Mechanics, 2014, 18(4): 434-440. (in Chinese). doi: 10.3969/j.issn.1007-7294.2014.04.011 [17] GIL-NEGRETE N, VINOLAS J, KARI L. A non-linear rubber material model combining fractional order viscoelasticity and amplitude dependent effects[J]. Journal of Applied Mechanics, 2009, 76(1): 1-30. [18] BERG M. A model for rubber springs in the dynamic analysis of rail vehicles[J]. Journal of Rail and Rapid Transit, 1997, 211(2): 95-108. doi: 10.1243/0954409971530941 [19] 潘孝勇, 上官文斌, 柴国钟, 等. 基于超弹性、分数导数和摩擦模型的碳黑填充橡胶隔振器动态建模[J]. 振动与冲击, 2007, 26(10): 6-10, 15. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200710003.htmPAN Xiao-yong, SHANGGUAN Wen-bin, CHAI Guo-zhong, et al. Dynamic modeling for carbon-filled rubber isolators based on hyperelasticity, fractional derivative and a generalized frictional model[J]. Journal of Vibration and Shock, 2007, 26(10): 6-10, 15. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200710003.htm [20] 唐振寰, 罗贵火, 陈伟, 等. 橡胶隔振器黏弹性5参数分数导数并联动力学模型[J]. 航空动力学报, 2013, 28(2): 275-282. https://www.cnki.com.cn/Article/CJFDTOTAL-HKDI201302007.htmTANG Zhen-huan, LUO Gui-huo, CHEN Wei, et al. Parallel dynamic model of rubber isolator about five-parameter fractional derivative[J]. Journal of Aerospace Power, 2013, 28(2): 275-282. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-HKDI201302007.htm [21] SHI Huai-long, WU Ping-bo. A nonlinear rubber spring model containing fractional derivatives for use in railroad vehicle dynamic analysis[J]. Journal of Rail and Rapid Transit, 2016, 230(7): 1745-1759. doi: 10.1177/0954409715614871 [22] 谭富星, 石怀龙, 王玮, 等. 转向架橡胶件动态参数的高低温特性[J]. 交通运输工程学报, 2019, 19(4): 104-114. http://transport.chd.edu.cn/article/id/201904010TAN Fu-xing, SHI Huai-long, WANG Wei, et al. High and low temperature characteristics of rubber component dynamic parameters of a bogie[J]. Journal of Traffic and Transportation Engineering, 2019, 19(4): 104-114. (in Chinese). http://transport.chd.edu.cn/article/id/201904010 [23] 刘迪辉, 范迪, 欧阳雁峰, 等. 温度对橡胶隔振器力学性能的影响[J]. 噪声与振动控制, 2014, 34(3): 203-206, 210. https://www.cnki.com.cn/Article/CJFDTOTAL-ZSZK201403045.htmLIU Di-hui, FAN Di, OUYANG Yan-feng, et al. Temperature effect on mechanical properties of rubber isolators[J]. Noise and Vibration Control, 2014, 34(3): 203-206, 210. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZSZK201403045.htm [24] 宋春元, 罗仁. 低温条件悬挂参数变化对动力学性能的影响研究[C]//中国智能交通协会. 第八届中国智能交通年会论文. 合肥: 电子工业出版社, 2013: 309-314. SONG Chun-yuan, LUO Ren. Study on the effects of parameters on the dynamic performance of the low temperature suspension[C]∥ITS China. Proceedings of 8th Chinese Technological Transportation. Hefei: Publishing House of Electronics Industry, 2013: 309-314. (in Chinese). [25] 孙琳, 林化强, 林鹏, 等. 北方高寒地区轨道车辆橡胶材料的力学特性分析[J]. 材料开发与应用, 2016, 31(6): 88-92. https://www.cnki.com.cn/Article/CJFDTOTAL-CLKY201606020.htmSUN Lin, LIN Hua-qiang, LIN Peng, et al. Mechanical properties analysis of rubber materials for rail vehicles in the North China[J]. Development and Application of Materials, 2016, 31(6): 88-92. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-CLKY201606020.htm [26] 孙德伟, 张广玉. 橡胶隔振器迟滞阻尼特性识别的新方法[J]. 振动与冲击, 2010, 29(4): 164-168. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201004039.htmSUN De-wei, ZHANG Guang-yu. A new approach to identify hysteretic damping of a rubber isolator[J]. Journal of Vibration and Shock, 2010, 29(4): 164-168. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201004039.htm [27] 孙伟, 李以农, 刘万里, 等. 橡胶隔振器非线性动态特性建模及实验研究[J]. 振动与冲击, 2012, 31(23): 71-76. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201223015.htmSUN Wei, LI Yi-nong, LIU Wan-li, et al. Dynamic modeling and test for a nonlinear rubber damper[J]. Journal of Vibration and Shock, 2012, 31(23): 71-76. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201223015.htm [28] LUO Ren, SHI Huai-long, GUO Jin-ying, et al. A nonlinear rubber spring model for the dynamics simulation of a high-speed train[J]. Vehicle System Dynamics, 2019: 1-18. [29] HORGAN C O, MURPHY J G. Compression tests and constitutive models for the slight compressibility of elastic rubber-like materials[J]. International Journal of Engineering Science, 2009, 47(11/12): 1232-1239. [30] 滕万秀, 罗仁, 石怀龙, 等. 高寒动车组-40 ℃环境下动力学性能[J]. 机械工程学报, 2019, 55(4): 148-153. https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201904020.htmTENG Wan-xiu, LUO Ren, SHI Huai-long, et al. Dynamics behaviour of high-speed train at the low temperature of -40 ℃[J]. Journal of Mechanical Engineering, 2019, 55(4): 148-153. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201904020.htm -
点击查看大图
图(17) / 表(2)
计量
- 文章访问数: 895
- HTML全文浏览量: 424
- PDF下载量: 498
- 被引次数: 0
