留言板

尊敬的读者、作者、审稿人, 关于本刊的投稿、审稿、编辑和出版的任何问题, 您可以本页添加留言。我们将尽快给您答复。谢谢您的支持!

姓名
邮箱
手机号码
标题
留言内容
验证码

钢筋混凝土T型梁桥裂缝特征参数与结构评估试验研究

宋一凡,  崔军,  赵小星,  贺拴海

downloadPDF
文章导航 > 交通运输工程学报  > 2001 >  1(3): 46-49
宋一凡, 崔军, 赵小星, 贺拴海. 钢筋混凝土T型梁桥裂缝特征参数与结构评估试验研究[J]. 交通运输工程学报, 2001, 1(3): 46-49.
引用本文: 宋一凡, 崔军, 赵小星, 贺拴海. 钢筋混凝土T型梁桥裂缝特征参数与结构评估试验研究[J]. 交通运输工程学报, 2001, 1(3): 46-49.
shu
SONG Yi-fan, CUI Jun, ZHAO Xiao-xing, HE Shuan-hai. Testing Research on the Relation between the Crack Distinctive Feature and the Structural Performance of Reinforced Concrete T-beams[J]. Journal of Traffic and Transportation Engineering, 2001, 1(3): 46-49.
Citation: SONG Yi-fan, CUI Jun, ZHAO Xiao-xing, HE Shuan-hai. Testing Research on the Relation between the Crack Distinctive Feature and the Structural Performance of Reinforced Concrete T-beams[J]. Journal of Traffic and Transportation Engineering, 2001, 1(3): 46-49.
shu

钢筋混凝土T型梁桥裂缝特征参数与结构评估试验研究

  • 1.

    长安大学 公路学院, 陕西 西安 710064

  • 2.

    浙江大学 土木工程系, 浙江杭州 310027

详细信息
    作者简介:

    宋一凡(1960-), 男, 江苏睢宁人, 长安大学副教授, 博士生, 从事桥梁结构安全研究

  • 中图分类号: U441.4;U448.212

Testing Research on the Relation between the Crack Distinctive Feature and the Structural Performance of Reinforced Concrete T-beams

  • 1.

    School of Highway, Chang′an University, Xi′an 710064, China

  • 2.

    Department of Civil Engineering, Zhejiang University, Hangzhou 310027, China

    摘要
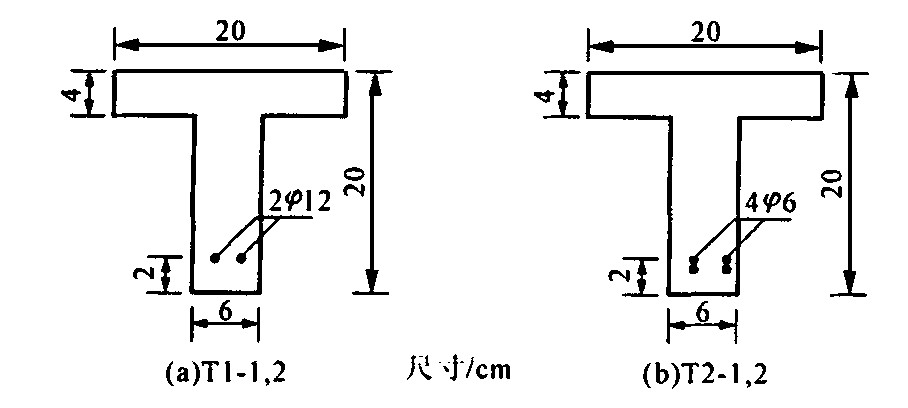
  • 摘要: 通过室内钢筋混凝土模型T梁的荷载试验, 将裂缝特征统计参数经回归分析并结合结构非线性分析, 建立了从裂缝统计特征参数推测结构配筋率的方法, 从而对已严重开裂的钢筋混凝土T型梁桥进行有效评估。将结构外观检查的定性评估提高为定量评估, 具有重要使用价值。

     

    Abstract: Many cracks in the existing reinforced concrete T beam bridges with low criterion are found.Based on crack distinctive feature obtained from the reinforced concrete T beam test in the laboratory, the structural steel ratio in reinforced concrete T beams with many cracks can be forecasted by the way of regressive and nonlinear analysis so that their structural performance can be effectively assessed.The method is simple in theory, convenient in application and reliable in precision. This method changes the assessment for structure from qualitative anlysis to the quantitative calculation.

     

    • HTML全文

  • 图  1  模型T梁截面与配筋

    下载: 全尺寸图片 幻灯片

    图  2  分配梁加载示意

    下载: 全尺寸图片 幻灯片

    图  3  T1-1梁的裂缝统计参数与弯矩M的关系曲线

    下载: 全尺寸图片 幻灯片

    图  4  受压区高度x与曲率φ

    下载: 全尺寸图片 幻灯片

    图  5  β与h¯/h的关系

    下载: 全尺寸图片 幻灯片

    图  6  α与∑δ/(l+lcr)的关系

    下载: 全尺寸图片 幻灯片

    表  1  裂缝特征参数

    M/kN·m h¯/mm ∑δ/mm lcr/mm
    4.576 52.0 0.52 90
    5.275 71.0 0.77 90
    5.882 72.0 0.91 80
    6.516 90.6 1.05 80
    7.171 94.7 1.14 80
    7.810 101.4 1.20 80
    8.460 107.4 1.40 70
    9.100 109.8 1.62 70
    下载: 导出CSV

    表  2  截面非线性分析与参数α、β计算

    梁号 M/kN·m x/mm φ (×10-3) h¯/h ∑δ/(l+lcr) β α
    T1-1 4.576 54.4 3.95 0.260 0.400 2.80 1.44
    5.275 54.4 4.56 0.355 0.592 2.05 1.12
    5.882 53.9 5.18 0.360 0.705 2.03 1.07
    6.516 53.9 5.73 0.453 0.814 1.61 1.03
    7.171 53.7 6.24 0.474 0.884 1.54 1.03
    7.810 53.7 6.80 0.507 0.930 1.44 1.07
    8.460 53.6 7.37 0.537 1.094 1.36 0.98
    9.100 53.6 7.95 0.549 1.266 1.33 0.92
    T1-2 4.865 54.5 4.21 0.349 0.600 2.08 1.02
    5.522 54.4 4.79 0.372 0.667 1.96 1.03
    6.208 54.3 5.46 0.433 0.679 1.68 1.17
    6.849 54.1 5.96 0.479 0.860 1.52 1.08
    7.511 54.0 6.54 0.504 0.866 1.45 1.10
    8.168 53.7 7.12 0.516 0.925 1.42 1.18
    8.778 53.2 7.65 0.558 1.098 1.32 1.03
    9.746 53.1 8.52 0.558 1.406 1.25 0.89
    T2-1 3.975 40.5 5.91 0.441 0.415 1.81 2.14
    4.534 40.5 6.76 0.500 0.577 1.61 1.87
    5.190 40.3 7.74 0.533 0.802 1.50 1.46
    5.782 40.1 7.81 0.549 0.976 1.46 1.43
    6.503 39.7 9.72 0.586 1.200 1.39 1.30
    T2-2 4.550 40.3 6.76 0.420 0.430 1.90 2.51
    5.200 40.1 7.73 0.446 0.624 1.79 1.98
    5.847 40.0 8.70 0.543 0.842 1.47 1.65
    6.515 39.6 9.71 0.620 1.144 1.29 1.36
    7.150 39.2 10.60 0.693 1.258 1.16 1.36
    下载: 导出CSV

    表  3  预测μ和Mj与实际μs和Ms

    梁号 加载M/kN·m μs μ μ-μsμs×100% Ms/kN·m Mj/kN·m Μj-ΜsΜs×100%
    T1-1 4.567 0.0209 0.0232 11.0 10.360 11.107 7.2
    5.275 0.0209 0.0229 9.6 10.360 10.970 5.9
    5.882 0.0209 0.0226 8.1 10.360 10.849 4.7
    6.516 0.0209 0.0217 3.8 10.360 10.401 0.4
    7.171 0.0209 0.0213 1.9 10.360 10.226 -1.3
    7.810 0.0209 0.0210 0.5 10.360 10.078 -2.7
    8.460 0.0209 0.0208 -0.5 10.360 9.959 -3.9
    9.100 0.0209 0.0210 0.5 10.360 10.096 -2.8
    T1-2 4.865 0.0209 0.2230 6.7 10.360 10.719 3.5
    5.522 0.0209 0.0219 4.8 10.360 10.491 1.3
    6.208 0.0209 0.0212 1.4 10.360 10.167 -1.9
    6.849 0.0209 0.0221 5.7 10.360 10.611 2.4
    7.511 0.0209 0.0208 -0.5 10.360 9.993 -3.6
    8.168 0.0209 0.0218 4.3 10.360 10.483 1.2
    8.778 0.0209 0.0218 4.3 10.360 10.478 1.2
    9.746 0.0209 0.0216 3.3 10.360 10.370 0.1
    T2-1 4.550 0.0105 0.0122 16.2 5.324 6.151 15.6
    5.200 0.0105 0.0117 11.4 5.324 5.862 10.1
    5.847 0.0105 0.0116 10.5 5.324 5.830 9.5
    6.515 0.0105 0.0113 7.6 5.324 5.680 6.7
    7.150 0.0105 0.0112 6.7 5.324 5.616 5.5
    T2-2 4.550 0.0105 0.0115 9.5 5.324 5.805 9.1
    5.200 0.0105 0.0113 7.6 5.324 5.700 7.1
    5.847 0.0105 0.0111 5.7 5.324 5.604 5.3
    6.515 0.0105 0.0105 0 5.324 5.292 -0.6
    7.150 0.0105 0.0105 0 5.324 5.240 -1.5
    下载: 导出CSV
    • 参考文献(6)
  • [1] 中华人民共和国交通部标准. 公路旧桥承载能力鉴定方法(试行)[S], 1988.
    [2] 丁大钧. 钢筋混凝土构件抗裂度裂缝和刚度[M]. 南京: 南京工学院出版社, 1986.
    [3] 朱伯龙. 钢筋混凝土非线性分析[M]. 上海: 同济大学出版社, 1985.
    [4] 中华人民共和国交通部标准. (JTJ 023-85) 公路钢筋混凝土和预应力混凝土桥涵设计规范[S], 1985.
    [5] RAINA V K. Concrete bridge-repair, strengthening, testing, load capacity, evaluation[M ]. Tata McGraw-Hill Publishing Company Limited, 1998.
    [6] 胡大琳. 桥涵工程试验检测技术[M]. 北京: 人民交通出版社, 2000.
    • 相关文章
    • 施引文献
  • 资源附件(0)
  • 加载中
WeChat 点击查看大图
图(6) / 表(3)
计量
  • 文章访问数:  257
  • HTML全文浏览量:  75
  • PDF下载量:  303
  • 被引次数: 0
出版历程
  • 收稿日期:  2001-05-20
  • 刊出日期:  2001-09-25

目录

    /

    返回文章
    返回

    版权所有《交通运输工程学报》编辑部陕ICP备05001904号-1

    地址：陕西省 西安市 南二环 路中段 长安大学《交通运输工程学报》编辑部（710064） 电话：029-82334388 Email：jygc@chd.edu.cn

    访问总数:今日访问:

    本系统由 北京仁和汇智信息技术有限公司 开发    百度统计