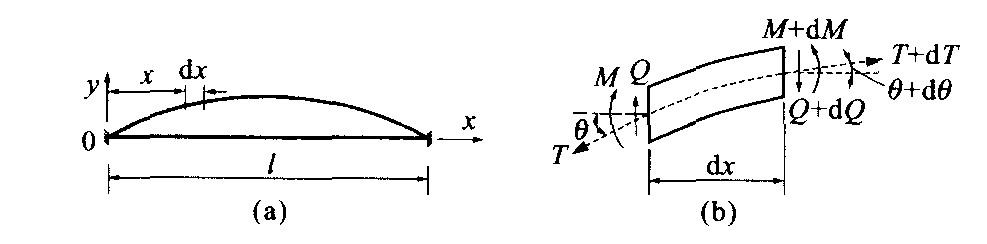柔索索力主频阶次误差及支承条件误差

陈常松; 陈政清; 颜东煌

柔索索力主频阶次误差及支承条件误差

1.
长沙理工大学桥梁与结构工程学院, 湖南长沙 410076
2.
中南大学土木建筑学院, 湖南长沙 410075

基金项目:

交通部重点科技攻关项目 95-05-01-43

详细信息

作者简介:
陈常松(1972-), 男, 湖南攸县人, 长沙理工大学副教授, 中南大学博士研究生, 从事桥梁结构非线性分析与施工控制研究

中图分类号: U441.5
计量
- 文章访问数: 289
- HTML全文浏览量: 93
- PDF下载量: 508
- 被引次数: 0
出版历程
- 收稿日期: 2004-06-20
- 刊出日期: 2004-12-25

Frequency number error and support condition of flexible cable tension

1.
School of Bridge and Structure Engineering, Changsha University of Science and Technology, Changsha 410076, China
2.
School of Civil Engineering and Architecture, Central South University, Changsha 410075, China

More Information

Author Bio:
CHEN Chang-song(1972-), male, associate professor, doctoral student, 86-731-5219360, changsongchen@vip.sina.com

摘要

摘要: 为研究柔索索力测试中拉索自振频率阶次和支承条件对索力测试误差的影响规律, 利用动平衡法推导了考虑弯曲刚度的柔索自振方程和自振频率公式, 采用瑞利能量法分析了弹性支承条件和附加质量对拉索自振频率的影响。研究发现频率阶次对索力计算公式的影响符合二次抛物线分布特征, 附加质量使拉索的自振频率减小, 传感器等测试用的质量块的影响可以忽略不计, 弹性支承引起的误差与弹性链杆刚度成线性关系, 而且与主频的阶次有关。分析和试验结论对大跨度柔索承重结构如斜拉桥和悬索桥的索力测试具有指导意义。
- 桥梁工程 /
- 索力 /
- 自振频率 /
- 频率阶次 /
- 支承条件 /
- 误差
Abstract: In order to study the test errors of cable tension due to natural frequency order and support condition, based on dynamical equilibrium of infinitesimal element, the formulas of natural frequency and cable tension were deduced under flexural rigidity. In terms of Ray-Leiy energy method, the influence of additional mass and elastic constrain on natural frequency was studied. The influence rule of test error due to test frequency order has the character of two times parabola. The natural frequency can decrease when added additional mass, and the influence of sensors mass may be neglected during test. The cable tension error due to elastic support has linear relation with the axial rigidity of support link, which is also related to natural frequency order. All this are instructive to cable tension measurement such as cable-stayed bridge, suspension bridge and other cable-stayed structures.
- bridge engineering /
- cable tension /
- natural frequency /
- frequency order /
- support condition /
- error

HTML全文

图 1 微元dx的受力平衡图

Figure 1. Force equilibrium of infinitesimal element dx

下载: 全尺寸图片幻灯片

图 2 i与ΔT_i的关系

Figure 2. Relation of i and ΔT_i

下载: 全尺寸图片幻灯片

图 3 λ与η的关系

Figure 3. Relation between λ and η

下载: 全尺寸图片幻灯片

图 4 误差ΔT_k与k的关系

Figure 4. Relation of error ΔT_k and k

下载: 全尺寸图片幻灯片

图 5 误差ΔT_k与μ的关系

Figure 5. Relation of error ΔT_k and μ

下载: 全尺寸图片幻灯片

表 1 试验索的主频阶次、主频率及相应的识别误差

Table 1. Main frequency numbers, main frequencies and corresponding identified errors

i	4	6	7	8	12	14	15	16	17
f_i/Hz	4.390	6.599	7.712	8.825	13.330	15.622	16.778	17.937	19.118
T_n/kN	3 101.3	3 112.6	3 123.9	3 135.3	3 155.3	3 208.8	3 224.3	3 238.9	3 259.3
ΔT_i/kN	16.66	36.28	55.89	75.69	110.41	203.28	230.19	255.53	290.95

下载: 导出CSV

参考文献(7)

[1]	季文美. 机械振动[M]. 北京: 科学出版社, 1985.
[2]	Bathe K J, Wilson E L. Numerical Methods in Finite Element Analysis [M]. Prentice-Hall Inc., 1976.
[3]	付志方. 振动模态分析与参数识别[M]. 北京: 机械工业出版社, 1990.
[4]	Clough R W, Penzien J. Dynamics of Structures[M]. Mcgraw-Hill Inc., 1975.
[5]	周传荣, 赵淳生. 机械振动参数识别及其应用[M]. 北京: 科学出版社, 1989.
[6]	李德葆. 振动模态分析及其应用[M]. 北京: 宇航出版社, 1989.
[7]	湖北省交通规划设计院, 长沙理工大学. 荆州长江公路大桥关键技术研究课题报告(第一册, 共三册)[R]. 长沙: 长沙理工大学, 2002.