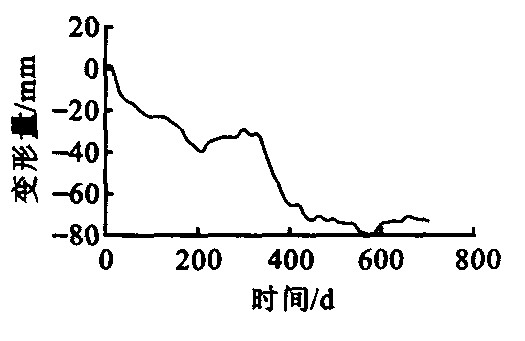
-
摘要: 应用最大Lyapunov指数预测多年冻土路基变形, 分析冻土路基变形的相空间重构方法和不同延迟时间及嵌入维数对最大Lyapunov指数的影响。发现当延迟时间为1, 嵌入维数为5时, 最大Lyapunov指数趋于稳定, 其值为0.00528。运用该指数, 进行冻土路基变形预测, 比较预测变形量和实测变形量, 得到最大相对误差为0.749%, 最小为0.135%。结果表明最大Lyapunov指数能够较好地反映冻土路基变形的混沌特征, 利用其进行冻土路基变形预测是可行的。
-
关键词:
- 路基工程 /
- 多年冻土 /
- 相空间重构 /
- Lyapunov指数 /
- 变形预测
Abstract: The rules of permafrost subgrade deformation were analyzed by maximal Lyapunov exponent method, the phase space reconstruction of subgrade deformation was discussed. It is pointed that various delay time and embedment dimension are two important influence factors on the exponent, when delay time equals 1, embedment dimension equals 5, steady maximal Lyapunov exponent is 0.005 28. Using the exponent value, the maximal relative error of deformation forecast value and measure value is 0.749%, the minimal relative error is 0.135%. The results indicate that maximal Lyapunov exponent ideally reflect the chaos characteristic of subgrade deformation, forecasting subgrade deformation by maximal Lyapunov exponent is feasible. -
表 1 实际观测与预测数据对比
Table 1. Comparison of calculated and measured deformations
时间/d 实测值/mm 预测值/mm 相对误差/% 540 -74.1 -73.9 0.270 555 -78.2 -77.7 0.639 570 -80.0 -79.6 0.500 585 -80.1 -79.5 0.749 600 -75.3 -75.6 0.398 615 -74.0 -74.1 0.135 630 -73.2 -73.4 0.273 645 -73.5 -73.3 0.272 660 -71.3 -71.7 0.561 675 -72.0 -72.1 0.139 690 -72.5 -72.8 0.414 705 -73.5 -74.2 0.52 注: 表中的值为累计变形量。 
下载: 导出CSV
-
[1] 毛雪松, 胡长顺, 侯忠杰. 冻土路基温度场室内足尺模型试验[J]. 长安大学学报(自然科学版), 2004, 24(1): 30-33. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200401008.htmMao Xue-song, Hu Chang-shun, Hou Zhong-jie. Laboratory large-scale test of temperature in permafrost subgrade[J]. Journal of Chang'an University(Natural Science Edition), 2004, 24(1): 30-33. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200401008.htm [2] 邓卫东, 张兴强, 陈波, 等. 路基不均匀沉降对沥青路面受力变形影响的有限元分析[J]. 中国公路学报, 2004, 17(1): 12-15. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200401003.htmDeng Wei-dong, Zhang Xing-qiang, Chen Bo, et al. Nonlinear FEM analysis of influence of asphalt pavement unde non-homogenous settlement of roadbed[J]. China Journal of Highway and Transport, 2004, 17(1): 12-15. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200401003.htm [3] 王东生, 曹磊. 混沌、分形及其应用[M]. 北京: 中国科学技术出版社, 1995. [4] 屈世显, 张建华. 复杂系统的分形理论与应用[M]. 西安: 陕西人民出版社, 1996. [5] 汪富泉, 李后强. 分形几何与动力系统[M]. 哈尔滨: 黑龙江教育出版社, 1993. [6] 陈继光. 基于Lyapunov指数的观测数据短期预测[J]. 水利学报, 2001, 39(9): 65-67. https://www.cnki.com.cn/Article/CJFDTOTAL-SLXB200109011.htmChen Ji-guang. Short term observation data forecasting based on Lyapunov exponent[J]. Journal of Hydraulic Engineering, 2001, 39(9): 65-67. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SLXB200109011.htm [7] 吴耿锋, 周佩玲, 储阅春, 等. 基于相空间重构的预测方法及其在天气预报中的应用[J]. 自然杂志, 1999, 21(2): 108-110. https://www.cnki.com.cn/Article/CJFDTOTAL-ZRZZ199902011.htmWu Geng-feng, Zhou Pei-ling, Chu Yue-chun, et al. Prediction based on phase construction and it's application in weather forecast[J]. Nature Magazine, 1999, 21(2): 108-110. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZRZZ199902011.htm [8] 周创兵, 陈益峰. 基于相空间重构的边坡位移预测[J]. 岩土力学, 2000, 21(3): 205-207. https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX200003003.htmZhou Chuang-bing, Chen Yi-feng. Application of phase space reconstruction in slope displacement forecasting[J]. Rock and Soil Mechanics, 2000, 21(3): 205-207. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX200003003.htm [9] 刘永智, 吴青柏, 张建明, 等. 青藏高原多年冻土地区公路路基变形[J]. 冰川冻土, 2002, 24(1): 11-12. https://www.cnki.com.cn/Article/CJFDTOTAL-BCDT200201001.htmLiu Yong-zhi, Wu Qing-bai, Zhang Jian-ming, et al. Deformation of highway roadbed in permafrost regions of the Tibetan plateau[J]. Journal of Glaciology and Geocryology, 2002, 24 (1): 11-12. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-BCDT200201001.htm -
点击查看大图
图(3) / 表(1)
计量
- 文章访问数: 267
- HTML全文浏览量: 74
- PDF下载量: 239
- 被引次数: 0
