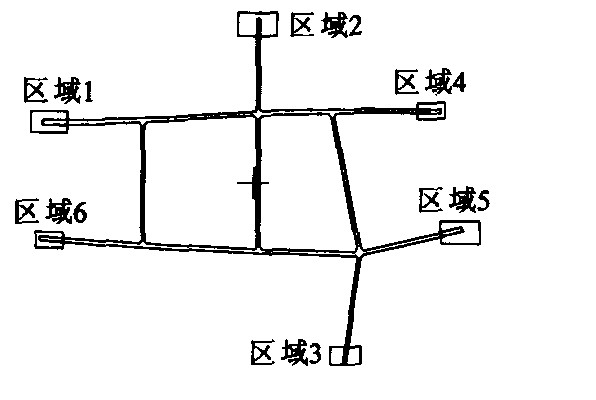
-
摘要: 针对以动态交通管理与控制为目标的动态交通需求计算问题, 分析了OD量与交叉口转向交通量的动态关系, 将其作为新的系统测量量引入, 以此建立了同时考虑路段断面交通量和交叉口转向交通量的状态空间模型, 得到了基于城市道路网络的动态OD估计模型, 给出了考虑不等式约束的卡尔曼滤波递推方程及相应算法过程。利用微观仿真软件Paramics所建立的实验平台对该模型进行了仿真验证。结果表明: 应用该模型进行交通量计算, 与传统的仅考虑路段断面交通量模型相比, 绝对误差平均减少9%, 相对误差平均减少20%, 而且其能更好地反映交通量真实值随时间变化的情况, 计算结果明显优于传统模型。Abstract: In order to study on dynamic traffic demand, which is based on dynamic traffic management and control, the time-varying relation between OD flows and intersection turning counts was analyzed. By introducing intersection turning counts as additional system measurement, a state-space model considering both link counts and intersection turning counts was established, and a new dynamic OD estimation model of urban network was set up, an efficient method based on inequality constrained Kalman filter was proposed to estimate OD matrices dynamically. The proposed model and method were tested by a synthetic network, which was generated by microscopic traffic simulation tool Paramics. The simulation results show that applying the new model, the absolute and relative estimation average errors are reduced by 9% and 20% respectively, and it has better tracking ability to the time-variation of dynamic OD flow, the results are superior to the computational results of traditional model that only considers link counts.
-
Key words:
- traffic planning /
- urban network /
- OD matrices estimation /
- turning counts /
- Kalman filter /
- Paramics software
-
表 1 估计误差分析
Table 1. Estimation error analysis
实验组别 1 2 3 4 5 eMAE/veh eMRE/veh eMAE/veh eMRE/veh eMAE/veh eMRE/veh eMAE/veh eMRE/veh eMAE/veh eMRE/veh 情况1 7.52 0.44 7.50 0.41 7.55 0.45 7.72 0.42 7.84 0.44 情况2 8.13 0.54 8.40 0.55 8.22 0.55 8.59 0.59 8.61 0.56 -
[1] Ashok K, Ben-akiva M E. Dynamic origin-destination matrix estimation and prediction for real-time traffic management systems[A]. Proceedings of the 12th International Symposium on the Theory of Traffic Flow and Transportation[C]. Elsevier Science, 1993. [2] Cascetta E, Inaudi D, Marquis G. Dynamic estimators of origin-destination matrices using traffic counts[J]. Transportation Science, 1993, 27(4): 363-373. [3] Chang G L, Tao X D. Estimation of dynamic O-D distributions for urban networks[A]. Proceedings of the 13th International Symposium on the Theory of Traffic Flow and Transportation [C]. Elsevier Science, 1996. [4] Hazelion M L. Estimation of origin-destination matrices from link flows on uncongested networks[J]. Transportation Research Part B, 2000, 34(7): 549-566. doi: 10.1016/S0191-2615(99)00037-5 [5] Sherali H D, Park T. Estimation of dynamic origin-destination trip tables for a general network[J]. Transportation Research Part B, 2001, 35(3): 217-235. doi: 10.1016/S0191-2615(99)00048-X [6] 王宏禹. 随机数字信号处理[M]. 北京: 科学出版社, 1988. [7] 贾沛璋, 朱征桃. 最优估计及其应用[M]. 北京: 科学出版社, 1984. [8] 常云涛, 彭国雄, 杨晓光. 基于进出口匝道流量的高速公路OD矩阵[J]. 交通运输工程学报, 2003, 3(4): 89-94. http://transport.chd.edu.cn/article/id/200304015Chang Yun-tao, Peng Guo-xiong, Yang Xiao-guang. Freeway OD matrices estimation with on/off ramps traffic counts[J]. Journal of Traffic and Transportation Engineering, 2003, 3 (4): 89-94. (in Chinese) http://transport.chd.edu.cn/article/id/200304015 [9] Simon D, Chia T. Kalman filtering with state equality constraints[J]. IEEE Transaction on Aerospace and Electronic Systems, 2002, 39(1): 128-136. [10] Bell M G H. The estimation of origin-destination matrices by constrained generalised least squares[J]. Transportation Research Part B, 1991, 25(1): 13-22. -





 下载:
下载: