随机疲劳长裂纹扩展率的新概率模型

赵永翔; 杨冰; 张卫华

随机疲劳长裂纹扩展率的新概率模型

西南交通大学牵引动力国家重点实验室, 四川成都 610031

基金项目:

国家自然科学基金项目 50375130

国家自然科学基金项目 50375003

国家自然科学基金项目 50575189

全国优秀博士学位论文作者专项基金项目 200234

新世纪优秀人才支持计划项目 NcET040890

长江学者和创新团队发展计划项目 PcsIRT0452

教育部优秀青年教师计划项目 2101

铁道部科技研究开发计划项目 2001J016

详细信息

作者简介:
赵永翔(1963-)，男，四川金堂人，西南交通大学教授，博士，从事可靠性、维修性和疲劳断裂研究

中图分类号: U270
计量
- 文章访问数: 329
- HTML全文浏览量: 90
- PDF下载量: 223
- 被引次数: 0
出版历程
- 收稿日期: 2005-04-10
- 刊出日期: 2005-12-25

Probabilistic model of random-long fatigue crack propagation rates

State Key Laboratory of Traction Power, Southwest Jiaotong University, Chengdu 610031, China

More Information

Author Bio:
Zhao Yong-xiang(1963-), male, PhD, professor, 86-28-87600935, yxzhao@home.switu.edu.cn

摘要

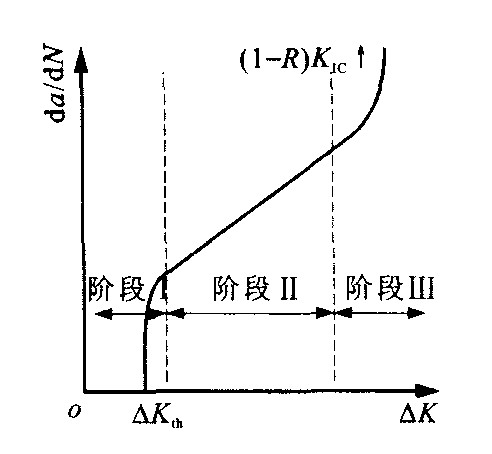
摘要: 为实现在全应力强度因子范围合理进行结构安全性分析, 提出了概全门槛值和断裂韧度的随机疲劳长裂纹扩展率的新概率模型。考虑了平均应力效应, 以给定应力强度因子下裂纹扩展率服从对数正态分布为基础, 考虑数据分散性规律和试样数量对概率评价的影响, 将存活概率和置信度相融合, 由线性回归结合极大似然原理确定概率模型的参数。通过对铁道车辆LZ50车轴钢试验数据的分析表明, 模型从数学上良好描述了疲劳长裂纹从裂纹启裂到瞬时断裂的整个随机过程, 比较Paris、Elber和Forman模型拟合试验数据表明, 该模型相关系数最大, 拟合效果最好。
- 铁道车辆 /
- 疲劳长裂纹 /
- 扩展率 /
- 概率模型 /
- 对数正态分布
Abstract: For the reasonable-probabilistic safety assessment in entire stress intensity factor range, a probabilistic model was presented for characterizing the random-long fatigue crack propagation rates covering the factor range from threshold to fracture roughness. Based on the rates following lognormal distribution, the effects of the scattering regularity of test data and sampling size on the probabilistic assessment were taken into account, and the survival probability and the confidence were coupled in the model. The parameters of the model were measured by linear regression technique and maximum likelihood method. The model was applied to analyze the test data of LZ50 axle steel about Chinese railway vehicles. The result indicates that the entire random process of long fatigue crack growth from crack initiation to fracture can be mathematically described by the model, the correlation coefficient of the model is maximum compared to Paris, Elber and Forman models.
- railway vehicle /
- long fatigue crack /
- growth rate /
- probabilistic model /
- lognormal distribution

HTML全文

图 1 疲劳长裂纹扩展规律

Figure 1. Propagation rule of long fatigue crack

下载: 全尺寸图片幻灯片

图 2 存活概率曲线

Figure 2. Survival probability curves

下载: 全尺寸图片幻灯片

图 3 置信度曲线

Figure 3. Confidence curves

下载: 全尺寸图片幻灯片

图 4 综合概率曲线(C=95%)

Figure 4. Synthetic curves of 95% confidence

下载: 全尺寸图片幻灯片

图 5 本文模型和Paris模型比较

Figure 5. Comparison of presented model and Paris model

下载: 全尺寸图片幻灯片

图 6 本文模型和Elber模型比较

Figure 6. Comparison of presented model and Elber model

下载: 全尺寸图片幻灯片

图 7 本文模型和Forman模型比较

Figure 7. Comparison of presented model and Forman model

下载: 全尺寸图片幻灯片

表 1 模型参数

Table 1. Typical parameters of models

P	C/%	D_PC(×10^-5)	m_PC	ΔK_thPC/MPa·mm^1/2	K_ICPC/MPa·mm^1/2
0.5	50	0.241 788	1.525 5	78.273 6	1 341.06
	90	0.066 577	1.849 1	70.327 7	1 320.86
	95	0.049 553	1.928 9	67.895 8	1 314.68
	99	0.028 795	2.080 3	62.917 0	1 302.02
0.9	50	0.248 99	1.576 1	70.655 9	1 321.69
	90	0.118 29	1.800 1	62.710 0	1 301.49
	95	0.097 15	1.862 5	60.278 1	1 295.31
	99	0.066 94	1.984 6	55.299 2	1 282.65
0.99	50	0.431 44	1.524 9	64.445 4	1 305.90
	90	0.259 28	1.707 9	56.499 5	1 285.70
	95	0.225 83	1.760 5	54.067 6	1 279.52
	99	0.173 63	1.864 3	49.088 8	1 266.86
0.999	50	0.777 06	1.456 43	59.904 8	1 294.36
	90	0.535 57	1.616 34	51.958 9	1 274.16
	95	0.484 06	1.662 76	49.527 0	1 267.98
	99	0.399 63	1.754 96	44.548 1	1 255.32

下载: 导出CSV

参考文献(7)

[1]	Wang G S. Intrinsic statistical characteristics of fatigue crack growth rate[J]. Engineering Fracture Mechanics, 1995, 51(5): 787—803. doi: 10.1016/0013-7944(94)00322-9
[2]	Wang K S, Chang S T, Shen Y C. Dynamic reliability models for fatigue crack growth problem[J]. Engineering Fracture Mechanics, 1996, 54(4): 543—556. doi: 10.1016/0013-7944(95)00216-2
[3]	Rocha M M, Schueller G I. A probabilistic criterion for evaluatingthe goodness of fatigue crack growth models[J]. Engineering Fracture Mechanics, 1996, 53(5): 707—731. doi: 10.1016/0013-7944(95)00132-8
[4]	Paris P, Erdogan F. Acritical analysis of crack growth laws[J]. Journal of Basic Engineering, 1963, 85(10): 528—534.
[5]	Forman R G, Kearney V E, Engle R M. Numerical analysis of crack propagation in cyclic-loaded structure[J]. Journal of Basic Engineering, 1967, 89(9): 459—464.
[6]	赵永翔, 杨冰, 高庆, 等. 提速货车RD2轴的疲劳断裂可靠性与安全性研究[R]. 成都: 西南交通大学, 2004.
[7]	赵永翔, 黄郁仲, 高庆. 铁道车辆LZ50车轴钢的概率机械性能[J]. 交通运输工程学报, 2003, 3(2): 11—17. doi: 10.3321/j.issn:1671-1637.2003.02.003 Zhao Yong-xiang, Huang Yu-zhong, Gao Qing. Probabilistic mechanical properties of LZ50 axle steel for rail way vehicles[J]. Journal of Traffic and Transportation Engineering, 2003, 3(2): 11—17. (in Chinese) doi: 10.3321/j.issn:1671-1637.2003.02.003