Probabilistic Models of Random Fatigue Crack Propagation Based on Forman Equation
-
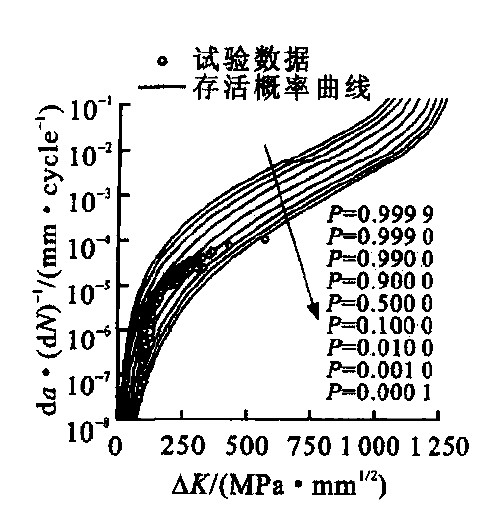
摘要: 为了提高随机疲劳长裂纹扩展率预测精度, 基于Forman方程, 发展了随机疲劳长裂纹扩展概率模型及其参数测定方法, 考虑数据分散性规律和试样数量对概率评价的影响, 得到了包含存活概率曲线、置信度曲线和两者融合曲线在内的长裂纹扩展率关系曲线, 在给定应力强度因子范围内, 裂纹扩展率服从对数正态分布条件下, 采用线性回归和极大似然法测定模型参数。对铁道车辆LZ50车轴钢裂纹扩展数据分析表明, 该模型反映了材料断裂韧度对长裂纹扩展率的影响, 克服了基于Paris-Er-dogan方程的概率模型在高应力强度因子范围预测偏于危险的缺陷, 验证了该模型的合理性。Abstract: In order to improve the prediction precision of random fatigue crack propagation, its probabilistic models based on Forman equation and the estimation method of its parameters were developed, the effect of test data scattering regularity and sampling size on probabilistic assessment was taken into account, the models consisted of survival probability-based curves, confidence-based curves and survival-probability-and-confidence-based curves, their parameters of were measured by linear regression technique and maximum likelihood method.The prediction data of LZ50 axle steel indicate that the models show the effect of material rupture temper on long crack propagation, over come the non-conservative prediction of conventional models based on Paris-Erdogan equation in high intensity factor range, which shows that the models are reasonable and feasible.
-
Key words:
- vehicle engineering /
- long fatigue crack /
- probabilistic model /
- Forman equation
-
表 1 典型P-d a/d N-ΔK曲线方程参量
Table 1. Tab.1 Parameters of P-da/dN-ΔK Curves
存活概率P 材料常数DP 断裂韧度KICP/ (MPa·mm1/2) 材料指数mP 0.500 0 0. 663 972×10-10 1 341.06 3.563 79 0.900 0 0. 521 662×10-9 1 309.24 3.320 01 0.990 0 0. 280 245×10-8 1 283.29 3.121 02 0.999 0 0. 958 435×10-8 1 264.32 2.975 36 0.999 9 0. 263 775×10-7 1 248.71 2.855 38 表 2 典型C-d a/d N-ΔK曲线方程参量
Table 2. Parameters of C-d a/d N-ΔK Curves
置信度C/% 材料常数DC 断裂韧度KICC/ (MPa·mm1/2) 材料指数mC 50 0. 663 972×10-10 1 341.06 3.563 79 90 0. 169 706×10-9 1 341.06 3.563 79 95 0. 226 508×10-9 1 341.06 3.563 79 99 0. 410 499×10-9 1 341.06 3.563 79 表 3 典型P-C-d a/d N-ΔK曲线方程参量
Table 3. Parameters of P-C-d a/d N-ΔK Curves
存活概率P 置信度C/% t分布函数t1-C (ns-2) 材料常数DP-C 断裂韧度KICP-C/ (MPa·mm1/2) 材料指数mP-C 0.900 0 50 0 0. 521 662×10-9 1 309.24 3.320 01 90 1.414 9 0. 133 333×10-8 1 309.24 3.320 01 95 1.894 6 0. 177 960×10-8 1 309.24 3.320 01 99 2.998 0 0. 322 516×10-8 1 309.24 3.320 01 0.990 0 50 0 0. 280 245×10-8 1 283.29 3.121 02 90 1.414 9 0. 716 286×10-8 1 283.29 3.121 02 95 1.894 6 0. 956 029×10-8 1 283.29 3.121 02 99 2.998 0 0. 173 261×10-7 1 283.29 3.121 02 0.999 0 50 0 0. 958 435×10-8 1 264.32 2.975 36 90 1.414 9 0. 244 969×10-7 1 264.32 2.975 36 95 1.894 6 0. 326 961×10-7 1 264.32 2.975 36 99 2.998 0 0. 592 550×10-7 1 264.32 2.975 36 0.999 9 50 0 0. 263 775×10-7 1 248.71 2.855 38 90 1.414 9 0. 674 190×10-7 1 248.71 2.855 38 95 1.894 6 0. 899 843×10-7 1 248.71 2.855 38 99 2.998 0 0. 163 078×10-6 1 248.71 2.855 38 -
[1] WANG G S. Intrinsic Statistical Characteristics of Fatigue Crack Growth Rate[J]. Engineering Fracture Mechanics, 1995, 51 (5): 787-803. doi: 10.1016/0013-7944(94)00322-9 [2] WANG KS, CHANGS T, SHEN YC. Dynamic Reliability Models for Fatigue Crack Growth Problem[J]. Engineering Fracture Mechanics, 1996, 54 (4): 543-556. doi: 10.1016/0013-7944(95)00216-2 [3] ROCHA M M, SCHUELLER GI. A Probabilistic Criterion for Evaluating the Goodness of Fatigue Crack Growth Models[J]. Engineering Fracture Mechanics, 1996, 53 (5): 707-731. doi: 10.1016/0013-7944(95)00132-8 [4] LIU WK, BELYTSCHKO T, LIU YJ. Three Reliability Methods for Fatigue Crack Growth[J]. Engineering Fracture Mechanics, 1996, 53 (5): 733-752. doi: 10.1016/0013-7944(95)00133-6 [5] SHI P, MAHADEVA S. Damage Tolerance Approach for Probabilistic Pitting Corrosion Fatigue Life Prediction[J]. Engineering Fracture Mechanics, 2001, 68 (13): 1 493-1 507. doi: 10.1016/S0013-7944(01)00041-8 [6] SHEN W, SOBOYEJO A B O, SOBOYEJO W O. Probabilistic Modeling of Fatigue Crack Growthin Ti-6Al-4V[J]. International Journal of Fatigue, 2001, 23 (10): 917-925. doi: 10.1016/S0142-1123(01)00045-7 [7] SOBOYEJO W O, SHEN W, LOU J, et al. A Probabilistic Framework for the Modeling of Fatigue in a Lamellar XDTM Gamma Titanium Alloy[J]. International Journal of Fatigue, 2002, 24 (1): 69-81. doi: 10.1016/S0142-1123(01)00043-3 [8] RAY A, TANGIRALAS, RHOHAS. Stochastic Modeling of Fatigue Crack Propagation[J]. Applied Mathematical Modelling, 1998, 22 (3): 197-204. doi: 10.1016/S0307-904X(98)00013-4 [9] 陈国华, 周昌玉, 黄文龙. 疲劳裂纹随机扩展规律分析方法[J]. 南京化工大学学报, 1996, 18 (3): 63-66. https://www.cnki.com.cn/Article/CJFDTOTAL-NHXB603.011.htmCHEN Guo-hua, ZHOU Chang-yu, HUANG Wen-long. Studyon Analysis Method of Fatigue Crack Random Growth Rate[J]. Journal of Nanjing University of Chemical Technology, 1996, 18 (3): 63-66. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-NHXB603.011.htm [10] 洪延姬, 金星, 钟群鹏. 16 Mn R钢疲劳可靠性分析单随机变量模型[J]. 工程力学, 2002, 19 (2): 115-118. https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201008006.htmHONG Yan-ji, JIN Xing, ZHONG Qun-peng. A New Method for Fatigue Reliability Analysis of Chinese Steel[J]. Engineering Mechanics, 2002, 19 (2): 115-118. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201008006.htm [11] PARIS P, ERDOGAN F. A Critical Analysis of Crack Growth Laws[J]. Journal of Basic Engineering, 1963, 85 (3): 528-534. [12] FORMANR G, KEARNEY VE, ENGLE R M. Numerical Analysis of Crack Propagation in Cyclic-Loaded Structure[J]. Journal of Basic Engineering, 1967, 89 (3): 459-464. [13] 肖纪美. 铁路车轴钢40与50的比较[J]. 材料导报, 2000, 14 (6): 7-8.XIAO Ji-mei. Some Comparison Between Steels 50 and 40 Used for Railroad Axles[J]. Transactions on Materials, 2000, 14 (6): 7-8. (in Chinese) [14] 钟群鹏. 对40、50轴钢的几点看法[J]. 材料导报, 2000, 14 (6): 9-10. https://www.cnki.com.cn/Article/CJFDTOTAL-CLDB200006005.htmZHONG Qun-peng. The Views on Carbon Steel 40 and 50 for the Vehicle Shaft[J]. Transactions on Materials, 2000, 14 (6): 9-10. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-CLDB200006005.htm [15] ZHAO Yong-xiang, HE Chao-ming, YANG Bing, et al. Probabilistic Models for the Long Fatigue Crack Growth Rates of LZ50 Axle Steel[J]. Applied Mathematics and Mechanics, 2005, 26 (8): 1 093-1 099. [16] 赵永翔, 黄郁仲, 高庆. 铁道车辆LZ50车轴钢的概率机械性能[J]. 交通运输工程学报, 2003, 3 (2): 11-17. http://transport.chd.edu.cn/article/id/200302024ZHAO Yong-xiang, HUANG Yu-zhong, GAO Qing. Probabilistic Mechanical Properties of LZ50 Axle Steel for Railway Vehicles[J]. Journal of Traffic and Transportation Engineering, 2003, 3 (2): 11-17. (in Chinese) http://transport.chd.edu.cn/article/id/200302024 -





 下载:
下载: