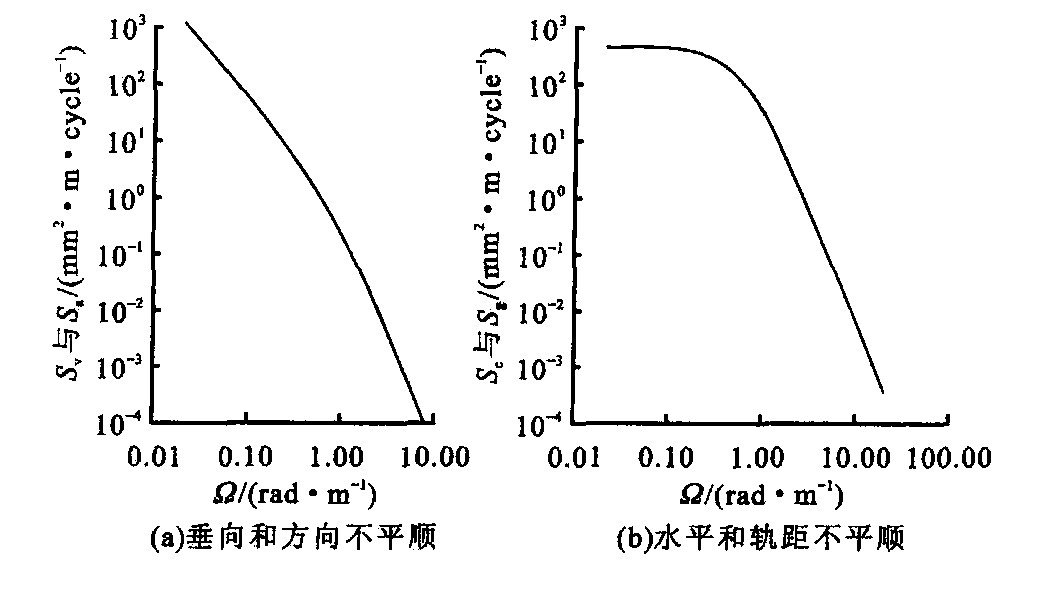
-
摘要: 为了提高将轨道功率谱密度函数(PSD) 通过数值模拟方法转换为时域样本的可靠度, 分析了国内外常用的轨道不平顺数值模拟方法的模拟原理和步骤, 采用计算机仿真程序模拟出各种方法生成的时域样本, 并以此作为车辆垂向动力学模型的输入激励, 通过数值仿真得出系统的时间-响应历程。各种模拟方法模拟结果比较表明采用各种方法模拟出的轨道不平顺时域样本是正确的, 用三角级数法和逆Fourier变换法模拟出的时域样本的离散度较小, 而用白噪声滤波法和二次滤波法模拟的时域样本离散度较大, 因此后两种方法模拟出的轨道不平顺时域样本不宜作为研究系统时域响应时的轨道激励。Abstract: In order to improve the reliability that the power spectrum densities of railway track were transformed into time domain samples by numerical simulation methods, the principles and steps of the methods were analyzed, some simulation samples in time domain were achieved by simulating programs respectively, the samples were taken as the inputting disturbances of a vehicle vertical dynamics model, the time response courses of vehicle system were obtained by numerical simulation.The comparison of simulation results show that each simulated sample in time domain is true, the samples simulated by trigonometric series and IFFT method have little discretenesses, but the samples simulated by white noise filter and secondary filter method have greater discretenesses and are not feasible to act as the track disturbances of studying non-linear system time response.
-
表 1 模型参数
Table 1. Model Parameters
参数 数值 车体质量/kg 34 000 车体绕y轴转动惯量/ (kg·m2) 2.277×106 构架质量/kg 3 000 一系垂向刚度(每轴箱) / (MN·m-1) 0.665 一系垂向阻尼(每轴箱) / (kN·s·m-1) 15 二系垂向刚度(每侧) / (MN·m-1) 0.35 二系垂向阻尼(每侧) / (kN·s·m-1) 80 转向架轴距/m 2.5 车辆定距/m 18 
下载: 导出CSV
-
[1] 许昭鑫. 随机振动[M]. 北京: 高等教育出版社, 1990. [2] 侯传亮, 张永林. 工程平稳随机过程的数值模拟研究[J]. 武汉工业学院学报, 2003, 22 (3): 27-29. https://www.cnki.com.cn/Article/CJFDTOTAL-WHSP200303010.htmHOU Chuan-liang, ZHANG Yong-lin. Numerical Simulation of Stationary Stochastic Process in Engineering[J]. Journal of Wuhan Polytechnic University, 2003, 22 (3): 27-29. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-WHSP200303010.htm [3] 王元丰, 王颖, 王东军. 铁路轨道不平顺模拟的一种新方法[J]. 铁道学报, 1997, 19 (6): 110-115. https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB706.019.htmWANG Yuan-feng, WANG Ying, WANG Dong-jun. A New Simulating Method for the Irregularities of Railway Tracks[J]. Journal of the China Railway Society, 1997, 19 (6): 110-115. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB706.019.htm [4] AVERILL ML, DAVID K. Simulation Modelingand Analysis[M]. New York: Mc Graw-Hill Book Company, 1982. [5] 翟婉明. 车辆轨道耦合动力学[M]. 北京: 中国铁道出版社, 2002. [6] 许慰平. 大跨度铁路桥梁车桥空间耦合振动研究[D]. 北京: 铁道科学研究院, 1989. [7] 陈果, 翟婉明. 铁路轨道不平顺随机过程的数值模拟[J]. 西南交通大学学报, 1999, 34 (2): 138-141. https://www.cnki.com.cn/Article/CJFDTOTAL-XNJT902.002.htmCHEN Guo, ZHAI Wan-ming. Numerical Simulation of the Stochastic Process of Railway Track Irregularities[J]. Journal of Southwest Jiaotong University, 1999, 34 (2): 138-141. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XNJT902.002.htm [8] 王开云, 翟婉明, 蔡成标. 左右轨道不平顺功率谱转换中心线功率谱的方法[J]. 交通运输工程学报, 2002, 2 (3): 27-29. http://transport.chd.edu.cn/article/id/200203006WANG Kai-yun, ZHAI Wan-ming, CAI Cheng-biao. Method of Equivalency Transform for Power Spectrum Density of Track Irregularity on Central Line From on Left/Right Rail[J]. Journal of Traffic and Transportation Engineering, 2002, 2 (3): 27-29. (in Chinese) http://transport.chd.edu.cn/article/id/200203006 [9] 钱雪军. 轨道不平顺的时域模拟法[J]. 铁道学报, 2000, 22 (4): 94-98. https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200004023.htmQIAN Xue-jun. Track Irregularity Simulation in the Time Domain[J]. Journal of the China Railway Society, 2000, 22 (4): 94-98. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200004023.htm [10] 王福天, 周劲松, 任利惠. 用于高速车辆动态仿真的轨道谱分析[J]. 铁道学报, 2002, 24 (5): 21-27. https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200205005.htmWANG Fu-tian, ZHOU Jin-song, REN Li-hui. Analysis on Track Spectrum Density for Dynamic Simulations of High-Speed Vehicles[J]. Journal of the China Railway Society, 2002, 24 (5): 21-27. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200205005.htm -
点击查看大图
图(3) / 表(1)
计量
- 文章访问数: 465
- HTML全文浏览量: 173
- PDF下载量: 298
- 被引次数: 0
