Calculation of Longitudinal Force and Displacement of Seamless Turnout on Continuous Beam Bridge
-
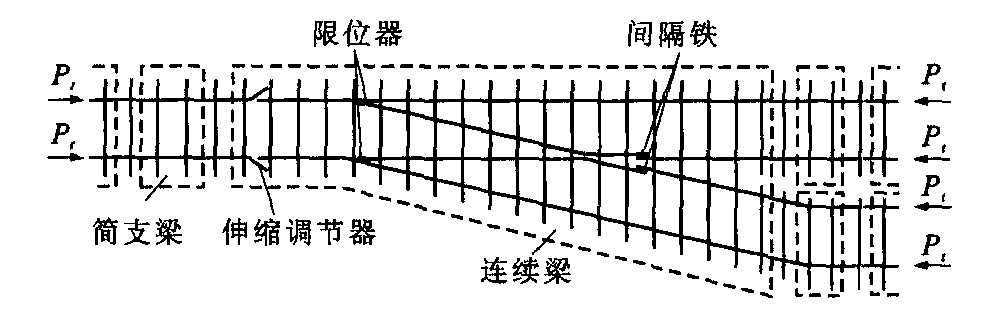
摘要: 将钢轨和梁体视为杆单元, 轨枕视为梁单元, 扣件阻力、道床阻力和桥墩刚度视为弹簧单元, 建立了计算连续梁桥上无缝道岔伸缩力与位移的有限元力学模型, 根据变分原理和“对号入座”法则建立了模型求解的非线性方程组, 分析了道岔设计参数对桥上无缝道岔伸缩力和位移的影响。研究结果表明: 伸缩调节器布置在道岔的后端, 连续梁固定墩的纵向力可降低43.2%;增加连续梁固定墩纵向刚度有利于减小钢轨位移; 连续梁固定支座的位置对系统的受力与变形有双重影响, 实际设计时应综合考虑。Abstract: Rail and beam were regarded as bar elements, sleeper was regarded as beam element, fastening resistance, ballast resistance and pier stiffness were considered as spring elements, a finite element mechanics model was proposed to calculate the longitudinal force and displacement of seamless turnout on continuous beam bridge, the nonlinear equations of the model were established by variational principle and "set-in-right-position" rule, the influences of turnout design parameters on the longitudinal force and displacement were analyzed. Calculation result shows that the longitudinal force on the fixed pier of continuous beam decreases by 43.2% when the expansion joints are laid in the back of seamless turnouts, the longitudinal stiffness increase of the fixed pier leads to the decrease of rail displacement, the fixed support location of continuous beam should be taken into consideration in the system design because it influences the longitudinal force and displacement of the system.
-
表 1 纵向力与位移比较
Table 1. Comparisons of Longitudinal Forces and Displacements
项目 限位器处基本轨位移/mm 尖轨跟端位移/mm 尖轨跟端与连续梁相对位移/mm 心轨跟端位移/mm 心轨跟端与连续梁相对位移/mm 限位器最大阻力/kN 限位器处基本轨纵向力/kN 连续梁端基本轨最大纵向力/kN 连续梁固定墩纵向力/kN 路基无缝道岔 4.74 11.74 - 1.25 - 197.34 1488.21 - - 桥上无缝线路 - - - - - - - 1163.04 2317.68 桥上无缝道岔 计算工况1 22.52 29.52 9.98 5.65 2.68 21.14 1159.51 1065.73 4027.57 计算工况2 -2.65 4.35 9.81 -21.57 1.00 18.03 1180.60 1182.86 2286.54 表 2 纵向力与位移比较
Table 2. Comparisons of Longitudinal Forces and Displacements
项目 限位器处基本轨位移/mm 尖轨跟端位移/mm 尖轨跟端与连续梁相对位移/mm 心轨跟端位移/mm 心轨跟端与连续梁相对位移/mm 限位器最大阻力/kN 限位器处基本轨纵向力/kN 连续梁端基本轨最大纵向力/kN 连续梁固定墩纵向力/kN 连续梁固定墩纵向刚度 减小 工况1 27.06 34.06 9.93 9.00 1.50 18.67 1157.13 942.01 3602.87 工况2 -5.77 1.23 9.82 -24.71 1.00 16.71 1178.42 1116.31 2153.52 增大 工况1 18.76 25.76 10.03 3.09 3.89 23.12 1162.09 1134.54 4328.56 工况2 -0.32 6.68 9.79 -19.21 1.00 19.05 1182.49 1228.04 2373.06 钢轨温度变化幅度/℃ 50 工况1 19.82 26.82 9.32 4.24 3.24 0 937.19 915.34 3432.63 工况2 -2.18 5.82 9.15 -20.26 1.03 0 952.48 1015.85 1949.32 40 工况1 17.17 24.17 8.68 2.72 3.69 0 706.99 761.13 2841.02 工况2 -1.67 6.33 8.54 -19.24 0.78 0 719.77 848.29 1610.38 -
[1] 卢耀荣, 冯淑卿. 桥上无缝线路挠曲力计算[J]. 铁道学报, 1987, 9 (2): 56-67. https://www.cnki.com.cn/Article/CJFDTOTAL-TDBS198504003.htmLOU Yao-rong, FENG Shu-qing. Calculation Method for the Discrete Force of CWR on Railway Bridge[J]. Journal of the China Railway Society, 1987, 9 (2): 56-67. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDBS198504003.htm [2] 梨国清. 高速铁路桥上无缝线路附加力的研究[J]. 中国铁道科学, 1997, 18 (3): 15-23. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK703.001.htmLI Guo-qing. Study on Longitudinal Additional Forces in Continuously Welded Rails on High-Speed Railway Bridges[J]. China Railway Science, 1997, 18 (3): 15-23. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK703.001.htm [3] 蒋金洲. 桥上无缝线路钢轨附加纵向力及其对桥梁墩台的传递[J]. 中国铁道科学, 1998, 19 (2): 67-75. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK802.008.htmJI ANG Jin-zhou. Additional Longitudinal Forces in Continuously Welded Rails and Their Transmission on Railway Bridges[J]. China Railway Science, 1998, 19 (2): 67-75. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK802.008.htm [4] 徐庆元, 陈秀方. 小阻力扣件桥上无缝线路附加力[J]. 交通运输工程学报, 2003, 3 (1): 25-29. http://transport.chd.edu.cn/article/id/200301006XU Qing-yuan, Chen Xiu-fang. Additional Longitudinal Forces Transmission between Bridges and Continuously Welded Railswith Small Resistance Fastener[J]. Journal of Traffic and Transportation Engineering, 2003, 3 (1): 25-29. (in Chinese) http://transport.chd.edu.cn/article/id/200301006 [5] XU Qing-yuan, ZHOU Liao-lin, ZENG Zhi-ping, et al. Mechanics Model of Additional Longitudinal Force Transmission between Bridges and Continuously Welded Rails with Small Resistance Fasteners[J]. Journal of Central South Universityof Technology, 2003, 11 (3): 336-339. [6] 蔡成标. 高速铁路特大桥上无缝线路纵向附加力计算[J]. 西南交通大学学报, 2003, 38 (5): 609-614. https://www.cnki.com.cn/Article/CJFDTOTAL-XNJT200305030.htmCAI Cheng-biao. Calculation of Additional Longitudinal Forces in Continuously Welded Rails on Supper Large Bridges of High-Speed Railways[J]. Journal of Southwest Jiaotong University, 2003, 38 (5): 609-614. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XNJT200305030.htm [7] 范俊杰, 谷爱军, 陈岳源. 无缝道岔的理论与试验研究[J]. 铁道学报, 2000, 22 (2): 55-59. https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200002015.htmFAN Jun-jie, GU Ai-jun, CHEN Yue-yuan. Theoretical Calculation and Testing Analysis for Continuous Welded Turnouts[J]. Journal of China Railway Society, 2000, 22 (2): 55-59. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200002015.htm [8] 王平, 黄时寿. 可动心轨无缝道岔非线性理论计算研究[J]. 中国铁道科学, 2001, 22 (1): 84-91. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK200101015.htmWANG Ping, HUANG Shi-shou. Study on the Nonlinear Theory of Welded Turnout with Movable-Point Frog[J]. China Railway Science, 2001, 22 (1): 84-91. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK200101015.htm -





 下载:
下载: