-
摘要: 为了缓解城市交通拥挤, 分析了城市单点交叉口交通流特性与通行能力, 建立以平均延误时间最短、平均停车次数最少为目标, 以相位有效绿灯时间、饱和度及周期时长为约束条件的城市单点交叉口信号配时优化非线性函数模型, 用遗传算法及遗传模拟退火算法对其进行求解。求解结果表明, 遗传算法及遗传模拟退火算法均可将平均延误由现状的40.394 s优化至10-3 s, 减少了平均停车次数, 获得较为理想的效益值, 将各交叉口服务水平由C级提升到A级。Abstract: In order to mitigate city traffic jam, the traffic flow characteristics and capabilities of urban intersections were analyzed, a nonlinear function model of urban single-point intersections was put forward, in which shortest average delay and stopping time were taken as objectives, and phase effective green time, saturation degree and cycle time length were taken as restrictions, the objective function of the model was solved by genetic algorithm and genetic simulated annealing algorithm. Solved result indicates that average delay is optimized from present 40. 394 s to 10-3 s by genetic algorithm and genetic simulated annealing algorithm, the average stopping time is reduced and the good benefit value of the function is obtained, the intersection service level is improved from level C to level A. 7 tabs, 6 figs, 8 refs.
-
表 1 第1个交叉口参数
Table 1. Parameters of first intersection
交通量/(pcu·h-1) 通行能力/(pcu·h-1) 饱和流量/(pcu·h-1) 流量比 东进口 414 650 1 800 0.22 西进口 496 700 1 800 0.28 南进口 1 432 1 800 2 800 0.51 北进口 1 484 1 800 2 800 0.53 
下载: 导出CSV
表 2 算法优化结果对比
Table 2. Comparison of algorithm optimization results
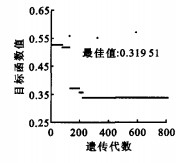
一相位有效绿时/s 二相位有效绿时/8 周期时长/s 平均延误/(s·veh-1) 平均停车次数 效益值 进化代数 现状 41.000 00 8Z.000 00 133.000 00 34.891 80 0.596 0 17.743 80 — Webster 34.000 00 61.000 00 105.000 00 32.951 20 0.628 4 16.789 70 — 遗传算法 30.605 14 70.715 86 111.321 00 0.052 82 0.586 2 0.319 51 823 遗传退火算法 25.189 90 82.112 64 117.302 50 0.031 70 0.532 2 0.281 96 417
下载: 导出CSV
表 3 交叉口机动车延误与服务水平
Table 3. Vehicle delay and service level of intersection
服务水平 每车停车延误/s A ≤10 B 11~20 C 21~35 D 36~45
下载: 导出CSV
表 4 第2个交叉口参数
Table 4. Parameters of second intersection
交通量/(pcu·h-1) 通行能力/(pcu·h-1) 饱和流量/(pcu·h-1) 流量比 东进口 1 320 1 800 3 000 0.44 西进口 1 254 1 800 3 000 0.42 南进口 1 450 1 800 3 400 0.43 北进口 1 540 1 800 3 400 0.45
下载: 导出CSV
表 5 算法优化结果对比
Table 5. Comparison of algorithm optimization results
一相位有效绿时/s 二相位有效绿时/s 周期时长/s 平均延误/(s·veh-1) 平均停车次数 效益值 进化代数 现状 90.000 00 100.000 00 200.000 00 44.394 00 0.777 0 22.585 00 — Webster 90.00000 92.000 00 192.00000 3Z.927 40 0.776 2 16.851 80 — 遗传算法 47.301 95 56.586 22 113.88817 0.002 20 0.765 4 0.383 89 16 遗传退火算法 51.882 33 62.951 58 124.833 91 0.001 20 0.755 2 0.378 20 477
下载: 导出CSV
表 6 第3个交叉口参数
Table 6. Parameters of third intersection
交通量/(pcu·h-1) 通行能力/(pcu·h-1) 饱和流量/(pcu·h-1) 流量比 东进口 1 240 1 800 2 800 0.44 西进口 1 230 1 800 2 800 0.44 南进口 1 180 2 400 3 400 0.35 北进口 1 480 2 400 3 400 0.44
下载: 导出CSV
表 7 算法优化结果对比
Table 7. Comparison of algorithm optimization results
一相位有效绿时/s 二相位有效绿时/s 周期时长/s 平均延误/(s·veh-1) 平均停车次数 效益值 进化代数 现状 75.000 00 92.000 00 177.000 00 40.394 00 0.787 0 20.590 50 — Webster 78.000 00 79.000 00 167.000 00 30.208 70 0.776 2 15.492 45 — 遗传算法 43.061 50 47.090 50 100.151 20 0.000 18 0.747 4 0.373 77 41 遗传退火算法 68.927 43 80.440 69 159.368 12 0.001 80 0.725 4 0.363 59 437
下载: 导出CSV
-
[1] 李峰, 王秋平. 单点信号交叉口通行能力和服务水平分析[J]. 城市问题, 2003, 22(2): 30-33. doi: 10.3969/j.issn.1002-2031.2003.02.009Li Feng, Wang Qiu-ping. Capacity and Ievel analysis of singlepoint intersection[J]. urban Problems, 2003, 22(2): 30-33. (in Chinese) doi: 10.3969/j.issn.1002-2031.2003.02.009 [2] 徐良杰, 王炜. 信号交叉口行人过街时间模型[J]. 交通运输工程学报, 2005, 5(1): 111-115. http://transport.chd.edu.cn/article/id/200501026Xu Liang-jie, Wang Wei. Model of pedestrians crossing time at signalized intersection[J]. Joumal of Traffic and Transportation Engineering, 2005, 5(1): 111-115. (in Chinese) http://transport.chd.edu.cn/article/id/200501026 [3] 陈群, 晏克非. 基于遗传算法的城市交叉口实时信号控制研究[J]. 交通与计算机, 2005, 23(1): 15-18. https://www.cnki.com.cn/Article/CJFDTOTAL-JTJS200501003.htmChen Qun, Yan Ke-fei. Real-time signal control of urban intersection based on genetic algorithm[J]. Computer and communications, 2005, 23(1): 15-18. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JTJS200501003.htm [4] 杨佩昆, 张树升. 交通管理与控制[M]. 北京: 人民交通出版社, 1995. [5] 蒲琪, 谭永朝, 杨超. 交叉口信号配时优化模型研究[J]. 上海铁道大学学报, 1994, 15(4): 31-34. https://www.cnki.com.cn/Article/CJFDTOTAL-SHTY199904004.htmPu Qi, Tan Yong-chao, Yang Chao. Signal-planning optimal model for intersection[J]. Journal of shanghai Tiedao universjty, 1994, 15(4): 31-34. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SHTY199904004.htm [6] 邬晓光, 冯祁, 郭扬. 基于遗传算法的桥梁施工资源有限优化[J]. 长安大学学报: 自然科学版, 2004, 24(6): 33-36. doi: 10.3321/j.issn:1671-8879.2004.06.008Wu Xiao-guang, Feng Qi, Guo Yang. Finite optimization of bridge construction resources based on genetic algorithms[J]. Journal of Chang'an University: Natural Science Edition, 2004, 24(6): 33-36. (in Chinese) doi: 10.3321/j.issn:1671-8879.2004.06.008 [7] 张波, 叶家玮, 胡郁葱. 模拟退火算法在路径优化问题中的应用[J]. 中国公路学报, 2004, 17(1): 79-81. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200401019.htmZhang Bo, Ye Jia-wei, Hu Yu-cong. Application of optimizing the path by simulated annealing[J]. China Journal Highway and Transport, 2004, 17(1): 79-81. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200401019.htm [8] 杨晓光. 城市道路交通设计指南[M]. 北京: 人民交通出版社, 2003. -
点击查看大图
计量
- 文章访问数: 363
- HTML全文浏览量: 139
- PDF下载量: 739
- 被引次数: 0
