Influence of non-steady state loading on two-dimensional wheel-rail pure rolling contact stresses and deformation
-
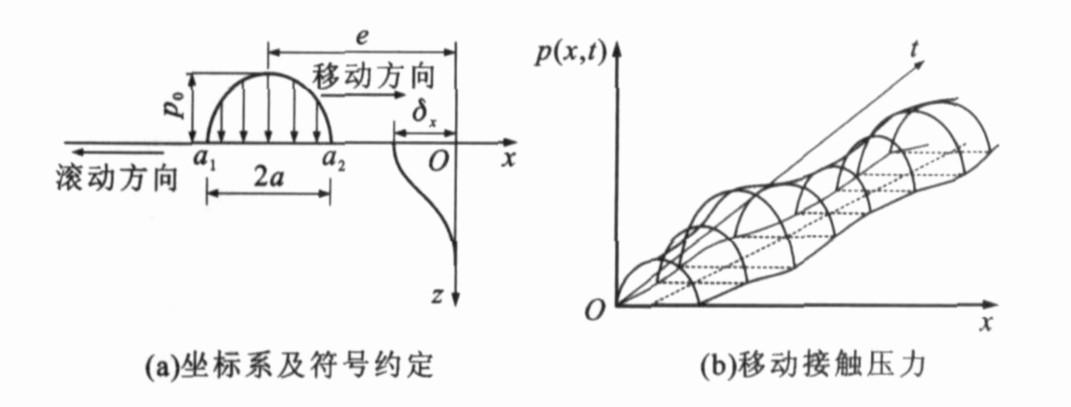
摘要: 为了揭示轮轨波状表面与非稳态载荷的内在联系, 利用有限元法, 建立了二维弹塑性轮轨纯滚动接触计算模型, 分析法向接触载荷波动值对钢轨残余应力、应变和变形的影响。模型中材料本构采用考虑棘轮效应的Jiang-Sehitoglu模型, 非稳态仅考虑法向接触载荷的简谐变化, 用弹塑性无限半空间表面上重复移动赫兹法向压力分布模拟反复纯滚动接触过程。发现非稳态法向接触载荷作用下产生同样波长的波状接触表面; 随滚动次数的增加, 残余应力增大, 但很快趋于稳定, 而残余应变也增大, 但增大速率衰减; 载荷波动值越大, 波谷和波峰处的纵向残余应力越大, 波谷处的轴向残余应力、残余剪应变和表面纵向位移越大, 而波峰处的轴向残余应力、残余剪应变和表面纵向位移越小, 波深越大。Abstract: In order to study the relation between wheel-rail corrugation surface and non-steady state loading, a two-dimensional elastic-plastic model of repeated frictionless wheel-rail rolling contact was established, the effects of the fluctuating amplitude coefficient of normal contact pressure on the residual stresses, strains and displacements of rail were analyzed.An advanced cyclic plasticity model developed by Jiang and Sehitoglu was used, material ratchetting effect was considered, non-steady state rolling contact was only considered as the harmonic variation of normal contact pressure, repeated rolling contact was simulated by the multiple translations of varying semielliptical Hertzian pressure distribution across elastic-plastic semi-infinite half space.Analysis result shows that non-steady state normal contact pressure results in a wavy rail surface profile with the same wavelength as the pressure; as rolling time increases, rail residual stress increases, but tends to stabilize, rail residual strain also increases, but ratchetting rate decays; when preassure fluctuating amplitude coefficient increases, rail residual stress in rolling direction below the trough and crest of wavy deformation increase respectively, rail residual stress in axial direction, rail residual shear strain and surface displacement in longitudinal direction below the trough increase respectively, but rail residual stress in axial direction, rail residual shear strain and rail surface displacement in longitudinal direction below the crest decrease respectively, the wave depth increases.
-
图 9 C0对残余应力和残余剪应变的影响
Figure 9. Influences of C0 onresidual stresses and residual shear strains
表 1 塑性模型
Table 1. Plasticity model
屈服准则 f= (S-α) · (S-α) -2k2=0 S为偏应力张量; α为背应力张量; k为剪切屈服强度 流动准则 n为屈服面的法线方向; h为塑性模量; εp为塑性应变张量; 〈〉为Macauley括号, 即〈x〉=0.5 (x+x) 硬化准则 α= αi为第i个背应力张量分量; M为背应力张量分量总数; dp为等效塑性应变增量; ci、ri、χi为材料常数 
下载: 导出CSV
表 2 材料常数
Table 2. Material constants
E=210 GPa, υ=0.3, k=100 MPa, M=5 c1=1632.3, c2=493.0, c3=149.0, c4=45.0, c5=13.6 r1=120.5 MPa, r2=76.3 MPa, r3=89.6 MPa, r4=100.4 MPa, r5=152.5 MPa χ1 =χ2 =χ3 =χ4=χ5=5
下载: 导出CSV
-
[1] Bower A F, Johnson K L. Plastic flow and shakedown of the rail surface in repeated wheel-rail contact[J]. Wear, 1991, 144(1/2): 1-18. [2] Jiang Yan-yao, Sehitoglu H. Rolling contact stress analysis with the application of a new plasticity model[J]. Wear, 1996, 191(1/2): 35-44. [3] Mcdowell D L, Moyar G J. Effects of non-linear kinematic hardening on plastic deformation and residual stresses in rolling line contact[J]. Wear, 1991, 144(1/2): 19-37. [4] Hearle A D, Johnson K L. Cumulative plastic flow in rolling and sliding line contact[J]. ASME Journal of Applied Mechanics, 1987, 54(1): 1-7. doi: 10.1115/1.3172958 [5] Yu M, Moran B, Keer L M. A direct analysis of two-dimensional elastic-plastic rolling contact[J]. ASME Journal of Tribology, 1993, 115(2): 227-236. doi: 10.1115/1.2920996 [6] Yu M, Moran B, Keer L M. A direct analysis of three-dimensional elastic-plastic rolling contact[J]. ASME Journal of Tribology, 1995, 117(2): 234-243. doi: 10.1115/1.2831236 [7] Jiang Yan-yao, Sehitoglu H. An analytical approach to elastic-plastic stress analysis of rolling contact[J]. ASME Journal of Tribology, 1994, 116(3): 577-587. doi: 10.1115/1.2928885 [8] Jiang Yan-yao, Sehitoglu H. A model for rolling contact failure[J]. Wear, 1999, 224(1): 38-49. doi: 10.1016/S0043-1648(98)00311-1 [9] Bhargava V, Hahn G T, Rubin C A. An elastic-plastic finite element model of rolling contact[J]. ASME Journal Applied Mechanics, 1985, 52(1): 66-82. [10] Bhargava V, Hahn G T, Rubin C A. Analysis of rolling contact with kinematic hardening for rail steel properties[J]. Wear, 1988, 122(3): 267-283. doi: 10.1016/0043-1648(88)90014-2 [11] Ham G L, Hahn G T, Rubin C A, et al. Finite element analysis of the influence of kinematic hardening in two-dimensional, repeated, rolling-sliding contact[J]. Tribology Transactions, 1989, 32(3): 311-316. doi: 10.1080/10402008908981894 [12] Kulkarni S M, Rubin C A, Hahn G T. Elasto-plastic coupled temperature-displacement finite element analysis of two-dimensional rolling-sliding contact with a translating heat source[J]. ASME Journal of Tribology, 1991, 113(1): 93-101. doi: 10.1115/1.2920609 [13] Howell M, Hahn G T, Rubin C A, et al. Finite element analysis of rolling contact for non-linear kinematic hardening bearing steel [J]. ASME Journal of Tribology, 1995, 117(4): 729-736. doi: 10.1115/1.2831544 [14] Jiang Yan-yao, Chang Jian-jun, Xu Bi-qiang. Elastic-plastic finite element stress analysis of two-dimensional rolling contact[A]// Hydraulic Failure Analysis: Fluids, Components and System Effects[C]. West Conshohocken: American Society for Testing and Materials, 2001. [15] Jiang Yan-yao, Xu Bi-qiang, Sehitoglu H. Three-dimensional elastic-plastic stress analysis of rolling contact[J]. ASME Journal of Tribology, 2002, 124(4): 699-708. doi: 10.1115/1.1491978 [16] Xu Bi-qiang, Jiang Yan-yao. Elastic-plastic finite element analysis of partial slip rolling contact[J]. ASME Journal of Tribology, 2002, 124(1): 20-26. doi: 10.1115/1.1395630 [17] Dang Van K, Maitournam M H. Steady-state flow in classical elastoplasticity: applications to repeated rolling and sliding contact[J]. Journal of the Mechanics and Physics of Solids, 1993, 41(11): 1 691-1 710. doi: 10.1016/0022-5096(93)90027-D [18] ShimaM, OkadaK. Measurements of subsurface plasticflow in rolling contact[J]. Journal of Japan Society of Lubrication Engineers, 1981, 26(1): 75-80. [19] Hahn G T, Huang Q. Rolling contact deformation of 1100 aluminum disks[J]. Metallurgical Transactions A, 1986, 17(7): 1 561-1 572. [20] Krause H, Senuma T. Investigation into the influence of dynamic forces on the tribological behavior of bodies in rolling/sliding contact with particular regard to surface corrugations[J]. ASME Journal of Lubrication Technology, 1981, 103(1): 26-34. doi: 10.1115/1.3251610 [21] Johnson K L. Contact Mechanics[M]. Cambridge: Cambridge University Press, 1985. [22] Jiang Yan-yao, Sehitoglu H. Modeling of cyclic ratchetting plasticity: partⅠ-development of constitutive equations[J]. ASME Journal of Applied Mechanics, 1996, 63(4): 720-725. [23] Jiang Yan-yao, Sehitoglu H. Modeling of cyclic ratchetting plasticity: partⅡ-implement of the new model and comparison of theory with experiments[J]. ASME Journal of Applied Mechanics, 1996, 63(4): 726-733. [24] Ohno N, Wang J D. Kinematic hardening rules for simulation of ratchetting behavior[J]. European Journal of Mechanics A/Solids, 1994, 13(4): 519-531. [25] Hibbitt D, Karlsson B, Sorensen P. ABAQUS/Standard User's Manual[M]. Pawtucket RI: ABAQUS, Inc., 2003. [26] Jin Xue-song, Wen Ze-feng, Wang Kai-yu, et al. Effect of rail corrugation formation on dynamical behaviour of rail vehicle and track[J]. Journal of Sound and Vibration, 2006, 293(3/4/5): 830-855. [27] Jiang Yan-yao, Sehitoglu H. Cyclic ratchetting of 1070 steel under multiaxial stress state[J]. International Journal of Plasticity, 1994, 10(5): 579-608. [28] Jiang Yan-yao, Sehitoglu H. Multiaxial cyclic ratchetting under multiple step loading[J]. International Journal of Plasticity, 1994, 10(8): 849-870. [29] 金学松, 温泽峰, 王开云. 钢轨磨耗型波磨计算模型与数值方法[J]. 交通运输工程学报, 2005, 5(2): 12-18. http://transport.chd.edu.cn/article/id/200502004Jin Xue-song, Wen Ze-feng, Wang Kai-yun. Theoretical model and numerical method of rail corrugation[J]. Journal of Traffic and Transportation Engineering, 2005, 5(2): 12-18. (in Chinese) http://transport.chd.edu.cn/article/id/200502004 [30] Jiang Yan-yao. A fatigue criterion for general multiaxial loading[J]. Fatigue & Fracture of Engineering Materials & Structures, 2000, 23(1): 19-32. -
点击查看大图
图(13) / 表(2)
计量
- 文章访问数: 200
- HTML全文浏览量: 69
- PDF下载量: 246
- 被引次数: 0
