Optimization algorithm of multi-truck multi-category goods loading based on benchmark methods
Article Text (Baidu Translation)
-
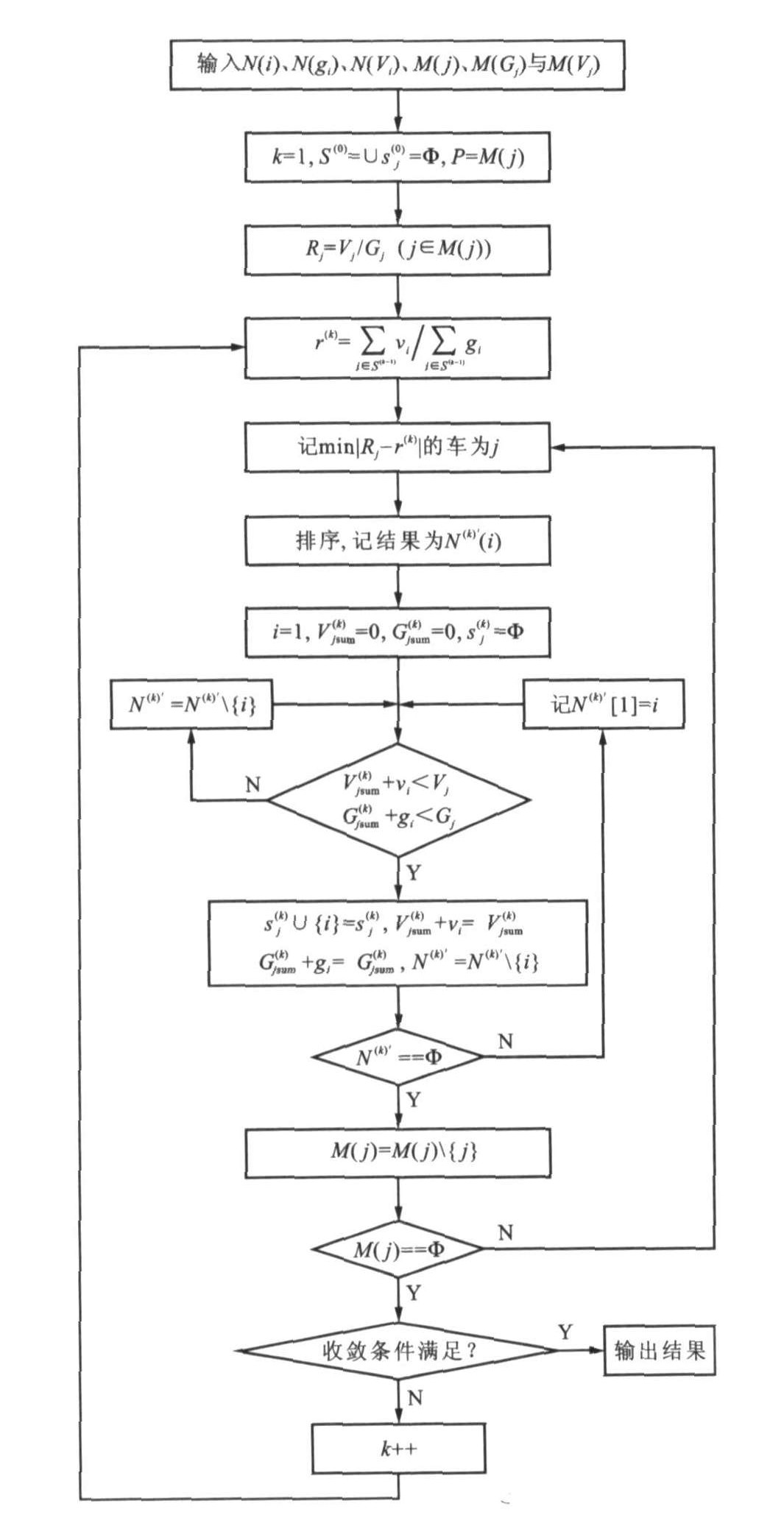
摘要: 根据货物与货车体积质量比差异情况, 结合组合理论, 设计基于不同标杆的优化算法, 充分利用车辆的载质量与容积, 以提高装载效率。对于轻质货物, 以货车的载质量为标杆, 在充分利用货车容积的同时, 尽可能地提高货车的载质量利用率; 对于重质货物, 以货车的容积为标杆; 匀质货物的体积和质量相对货车都比较均衡, 以货车的体积质量比为标杆, 对货车的容积和载质量利用率同时优化。数值仿真结果表明标杆算法的效率普遍优于其他算法, 标杆算法下体积利用率曲线和载质量利用率曲线及其趋势线比较平稳, 算法的稳定性强, 适合大规模多车多品种货物的装载。Abstract: According to the ratio difference of volume and mass between trucks and goods, an optimization algorithm was designed by combinatorial theory on the basis of different benchmarks in order to make full use of the loading mass and volume of trucks. Light goods were marked with trucks' loading mass so as to promote the mass utilization rate of trucks, on the premise that the loading volume of trucks was fully used; heavy goods were marked with trucks' loading volume; even goods were marked with the ratio of volume and mass to optimize the trucks' loading volume and mass spontaneously, as both the dimension and load of goods were even relative to trucks. The comparison result between benchmark algorithm and other optimization algorithms shows that the efficiency of benchmark algorithm is prior to other algorithms, it has strong robustness, and especially fits to large-scale multi-truck multi-category goods loading.
-
Key words:
- logistics engineering /
- goods loading /
- benchmark method /
- optimization algorithm /
- multi-truck /
- multi-category
-
表 1 算法对比
Table 1. Comparison of algorithms

-
[1] Dowsland K A, Dowsland WB. Packing problems[J]. European Journal of Operational Research, 1992, 56(1): 2-14. doi: 10.1016/0377-2217(92)90288-K [2] Martello S, Vigo D. Exact solution of the two-dimensional finite bon packing problem[J]. Management Science, 1998, 44(3): 388-399. doi: 10.1287/mnsc.44.3.388 [3] Bhattacharya S, Roy R. An exact depth-first algorithmfor the pallet loading problem[J]. European Journal of Operational Research, 1998, 110(3): 610-625. doi: 10.1016/S0377-2217(97)00272-5 [4] Scheithauer G, Sommerweib U. 4-block heuristic for the rectangle packing problem[J]. European Journal of Operational Research, 1998, 108(3): 509-526. doi: 10.1016/S0377-2217(96)00359-1 [5] Berghammer R, Reuter F. Alinear approxi mation algorithm for bin packing with absolute approxi mation factor[J]. Science of Computer Programming, 2003, 48(1): 67-80. doi: 10.1016/S0167-6423(03)00011-X [6] Armstrong R D, Jin Zhi-ying. Strongly polynomial simplex algorithm for bipartite vertex packing[J]. Discrete Applied Mathematics, 1996, 64(2): 97-103. doi: 10.1016/0166-218X(94)00122-T [7] 孙焰, 李致中. 求双目标配装的多项式近似算法[J]. 长沙铁道学院学报, 1997, 15(2): 33-39. https://www.cnki.com.cn/Article/CJFDTOTAL-CSTD199702006.htmSun Yan, Li Zhi-zhong. The polynomial algorithms for the allocation problem with two aims[J]. Journal of Changsha Rail way University, 1997, 15(2): 33-39. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-CSTD199702006.htm [8] Kenyon C, Remila E. A near-optimal solution to a two-dimensional cutting stock problem[J]. Mathematics of Operations Research, 2000, 25(4): 645-656. doi: 10.1287/moor.25.4.645.12118 [9] Shachnai H, Tamir T. Polynomial ti me approxi mation schemes for class-constrained packing problems[J]. Journal of Scheduling, 2001, 4(6): 312-338. [10] Lodi A, Martello S, Vigo D. Recent advances on two-dimensional bin packing problems[J]. Discrete Applied Mathematics, 2002, 123(1/3): 379-396. [11] 卜雷, 尹传忠, 蒲云. 优化普零货物拼箱配装的遗传算法[J]. 交通运输工程学报, 2004, 4(4): 84-87. http://transport.chd.edu.cn/article/id/200404021Bu Lei, Yin Chuan-zhong, Pu Yun. Genetic algorithm for optimal arrangement of general piece goods[J]. Journal of Traffic and Transportation Engineering, 2004, 4(4): 84-87. (in Chinese) http://transport.chd.edu.cn/article/id/200404021 [12] Chen Ping, Fu Zhao-hui, Li m A, et al. The two-dimensional packing problemfor irregular objects[J]. International Journal on Artificial Intelligence Tools, 2004, 13(3): 429-448. doi: 10.1142/S0218213004001624 [13] Coff man E G, Garey MR, Johnson D S. Approxi mation Al-gorithms for NP-Hard Problems[M]. Boston: PWS Publishing Company, 1997. [14] Kang J, Park S. Algorithms for the variable sized bin packing problem[J]. European Journal of Operational Research, 2003, 147(2): 365-372. [15] 徐天亮, 刘小群. 多品种货物配装的优化方法[J]. 华中科技大学学报: 自然科学版, 2003, 31(9): 15-17. https://www.cnki.com.cn/Article/CJFDTOTAL-HZLG200309005.htmXu Tian-liang, Liu Xiao-qun. Optimization for the loading of multi-category goods[J]. Journal of Huazhong University of Science and Technology: Nature Science Edition, 2003, 31(9): 15-17. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-HZLG200309005.htm [16] 刘小群, 马士华, 徐天亮. 装载能力有限下多品种货物配装的容重比平衡法[J]. 工业工程与管理, 2004, 9(3): 62-66. https://www.cnki.com.cn/Article/CJFDTOTAL-GYGC200403014.htmLiu Xiao-qun, Ma Shi-hua, Xu Tian-liang. The cubadge-weight balance algorithm for the loading of multi-category goods under the li mited loading capacity[J]. Industrial Engineering and Management, 2004, 9(3): 62-66. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GYGC200403014.htm [17] 徐同连, 栾琨, 贾洪飞. 共同配送合并策略及其配送成本[J]. 长安大学学报: 自然科学版, 2006, 26(3): 68-71. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200603016.htmXu Tong-lian, Luan Kun, Jia Hong-fei. Consolidation strategy and distribution cost of joint distribution[J]. Journal of Chang an University: Natural Science Edition, 2006, 26(3): 68-71. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200603016.htm -





 下载:
下载: