Optimization model of network distribution planning on regional container ports
-
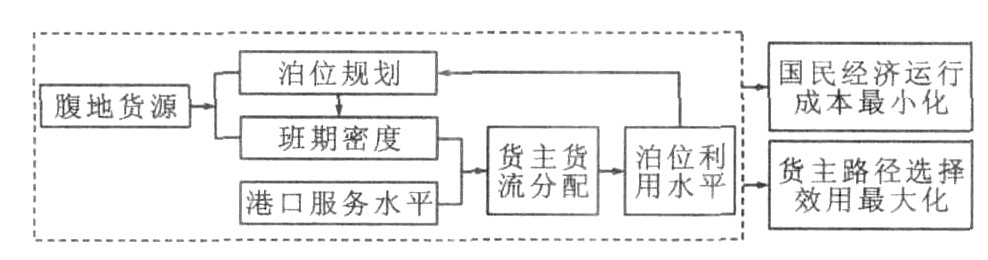
摘要: 为了避免运输网络内港口重复建设, 实现港口资源的优化配置, 综合考虑港口规划者与港口使用者之间的相互作用及决策均衡, 以Stackelberg模型为基础, 建立了区域内集装箱港口网络布局规划优化模型。为了有效地反映港口使用者个体的特殊性, 采用非集计理论, 从其自身属性出发, 分析了微观货主的选择行为。以各港口的泊位数和相应航线班期密度组成的矩阵作为染色体的一个基因串, 考虑模型约束的特殊性, 设计了模型求解的遗传算法。模拟实例计算结果表明: 在某运输网络内, 模拟得到的港口网络布局规划的近似最优解符合实际分析结果, 能表明货物运输主体的行为特性和影响因素, 利用该模型有利于实现港口网络内资源的优化配置。
-
关键词:
- 交通规划 /
- 集装箱港口网络 /
- Stackelberg模型 /
- 遗传算法 /
- 非集计理论
Abstract: In order to avoid the repetitive construction of container ports in transportation network, and realize the optimization distribution of ports, the general interaction and decision equilibrium between container port planner and user according to Stackelberg model were considered, and an optimization model of network distribution planning on regional container ports was established. In order to effectively reflect the individual properties of port users, the choice behaviors of micro-shippers were analyzed by using disaggregation theory according to their attributes. A genetic algorithm was designed to solve the model, its initial chromosome was generated by the matrix composed with the numbers of berths and corresponding voyage densities of different container ports, and the particularity of the model restrictions was considered. Example simulation result shows that the model is feasible, the approximative optimization port network planning of example computation accords with real analysis situation, the behaviors and influence factors of shippers can be reflected, and port optimization distribution can be realized. -
表 1 非集计模型估计结果
Table 1. Estimation result by using disaggregation model
选择变量 估计系数 标准差 t检验 选择枝常数项 港口1 -0.394 9 0.310 3 -1.272 8 港口2 1.585 5 0.318 7 4.974 7 港口3 0.868 1 0.336 1 2.583 1 特性变量 全程运输时间 -2.181 8 0.233 3 -9.351 5 全程运输成本 -0.009 48 0.001 19 -7.941 90 统计结果 θk为0时, 对数极大似然函数L(0)为-1 052.2, 最大似然函数为-287.97, 观测样本数为759, 命中率为83.926%, 极大似然比ρ2为0.726 3 表 2 集装箱港口网络布局规划计算结果
Table 2. Computation result of container port network planning
港口1 港口2 港口3 港口4 D1 D2 D3 D1 D2 D3 D1 D2 D3 D1 D2 D3 规划泊位 1 6 3 2 班期密度/(班·周-1) 2 2 2 16 17 9 8 8 5 3 4 7 货流分配/104 TEU O1 0.77 0.82 1.27 11.73 12.51 8.65 8.23 8.70 5.49 1.62 1.73 2.69 O2 0.10 0.11 0.16 20.90 22.26 15.21 0.52 0.50 0.29 0.10 0.11 1.05 O3 0.09 0.10 0.33 2.65 2.80 1.71 7.86 8.32 5.07 0.47 0.55 1.86 O4 3.47 3.74 3.46 2.82 3.04 1.86 1.11 1.06 0.63 0.13 0.15 0.14 O5 0.07 0.08 0.07 2.06 2.25 1.38 2.99 2.86 1.69 7.62 8.35 7.17 O6 1.10 1.24 2.04 13.00 14.64 8.63 12.47 11.85 7.62 2.56 3.23 5.32 -
[1] 王伟. 港口码头重复建设的博弈分析[J]. 水运工程, 2004, 30(4): 40-42. https://www.cnki.com.cn/Article/CJFDTOTAL-SYGC200404011.htmWang Wei. Game analysis for repeated construction of port terminals[J]. Port and Waterway Engineering, 2004, 30(4): 40-42. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SYGC200404011.htm [2] 姚荣涵, 王殿海. 居民出行分布的熵模型及其参数标定[J]. 交通运输工程学报, 2005, 5(4): 106-110. http://transport.chd.edu.cn/article/id/200504022Yao Rong-han, Wang Dian-hai. Entropy models of inhabitant trip distributions and parameters calibration[J]. Journal of Traffic and Transportation Engineering, 2005, 5(4): 106-110. (in Chinese) http://transport.chd.edu.cn/article/id/200504022 [3] 黄亚飞, 刘涛. 路网最优费率的双层规划模型及算法[J]. 交通运输工程学报, 2006, 6(4): 105-111. http://transport.chd.edu.cn/article/id/200604024Huang Ya-fei, Liu Tao. Bi-level programming model and algorithmof optimal toll rate for highway network[J]. Journal of Traffic and Transportation Engineering, 2006, 6(4): 105-111. (in Chinese) http://transport.chd.edu.cn/article/id/200604024 [4] 鲁子爱. 港口建设规模优化研究[J]. 河海大学学报: 自然科学版, 2002, 30(5): 62-66. https://www.cnki.com.cn/Article/CJFDTOTAL-HHDX200205013.htmLu Zi-ai. Port scale optimization[J]. Journal of Hohai University: Natural Sciences, 2002, 30(5): 62-66. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-HHDX200205013.htm [5] 马良成, 张培林. 单一港口发展动态规划[J]. 水运管理, 2004, 26(4): 23-25. https://www.cnki.com.cn/Article/CJFDTOTAL-SYGL200404008.htmMa Liang-cheng, Zhang Pei-lin. Dynamic planning for single port development[J]. Shipping Management, 2004, 26(4): 23-25. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SYGL200404008.htm [6] 胡明. 港口网络体系规划方法研究[D]. 大连: 大连海事大学, 2004. [7] 韩增林, 安筱鹏, 王利, 等. 中国国际集装箱运输网络的布局与优化[J]. 地理学报, 2002, 57(4): 479-488. https://www.cnki.com.cn/Article/CJFDTOTAL-DLXB200204012.htmHan Zeng-lin, An Xiao-peng, Wang Li, et al. Distribuion and optimization of container transportation network in China[J]. Journal of Geography, 2002, 57(4): 479-488. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-DLXB200204012.htm [8] 张维迎. 博弈论与信息经济学[M]. 上海: 上海人民出版社, 2002. [9] 朱晓宁. 集装箱多式联运通道规划理论与方法[M]. 北京: 中国铁道出版社, 2002. [10] 王显锋. 网络配流在拟建港口国民经济评价中的应用[D]. 大连: 大连海事大学, 2003. [11] Ben-Akiva M E, Lerman S R. Discrete Choice Analysis: Theory and Application to Travel Demand[M]. Massachusetts: MIT Press, 1997. [12] 陆化普, 殷亚峰. 规划理论的非集计分析方法及其应用[J]. 公路交通科技, 1996, 13(1): 22-28. https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK601.003.htmLu Hua-pu, Yin Ya-feng. Disaggregate analysis method and its application in planning[J]. Journal of Highway and Transportation Research and Development, 1996, 13(1): 22-28. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK601.003.htm [13] Mattew B M, Adib K. Adisaggregate analysis on port selection[J]. Transportation Research: Part E, 2004, 40(4): 317-337. [14] Torabi S A, Fatemi Ghomi S M T, Kari mi B. A hybrid genetic algorithmfor the finite horizon economiclot and delivery schedulingin supply chains[J]. European Jorunal of Operational Research, 2006, 173(1): 173-189. [15] Prabhat S, Margaret O M. A model for development of optimized feeder routes and coordinated schedules—a genetic algorithmapproach[J]. Transport Policy, 2006, 13(5): 413-425. [16] Ji Ming-jun, Tang Huan-wen, Guo Juan. A single-point mutation evolutionary programming[J]. Information Processing Letters, 2004, 90(6): 293-299. -





 下载:
下载: