预应力混凝土梁超载能力分析

赵卫国

预应力混凝土梁超载能力分析

赵卫国

1.
长安大学公路学院陕西西安 710064
2.
河北省交通厅公路局河北石家庄 050051

基金项目:

国家西部变通建设科技项日 2005 318 223 16

河北省交通科技项目 Y-030201

详细信息

作者简介:
赵卫国(1971-), 男, 河北石家庄人, 河北省交通厅公路局高级工程师, 从事桥梁加固研究

中图分类号: U448.35
计量
- 文章访问数: 379
- HTML全文浏览量: 134
- PDF下载量: 241
- 被引次数: 0
出版历程
- 收稿日期: 2007-04-13
- 刊出日期: 2007-10-25

Overload capacity analysis of prestressed reinforced concrete beam

ZHAO Wei-guo

1.
School of Highway, Chang'an University, Xi'an 710064, Shaanxi, China
2.
Bureau of Highway, Department of Communications of Hebei Province, Shijiazhuang 050051, Hebei, China

More Information

Author Bio:
Zhao Wei-guo(197l-), male, senior engineer, +86—311—83035215, highway27@sohu.com

摘要

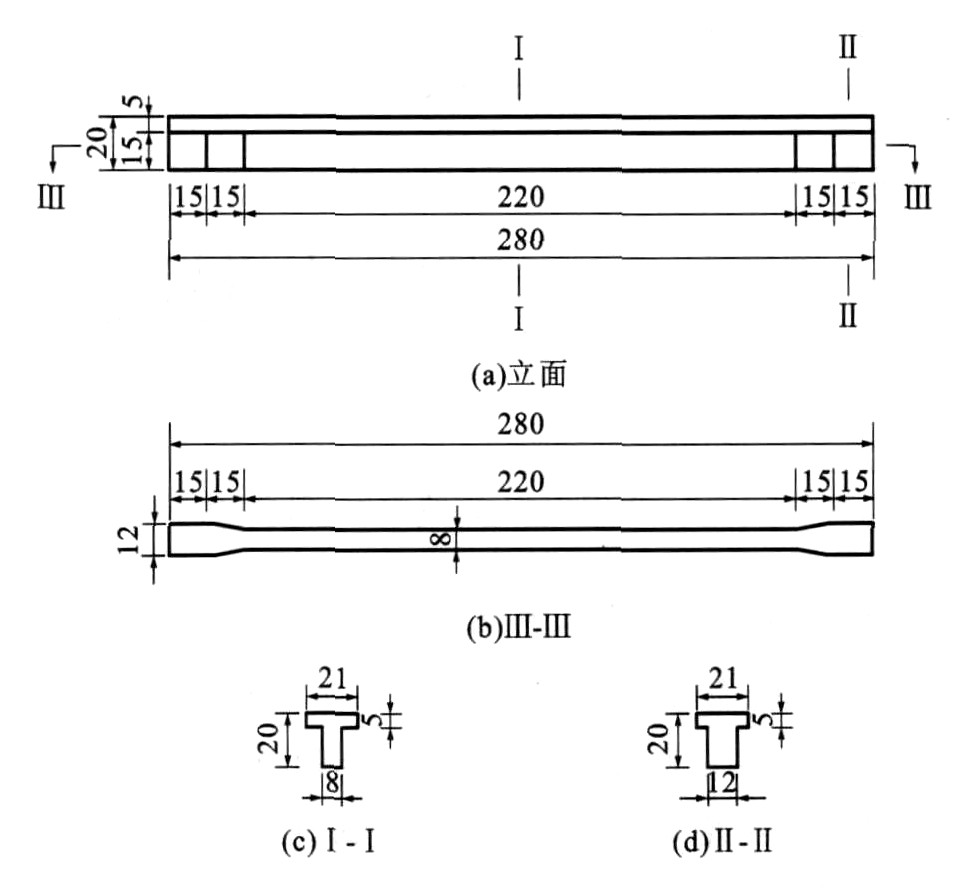
摘要: 为了验证利用有限元法分析预应力混凝土梁极限承载能力的准确性, 对T形预应力混凝土模型梁进行了极限承载力加载破坏试验, 采用ANSYS有限元程序, 建立了T梁的分离式有限元模型, 分析了模型梁从加载到破坏全过程的受力和变形。发现利用实验与有限元法得到T梁的荷载-挠度曲线与荷载-应变曲线的变化趋势一致, 并呈现良好的非线性, 但是通过荷载试验得到T梁的超载能力为9.07 kN·m, 按照有限元分析得到的超载能力为12.48 kN·m, 偏差较大, 原因是分析模型偏于理想化。分析结果表明: 利用有限元法在总体上能够有效地模拟钢筋混凝土梁受力全过程中各个量的非线性变化, 对超载能力的求解是可行的。
- 桥梁工程 /
- 预应力混凝土梁 /
- 极限承载力 /
- 有限元法
Abstract: In order to attested the analysis veracity of ultimate bearing capacity for prestressed concrete beam with finite element method, an loading experiment of ultimate bearing capacity was carried out, a detached finite element model of T beam was designed by using ANSYS finite element program, and the whole processes of T beam's stress and deformation from loading to destructing were analyzed.It is pointed that the variational trends of T beam's load-deflection curves and load-strain curves gained by experiment and the model are accordant, and the curves have good nonlinearity, but the experimental value of ultimate bearing capacity is 9.07 kN·m, the computational value is 12.48 kN·m, their error is appreciably big because of the idealization of the model.Analysis result shows that the nonlinear variations of all variables for prestressed reinforced concrete beam may are simulated by using finite element method in loading process as a whole, and the solution of ultimate bearing capacity is feasible.
- bridge engineering /
- prestressed reinforced concrete beam /
- ultimate bearing capacity /
- finite element method

HTML全文

图 1 试验梁

Figure 1. Test beam

下载: 全尺寸图片幻灯片

图 2 配筋

Figure 2. Steel distribution

下载: 全尺寸图片幻灯片

图 3 加载

Figure 3. Loading

下载: 全尺寸图片幻灯片

图 4 加载梁

Figure 4. Loading beam

下载: 全尺寸图片幻灯片

图 5 压溃梁

Figure 5. Compression failure beam

下载: 全尺寸图片幻灯片

图 6 荷载-挠度曲线

Figure 6. Load-deflection curves

下载: 全尺寸图片幻灯片

图 7 荷载-应变曲线

Figure 7. Load-strain curves

下载: 全尺寸图片幻灯片

图 8 钢绞线的荷载-应变曲线

Figure 8. Load-strain curves of prestressed wire

下载: 全尺寸图片幻灯片

表 1 挠度、应力与应变计算值

Table 1. Calculation values of deflection, stress and strain

序号	荷载/kN	应变10⁶ε	应力/MPa	挠度/mm
1	0.00	4 254.5	808.37	2.847
2	4.17	4 262.1	809.75	2.107
3	7.83	4 282.7	813.62	1.641
4	11.83	4 302.4	817.35	0.916
5	15.83	4 310.2	818.88	0.231
6	18.00	4 325.9	821.78	-0.154
7	22.00	4 349.8	826.34	-0.891
8	24.00	4 360.1	828.52	-1.259
9	25.33	4 380.3	832.22	-1.812
10	25.83	4 387.8	837.57	-1.899
11	26.83	4 397.5	838.63	-2.435
12	27.83	4 408.6	839.09	-2.778
13	29.83	4 427.3	843.91	-3.849
14	32.00	4 452.1	847.03	-5.157
15	33.00	4 463.5	851.93	-5.757
16	34.67	4 488.3	856.88	-6.678
17	37.67	4 541.8	862.80	-8.688
18	39.83	4 582.9	870.59	-10.317
19	42.00	4 652.6	883.85	-12.213
20	45.00	4 720.3	896.79	-15.615

下载: 导出CSV

表 2 试验数据

Table 2. Test data

下载: 导出CSV

参考文献(8)

[1]	夏荣泉. 预应力混凝土梁极限承载力非线性有限元研究[D]. 西安: 长安大学, 2005.
[2]	谢素超, 田红旗, 姚松. 板梁偏心连接结构有限元分析[J]. 交通运输工程学报, 2006, 6(4): 5-9. https://www.cnki.com.cn/Article/CJFDTOTAL-JYGC200604001.htm Xie Su-chao, Tian Hong-qi, Yao Song. Finite element analysis of composite structure of eccentric beam and plate[J]. Journal of Traffic and Transportation Engineering, 2006, 6(4): 5-9. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JYGC200604001.htm
[3]	郑文忠, 王英, 计学闰, 等. 预应力混凝土结构极限承载力分析[J]. 工业建筑, 1999, 29(3): 15-18. https://www.cnki.com.cn/Article/CJFDTOTAL-GYJZ903.003.htm Zheng Wen-zhong, Wang Ying, Ji Xue-run, et al. The analysis of prestressed concrete structure on bearing capacity[J]. Industrial Construction, 1999, 29(3): 15-18. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GYJZ903.003.htm
[4]	赵曼, 王新敏, 高静. 预应力混凝土结构有限元数值分析[J]. 石家庄铁道学院学报, 2004, 17(1): 84-88. https://www.cnki.com.cn/Article/CJFDTOTAL-SJZT200401020.htm Zhao Man, Wang Xin-min, Gao Jing. FEMnumerical analysis of prestressed concrete structure[J]. Journal of Shijiazhuang Railway Institute, 2004, 17(1): 84-88. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SJZT200401020.htm
[5]	赵均海, 翟慧娟, 计琳, 等. 钢筋混凝土特种楼梯非线性有限元分析[J]. 长安大学学报: 自然科学版, 2006, 26(6): 52-54. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200606011.htm Zhao Jun-hai, Zhai Hui-juan, Ji Lin, et al. Nonlinear finite element analysis on special reinforced concrete stair[J]. Journal of Chang'an University: Natural Science Edition, 2006, 26(6): 52-54. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200606011.htm
[6]	汤文锋, 王毅红, 史耀华. 新型钢管混凝土节点的非线性有限元分析[J]. 长安大学学报: 自然科学版, 2004, 24(5): 60-63. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200405015.htm Tang Wen-feng, Wang Yi-hong, Shi Yao-hua. Nonlinear finite element analysis of new joint in concrete filled steel tubular[J]. Journal of Chang an University: Natural Science Edition, 2004, 24(5): 60-63. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200405015.htm
[7]	刘小燕, 颜东煌, 张峰, 等. 预应力高强混凝土梁极限承载力分析[J]. 中国公路学报, 2006, 19(1): 58-61. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200601011.htm Liu Xiao-yan, Yan Dong-huang, Zhang Feng, et al. Ultimate load analysis of prestressed high-strength concrete beam[J]. China Journal of Highway and Transport, 2006, 19(1): 58-61. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200601011.htm
[8]	钟铭, 王海龙, 王海良. 高强混凝土梁在疲劳荷载作用下的裂缝宽度计算[J]. 中国公路学报, 2005, 18(4): 48-53. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200504009.htm Zhong Ming, Wang Hai-long, Wang Hai-liang. Calculation of crack width of high-strength concrete beams under fatigue loading[J]. China Journal of Highway and Transport, 2005, 18(4): 48-53. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200504009.htm