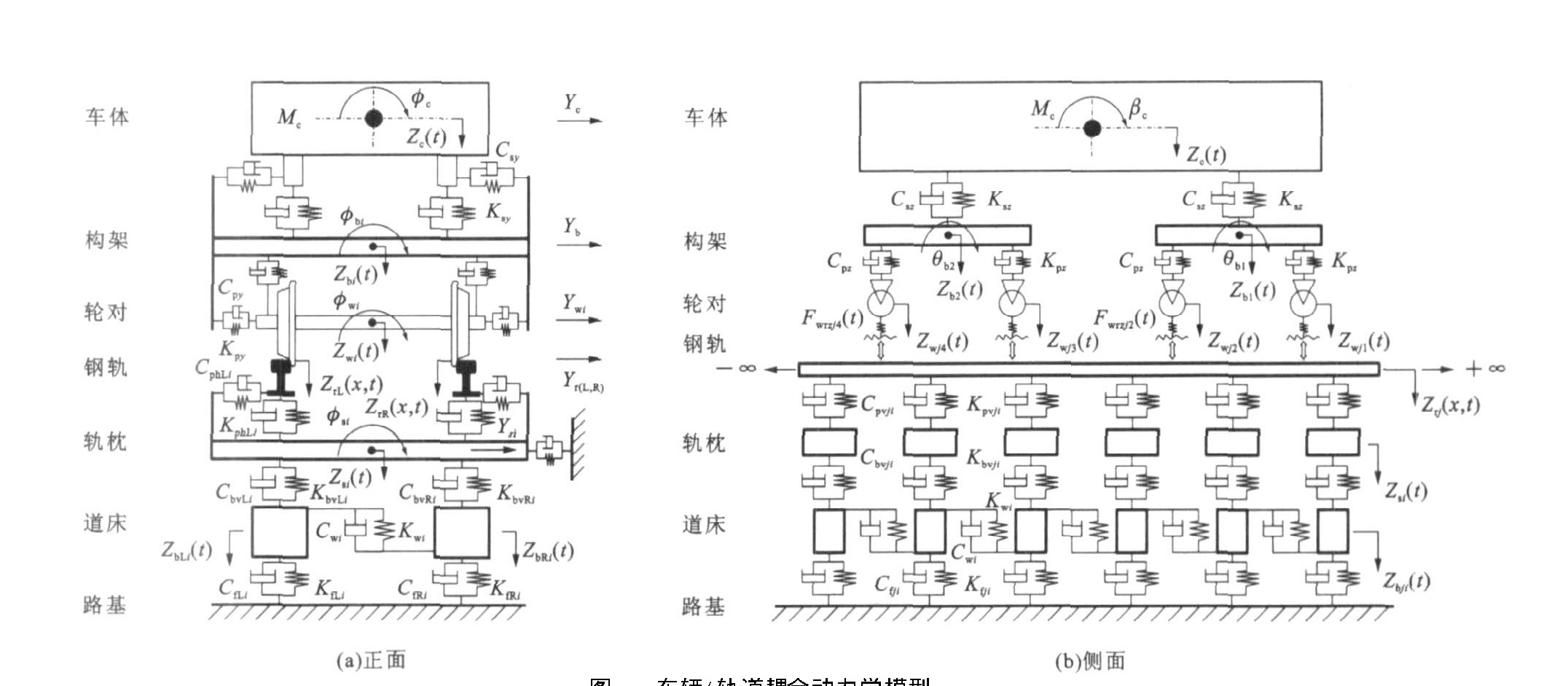
-
摘要: 采用35自由度的多刚体车辆系统与三层弹性离散点支撑轨道模型, 建立了基于Timosh-enko梁模型的车辆/轨道耦合动力学模型, 应用新型显式积分法求解其运动特性。考虑钢轨横向、垂向和扭转运动对轮轨滚动接触几何关系的影响, 分别由Hertz法向接触理论和沈氏蠕滑理论计算了轮轨法向力和轮轨滚动接触蠕滑力。假设轨枕垂向支撑高度沿纵向非均匀分布来模拟轨枕支撑硬点, 基于移动轨下支撑模型, 分析了不同轨枕支撑硬点个数和高度对系统动力响应的影响。分析结果表明, 轨枕支撑硬点对轨枕的动力响应影响显著。当硬点高度为1.0 mm时, 最大钢轨/轨枕作用力约为正常状态下的2倍, 最大钢轨/轨枕拉力约为正常状态的10倍, 这将加速轨枕、轨下垫层及钢轨扣件状况的恶化。而支撑硬点个数对系统动力响应的影响很小。Abstract: a vehicle-track coupling dynamics model was established based on Timoshenko beam model, vehicle was modeled as a 35 DOF multi-body system, track was modeled as a 3-layer discrete elastic support model, and the motion properties of vehicle/track coupling system were solved by using new-explicit-integration method.The effect of rail vertical, torsional and lateral motions on wheel/rail rolling contact geometry relationship was taken into account, wheel/rail normal forces were calculated by using Hertzian contact theory, and wheel/rail creep forces in rolling contact were computed by using Shen nonlinear creep theory.The support hard spots of sleepers with non-uniform support heights along track were simulated, and the effect of their number and heights on the model was analyzed by using moving rail-supported model.Numerical simulation result indicates that the number of hard spots greatly affects the dynamic responses of track system rather than vehicle system, especially railway sleeper.As the height is 1.0 mm, the maximum rail/sleeper force and tension are almost 2 and 10 times larger than normal values respectively.However, the dynamic responses of vehicle-track coupling system aren't sensitive to the number of hard spots.
-
Key words:
- railway engineering /
- vehicle /
- track /
- railway sleeper /
- hard spot /
- coupling dynamics /
- dynamic response
-
[1] Lundqvist A, Dahlberg T. Numerical si mulation of train-track dynamics[R]. Linkoeping: Linkoeping University, 2005. [2] Lei Xiao-yan, Mao Li-jun. Dynamic response analysis of ve-hicle and track coupled system on track transition of conven-tional high speed rail way[J]. Journal of Sound and Vibra-tion, 2004, 271(3/4/5): 1 133-1 146. [3] Dong R G. Vertical dynamics of rail way vehicle-tracksystem[D]. Concordia: Concordia University, 1994. [4] Nielsen J C O, Igeland A. Vertical dynamic interaction be-tween train and track[J]. Journal of Sound and Vibration, 1995, 187(5): 825-839. doi: 10.1006/jsvi.1995.0566 [5] Zhai Wan-ming, Sun Xiang. Adetailed model for investigating vertical interactions betweenrailway vehicle andtrack[J]. VehicleSystem Dymanics, 1994, 23(S): 603-615. [6] Zhai Wan-ming, Cai Cheng-biao, Guo Shi-zhang. Couplingmodel of vertical and lateral vehicle/track interactions[J]. Vehicle System Dymanics, 1996, 26(1): 61-79. doi: 10.1080/00423119608969302 [7] Knothe K L, Grassie S L. Modeling of rail way track and ve-hicle/trackinteraction at highfrequencies[J]. Vehicle SystemDynamics, 1993, 22(3/4): 209-262. [8] Jin Xue-song, Wu Ping-bo, Wen Ze-feng. Effects of struc-ture elastic deformations of wheelset andtrack on creepforcesof wheel/rail in rolling contact[J]. Wear, 2002, 253(1/2): 247-256. [9] 肖新标, 金学松, 温泽峰. 钢轨扣件失效对列车动态脱轨的影响[J]. 交通运输工程学报, 2006, 6(1): 10-15. doi: 10.3321/j.issn:1671-1637.2006.01.002Xiao Xin-biao, Jin Xue-song, Wen Ze-feng. Influence of railfastener failure on vehicle dynamic derail ment[J]. Journal ofTraffic and Transportation Engineering, 2006, 6(1): 10-15. (in Chinese) doi: 10.3321/j.issn:1671-1637.2006.01.002 [10] Jin Xue-song, Wen Ze-feng, Wang Kai-yun. Effect of trackirregularities on initiation and evolution of rail corrug-ation[J]. Journal of Sound and Vibration, 2005, 285(1/2): 121-148. [11] Xiao Xin-biao, Jin Xue-song, Wen Ze-feng. Effect of disabledfastening systems and ballast on vehicle derail ment[J]. Jour-nal of Acoustic and Vibration, 2007, 129(2): 217-229. doi: 10.1115/1.2424978 -
下载:
点击查看大图
图(12)
计量
- 文章访问数: 299
- HTML全文浏览量: 116
- PDF下载量: 415
- 被引次数: 0
