Control method of time on wing for civil aeroengine
-
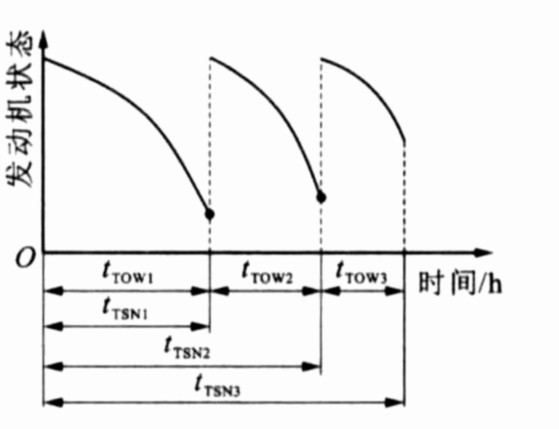
摘要: 为了通过视情维修策略控制民航发动机在翼时间, 分析了发动机维修与在翼时间的特点, 建立了反映在翼时间与状态监测变量和故障强度之间关系的比例强度模型, 确立了性能衰退下的在翼时间控制限与单位时间总成本期望值最小时的最优预防性维修间隔, 并进行了CF6-80C2A5型发动机运营数据的实例验证。结果表明: 故障强度小, 则在翼时间控制限低; 预防性维修与事后维修成本率会影响最优预防性维修间隔; 完备的发动机拆换与在翼历史记录、维修成本、适航与制造商要求、寿命件限制等数据是提高在翼时间控制准确性的关键。Abstract: In order to apply condition based maintenance (CBM) to control civil aeroengine time on wing (TOW), the features of aeroengine CBM and TOW were discussed, and the proportional intensity model was established to describe the relation among TOW, condition monitoring variables and failure intensity. TOW control limit due to performance deterioration and optimal preventive maintenance (PM) interval under minimizing expected total maintenance cost per unit time were implemented. The operation data of a CF6-80C2A5 engine were studied. It is pointed that the less failure intensity is, the lower TOW control limit is; moreover, the cost rate between PM and corrective maintenance can affect optimal PM interval; sufficient engine operation data are the keys of accurate TOW control, such as engine removal and on wing history records, maintenance cost, requirements of airworthiness and manufacturer, and time limits of life limited parts etc.
-
Key words:
- aeroengine /
- condition based maintenance /
- proportional intensity model /
- time on wing
-
表 1 发动机拆换历史记录
Table 1. Engine removal history records
序号 tTOW/h δ X1/℃ X2/% X3/% X4/Pa X5/% X6/% X7/℃ X8/℃ 149 7 095 1 9.122 3.372 1.783 47 492.760 0.314 0.458 9.278 32.746 243 7 801 1 24.369 4.747 2.092 60 193.350 0.028 0.518 -0.162 29.956 594 7 820 0 4.870 2.238 1.479 -29 641.605 0.163 0.871 1.509 30.447 271 3 282 0 22.688 7.579 2.123 -7 384.545 0.645 0.992 -10.376 26.997 表 2 协变量之间的相关性
Table 2. Correlation among covariates
协变量 X1 X2 X3 X4 X5 X6 X7 X2 0.628 X3 0.164 0.272 X4 -0.069 -0.190 0.354 X5 0.248 0.235 0.223 -0.145 X6 0.082 -0.058 -0.014 0.153 -0.026 X7 -0.733 -0.046 -0.040 0.232 -0.146 0.034 X8 -0.732 -0.465 -0.040 0.232 -0.145 0.037 1.000 表 3 发动机拆换后在翼时间统计
Table 3. tTOWstatistics after engine removals
序号 拆换时tTOW/h 发动机拆换原因 271 7 721 性能衰退 272 4 656 LLP到寿 275 7 947 LLP到寿 281 6 198 AD指令 582 7 335 性能衰退 601 5 988 性能衰退 608 9 882 性能衰退 -
[1] 梁剑. 基于成本优化的民用航空发动机视情维修决策研究[D]. 南京: 南京航空航天大学, 2005. [2] 左洪福, 张海军, 戎翔. 基于比例风险模型的航空发动机视情维修决策[J]. 航空动力学报, 2006, 21(4): 716-721. doi: 10.3969/j.issn.1000-8055.2006.04.018Zuo Hong-fu, Zhang Hai-jun, Rong Xiang. Condition based aero-engine maintenance decision method using proportional hazards model[J]. Journal of Aerospace Power, 2006, 21(4): 716-721. (in Chinese) doi: 10.3969/j.issn.1000-8055.2006.04.018 [3] Rong Xiang, Zuo Hong-fu, Zhang Hai-jun, et al. Research on an integrated model-based CBM decision support system for aeroengine[C]//Wang Wen-bin, Jia Xi-sheng, Huang Hong-zhong. Proceeding of the First International Conference on Maintenance Engineering. Beijing: Science Press, 2006: 216-221. [4] 富涛, 许春生. 在翼航空发动机剩余寿命预测[J]. 中国民航飞行学院学报, 2006, 17(3): 18-21. https://www.cnki.com.cn/Article/CJFDTOTAL-MHFX200603004.htmFu Tao, Xu Chun-sheng. Residual life prediction for aero-engine on wing[J]. Journal of Civil Aviation Flight University of China, 2006, 17(3): 18-21. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-MHFX200603004.htm [5] 李建国, 叶新农, 徐可君. 涡扇发动机寿命管理研究[J]. 海军航空工程学院学报, 2005, 20(4): 468-472.Li Jian-guo, Ye Xin-nong, Xu Ke-jun. Life management research of military turbofan engine[J]. Journal of Naval Aero-nautical Engineering Institute, 2005, 20(4): 468-472. (in Chinese) [6] Vittal S, Hajela P, Joshi A. Reviewof approaches to gas tur-bine life management[C]//AIAA. Proceeding of 10 th AIAA/ISSMO Multidisciplinary Analysis and Optimization Conference. New York: AI AA, 2004: 1-11. [7] Jiang S T, Landers T L, Rhoads T R. Assessment of repairable-systemreliability using proportional intensity models: a review[J]. IEEE Transactions on Reliability, 2006, 55(2): 328-336. doi: 10.1109/TR.2006.874938 [8] Jiang S T, Landers T L, Rhoads T R. Semi-parametric proportional intensity models robustness for right-censored recurrent failure data[J]. Reliability Engineering and System Safety, 2005, 90(1): 91-98. doi: 10.1016/j.ress.2004.11.017 [9] Percy D F, Kobbacy K A H, Ascher HE. Using proportional-intensities models to schedule preventive-maintenance intervals[J]. I MA Journal of Mathematics Applied in Business and Industry, 1998, 9(3): 289-302. [10] Naeem M, Singh R, Probert D. Consequences of aero-engine deteriorations for military aircraft[J]. Applied Energy, 2001, 70(2): 103-133. doi: 10.1016/S0306-2619(01)00028-9 [11] Wang Wen-bin, Zhang Wen-juan. A model to predict the residual life of aircraft engines based upon oil analysis data[J]. Naval Research Logistics, 2005, 52(3): 276-284. [12] Jaw L C. Recent advancementsin aircraft engine health man-agement(EHM)technologies and recommendations for the next step[C]//ASME. Proceeding of Turbo Expo2005: 50th ASMEInternational Gas Turbine & Aeroengine Techni-cal Congress. New York: ASME, 2005: 1-13. [13] Weckman G R, Shell R L, Marvel J H. Modeling the reliability of repairable systems in the aviationindustry[J]. Computers and Industrial Engineering, 2001, 40(1/2): 51-63. [14] Guo Huai-rui, Liao Hai-tao, Zhao Wen-biao, et al. A new stochastic model for systems under general repairs[J]. IEEE Transactions on Reliability, 2007, 56(1): 182-188. -





 下载:
下载: