Path optimization model and algorithm of multimodal transport for long and bulky cargo
-
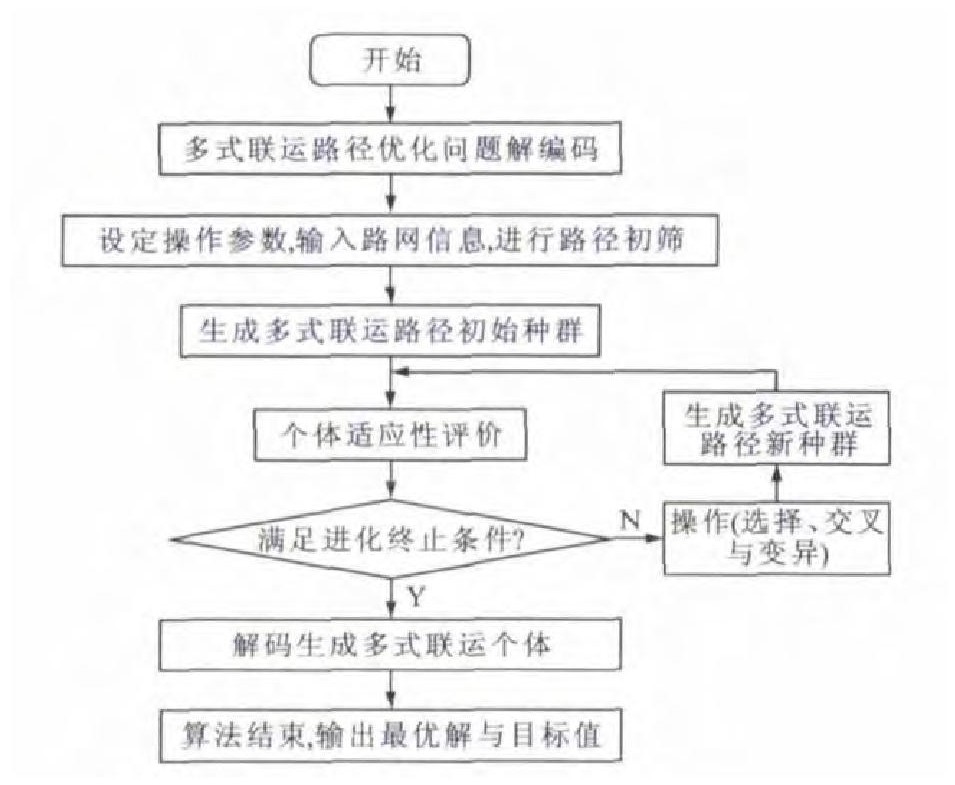
摘要: 基于可行性与合理性的角度, 分析了长大货物多式联运路径优化的影响因素。以最小运输时间、里程与费用为目标函数, 以线路限界、桥梁承载能力、起重设备的起重能力为约束条件, 建立了长大货物多式联运路径优化原始模型。考虑了约束条件的改造性特征, 将原始模型扩展优化, 设计了二维序列编码策略, 运用遗传算法求解扩展模型。计算结果表明: 运用提出的优化模型与遗传算法, 最优运输时间、里程和费用分别为12.5d、1 116km、58.18万元, 运用提出的优化模型与模拟退火算法, 最优运输时间、里程和费用分别为15.5d、1 131km、67.74万元; 运用单一的铁路运输方式与遗传算法, 最优运输时间、里程和费用分别为12.7d、1 152km、56.50万元。与其他2种情况比较, 提出的优化模型与遗传算法的综合优化程度分别提高52.22%与8.95%, 可见, 模型可行, 算法有效。Abstract: Based on the view of feasibility and rationality, the path optimization influence factors of multimodal transport for long and bulky cargo were analyzed. The minimum transport time, mileage and cost were taken as objective functions, the line boundary, bridge bearing capacity and lifting equipment capacity were taken as constraint conditions, and the original path optimization model of multimodal transport for long and bulky cargo was set up. By considering the transformation characteristics of constraint conditions, the original model was extended and optimized, two-dimensional sequence coding strategy was designed, and the extensional model was solved by using genetic algorithm. Calculation result shows that by using the extensional model and genetic algorithm, the optimal transport time, mileage and cost are 12.5 d, 1 116 km and 581 800 yuan respectively. By using the extensional model and simulated annealing algorithm, the optimal transport time, mileage and cost are 15.5 d, 1 131 km and 677 400 yuan respectively. By using single-railway transport mode and genetic algorithm, the optimal transport time, mileage and cost are 12.7 d, 1 152 km and 565 000 yuan respectivelg. By using the extensional model and genetic algorithm, the integrated optimization degree rises by 52.22% and 8.95% compared with the other 2 conditions. Obviously, the extensional model is feasible, and genetic algorithm is effective.
-
[1] 蔡建华, 张剑飞. 超限运输的成因及治理对策[J]. 中国公路学报, 2006, 19 (3): 100-105. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200603018.htmCAI Jian-hua, ZHANG Jian-fei. Cause of overweight transportation and administration countermeasure[J]. China Journal of Highway and Transport, 2006, 19 (3): 100-105. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200603018.htm [2] 尹晔飞. 超限超重货物运输方案研究[D]. 长沙: 中南大学, 2009.YIN Ye-fei. Research on project of the special train of out-ofgauge and overweight goods transportation[D]. Changsha: Central South University, 2009. (in Chinese). [3] 赵晓光, 何建中, 宋德星. 国内水路运输管理条例释义[M]. 北京: 人民交通出版社, 2012.ZHAO Xiao-guang, HE Jian-zhong, SONG De-xing. China Waterway Transportation Management Regulation Explanation[M]. Beijing: China Communications Press, 2012. (in Chinese). [4] 雷定猷. 货物装运优化理论与应用研究[D]. 长沙: 中南大学, 2005.LEI Ding-you. On optimizing theory and application of goods loading and carrying[D]. Changsha: Central South University, 2005. (in Chinese). [5] 吴强. 大件货物加氢反应器运输方案设计与实施[D]. 大连: 大连海事大学, 2006.WU Qiang. The design and implementation of hydrogenation reactor transport scheme[D]. Dalian: Dalian Maritime University, 2006. (in Chinese). [6] RAY J J. A web-based spatial decision support system optimizes routes for oversize/overweight vehicles in delaware[J]. Decision Support Systems, 2007, 43 (4): 1171-1185. doi: 10.1016/j.dss.2005.07.007 [7] 乔国会, 张东杰, 聂钦中, 等. 大件货物公路运输线路选择方法研究[J]. 物流技术, 2010, 29 (7): 55-57. https://www.cnki.com.cn/Article/CJFDTOTAL-WLJS201007019.htmQIAO Guo-hui, ZHANG Dong-jie, NIE Qin-zhong, et al. Research on the route selection of outsize cargo highway transportation[J]. Logistics Technology, 2010, 29 (7): 55-57. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-WLJS201007019.htm [8] 罗建. 公路大件运输线路选择方案及模型研究[J]. 西华大学学报: 自然科学版, 2013, 32 (4): 71-76. https://www.cnki.com.cn/Article/CJFDTOTAL-SCGX201304019.htmLUO Jian. Study on the route selection scheme and model about heavy-cargo highway transportation[J]. Journal of Xihua University: Natural Science Edition, 2013, 32 (4): 71-76. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-SCGX201304019.htm [9] 汤波, 雷定猷, 张英贵. 铁路超限超重货物运输径路综合优化模型与算法[J]. 计算机应用研究, 2012, 29 (8): 2876-2881. https://www.cnki.com.cn/Article/CJFDTOTAL-JSYJ201208021.htmTANG Bo, LEI Ding-you, ZHANG Ying-gui. Integrated optimizing model and algorithms of transportation route in out-of-gauge and overweight freights of railway[J]. Application Research of Computers, 2012, 29 (8): 2876-2881. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JSYJ201208021.htm [10] 汤波. 铁路超限超重货物运输优化研究[D]. 长沙: 中南大学, 2012.TANG Bo. Optimization research on out-of-gauge and overweight goods transportation by railway[D]. Changsha: Central South University, 2012. (in Chinese). [11] LOZANO A, STORCHI G. Shortest viable path algorithm in multimodal networks[J]. Transportation Research Part A: Policy and Practice, 2001, 35 (3): 225-241. doi: 10.1016/S0965-8564(99)00056-7 [12] LAM S K, SRIKANTHAN T. Accelerating the K-shortest paths computation in multimodal transportation networks[C]∥IEEE. The IEEE 5th International Conference on Intelligent Transportation System. Singapore: IEEE, 2002: 491-495. [13] BOUSSEDJRA M, BLOCH C, MOUDNI A E. An exact method to find the intermodal shortest path[C]∥IEEE. 2004IEEE International Conference on Networking, Sensing and Control. Taipei: IEEE, 2004: 1075-1080. [14] 王涛, 王刚. 一种多式联运网络运输方式的组合优化模式[J]. 中国工程科学, 2005, 7 (10): 46-50. https://www.cnki.com.cn/Article/CJFDTOTAL-GCKX200510009.htmWANG Tao, WANG Gang. A combined optimization model for transportation modes of multimodal transport[J]. Engineering Sciences, 2005, 7 (10): 46-50. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GCKX200510009.htm [15] 苏印, 李铁柱. 国际多式联运线路选择的方法研究[J]. 交通运输系统工程与信息, 2006, 6 (2): 91-94. https://www.cnki.com.cn/Article/CJFDTOTAL-YSXT200602017.htmSU Yin, LI Tie-zhu. A route choice method for international multi-modal transportation[J]. Journal of Transportation Systems Engineering and Information Technology, 2006, 6 (2): 91-94. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-YSXT200602017.htm [16] 康凯, 牛海娇, 朱越杰, 等. 基于粒子群蚁群算法求解多式联运中运输方式与运输路径集成优化问题[J]. 物流工程与管理, 2009, 31 (10): 61-65. https://www.cnki.com.cn/Article/CJFDTOTAL-SPCY200910026.htmKANG Kai, NIU Hai-jiao, ZHU Yue-jie, et al. Research on improved integrated optimization model for mode and route in multimodal transportation basing on the PSO-ACO[J]. Logistics Engineering and Management, 2009, 31 (10): 61-65. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-SPCY200910026.htm [17] 王金华. 基于运输合理化的多式联运路径优化[D]. 上海: 上海交通大学, 2010.WANG Jin-hua. Optimization of the path of the multimodal network on the base of transport rationalization[D]. Shanghai: Shanghai Jiaotong University, 2010. (in Chinese). [18] 刘尧. 公路桥梁在大件运输中的若干问题研究[D]. 杭州: 浙江大学, 2006.LIU Yao. The study on the problems of the bridges in the project of the large-scale equipments transportation[D]. Hangzhou: Zhejiang University, 2006. (in Chinese). [19] 肖晓伟, 肖迪, 林锦国, 等. 多目标优化问题的研究概述[J]. 计算机应用研究, 2011, 28 (3): 805-808. https://www.cnki.com.cn/Article/CJFDTOTAL-JSYJ201103001.htmXIAO Xiao-wei, XIAO Di, LIN Jin-guo, et al. Overview on multi-objective optimization problem research[J]. Application Research of Computers, 2011, 28 (3): 805-808. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JSYJ201103001.htm [20] 康凯, 牛海娇, 朱越杰, 等. 多式联运中运输方式与运输路径集成优化模型研究[J]. 计算机应用研究, 2010, 27 (5): 1672-1675. https://www.cnki.com.cn/Article/CJFDTOTAL-JSYJ201005019.htmKANG Kai, NIU Hai-jiao, ZHU Yue-jie, et al. Research of improved integrated optimization model for mode and route in multimodal transportation[J]. Application Research of Computers, 2010, 27 (5): 1672-1675. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JSYJ201005019.htm [21] 符卓. 开放式车辆路径问题及其应用研究[D]. 长沙: 中南大学, 2003.FU Zhuo. The open vehicle routing problems and their application[D]. Changsha: Central South University, 2003. (in Chinese). -
下载:
点击查看大图
图(5) / 表(5)
计量
- 文章访问数: 588
- HTML全文浏览量: 96
- PDF下载量: 1355
- 被引次数: 0
