BFRP加固损伤混凝土梁挠度计算方法

秦丽辉; 李岩; 王宗林; AL-DULAIMIA F N

BFRP加固损伤混凝土梁挠度计算方法

1.
东北农业大学水利与建筑学院, 黑龙江哈尔滨 150030
2.
哈尔滨工业大学交通科学与工程学院, 黑龙江哈尔滨 150090
3.
姆萨巴技术学院建筑工程系, 巴比伦姆萨巴

基金项目:

国家自然科学基金项目 51108132

详细信息

作者简介:
秦丽辉(1977-), 女, 吉林舒兰人, 东北农业大学讲师, 工学博士, 从事桥梁加固研究

中图分类号: U441.3
计量
- 文章访问数: 587
- HTML全文浏览量: 144
- PDF下载量: 807
- 被引次数: 0
出版历程
- 收稿日期: 2014-06-18
- 刊出日期: 2014-12-25

Deflection calculating method of damaged concrete beams strengthened with BFRP

1.
School of Water Conservancy and Civil Engineering, Northeast Agricultural University, Harbin 150030, Heilongjiang, China
2.
School of Transportation Science and Engineering, Harbin Institute of Technology, Harbin 150090, Heilongjiang, China
3.
Department of Building and Construction Engineeing, Al-MussaibTechnical College, Al-Mussaib, Babylon, Iraq

More Information

Author Bio:
QIN Li-hui (1977-), female, lecturer, PhD, +86-451-55191534, qinlh1977@126.com

摘要

摘要: 为了准确计算玄武岩纤维增强复合材料(BFRP) 加固损伤混凝土梁的挠度, 为BFRP加固损伤混凝土梁的设计与施工提供理论依据, 按照配筋率不同设计了11片试验梁进行试验, 每组试验梁设置不同的BFRP布加固量与加载方法。计算了各试验梁的荷载-挠度曲线, 分析了未加固梁、1层BFRP布加固梁、2层BFRP布加固梁在不同初始荷载下的挠度变化规律。给出了BFRP加固损伤混凝土梁跨中挠度的计算公式, 对比了挠度计算值与实测值。分析结果表明: BFRP加固混凝土梁的挠度受初始荷载和加固量的影响, 有初始荷载的加固梁挠度较无初始荷载的加固梁挠度增大30%~4%, 2层BFRP布加固梁的挠度较1层加固梁的挠度增大19%~2%, 计算挠度时需考虑BFRP布滞后应变的影响; 挠度计算值与实测值的最大差值平均为7.26 mm, 初始荷载小与配筋率高的试验梁挠度计算值与实测值较为接近, 可以用于实际工程计算。
- 桥梁工程 /
- 损伤混凝土梁 /
- 玄武岩纤维增强复合材料 /
- 挠度 /
- 加固 /
- 初始荷载
Abstract: In order to accurately calculate the deflection of damaged concrete beam strengthened with basalt fiber reinforced plastics (BFRP) and provide theoretical foundation for the design and construction of the beam, according to different reinforcement ratios, eleven test beams with different amounts of strengthened BFRP sheet and loading methods were designed.The loaddeflection curves of different test beams were obtained.Under different pre-loading programs, the changing rules of deflection of un-strengthened beams, strengthened beams with one layer and two layers of BFRP sheet were analyzed respectively.The calculation formulas of mid-span deflection of damaged concrete beam strengthened with BFRP were presented, and the calculated deflections and measured values were compared.Analysis result indicates that the deflections of damaged concrete beams strengthened with BFRP are influenced by initial load and amount of BFRP sheet layer.The deflections of strengthened beams with initial load increase by 30%-94%compared with strengthened beams without initial load, and the deflections of strengthenedbeams with two layers of BFRP sheet increase by 19%-42% compared with strengthened beams with one layer of BFRP sheet.The impact of post-strain of BFRP should be considered in deflection calculation.The average value of the maximum difference between the calculated deflection and measured value is 7.26 mm.When the initial load is small and the reinforcement ratio is high, the calculated deflection of concrete beam is closer to the measured value, and the method can be used in the engineering practice.
- bridge engineering /
- damaged concrete beam /
- BFRP /
- deflection /
- strengthening /
- initial load

HTML全文

图 1 试验梁配筋

Figure 1. Reinforcement of test beam

下载: 全尺寸图片幻灯片

图 2 试验梁BFRP加固

Figure 2. Test beams strengthened with BFRP

下载: 全尺寸图片幻灯片

图 3 加载装置与应变测点布置

Figure 3. Loading device and layout of strain test points

下载: 全尺寸图片幻灯片

图 4 未加固梁荷载-挠度曲线

Figure 4. Load-deflection curves of un-strengthened beams

下载: 全尺寸图片幻灯片

图 5 加固无损伤梁荷载-挠度曲线

Figure 5. Load-deflection curves of strengthened nondestructive beams

下载: 全尺寸图片幻灯片

图 6 梁A2-2与B2-2的荷载-挠度曲线

Figure 6. Load-deflection curves of A2-2 and B2-2 beams

下载: 全尺寸图片幻灯片

图 7 梁A1-1与A1-2的荷载-挠度曲线

Figure 7. Load-deflection curves of A1-1 and A1-2 beams

下载: 全尺寸图片幻灯片

图 8 梁A2-1、A2-2的荷载-挠度曲线

Figure 8. Load-deflection curves of A2-1 and A2-2 beams

下载: 全尺寸图片幻灯片

图 9 梁B2-2、B2-3的荷载-挠度曲线

Figure 9. Load-deflection curves of B2-2 and B2-3 beams

下载: 全尺寸图片幻灯片

图 10 试验梁最大挠度对比

Figure 10. Comparison of maximum deflections of test beams

下载: 全尺寸图片幻灯片

图 11 应力-应变关系

Figure 11. Relationships between stress and strain

下载: 全尺寸图片幻灯片

图 12 挠度计算值与实测值的对比

Figure 12. Comparison of calculated and measured deflections

下载: 全尺寸图片幻灯片

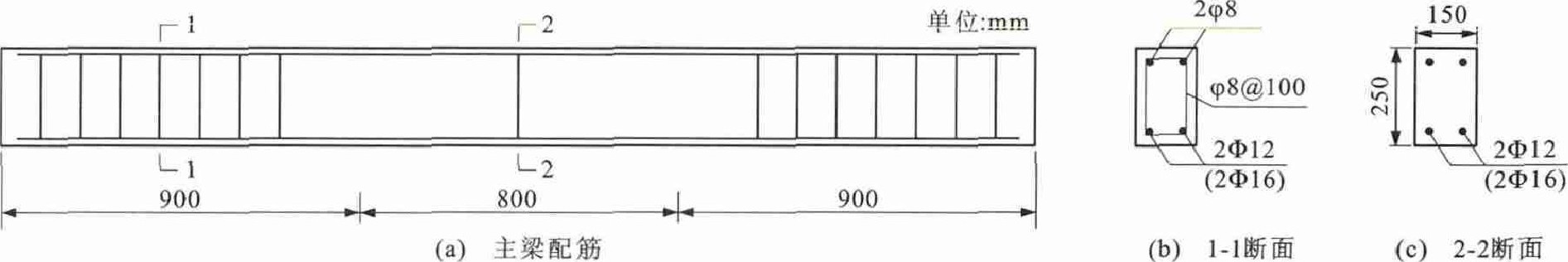
表 1 试验梁参数

Table 1. Parameters of test beams

下载: 导出CSV

表 2 试验梁加载方法

Table 2. Loading methods of test beams

下载: 导出CSV

参考文献(26)

[1]	李春霞. CFRP加固负载混凝土梁抗弯承载力及可靠度研究[D]. 武汉: 武汉理工大学, 2012. LI Chun-xia. Flexural bearing and reliability of loaded concrete beams strengthened with CFRP[D]. Wuhan: Wuhan University of Technology, 2012. (in Chinese).
[2]	CHOI W C, YUN H D. Long-term deflection and flexural behavior of reinforced concrete beams with recycled aggregate[J]. Materials and Design, 2013, 51 (5): 742-750. https://www.sciencedirect.com/science/article/pii/S0261306913003671
[3]	CHA P, CARBON K. An efficient approach to approximate the deflection curve of an arbitrarily supported beam subjectto external loads[J]. International Journal of Mechanical Engineering Education, 2013, 41 (2): 146-168. doi: 10.7227/IJMEE.41.2.7
[4]	MOHAMMADHASSANI M, NEZAMABADI-POUR H, JUMAAT M Z, et al. Application of artificial neural networks (ANNs) and linear regressions (LR) to predict the deflection of concrete deep beams[J]. Computers and Concrete, 2013, 11 (3): 237-252. doi: 10.12989/cac.2013.11.3.237
[5]	MOHAMMADHASSANI M, NEZAMABADI-POUR H, JUMAAT M Z, et al. Application of the ANFIS model in deflection prediction of concrete deep beam[J]. Structural Engineering and Mechanics, 2013, 45 (3): 319-332.
[6]	GRIBNIAK V, CERVENKA V, KAKLAUSKAS G. Deflection prediction of reinforced concrete beams by design codes and computer simulation[J]. Engineering Structures, 2013, 56 (11): 2175-2186. https://www.sciencedirect.com/science/article/pii/S0141029613004124
[7]	CASTEL A, FRANÇOIS R. Calculation of the overall stiffness and irreversible deflection of cracked reinforced concrete beams[J]. Advances in Structural Engineering, 2013, 16 (12): 2035-2042. doi: 10.1260/1369-4332.16.12.2035
[8]	MARAÍ R, OLLER E, BAIRÁN J M, et al. Simplified method for the calculation of long-term deflections in FRPstrengthened reinforced concrete beams[J]. Composites Part B: Engineering, 2013, 45 (1): 1368-1376. doi: 10.1016/j.compositesb.2012.07.003
[9]	RAFI M M, NADJAI A. A suggested model for European code to calculate deflection of FRP reinforced concrete beams[J]. Magazine of Concrete Research, 2011, 63 (3): 197-214. doi: 10.1680/macr.9.00085
[10]	MIÀS C, TORRES L, TURON A, et al. Effect of material properties on long-term deflections of GFRP reinforced concrete beams[J]. Construction and Building Materials, 2013, 41 (4): 99-108. https://www.sciencedirect.com/science/article/pii/S0950061812008914
[11]	MIÀS C, TORRES L, TURON A, et al. Experimental study of immediate and time-dependent deflections of GFRP reinforced concrete beams[J]. Composite Structures, 2013, 96 (2): 279-285. https://dugi-doc.udg.edu/handle/10256/11963
[12]	KARA I F, DUNDAR C. Prediction of deflection of high strength steel fiber reinforced concrete beams and columns[J]. Computers and Concrete, 2012, 9 (2): 133-151. doi: 10.12989/cac.2012.9.2.133
[13]	MOHAMED H M, MASMOUDI R. Deflection prediction of steel and FRP-reinforced concrete-filled FRP tube beams[J]. Journal of Composites for Construction, 2011, 15 (3): 462-472. doi: 10.1061/(ASCE)CC.1943-5614.0000172
[14]	高丹盈, 张明. 基于有效惯性矩的钢纤维高强混凝土梁刚度计算方法[J]. 中国公路学报, 2013, 26 (5): 62-68, 139. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201305013.htm GAO Dan-ying, ZHANG Ming. Calculation method for stiffness of steel fiber reinforced high-strength concrete beams based on effective moment of inertia[J]. China Journal of Highway and Transport, 2013, 26 (5): 62-68, 139. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201305013.htm
[15]	余琼, 张燕语. 预应力碳纤维布加固钢筋混凝土梁挠度研究[J]. 结构工程师, 2011, 27 (1): 139-143. https://www.cnki.com.cn/Article/CJFDTOTAL-JGGC201101024.htm YU Qiong, ZHANG Yan-yu. Study on deflection of RC beams strengthened with prestressed CFRP[J]. Structural Engineers, 2011, 27 (1): 139-143. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JGGC201101024.htm
[16]	唐义军. 碳纤维布加固RC梁后的荷载-挠度全曲线的简化计算[J]. 四川建筑科学研究, 2012, 38 (3): 109-111. https://www.cnki.com.cn/Article/CJFDTOTAL-ACZJ201203027.htm TANG Yi-jun. The simplification computation of entire loaddeflection curve of reinforced concrete beams strengthened with carbon fiber sheet[J]. Sichuan Building Science, 2012, 38 (3): 109-111. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ACZJ201203027.htm
[17]	王文炜, 赵国藩. 玻璃纤维布加固的钢筋混凝土梁挠度计算[J]. 四川建筑科学研究, 2004, 30 (3): 33-36. https://www.cnki.com.cn/Article/CJFDTOTAL-ACZJ200403012.htm WANG Wen-wei, ZHAO Guo-fan. Calculation of deflection of RC beams strengthened with GFRP sheets[J]. Sichuan Building Science, 2004, 30 (3): 33-36. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ACZJ200403012.htm
[18]	李春红, 魏德敏, 郑愚. GFRP筋混凝土板在拱效应下的挠度计算[J]. 江苏大学学报: 自然科学版, 2012, 33 (4): 474-479. doi: 10.3969/j.issn.1671-7775.2012.04.020 LI Chun-hong, WEI De-min, ZHENG Yu. Deflection estimation of concrete slab reinforced with GFRP bars with consideration of arching action[J]. Journal of Jiangsu University: Natural Science Edition, 2012, 33 (4): 474-479. (in Chinese). doi: 10.3969/j.issn.1671-7775.2012.04.020
[19]	张岩, 段树金, 郑岗. 考虑混凝土损伤的双面组合连续梁挠度和裂缝宽度研究[J]. 石家庄铁道大学学报: 自然科学版, 2011, 24 (3): 24-28. https://www.cnki.com.cn/Article/CJFDTOTAL-SJZT201103005.htm ZHANG Yan, DUAN Shu-jin, ZHENG Gang. Study on deflection and crack width of double composite continuous beam considering concrete damge[J]. Journal of Shijiazhuang Tiedao University: Natural Science, 2011, 24 (3): 24-28. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-SJZT201103005.htm
[20]	周勇军, 蔡军哲, 石雄伟, 等. 基于加权法的桥梁冲击系数计算方法[J]. 交通运输工程学报, 2013, 13 (4): 29-36. http://transport.chd.edu.cn/article/id/201304005 ZHOU Yong-jun, CAI Jun-zhe, SHI Xiong-wei, et al. Computing method of bridge impact factor based on weighted method[J]. Journal of Traffic and Transportation Engineering, 2013, 13 (4): 29-36. (in Chinese). http://transport.chd.edu.cn/article/id/201304005
[21]	徐荣桥, 陈德权. 组合梁挠度计算的改进折减刚度法[J]. 工程力学, 2013, 30 (2): 285-291. https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201302041.htm XU Rong-qiao, CHEN De-quan. Modified reduced stiffness method for calculating the deflection of composite beams[J]. Engineering Mechanics, 2013, 30 (2): 285-291. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201302041.htm
[22]	江南, 沈锐利. 矢跨比对悬索桥结构刚度的影响[J]. 土木工程学报, 2013, 46 (7): 90-97. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC201307014.htm JIANG Nan, SHEN Rui-li. Influence of rise-span ratio on structural stiffness of suspension bridge[J]. China Civil Engineering Journal, 2013, 46 (7): 90-97. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC201307014.htm
[23]	张元海, 林丽霞, 刘勇. 剪力滞效应对箱形梁挠度影响的研究[J]. 计算力学学报, 2012, 29 (4): 625-630. https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG201204026.htm ZHANG Yuan-hai, LIN Li-xia, LIU Yong. Influence of shear lag effect on deflection of box girder[J]. Chinese Journal of Computational Mechanics, 2012, 29 (4): 625-630. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG201204026.htm
[24]	杜进生, 区达光. UPPC梁的开裂截面惯性矩及挠度计算研究[J]. 工程力学, 2014, 31 (2): 170-176. https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201402025.htm DU Jin-sheng, AU F T K. Moment of inertia of cracked sections and deflections for UPPC beams[J]. Engineering Mechanics, 2014, 31 (2): 170-176. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201402025.htm
[25]	孟刚, 贾金青, 王吉忠. 预应力超高强混凝土简支梁抗弯性能分析[J]. 哈尔滨工程大学学报, 2013, 34 (5): 575-580. https://www.cnki.com.cn/Article/CJFDTOTAL-HEBG201305008.htm MENG Gang, JIA Jin-qing, WANG Ji-zhong. Study on flexural behavior of prestressed ultra-high strength concrete beams[J]. Journal of Harbin Engineering University, 2013, 34 (5): 575-580. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-HEBG201305008.htm
[26]	丁敏, 蒋秀根, 孟石平, 等. 整体-局部弯曲模型及其在简支组合梁中的应用[J]. 工程力学, 2012, 29 (12): 233-240. https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201212035.htm DING Min, JIANG Xiu-gen, MENG Shi-ping, et al. Global-local bending model and its application in simply supported compositebeam[J]. Engineering Mechanics, 2012, 29 (12): 233-240. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201212035.htm