Numerical simulation of critical hydroplaning speed of aircraft tire under wet pavement condition
-
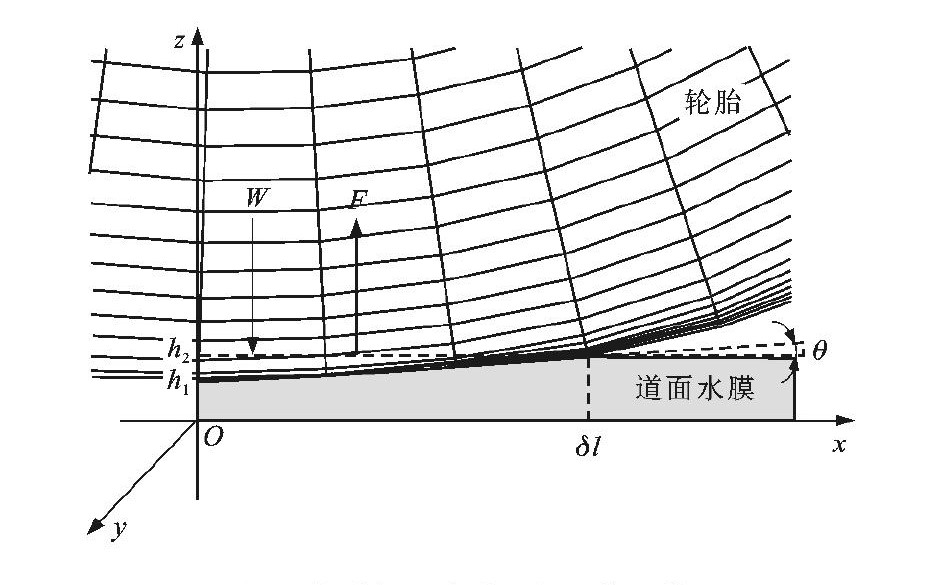
摘要: 采用ABAQUS建立了基于CEL算法的飞机轮胎与积水道面流固耦合分析模型, 推导了轮胎接触面动水压强与道面竖向支撑力表达式, 对比了飞机起飞与着陆过程中的滑行状态, 提出了临界滑水速度的上下限解概念, 校核了轮胎模型静态变形与动态滑水特征, 研究了胎压、胎纹与水膜厚度的影响规律, 分析了轮胎接地面积与动水压强分布。仿真结果表明: 在76.6kN轴载作用下, 轮胎模型接地面积为0.076m2, 轮胎中心竖向变形约为3.27cm, 轮胎临界滑水速度为128.5~222.4km·h-1, 与NASA轮胎滑水试验数据一致, 验证了仿真模型的合理性和适用性; 在胎压为1 140kPa时, 减速冲击条件下飞机轮胎临界滑水速度为163km·h-1, 小于加速冲击时的上限226km·h-1, 轮胎接地面积明显减小, 道面支撑力低于机轮轴载的10%;在450~1 109kPa胎压范围内, 减速冲击时临界滑水速度下限较NASA经验公式计算结果更为保守, 两者相差3070km·h-1; 轮胎纵向沟槽排水可降低轮胎前缘动水压强峰值, 增大轮胎接地面积, 减速冲击时带纹轮胎临界滑水速度较光滑轮胎提高了26.9%~28.8%, 增幅约为加速冲击时的2倍; 当道面水膜厚度由3mm增加至13mm时, 胎压为1 140kPa的飞机轮胎临界滑水速度上下限分别降低了85km·h-1和43km·h-1; 在低胎压、厚水膜与减速冲击条件下, 临界滑水速度下限仅为127km·h-1, 低于常见飞机进近接地速度205~250km·h-1, 因此, 滑水事故风险增加。Abstract: A fluid-solid coupling analysis model of aircraft tire and wet pavement based on CEL algorithm was developed by using ABAQUS.The expressions of hydrodynamic pressure of tire contact and vertical supporting force of pavement were derived.The taxiing conditions between aircraft take-off and landing process were compared.The concepts of upper and lower limit solutions of critical hydroplaning speed were proposed.The features of static deformation and dynamic hydroplaning of tire model were verified.The influence rules of tire pressure, tire pattern and water-film thickness were discussed. The contact area and distribution of hydrodynamic pressure for tire were analyzed.Simulation result indicates that the tire contact area is0.076 m2 under axle load of 76.6 kN, the vertical deformation at the centre of tire is 3.27 cm, and thecritical hydroplaning speed is 128.5-222.4 km·h-1, which is in consistence with the result of NASA's tire hydroplaning test.Therefore, the rationality and feasibility of simulation model are proved.When tire pressure is 1 140 kPa, the critical hydroplaning speed of aircraft tire under decelerating impact is 163 km·h-1 and lower than upper limit of accelerating impact (226 km·h-1), the tire contact area obviously reduces, and the supporting force from the pavement to the tire is less than 10% of wheel load.In comparison with the calculation result of NASA's empirical equation, the lower limits of critical hydroplaning speed under decelerating impact are more conservative within the scope of tire pressure from 450 kPa to 1 109 kPa, and the difference is30-70 km·h-1.The drainage effect of radial tire pattern can reduce the peak value of hydrodynamic pressure at the leading edge of aircraft tire and increase the tire contact area.The critical hydroplaning speed of aircraft tire with tire pattern under decelerating impact increases by26.9%-28.8% comparing with the speed of smooth tire, and the amplification is twice as much as that of accelerating impact.As the water-film thickness increases from 3 mm to 13 mm, the upper and lower limits of critical hydroplaning speed of aircraft tire respectively reduce by 85 km·h-1 and 43 km·h-1 when the tire pressure is 1 140 kPa.In case of lower tire pressure and thicker water-film, the lower limit of critical hydroplaning speed is merely 127 km·h-1 under decelerating impact and lower than most aircrafts'landing speeds 205-250 km·h-1, so the risk of hydroplaning accident increases.
-
表 1 轮胎参数
Table 1. Tire parameters

表 2 流体材料参数
Table 2. Parameters of fluid material

表 3 临界滑水速度比较
Table 3. Comparison of critical hydroplaning speeds km·h-1

表 4 胎纹对轮胎临界滑水速度影响
Table 4. Influence of tire pattern on critical hydroplaning speed

-
[1] SRIRANGAM S K, ANUPAM K, SCARPAS A, et al. Hydroplaning of rolling tires under different operating conditions[C]//ASCE. Airfield and Highway Pavement2013: Sustainable and Efficient Pavements. Reston: ASCE, 2013: 561-572. [2] 霍志勤, 茹毅, 韩松臣. 民航运输航空器着陆阶段偏出跑道事件分析模型[J]. 西南交通大学学报, 2012, 47 (5): 895-900. doi: 10.3969/j.issn.0258-2724.2012.05.026HUO Zhi-qin, RU Yi, HAN Song-chen. Analysis model of transport aircraft veering off runway during landing phase[J]. Journal of Southwest Jiaotong University, 2012, 47 (5): 895-900. (in Chinese). doi: 10.3969/j.issn.0258-2724.2012.05.026 [3] 霍志勤. 中国民航运输航空器偏/冲出跑道统计分析[J]. 中国安全生产科学技术, 2012, 8 (7): 127-132. https://www.cnki.com.cn/Article/CJFDTOTAL-LDBK201207029.htmHUO Zhi-qin. Statistical analysis on runway excursion of transport aircraft in China[J]. Journal of Safety Science and Technology, 2012, 8 (7): 127-132. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-LDBK201207029.htm [4] 余治国, 李曙林, 朱青云. 机轮动力滑水机理分析[J]. 空军工程大学学报: 自然科学版, 2004, 5 (5): 9-11. doi: 10.3969/j.issn.1009-3516.2004.05.003YU Zhi-guo, LI Shu-lin, ZHU Qing-yun. Mechanism analysis of an aircraft tire dynamic hydroplaning[J]. Journal of Air Force Engineering University: Natural Science Edition, 2004, 5 (5): 9-11. (in Chinese). doi: 10.3969/j.issn.1009-3516.2004.05.003 [5] 谷润平, 王鹏. 基于多元线性回归的湿/污染跑道着陆距离估算[J]. 中国民航大学学报, 2014, 32 (3): 20-22. doi: 10.3969/j.issn.1674-5590.2014.03.005GU Run-ping, WANG Peng. Estimation of wet and contaminated runway landing distance based on multiple linear regression[J]. Journal of Civil Aviation University of China, 2014, 32 (3): 20-22. (in Chinese). doi: 10.3969/j.issn.1674-5590.2014.03.005 [6] 李少波, 张宏超, 孙立军. 动水压力的形成与模拟测量[J]. 同济大学学报: 自然科学版, 2007, 35 (7): 915-918. doi: 10.3321/j.issn:0253-374X.2007.07.011LI Shao-bo, ZHANG Hong-chao, SUN Li-jun. Development and simulation measurement of dynamic hydraulic pressure[J]. Journal of Tongji University: Natural Science, 2007, 35 (7): 915-918. (in Chinese). doi: 10.3321/j.issn:0253-374X.2007.07.011 [7] 季天剑, 高玉峰, 陈荣生. 轿车轮胎动力滑水分析[J]. 交通运输工程学报, 2010, 10 (5): 57-60. doi: 10.3969/j.issn.1671-1637.2010.05.010JI Tian-jian, GAO Yu-feng, CHEN Rong-sheng. Dynamic hydroplaning analysis of car tire[J]. Journal of Traffic and Transportation Engineering, 2010, 10 (5): 57-60. (in Chinese). doi: 10.3969/j.issn.1671-1637.2010.05.010 [8] 高俊启, 陈昊, 季天剑, 等. 沥青路面动水压力光纤传感测量研究[J]. 传感器与微系统, 2009, 28 (9): 59-61. https://www.cnki.com.cn/Article/CJFDTOTAL-CGQJ200909020.htmGAO Jun-qi, CHEN Hao, JI Tian-jian, et al. Study of dynamic hydraulic pressure measurement on asphalt pavement using fiber-optic sensing[J]. Transducer and Microsystem Technologies, 2009, 28 (9): 59-61. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-CGQJ200909020.htm [9] 吕栋, 胡小弟, 周永莲, 等. 基于五维光纤传感器的沥青路面动水压力测量的研究[J]. 武汉工程大学学报, 2016, 38 (3): 268-272. https://www.cnki.com.cn/Article/CJFDTOTAL-WHHG201603013.htmLU Dong, HU Xiao-di, ZHOU Yong-lian, et al. Measurement of dynamic water pressure of asphalt pavement by fivedimensional optical fiber sensor[J]. Journal of Wuhan Institute of Technology, 2016, 38 (3): 268-272. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-WHHG201603013.htm [10] WRAYG A, EHRLICH I R. A systematic experimental investigation of significant parameters affecting model tire hydroplaning[R]. Hoboken: Stevens Institute of Technology, 1973. [11] BROWNE A L. Tire deformation during dynamic hydroplaning[J]. Tire Science and Technology, 1975, 3 (1): 16-28. doi: 10.2346/1.2167192 [12] WIES B, ROEGER B, MUNDL R. Influence of pattern void on hydroplaning and related target conflicts[J]. Tire Science and Technology, 2009, 37 (3): 187-206. doi: 10.2346/1.3137087 [13] AGRAWAL S K, HENRY J J. A simple tire deformation model for the transient aspect of hydroplaning[J]. Tire Science and Technology, 1980, 8 (3): 23-36. doi: 10.2346/1.2151019 [14] HORNE W B, DREHER R C. Phenomena of pneumatic tire hydroplaning[R]. Washington DC: National Aeronautics and Space Administration, 1963. [15] SETA E, NAKAJIMA Y, KAMEGAWA T, et al, Hydroplaning analysis by FEM and FVM: effect of tire rolling and tire pattern on hydroplaning[J]. Tire Science and Technology, 2000, 28 (3): 140-156. doi: 10.2346/1.2135997 [16] CHO J R, KIM K W, YOO W S, et al. Mesh generation considering detailed tread blocks for reliable 3Dtire analysis[J]. Advances in Engineering Software, 2004, 35 (2): 105-113. doi: 10.1016/j.advengsoft.2003.10.002 [17] CHO J R, KIM K W, JEON D H, et al. Transient dynamic response analysis of 3-D patterned tire rolling over cleat[J]. European Journal of Mechanics A: Solids, 2005, 24 (3): 519-531. doi: 10.1016/j.euromechsol.2005.01.004 [18] CHO J R, LEE H W, SOHN J S, et al. Numerical investigation of hydroplaning characteristics of three-dimensional patterned tire[J]. European Journal of Mechanics A: Solids, 2006, 25 (6): 914-926. doi: 10.1016/j.euromechsol.2006.02.007 [19] OH C W, KIM T W, JEONG H Y, et al. Hydroplaning simulation for a straight-grooved tire by using FDM, FEM and an asymptotic method[J]. Journal of Mechanical Science and Technology, 2008, 22 (1): 34-40. doi: 10.1007/s12206-007-1004-y [20] 赵珍辉, 李子然, 汪洋. 带复杂花纹的轮胎滑水显式动力学分析[J]. 汽车技术, 2010 (4): 34-38. doi: 10.3969/j.issn.1000-3703.2010.04.009ZHAO Zhen-hui, LI Zi-ran, WANG Yang. Explicit dynamic analysis of hydroplaning for tire with complex tread pattern[J]. Automobile Technology, 2010 (4): 34-38. (in Chinese). doi: 10.3969/j.issn.1000-3703.2010.04.009 [21] 臧孟炎, 陈高军, 林银辉. 湿滑路面轮胎制动距离有限元仿真分析[J]. 中国机械工程, 2012, 23 (10): 1246-1251. doi: 10.3969/j.issn.1004-132X.2012.10.024ZANG Meng-yan, CHEN Gao-jun, LIN Yin-hui. FEM analysis on wet-road braking distance of tire[J]. China Mechanical Engineering, 2012, 23 (10): 1246-1251. (in Chinese). doi: 10.3969/j.issn.1004-132X.2012.10.024 [22] SRIRANGAM S K, ANUPAM K, SCARPAS A, et al. Safety aspects of wet asphalt pavement surfaces through field and numerical modeling investigations[J]. Transportation Research Record, 2014 (2446): 37-51. [23] ANUPAM K, SRIRANGAM S K, SCARPAS A, et al. Study of cornering maneuvers of a pneumatic tire on asphalt pavement surfaces using the finite element method[J]. Transportation Research Record, 2014 (2457): 129-139. [24] FWA T F, ANUPAM K, ONG G P. Relative effectiveness of grooves in tire and pavement in reducing vehicle hydroplaning risk[C]//TRB. TRB 2010 Annual Meeting. Washington DC: TRB, 2009: 1-21. [25] PASINDU H R, FWA T F, ONG G P. Computation of aircraft braking distances[J]. Transportation Research Record, 2011 (2214): 126-135. -





 下载:
下载: