破舱倾覆船体扳正过程数值模拟

潘德位; 林成新; 周兆欣; 孙玉强; 刘志杰

破舱倾覆船体扳正过程数值模拟

1.
山东交通学院航海学院, 山东威海 264209
2.
大连海事大学交通运输装备与海洋工程学院, 辽宁大连 116026

基金项目:

交通运输部建设科技项目 2013328225080

高等学校博士学科点专项科研基金项目 20122125120013

国家科技支撑计划项目 2014BAK05B06

详细信息

作者简介:
潘德位(1986-), 男, 辽宁大连人, 山东交通学院讲师, 工学博士, 从事船舶打捞研究

通讯作者:
林成新(1963-), 男, 山东栖霞人, 大连海事大学教授, 工学博士

中图分类号: U698.6
计量
- 文章访问数: 733
- HTML全文浏览量: 186
- PDF下载量: 392
- 被引次数: 0
出版历程
- 收稿日期: 2017-06-10
- 刊出日期: 2017-10-25

Numerical simulation of righting process for damaged-capsized hull

1.
Navigation College, Shandong Jiaotong University, Weihai 264209, Shandong, China
2.
Transportation Equipment and Ocean Engineering College, Dalian Maritime University, Dalian 116026, Liaoning, China

More Information

Author Bio:
PANDe-wei(1986-), male, lecturer, PhD, wssrwsmt@163.com

Corresponding author: LIN Cheng-xin(1963-), male, professor, PhD, lch_xin@126.com

Article Text (Baidu Translation)

摘要

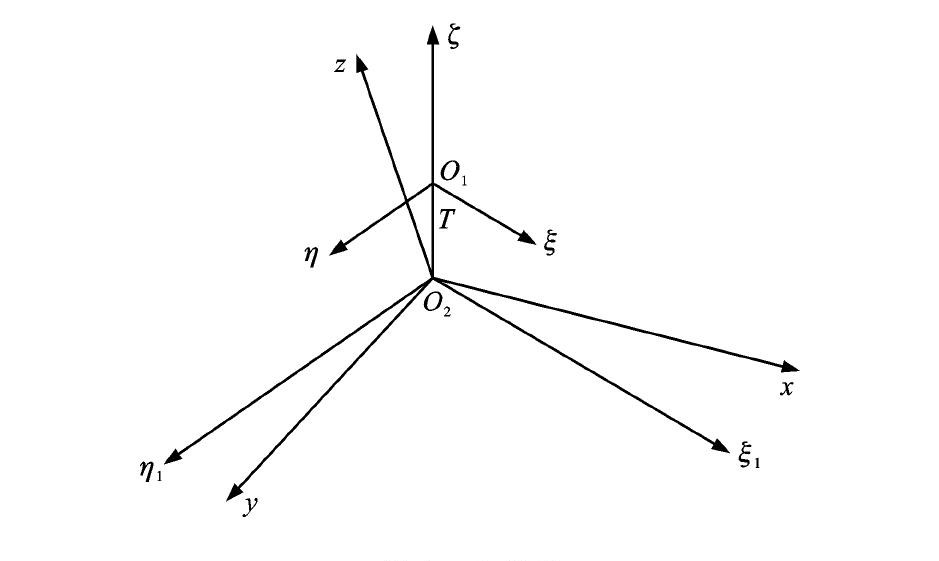
摘要: 考虑了破舱倾覆船体浮性和稳性, 研究了船体在扳正过程中空间位置和受力状态; 采用欧拉旋转变换方法建立了船体空间力学平衡方程, 根据船舶静力学原理, 得到了破舱倾覆船体稳性和扳正力数学模型; 根据伯努利定理计算了破舱进水量及其对船体重心和浮心位置的影响; 利用GHS软件模拟了破舱倾覆船体的扳正过程, 求解了其最大扳正力和进水量, 计算了船体纵向6个位置的剪力、弯矩和扭矩。计算结果表明: 在最初扳正时, 破舱进水导致倾覆船体扳正力矩降低了130.312 MN·m, 说明破舱进水降低了倾覆船体的稳性, 可以减小最初扳正力, 降低了扳正难度; 在扳正后期时, 破舱进水产生的倾斜力矩最大值为163.594 MN·m, 说明破舱进水降低了船体的稳性, 提高了扳正难度, 仍需要施加较大的扳正力平衡船体; 船体纵向强度分布会随着扳正力和破舱进水量的变化而改变, 多点扳正船体的最大扳正力小于单点最大扳正力的40%, 最大扭矩小于单点扭矩的50%;方案1~4的最大进水量分别为6 269.76、6 781.01、5 830.76、6 653.33t, 因此, 合理布置扳正点的位置, 单点扳正(方案1~3) 的进水量小于多点扳正(方案4)。
- 船舶工程 /
- 沉船打捞 /
- 倾覆船体 /
- 扳正方案 /
- 扳正力 /
- GHS
Abstract: The buoyancy and stability of damaged-capsized hull were considered, and the spatial position and mechanical of hull state were studied during righting process.The spatial mechanical equilibrium equation of hull was established by Euler rotation transformation method.The stability and righting mathematical model of hull were derived by using the hydrostatical theory of ship.The flooding quantity was calculated according to Bernoulli theorem and its impact on the positions of barycenter and buoyant centre were obtained.The righting process of damagedcapsized hull was simulated by using General HydroStatics (GHS) software, the maximum righting force and flooding quantity were solved, and the shear force, bending moment, torque of six longitudinal positions along the hull were calculated.Computation result shows that the righting moment of damaged-capsized hull decreases by 130.312 MN·m in the early righting process because water floods damaged cabins.So, the flooding water decreases the stability ofdamaged-capsized hull and the righting force, which results in the decrease of righting difficulty.In the later righting process, the maximum tilting moment of damaged-capsized hull is163.594 MN·m because water floods damaged cabins.Thus, flooding water decreases the stability of hull, increases the righting difficulty, and the larger righting force is needed to balance the hull.The longitudinal strength distribution of hull changes in response to the righting force and flooding quantity.The maximum force and torque of multi-point righting are less than40% and 50% of the corresponding values of single-point righting, respectively.The maximum flooding quantities in Schemes 1-4 are 6 269.76, 6 781.01, 5 830.76 and 6 653.33 t, respectively, which shows that the flooding quantities of single-point righting (Schemes 1-3) are less than the flooding quantity of multi-point righting (Scheme 4) through reaonably arranging the positions of righting points.
- ship engineering /
- wreck salvage /
- capsized hull /
- righting scheme /
- righting force /
- GHS

HTML全文

图 1 坐标系

Figure 1. Coordinate systems

下载: 全尺寸图片幻灯片

图 2 破舱进水

Figure 2. Damaged cabin bilging

下载: 全尺寸图片幻灯片

图 3 双体船

Figure 3. Catamaran

下载: 全尺寸图片幻灯片

图 4 船体和舱室

Figure 4. Hull and cabins

下载: 全尺寸图片幻灯片

图 5 倾覆船体

Figure 5. Capsized hull

下载: 全尺寸图片幻灯片

图 6 倾覆船体的稳性力矩曲线

Figure 6. Righting moment curves of capsized hull

下载: 全尺寸图片幻灯片

图 7 倾覆船体扳正过程中进水量

Figure 7. Flooding quantities of capsized hull during uprighting

下载: 全尺寸图片幻灯片

图 8 倾覆船体剪力曲线

Figure 8. Shear curves of capsized hull

下载: 全尺寸图片幻灯片

图 9 倾覆船体弯矩曲线

Figure 9. Bending moment curves of capsized hull

下载: 全尺寸图片幻灯片

图 10 倾覆船体扭矩曲线

Figure 10. Torque curves of capsized hull

下载: 全尺寸图片幻灯片

表 1 船体主尺度

Table 1. Principal dimensions of hull

下载: 导出CSV

参考文献(33)

[1]	BAČKALOV I, BULIAN G, ROSĚN A, et al. Improvement of ship stability and safety in intact condition through operational measures: challenges and opportunities[J]. Ocean Engineering, 2016, 120: 353-361. doi: 10.1016/j.oceaneng.2016.02.011
[2]	BAČKALOV I. Impact of contemporary ship stability regulations on safety of shallow-draught inland container vessels[J]. Safety Science, 2015, 72: 105-115. doi: 10.1016/j.ssci.2014.09.001
[3]	BOULOUGOURIS E, PAPANIKOLAOU A. Risk-based design of naval combatants[J]. Ocean Engineering, 2013, 65: 49-61. doi: 10.1016/j.oceaneng.2013.02.014
[4]	ELEFTHERIA E, APOSTOLOS P, MARKOS V. Statistical analysis of ship accidents and review of safety level[J]. Safety Science, 2016, 85: 282-292. doi: 10.1016/j.ssci.2016.02.001
[5]	GOERLANDT F, KUJALA P. Traffic simulation based ship collision probability modeling[J]. Reliability Engineering and System Safety, 2011, 96 (1): 91-107. doi: 10.1016/j.ress.2010.09.003
[6]	赵晓非. 船舶分舱和破舱稳性计算[J]. 大连理工大学学报, 1988, 28 (1): 87-92. https://www.cnki.com.cn/Article/CJFDTOTAL-DLLG198803017.htm ZHAO Xiao-fei. The calculation of subdivision and damage stability of ship[J]. Journal of Dalian University of Technology, 1988, 28 (1): 87-92. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-DLLG198803017.htm
[7]	林焰, 李铁骊, 纪卓尚. 破损船舶自由浮态计算[J]. 大连理工大学学报, 2001, 14 (1): 85-89. doi: 10.3321/j.issn:1000-8608.2001.01.019 LIN Yan, LI Tie-li, JI Zhuo-shang. Ship damage floating calculation[J]. Journal of Dalian University of Technology, 2001, 14 (1): 85-89. (in Chinese). doi: 10.3321/j.issn:1000-8608.2001.01.019
[8]	李玉明. 运木船破舱浮性、稳性、强度及破舱进水模拟研究[D]. 大连: 大连海事大学, 2002. LI Yu-ming. Study on the buoyancy, stability, longitudinal strength of lumber carrier and simulation of damaged flooding[D]. Dalian: Dalian Maritime University, 2002. (in Chinese).
[9]	RUPONEN P. Adaptive time step in simulation of progressive flooding[J]. Ocean Engineering, 2014, 78: 35-44. doi: 10.1016/j.oceaneng.2013.12.014
[10]	RUPONEN P. Progressive flooding of a damaged passenger ship[D]. Helsinki: Helsinki University of Technology, 2007.
[11]	VERMEER H, VREDEVELDT A W, JOURNÉE J M J. Mathematical modelling of motions and damaged stability of ro-ro ships in the intermediate stages of flooding[C]//Trove. Fifth International Conference on Stability of Ships and Ocean Structures. Canberra: Trove, 1994: 1-9.
[12]	VREDEVELDT A W, JOURNÉE J M J. Roll motions of ships due to sudden water ingress, calculations and experiments[C]//British Maritime Technology. International Conference on Ro-Ro Safety and Vulnerability the Way Ahead. London: British Maritime Technology, 1991: 1-17.
[13]	DOMEH V D K, SOBEY A J, HUDSON D A. A preliminary experimental investigation into the influence of compartment permeability on damaged ship response in waves[J]. Applied Ocean Research, 2015, 52: 27-36. doi: 10.1016/j.apor.2015.05.001
[14]	MANDERBACKA T, RUPONEN P. The impact of the inflow momentum on the transient roll response of a damaged ship[J]. Ocean Engineering, 2016, 120: 346-352. doi: 10.1016/j.oceaneng.2016.02.012
[15]	RODRIGUES J M, SOARES C G. A generalized adaptive mesh pressure integration technique applied to progressive flooding of floating bodies in still water[J]. Ocean Engineering, 2015, 110: 140-151. doi: 10.1016/j.oceaneng.2015.10.002
[16]	YAO T. Hull girder strength[J]. Marine Structures, 2003, 16 (1): 1-13. doi: 10.1016/S0951-8339(02)00052-7
[17]	IVANOV L D. On the relationship between maximum still water shear forces, bending moments, and radii of gyration of the total ship's weight and buoyancy forces[J]. Ships and Offshore Structures, 2007, 2 (1): 39-47. doi: 10.1533/saos.2006.0147
[18]	ŽAJA D, ZAMARIN A, HADJINA M. Longitudinal strength of a container ship[J]. Engineering Review, 2007, 27 (1): 55-66.
[19]	KHAN I A, DAS P K. Reliability analysis of intact and damaged ships considering combined vertical and horizontal bending moments[J]. Ships and Offshore Structures, 2008, 3 (4): 371-384. doi: 10.1080/17445300802369950
[20]	PAIK J K, THAYAMBALLI A K, PEDERSEN P T, et al. Ultimate strength of ship hulls under torsion[J]. Ocean Engineering, 2001, 28 (8): 1097-1133. doi: 10.1016/S0029-8018(01)00015-4
[21]	MOHAMMED E A, BENSON S D, HIRDARIS S E, et al. Design safety margin of a 10 000TEU container ship through ultimate hull girder load combination analysis[J]. Marine Structures, 2016, 46: 78-101. doi: 10.1016/j.marstruc.2015.12.003
[22]	ZHAO Meng-xin. Discussion on uprighting big-angle tilted sunken ships underwater in salvage operation[J]. China Ocean Engineering, 1989, 3 (2): 217-227.
[23]	赵孟信. 打捞工程中大倾度沉船水下扶正的探讨[J]. 海洋工程, 1988 (1): 11-18. https://www.cnki.com.cn/Article/CJFDTOTAL-HYGC198801001.htm ZHAO Meng-xin. The research of uprighting a sunken ship inclined at a big angle in salvage operation[J]. The Ocean Engineering, 1988 (1): 11-18. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-HYGC198801001.htm
[24]	赵孟信. "长征"轮打捞中关于扳正力的分析[J]. 航海技术, 1995 (2): 16-18, 20. https://www.cnki.com.cn/Article/CJFDTOTAL-HHJS199502005.htm ZHAO Meng-xin. Righting force analysis in Changzheng salvaging[J]. Marine Technology, 1995 (2): 16-18, 20. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-HHJS199502005.htm
[25]	DROBYSHEVSKI Y. A note on uprighting of a ship floating upside-down[J]. Ocean Engineering, 2004, 31 (11/12): 1447-1467.
[26]	ANTONINI A, LAMBERTI A, CECCARELLI G. What could happen if the parbuckling of Costa Concordia had failed: analytical and CFD-based investigation of possible generated wave[C]//LYNETT. Proceedings of 34th Conference on Coastal Engineering. Reston: Coastal Engineering Proceedings, 2014: 55-60.
[27]	DRUCE V. Costa Concordia's seafloor legacy[J]. New Scientist, 2013, 219 (2935): 6.
[28]	刘汉明, 顾泽月. 救捞作业场辅助设计软件的设计与实现[C]//中国航海学会. 救捞专业委员会2004年学术交流会论文集. 北京: 中国航海学会, 2004: 65-70. LIU Han-ming, GU Ze-yue. The design and realization of the auxiliary design software of layout for salvage boat[C]//China Institute of Navigation. Proceedings of Rescue and Salvage Conference 2004. Beijing: China Institute of Navigation, 2004: 65-70. (in Chinese).
[29]	潘德位. 倾覆船舶扳正过程中的受力分析与计算[D]. 大连: 大连海事大学, 2017. PAN De-wei. Force analysis and calculation for righting a capsized ship[D]. Dalian: Dalian Maritime University, 2017. (in Chinese).
[30]	马振伟. 静水中船舶破损稳性计算研究[D]. 武汉: 武汉交通科技大学, 1999. MA Zhen-wei. Calculation of damaged ship on still water[D]. Wuhan: Wuhan Transportation Technology University, 1999. (in Chinese).
[31]	PÉREZ F, CLEMENTE J A. Constrained design of simple ship hulls with B-spline surfaces[J]. Computer-Aided Design, 2011, 43 (12): 1829-1840. doi: 10.1016/j.cad.2011.07.008
[32]	PÉREZ-ARROABS F. Parametric generation of planing hulls[J]. Ocean Engineering, 2014, 81: 89-104.
[33]	CUI Hao, TURAN O, SAYER P. Learning-based ship design optimization approach[J]. Computer-Aided Design, 2012, 44 (3): 186-195.