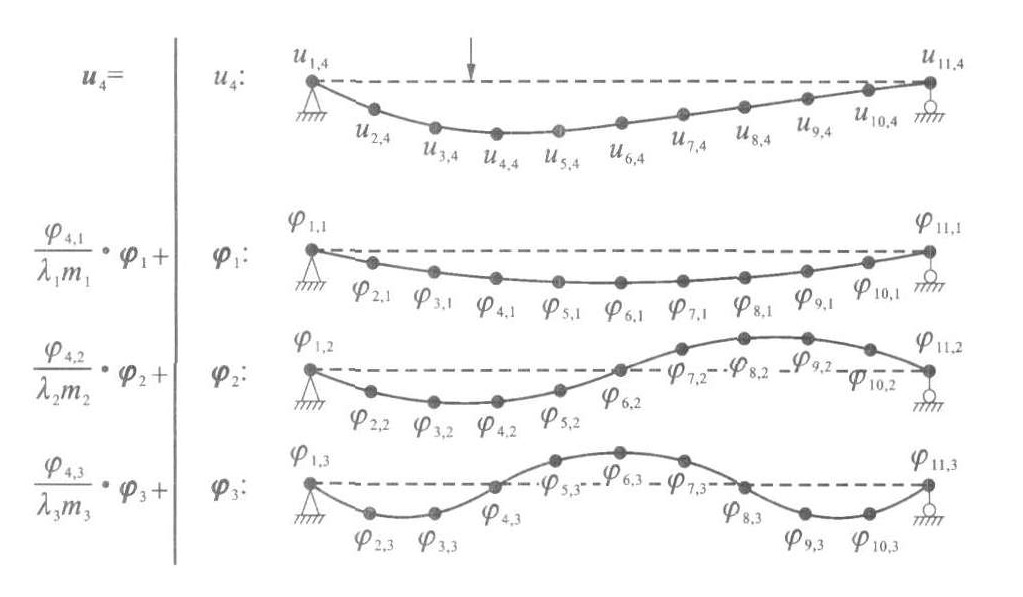
-
摘要: 以主梁挠度横向分布规律来确定桥梁荷载横向分布, 考虑了桥梁结构计算模态的固有频率、振型和模态质量, 提出了一种适用于各种结构形式桥梁的荷载横向分布系数计算方法, 即模态参数法。分别以一座有机玻璃模型试验桥梁和一座公路斜交T型桥梁为算例, 介绍了桥梁荷载横向分布系数的计算步骤。计算结果表明: 荷载横向分布系数的测量值与计算值最大误差为2.6%, 因此, 相比于传统的桥梁荷载横向分布系数计算方法, 模态参数法减小了对桥梁结构进行分类和假定带来的误差, 更具有通用性和准确性。Abstract: The load transversal distribution of bridge was confirmed according to the transversal distribution rule of main girder's vertical displacement. The modal parameters of bridge structure, such as natural frequency, modal shape and modal mass, were considered. A modal parameter method of calculating load transversal distribution factor was advanced for all kinds of bridge structure types. A test bridge model made of organic glass and a highway skew T bridge were selected to discuss the applying steps of the method. Computation result shows that the maximal difference between the computation value and the test value is only 2.6%, so the method for calculating the factor is more universal and exact than other traditional methods, and reduces the error resulted from the classification and assumption of bridge structures.
-
图 2 跨中荷载横向影响线
Figure 2. Middle section's influencing line of transversal load distribution
图 4 有跨中挠度的荷载横向分布
Figure 4. Influencing lines of transversal load distribution with middle section's flexibility
表 1 跨中截面荷载横向分布系数
Table 1. Middle section's transversal distribution factors
主梁 内横梁数 文献[6] 本文方法 中梁(3#梁) 0 0.464 0.452 1 0.416 0.413 3 0.410 0.410 
下载: 导出CSV
表 2 固有频率
Table 2. Natural frequencies
Hz 斜交角 阶次 1 2 3 4 0°(正交) 4.122 5.411 14.575 15.457 30°(斜交) 4.190 5.744 12.954 14.535 振型 对称弯曲 扭曲 反对称弯曲 扭曲
下载: 导出CSV
-
[1] 范立础. 桥梁工程[M]. 北京: 人民交通出版社, 2001 [2] 宋建永, 张浩阳, 张树仁. 公路桥梁荷载横向分布系数简化计算[J]. 东北公路, 2003, 26(4): 77-79. https://www.cnki.com.cn/Article/CJFDTOTAL-DBGL200304030.htmSONG Jian-yong, ZHANG Hao-yang, ZHANG Shu-ren. Si mplified calculation for distribution coefficients of highwaybridge load[J]. Northeast Highway, 2003, 26(4): 77-79. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-DBGL200304030.htm [3] 项贻强, 李新生, 申永刚, 等. 空间梁拱组合式桥梁的分析理论及试验研究[J]. 中国公路学报, 2002, 15(1): 67-81. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200201015.htmXI ANG Yi-qiang, LI Xin-sheng, SHEN Yong-gang, et al. Theory analysis and experi mental study of spatial girder and arch combination bridge[J]. China Journal of Highway and Transport, 2002, 15(1): 67-81. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200201015.htm [4] 项贻强, 杨万里, 潘仁泉, 等. 拱索体系加固的刚架拱桥荷载横向分布[J]. 中国公路学报, 2007, 20(4): 91-95. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200704017.htmXIANG Yi-qiang, YANG Wan-li, PAN Ren-quan, et al. Load Lateral distribution of rigid frame arch bridge strengthened with arch and stayed cable system[J]. China Journal of Highway and Transport, 2007, 20(4): 91-95. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200704017.htm [5] 赵煜, 张珂. 既有桥梁拓宽后承载潜力研究[J]. 长安大学学报: 自然科学版, 2003, 23(1): 51-53. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200301013.htmZHAO Yu, ZHANG Ke. Load carrying capacity of broadened existing bridge[J]. Journal of Chang an University: Natual Science Edition, 2003, 23(1): 51-53. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL200301013.htm [6] ZENG H, BERT C W. Free vibration of discretely stiffened skew plates[J]. International Journal of Structure Stability and Dynamics, 2001, 1(1): 125-144. doi: 10.1142/S0219455401000032 [7] 张阳, 邵旭东, 昌颖, 等. 大悬臂预应力组合桥面板实用计算方法[J]. 中国公路学报, 2007, 20(4): 79-94. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200706015.htmZHANG Yang, SHAO Xu-dong, CHANG Ying, et al. Applied computational method of long cantilevered pres-tressed composite bridge deck[J]. China Journal of Highway and Transport, 2007, 20(4): 79-94. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200706015.htm [8] KIN B H. Local damage detection using modal flexibility[D]. Texas: Texas A & amp; amp; M University, 2002. [9] CLOUGH R W, PENZIENJ. Dynamics of Structures[M]. 2nd Edition. New York: McGraw-Hill, 1996. [10] 李国豪, 石洞. 公路桥梁荷载横向分布计算[M]. 北京: 人民交通出版社, 1987. [11] JTG D60—2004, 公路桥涵设计通用规范[S]. -
点击查看大图
图(7) / 表(2)
计量
- 文章访问数: 696
- HTML全文浏览量: 324
- PDF下载量: 339
- 被引次数: 0
