Ship target detection algorithm on sea surface based on block chaos feature of image sequence
Article Text (Baidu Translation)
-
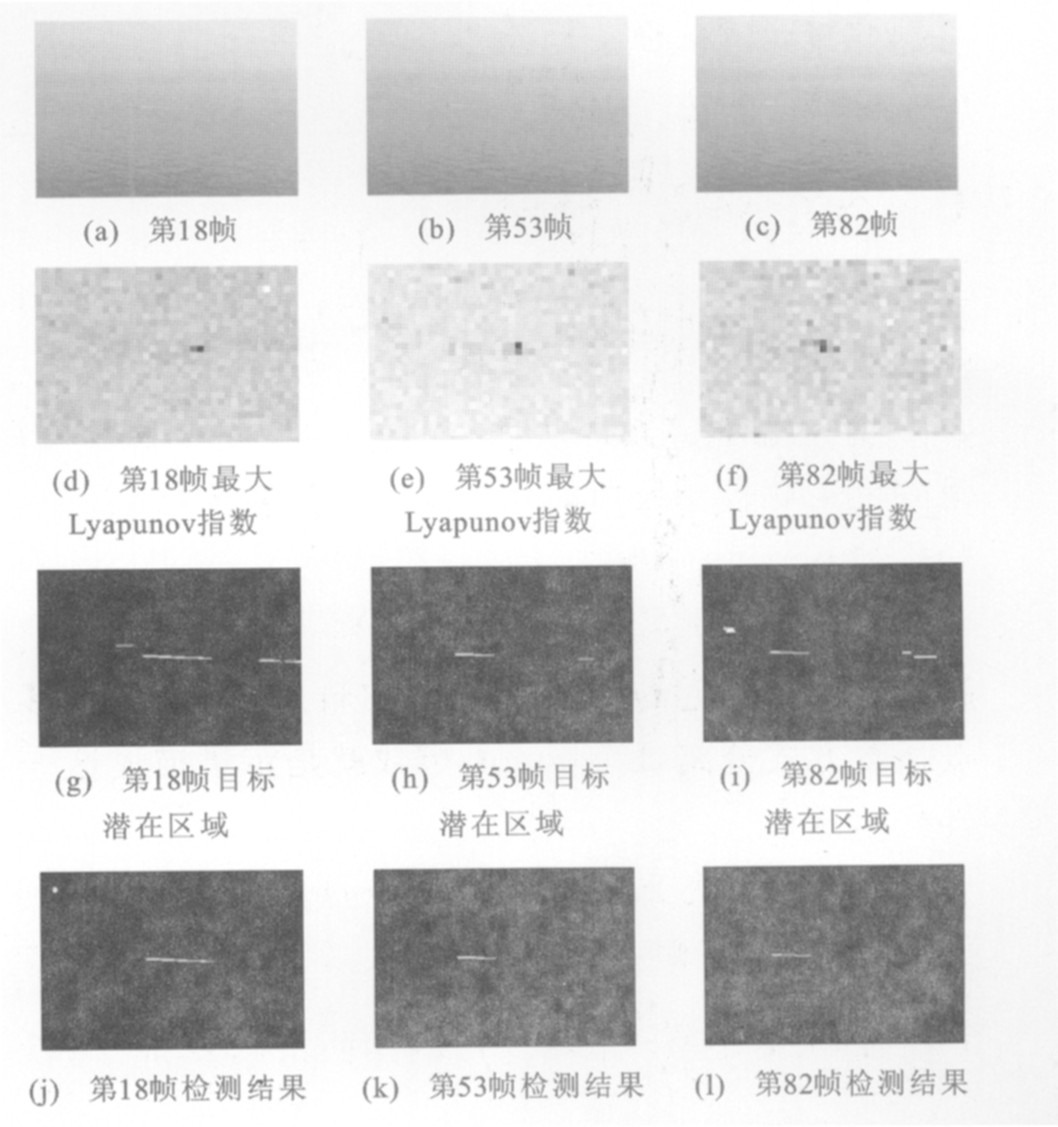
摘要: 为了检测复杂海面背景中的舰船目标, 提出了一种基于图像序列区域混沌特征的目标检测新算法, 算法利用小数据量法计算图像序列区域的最大Lyapunov指数, 分析运动目标存在时背景信号混沌特征的变化, 并利用混沌特征的变化差别检测淹没在混沌背景信号中的目标信号, 最后对100帧图像进行了目标检测。计算结果表明: 新算法检测率为100%, 虚警率为5%, 检测结果优于利用统计分析方法的结果。Abstract: In order to detect ship target on complex sea surface background, a new algorithm based on the block chaos feature of image sequence was proposed, the block largest Lyapunov exponent of image sequence was calculated by using small data sets, the change of background chaos feature was analyzed when moving target existed, ship target signal submerged by background signal was detected based on the change difference of chaos feature, and 100 frame images were used to detect ship target. Experiment result shows that the detection rate of the proposed algorithm is 100%, its false alarm rate is 5%, so the algorithm is superior to statistical analysis method.
-
Key words:
- traffic information engineering /
- image sequence /
- chaos feature /
- small data sets /
- target detection
-
表 1 检测结果比较
Table 1. Comparison of detection results
方法 检测目标个数 检测率/% 虚警目标个数 虚警率/% 文献[1]算法 98 98 8 8 本文算法 100 100 5 5 -
[1] LEE D S. Effective gaussian mixture learning for video back-ground subtraction[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2005, 27(5): 827-832. doi: 10.1109/TPAMI.2005.102 [2] 宿丁, 张启衡, 谢盛华. 一种强海杂波多目标分形分割算法[J]. 计算机工程与应用, 2006, 43(16): 12-14. doi: 10.3321/j.issn:1002-8331.2006.16.005SU Ding, ZHANG Qi-heng, XIE Sheng-hua. Segmentation algorithmfor multi-targetsin sea surf[J]. Computer Engineering and Applications, 2006, 43(16): 12-14. (in Chinese) doi: 10.3321/j.issn:1002-8331.2006.16.005 [3] 林三虎, 朱红, 赵亦工. 海杂波的混沌特性分析[J]. 系统工程与电子技术, 2004, 26(2): 178-180. doi: 10.3321/j.issn:1001-506X.2004.02.011LINSan-hu, ZHU Hong, ZHAO Yi-gong. Study of sea clutter chaotic dynamics[J]. Systems Engineering and Electronics, 2004, 26(2): 178-180. (in Chinese) doi: 10.3321/j.issn:1001-506X.2004.02.011 [4] 姜斌, 王宏强, 黎湘, 等. S波段雷达实测海杂波混沌分形特性分析[J]. 电子与信息学报, 2007, 29(8): 1809-1812. https://www.cnki.com.cn/Article/CJFDTOTAL-DZYX200708007.htmJI ANG Bin, WANG Hong-qiang, LI Xiang, et al. The anal-ysis of chaos and fractal characteristic based on the observed sea clutter of S-band radar[J]. Journal of Electronics andInformation Technology, 2007, 29(8): 1809-1812. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-DZYX200708007.htm [5] 何伍福, 王国宏, 刘杰. 海杂波环境中基于混沌的目标检测[J]. 系统工程与电子技术, 2005, 27(6): 1016-1020. doi: 10.3321/j.issn:1001-506X.2005.06.016HE Wu-fu, WANG Guo-hong, LI UJie. Target detection in sea clutter environment based on chaos[J]. Systems Engi-neering and Electronics, 2005, 27(6): 1016-1020. (in Chinese) doi: 10.3321/j.issn:1001-506X.2005.06.016 [6] WOLF A, SWIFTJ B, SWI NNEY HL, et al. Determining Lyapunov exponents froma ti me series[J]. Physica D, 1985, 16: 285-317. doi: 10.1016/0167-2789(85)90011-9 [7] ROSENSTEI N M T, COLLI NS J J, DE LUCA CJ. Aprac-tical method for calculating largest Lyapunov exponents from small data sets[J]. Physica D, 1993, 65: 117-134. doi: 10.1016/0167-2789(93)90009-P [8] KENNEL MB, BROWN R, ABARBANEL HDI. Determi-ning embedding di mension for phase-space reconstruction using a geometrical construction[J]. Physical Review A, 1992, 45(6): 3403-3411. doi: 10.1103/PhysRevA.45.3403 [9] RICHTER M, SCHREIBER T. Phase space embedding of electrocardiograms[J]. Physical Review E, 1998, 58(5): 6392-6398. doi: 10.1103/PhysRevE.58.6392 [10] LIEBERT W, SCHUSTER H G. Proper choice of the ti me delay for the analysis of chaotic ti me series[J]. Physics Let-ters A, 1989, 142: 107-128. doi: 10.1016/0375-9601(89)90169-2 [11] TAKENS F. Detecting strange attractors in turbulence[J]. Lecture Notes in Mathematics, 1981, 898: 366-381. [12] GRASSBERGER P, PROCACCI AI. Measuring the strange-ness of strange attractors[J]. Physica D, 1983, 9: 189-208. doi: 10.1016/0167-2789(83)90298-1 [13] ABRAHAM N B, ALBANO A M, DAS B, et al. Calculating the di mension of attractors fromsmall data sets[J]. Physics Letters A, 1986, 114: 217-221. doi: 10.1016/0375-9601(86)90210-0 [14] ZENG X, EYKHOLT R, PIELKE R A. Esti matingthe Lya-punov-exponent spectrumfromshort ti me series of lowpreci-sion[J]. Physical Review Letters, 1991, 66(25): 3229-3232. [15] ECKMANNJ P, KAMPHORST S O, RUELLE D, et al. Liapunov exponents fromti me series[J]. Physical Review A, 1986, 34(6): 4971-4979. doi: 10.1103/PhysRevA.34.4971 [16] ABARBANEL H D I, BROWN R, KENNEL M B. Local Lyapunov exponents computed from observed data[J]. Journal of Nonlinear Science, 1992, 2: 343-365. -





 下载:
下载: