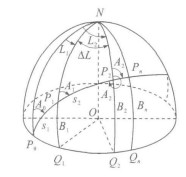
Improved algorithm for inverse solution of Bessel's geodetic problem
-
摘要: 为了解决目前贝塞尔大地主题反解算法不统一与存在适用条件限制的问题, 根据球面三角形正、余弦定理和拉格朗日级数理论, 用计算机代数系统推导并给出一种改进的大地主题直接反解的微分改正方法。该算法消除了逐次趋近计算, 适用传统反解算法中的奇异情况, 解决了求解三角方程中方位角的多值对应问题。试验结果表明, 当大地线长度小于20000km时, 算法精度高达0.0001s, 具有通用性, 特别适用于电算化, 对远洋大地线航法计算具有一定的应用价值。Abstract: In order solve the nonuniform algorithms of Bessel's inverse problem and the application restrictions of the algorithms, sine and cosine theories based on sphere triangle and Lagrange series theory were analyzed, and an improved arithmetic of differential correction for the direct inverse solution of geodetic problem was put forward by using computer algebra system. Simulation result shows that the improved algorithm is suitable for the arbitrarily special situations without iterative calculations, and has high accuracy of 0.000 1 s when geodetic length is less than 20 000 km. The azimuth multiple-valued corresponding problem in the solution of trigonometric equation is solved. The improved algorithm is also suitable for the program implementation without generality loss, and can be applied in the area of ocean geodetic line sailing computation.
-
表 1 长距离大地主题反算结果
Table 1. Inverse solution of geodetic problem on long distance
参数类型 算例1 a 6 378 388.0 m f 1.0/297.0 L1 010°00′00.″000 0 E B1 50°00′00.″000 0 N H1 0 m L2 105°05′38.″2 994 E B2 -62°57′03.″2 038 N H2 0 m s 14 999.999 996 457 848 km A1 140°00′00.″000 000 A2 294°46′41.″484 147 表 2 起点在最高纬度点处的大地主题反算结果
Table 2. Inverse solution of geodetic problem starting at the highest latitude
参数类型 算例2 a 6 378 245.0 m f 1.0/298.3 L1 35°00′00.″000 000 E B1 35°00′00.″000 000 N H1 0 m L2 173°13′02.″584 287 E B2 27°42′53.″569 421 N H2 0 m s 16 000.000 001 km A1 089°59′59.″999 859 A2 292°13′54.″917 935 表 3 终点在最高纬度点处的大地主题反算结果
Table 3. Inverse solution of geodetic problem ending at the highest latitude
参数类型 算例3 a 6 378 245.0 m f 1.0/298.3 L1 043°52′51.″265 641 E B1 24°00′11.″828 134 S H1 0 m L2 173°00′00.″000 000 E B2 35°00′00.″000 000 N H2 0 m s 15 000.000 000 km A1 063°47′27.″170 549 A2 270°00′00.″000 012 表 4 起点在南纬终点在北极的大地主题反算结果
Table 4. Inverse solution of geodetic problem starting at south latitude and ending at north pole
参数类型 算例4 a 6 378 245.0 m f 1.0/298.3 L1 070°00′00.″000 000 E B1 15°00′00.″000 000 S H1 0 m L2 070°00′00.″000 000 E B2 90°00′00.″000 000 N H2 0 m s 11 661.156 725 km A1 000°00′00.″000 000 A2 180°00′00.″000 000 -
[1] THOMAS C M, FEATHERSTONE W E. Validation of Vincenty s formulas for the geodesic using a newfourth-order extension of Kiviojas formula[J]. Journal of Surveying Engineering, 2005, 131(1): 20-26. doi: 10.1061/(ASCE)0733-9453(2005)131:1(20) [2] VI NCENTY T. Direct andinverse solution of geodesic onthe ellipsoid with application of nested equation[J]. Survey Review, 1975, 23(176): 88-93. doi: 10.1179/sre.1975.23.176.88 [3] BOWRI NG B R. Direct and inverse problems for short geodesic lines on the ellipsoid[J]. Surveying and Mapping, 1981, 41(2): 135-141. [4] BOWRI NG B R. Solutionfor azi muth of the geodesicin near-antipodal situations with special refernce to the behaviour of lines for whichthe azi muthisin the region of90°[J]. Journal of Geodesy, 1977, 51(1): 17-32. [5] KI VIOJA L A. Computation of geodetic direct and indirect problems by computers accumulating increments from geodetic line elements[J]. Bulletin Geodesque, 1971, 99(1): 55-63. doi: 10.1007/BF02521679 [6] 陈俊勇. 长距离大地主题反算的直接解法[C]//国家测绘总局测绘研究所. 大地测量研究专辑(第一辑). 北京: 测绘出版社, 1979: 126-135. [7] 纪兵, 边少锋. 大地主题问题的非迭代新解[J]. 测绘学报, 2007, 36(3): 269-273. doi: 10.3321/j.issn:1001-1595.2007.03.005JI Bing, BI AN Shao-feng. The new non-iterative solution to the geodetic problem[J]. Acta Geodaetica et Cartographica Sinica, 2007, 36(3): 269-273. (in Chinese) doi: 10.3321/j.issn:1001-1595.2007.03.005 [8] 史国友, 李伟, 贾传荧, 等. 高斯与墨卡托投影变换在船舶操纵模拟器中的应用[J]. 大连海事大学学报, 2002, 28(2): 25-28. doi: 10.3969/j.issn.1006-7736.2002.02.007SHI Guo-you, LI Wei, JI A Chuan-ying, et al. Application of transformation between Gauss-Kruger projection and Mercator projection in shiphandling si mulator[J]. Journal of Dalian Mariti me University, 2002, 28(2): 25-28. (in Chinese) doi: 10.3969/j.issn.1006-7736.2002.02.007 [9] 施一民, 范业明. 一种子午线正反解算的新方法[J]. 同济大学学报: 自然科学版, 2005, 33(7): 964-966. doi: 10.3321/j.issn:0253-374X.2005.07.023SHI Yi-min, FAN Ye-ming. New method for direct and inverse solution of meridian[J]. Journal of Tongji University: Natural Science, 2005, 33(7): 964-966. (in Chinese) doi: 10.3321/j.issn:0253-374X.2005.07.023 [10] BI AN Shao-feng, CHEN Yong-bing. Solving and inverse problem of a meridian arc in terms of computer algebra system[J]. Journal of Surveying Engineering, 2006, 132(1): 7-10. doi: 10.1061/(ASCE)0733-9453(2006)132:1(7) [11] 史国友, 周晓明, 贾传荧. 贝塞尔大地主题正解的改进算法[J]. 大连海事大学学报, 2008, 34(1): 15-19. https://www.cnki.com.cn/Article/CJFDTOTAL-DLHS200801003.htmSHI Guo-you, ZHOU Xiao-ming. JI A Chuan-ying. I mproved algorithmfor direct solution of Bessel s geodetic problem[J]. Journal of Dalian Mariti me University, 2008, 34(1): 15-19. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-DLHS200801003.htm -





 下载:
下载: