边坡稳定性联合评价方法

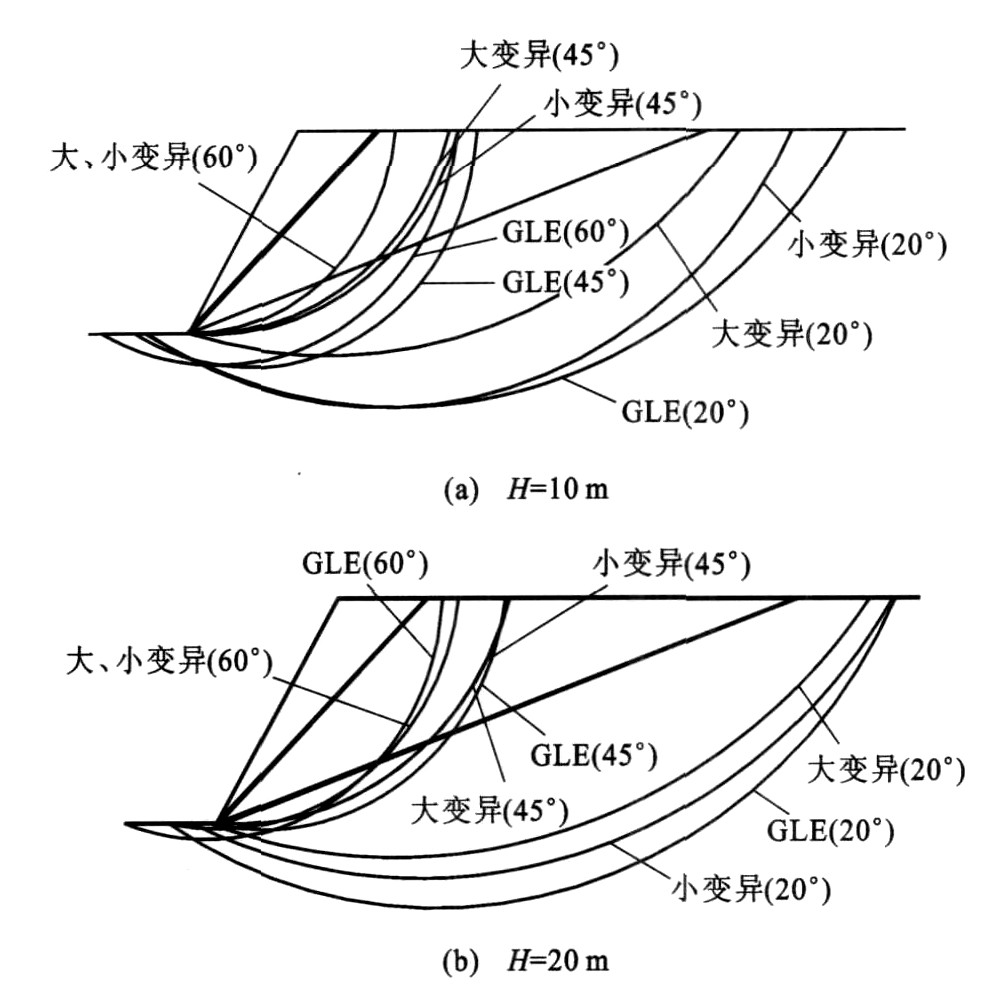
摘要: 借助MATLAB优化工具箱, 考虑土性参数变异性影响, 采用二次多项式序列响应面法求解边坡可靠指标和对应的安全系数, 并用可靠指标对应的失效概率对安全系数进行折减计算, 采用可靠指标与折减概率安全系数联合评价方法对边坡稳定性进行综合评价, 将计算结果和滑裂面图示与传统定值方法和二元指标方法的中值安全系数折减法进行对比分析。对比结果表明: 在土性参数变异性较大时, 即便传统定值方法计算出的安全系数明显大于1, 也会存在较大失稳风险; 但该方法利用失效概率对安全系数进行折减的过程, 实质是提取安全系数中可信部分的过程, 剔除了其中由于忽略不确定性因素而放大边坡稳定性的成分, 从而保证了评价结论的可靠性。
- 道路工程 /
- 边坡稳定性 /
- 安全系数 /
- 可靠指标 /
- 响应面法
Abstract: With MATLAB optimization toolbox, the parameter variability of soil was considered, the reliability index and corresponding safety factor of slope were computed by using response surface method(RSM)with quadratic sequence, and the safety factor was reduced by using the corresponding failure probability of the reliability index. A combined evaluation method, named reliability index and reduction safety factor evaluation method, was set up, the stability of slope was evaluated, and the calculation result and the failure surfaces obtained by the method were compared with the results obtained by traditional certification method and median reduction method of safety factor in dual index method. Comparison result indicates that slope may be unreliable with a safety factor(greater than 1)calculated by using traditional method when the parameter variability of soil is larger. In the present method, the reliable part of the safety factor is gotten by the reducing process, simultaneity, the unreliable part resulted from ignoring the uncertainty is deleted, so it can give a reasonable evaluation for practical slope.
- road engineering /
- slop stability /
- safety factor /
- reliability index /
- RSM

HTML全文

图 1 例1参数与滑裂面

Figure 1. Parameters and slipping surfaces of example 1

下载: 全尺寸图片幻灯片

图 2 例2参数与滑裂面

Figure 2. Parameters and slipping surface of example 2

下载: 全尺寸图片幻灯片

表 1 例1计算参数

Table 1. Calculation parameters of example 1

土性参数		c/kPa	φ/(°)	γ/(kN·m^-3)
平均值		35.0	15.0	17.50
小变异情况	变异系数	0.1	0.1	0.02
小变异情况	标准差	3.5	1.535	0.35
大变异情况	变异系数	0.3	0.2	0.10
大变异情况	标准差	10.5	3.068	1.75

下载: 导出CSV

表 2 例1计算结果

Table 2. Calculation result of example 1

坡度/(°)	坡高/m	变异性	β_min	F_{β_min}	F $_{β_{\min}}^{0}$	中值法F_min
20	10	小	8.080	2.451	2.451	2.577
	10	大	1.486	2.593	2.415	2.577
	20	小	5.657	1.699	1.699	1.808
	20	大	1.284	1.782	1.604	1.808
45	10	小	5.234	1.719	1.719	1.858
	10	大	0.913	1.737	1.423	1.858
	20	小	0.879	1.079	0.874	1.135
	20	大	0.169	1.079	0.612	1.135
60	10	小	4.099	1.488	1.488	1.759
	10	大	0.719	1.492	1.140	1.759
	20	小	5.01×10^-5	1.009	0.505	1.087
	20	大	9.99×10^-5	1.009	0.505	1.087

下载: 导出CSV

表 3 例2计算参数

Table 3. Calculation parameters of example 2

土层	土性参数		c/kPa	φ/(°)	γ/(kN·m^-3)
上层土	平均值		40.00	18.00	17.64
	小变异情况	变异系数	0.06	0.08	0.01
	小变异情况	标准差	2.40	1.44	0.18
	大变异情况	变异系数	0.20	0.10	0.05
	大变异情况	标准差	8.00	1.80	0.88
下层土	平均值		8.00	30.00	21.56
	小变异情况	变异系数	0.06	0.08	0.01
	小变异情况	标准差	0.48	2.40	0.22
	大变异情况	变异系数	0.20	0.10	0.05
	大变异情况	标准差	1.60	3.00	1.08

下载: 导出CSV

表 4 例2计算结果

Table 4. Calculation result of example 2

中值安全系数		F_min=1.249 0
变异性	β_min	F_{β_min}	F⁰_{β_min}	文献[7]
小	4.703 4	1.225	1.224 99	1.249 0
大	1.746 4	1.037	0.995 10	1.249 0
注: 文献[[7]]为利用二元指标体系方法计算得到的安全系数值。

下载: 导出CSV

参考文献(11)

[1]	何满潮, 苏永华, 景海河. 块状岩体的稳定可靠性分析模型及其应用[J]. 岩石力学与工程学报, 2002, 21(3): 343-348. doi: 10.3321/j.issn:1000-6915.2002.03.010 HE Man-chao, SU Yong-hua, JI NG Hai-he. Reliability analysis model of blocky rockmass stability andits application[J]. Chinese Journal of Rock Mechanics and Engineering, 2002, 21(3): 343-348. (in Chinese) doi: 10.3321/j.issn:1000-6915.2002.03.010
[2]	柳厚祥, 廖雪, 李宁, 等. 公路边坡稳定性分析的二维变分方法[J]. 中国公路学报, 2007, 20(4): 7-11. doi: 10.3321/j.issn:1001-7372.2007.04.002 LI U Hou-xiang, LI AO Xue, LI Ning, et al. 2-D Variational method of highway slope stability analysis[J]. China Journal of Highway and Transport, 2007, 20(4): 7-11. (in Chinese) doi: 10.3321/j.issn:1001-7372.2007.04.002
[3]	康海贵, 李炜. 基于安全系数解析式的边坡稳定评价体系研究[J]. 中国公路学报, 2008, 21(3): 1-5. doi: 10.3321/j.issn:1001-7372.2008.03.001 KANG Hai-gui, LI Wei. Research on evaluated system for slope reliability based on calculationformulae of safety factor[J]. China Journal of Highway and Transport, 2008, 21(3): 1-5. (in Chinese) doi: 10.3321/j.issn:1001-7372.2008.03.001
[4]	李炜, 康海贵. 土坡稳定分析最小折减安全系数评价体系研究[J]. 岩土工程学报, 2009, 31(5): 704-707. doi: 10.3321/j.issn:1000-4548.2009.05.010 LI Wei, KANG Hai-gui. Reduced safety factor evaluation systemfor soil slopes[J]. Chinese Journal of Geotechnical Engineering, 2009, 31(5): 704-707. (in Chinese) doi: 10.3321/j.issn:1000-4548.2009.05.010
[5]	康海贵, 李炜. 边坡稳定安全系数及其与土性参数及失效概率关系研究[J]. 大连理工大学学报, 2008, 48(6): 856-862. https://www.cnki.com.cn/Article/CJFDTOTAL-DLLG200806015.htm KANG Hai-gui, LI Wei. Research on slope safety factor and its relationship with shear strength parameters and failure probability[J]. Journal of Dalian University of Technology, 2008, 48(6): 856-862. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-DLLG200806015.htm
[6]	李炜, 康海贵. 边坡稳定性模糊随机可靠度分析[J]. 交通运输工程学报, 2010, 10(1): 19-23. doi: 10.3969/j.issn.1671-1637.2010.01.004 LI Wei, KANG Hai-gui. Fuzzy-randomreliability analysis of slope stability[J]. Journal of Traffic and Transportation Engineering, 2010, 10(1): 19-23. (in Chinese) doi: 10.3969/j.issn.1671-1637.2010.01.004
[7]	罗文强, 王亮清, 龚珏. 正态分布下边坡稳定性二元指标体系研究[J]. 岩石力学与工程学报, 2005, 24(13): 2288-2292. doi: 10.3321/j.issn:1000-6915.2005.13.015 LUO Wen-qiang, WANG Liang-qing, GONGJue. Study on slope stability by dual index system based on normal distribution[J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(13): 2288-2292. (in Chinese) doi: 10.3321/j.issn:1000-6915.2005.13.015
[8]	佟晓利, 赵国藩. 一种与结构可靠度分析几何法相结合的响应面方法[J]. 土木工程学报, 1997, 30(4): 51-57. doi: 10.3321/j.issn:1000-131X.1997.04.007 TONG Xiao-li, ZHAO Guo-fan. Aresponse method of struc-ture reliability degree jointed geometrical method[J]. China Civil Engineering Journal, 1997, 30(4): 51-57. (in Chinese) doi: 10.3321/j.issn:1000-131X.1997.04.007
[9]	武清玺, 卓家寿. 结构可靠度分析的变f序列响应面法及其应用[J]. 河海大学学报: 自然科学版, 2001, 29(2): 75-78. doi: 10.3321/j.issn:1000-1980.2001.02.017 WU Qing-xi, ZHUOJia-shou. Asequential response surface method with variousfand its application to structural reliability analysis[J]. Journal of Hohai University: Natural Sciences, 2001, 29(2): 75-78. (in Chinese) doi: 10.3321/j.issn:1000-1980.2001.02.017
[10]	韩玉芳, 刘德辅, 董胜. 边坡整体稳定的可靠性分析方法[J]. 海岸工程, 2001, 20(4): 7-14. doi: 10.3969/j.issn.1002-3682.2001.04.002 HAN Yu-fang, LIU De-fu, DONG Sheng. Reliability analysis method of slope stability[J]. Coastal Engineering, 2001, 20(4): 7-14. (in Chinese) doi: 10.3969/j.issn.1002-3682.2001.04.002
[11]	HUSEI N MALKAWI AI, HASSAN WF, ABDULLAF A. Uncertainty and reliability analysis applied to slope stability[J]. Structural Safety, 2000, 22(2): 161-187. doi: 10.1016/S0167-4730(00)00006-0