Finite element model of flexible airport pavement structure for large aircraft
-
摘要: 借助ABAQUS通用有限元软件, 建立了3层道面结构有限元模型, 分析了模型的几何尺寸、边界条件、层间接触条件、单元类型、网格的划分对大型飞机荷载作用下道面结构力学响应的影响, 提出了适应大型飞机的机场沥青道面结构有限元模型参数, 并用实测力学响应数据对模型的有效性进行了验证。研究结果表明: 在大型飞机全起落架荷载作用下, 有限元模型几何尺寸宜为30m×30m×10m, 层间完全连续选用tie连接; 单元类型宜采用C3D8R, 荷载区域的单元大小控制在不大于0.05m×0.05m;模型底部所有位移全部约束, 模型四周约束对应水平方向的位移。实测数据验证结果表明有限元模型有效。Abstract: The finite element model of three-layer pavement structure was set up by using general finite element software ABAQUS.The influences of model geometry size, boundary conditions, contact conditions among layers, element types, and grid divisions on the mechanical responses of pavement structure were analyzed under large aircraft load.The parameters of flexible airport pavement structure's finite element model were put forward to adapt the characteristic of large aircraft load, and the effectiveness of the model was verified by using the measured mechanical response data.Study result indicates that considering the whole landing gear load of large aircraft, the geometry size of finite element model is appropriately 30 m×30 m×10 m, and the tie is chosen for completely continuous interaction among layers.C3D8R is used as an optimal element type, and the element size of load region could be controlled not to exceed 0.05 m×0.05 m.All the displacements are constrained at the bottom of the model, horizontal displacement is corresponded with the model constraint around, and the finite element model is effective verified by measured data.
-
Key words:
- airport engineering /
- flexible airport pavement /
- finite element model /
- ABAQUS /
- large aircraft
-
表 1 道面结构参数
Table 1. Parameters of pavement structure
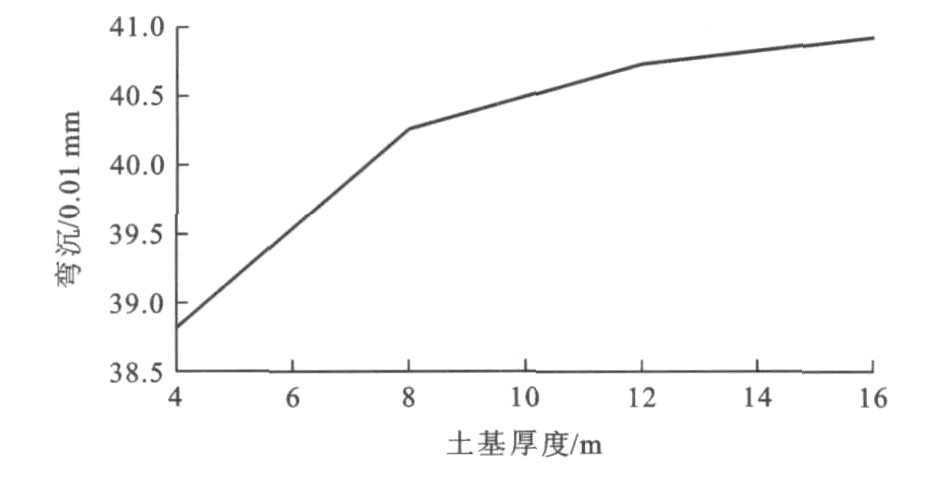
结构层 厚度/m 弹性模量/MPa 泊松比 面层 0.10 1 400 0.35 基层 1.16 500 0.35 土基 4.00、8.00、12.00、16.00 120 0.40 表 2 边界条件对计算结果的影响
Table 2. Influences of boundary conditions on calculation results
边界条件 整个CPU使用时间/s Mises应力/MPa 表面弯沉/mm 土基顶面压应变/10-5 约束某一个水平向的位移 603.1 0.586 5 0.406 9 -7.371 约束所有水平位移 381.7 0.584 9 0.404 4 -6.804 水平方向自由 7 699.3 0.586 6 0.408 7 -7.378 极差百分比/% 0.29 1.10 7.80 表 3 层间接触条件对计算结果的影响
Table 3. Influences of interfacing conditions on calculation results
类型 整个CPU使用时间/s Mises应力/MPa 表面弯沉/mm 土基顶面压应变/10-5 tie 576.5 0.586 3 0.403 8 -7.352 rough 8 217.8 0.607 2 0.417 4 -7.030 层间剖分 500.3 0.585 3 0.403 0 -7.345 极差百分比/% 3.6 3.4 4.4 表 4 三维六面体单元的基本性质
Table 4. Basic properties of 3D hexahedral elements
单元名称 单元类型 结点数/积分点数 位移形函数 优点 存在问题 C3D8 线性等参单元, 完全积分 8/8 一阶 计算成本低 剪切自锁, 精度较差 C3D8R 线性等参单元, 减缩积分 8/8 一阶 对位移的求解较精确, 弯曲时不易剪切自锁 沙漏 C3D8I 线性等参单元, 非协调模式 8/8 一阶 克服了剪切自锁, 在弯曲问题中计算成本降低 扭曲过大, 分析精度会降低 C3D20 二次等参单元, 完全积分 20/27 Serendipity二阶 对应力的计算很精确, 一般无剪切自锁 不能用于接触分析, 体积自锁, 弯曲自锁 C3D20R 二次等参单元, 减缩积分 20/8 Serendipity二阶 不会出现严重的沙漏问题, 对自锁问题不敏感 不能在接触分析中使用, 不适用于大变形问题 表 5 不同单元类型数值计算结果
Table 5. Numerical calculation results of different elements types
单元名称 C3D8 C3D8R C3D8I C3D20R 道面表面 竖向变形/mm 0.373 7 0.392 7 0.376 9 0.382 1 竖向压力/MPa -0.631 0 -0.650 9 -0.712 6 -0.801 0 土基顶面 竖向变形/mm 0.116 2 0.125 9 0.116 0 0.117 5 竖向压力/kPa -8.587 -8.626 -8.345 -9.715 整个CPU使用时间/s 164.7 131.7 235.6 2 185.2 表 6 不同网格密度计算结果
Table 6. Calculation results of different mesh densities
单元大小/m 0.2×0.2 0.1×0.1 0.05×0.05 道面表面 竖向变形/mm 0.495 5 0.392 7 0.404 4 竖向压力/MPa -0.586 5 -0.650 9 -0.683 9 单元总数 17 408 33 282 69 696 整个CPU使用时间/s 53.5 131.7 381.7 表 7 材料参数
Table 7. Material parameters
结构层 厚度/cm 回弹模量/MPa 泊松比 沥青混凝土面层 12.7 8 963 0.35 沥青混凝土基层 12.4 8 963 0.35 级配碎石底基层 21.6 450 0.38 土基 258.1 55 0.40 -
[1] PATTERSON J W. Impact of new large aircraft on airport design[R]. Washington DC: US Department of Transporta-tion, 1998. [2] WILLIS M, JOHNSON D, SUKUMARAN B. Three-dimen-sional finite element analyses of flexible airport pavements for the next generation of aircrafts[C]//IMAD L A. Airfield and Highway Pavements. Urbana: ASCE, 2006: 13-24. [3] HAYHOE G F. Alpha factor determination using data col-lected at the national airport pavement test facility[R]. Washington DC: US Department of Transportation, 2006. [4] Federal Aviation Administration. Airport pavement design for the Boeing777airplane[R]. Washington DC: US Department of Transportation, 1995. [5] Federal Aviation Administration. Airport pavement design and evaluation[R]. Washington DC: US Department of Transportation, 1995. [6] Federal Aviation Administration. Airport pavement design and evaluation[R]. Washington DC: US Department of Transportation, 2009. [7] THOMPSON M R, GARG N. Wheel load interaction: crit-ical airport pavement responses[R]. Urbana: University of Illinois at Urbana-Champaign, 1999. [8] 赵鸿铎. 适应大型飞机的沥青道面交通荷载分析方法及参数的研究[D]. 上海: 同济大学, 2006.ZHAO Hong-duo. New generation large aircraft oriented load analysis method and parameters for asphalt pavement design[D]. Shanghai: Tongji University, 2006. (in Chinese) [9] SUKUMARAN B, WILLIS M, CHAMALA N. Three dimen-sional finite element modeling of flexible pavements[C]//SCHW-ARTZ C W, TUTUMLUER E, TASHMAN L. Advances in Pavement Engineering(GSP130). Austin: ASCE, 2005: 1-12. [10] SAAD B, MITRI H, POOROOSHASB H. Three-dimen-sional dynamic analysis of flexible conventional pavement foundation[J]. Journal of Transportation Engineering, 2005, 131(6): 460-469. doi: 10.1061/(ASCE)0733-947X(2005)131:6(460) [11] KUO C M, CHOU F J. Development of3-D finite element model for flexible pavements[J]. Journal of the Chinese Institute of Engineers, 2004, 27(5): 707-717. doi: 10.1080/02533839.2004.9670918 [12] JOHNSON D J. Investigation of the performance of flexible pavement systems under moving loads using finite element analyses[D]. Glassboro: Rowan University, 2008. [13] PARK D W. Characterization of permanent deformation in asphalt concrete using a laboratory prediction method and an elastic-viscoplastic model[D]. College Station of Texas: Texas A & amp; amp; M University, 2004. [14] LIVNEH M, DIVINSKY M. Comparative analysis of the old MWHGL and the new NAPTF data[J]. Journal of Transpor-tation Engineering, 2007, 133(1): 20-29. doi: 10.1061/(ASCE)0733-947X(2007)133:1(20) -





 下载:
下载: