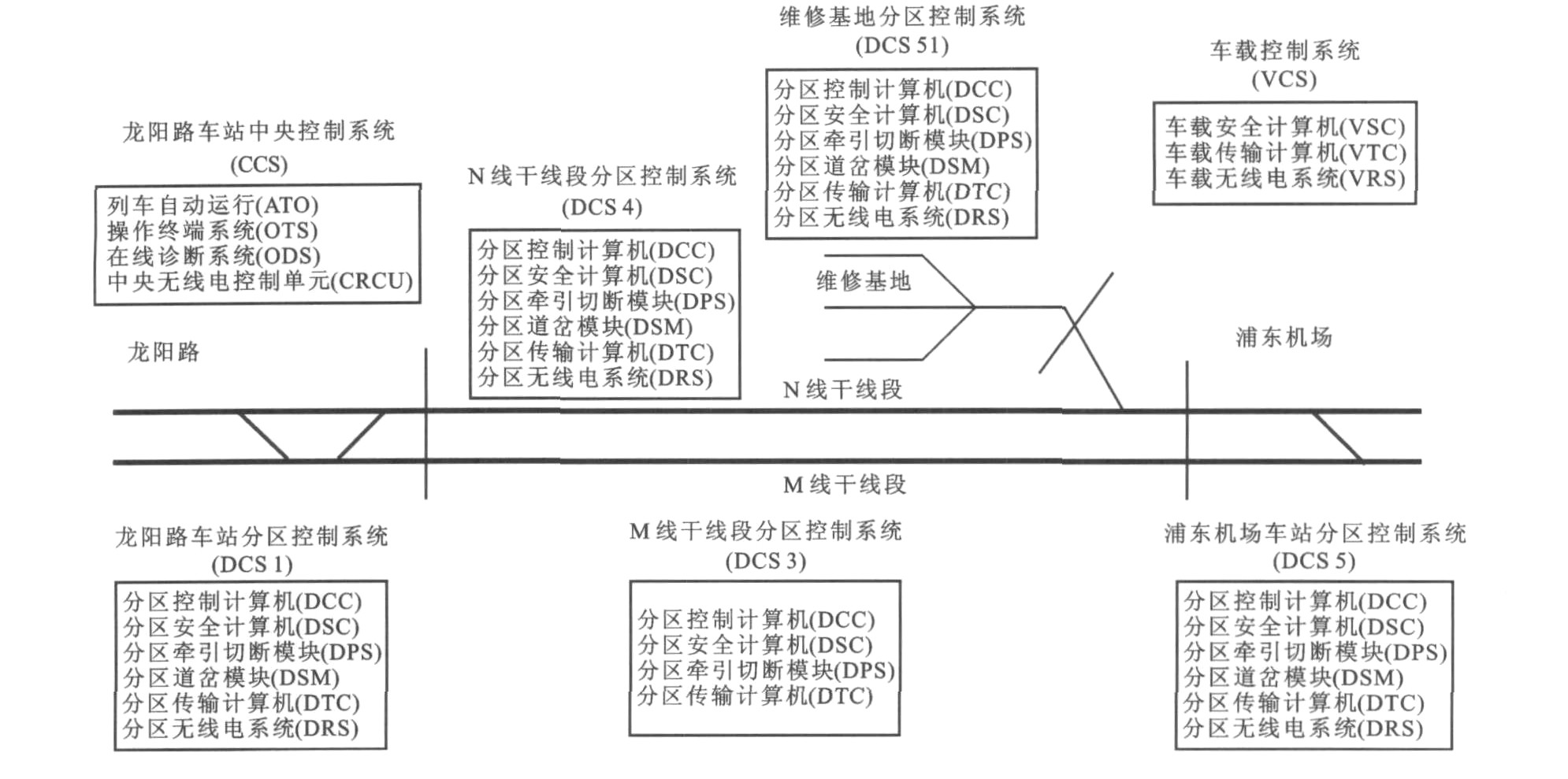
-
摘要: 根据磁悬浮列车跨系统运行需求, 研究了其运行控制系统的总体框架, 明确了需要增加的功能子系统。基于系统理论, 采用Petri网对系统关键属性、列车运行过程及各子系统的功能进行了层次化的建模。最高层模型描述系统整体关键属性, 低层模型描述列车运行过程及可靠性。此模型可用来定量分析磁悬浮列车系统层面上跨系统运行时, 失败率与各子系统部件可靠性之间的关系。如每年磁悬浮列车跨系统运行失败次数不超过1次, 则连接相邻列控系统的2个通信网, 其失效率都需低于10-6次·h-1。当列车跨系统运行触发时间分别为0.2、2.0min, 步进时间分别为4、16min时, 则跨线运行失败率分别为1.95×10-5、1.65×10-5次·h-1。仿真结果表明: 列车跨系统失败率随a网和b网可靠性的提高而降低, 同时随着跨系统触发时间和步进时间的增加而降低。层次化建模分析方法可以根据系统层面的关键属性要求, 定量确定各子系统部件的可靠性需求。Abstract: The general framework of running control system on maglev train was studied according to the running requirements of maglev train running across different control systems.Functional subsystems need to be added was defined.The hierarchical models of system key attributes, maglev operation procedures and the subsystem function were built based on the system theory by using Petri net.The key attributes of whole system were described by the highest model, and the operation procedures of maglev train and the reliabilities of subsystems were presented in the lower level model.The relationship between the failure rates of maglev train running across different control systems and the reliabilities of subsystem components was quantitatively analyzed with the model.It is pointed that the loss ratio of network connecting neibouring control systems should be lower than 10-6 times per hour when the required failure number of maglev train running across different systems is no more than 1 time per year.The failure rates of maglev train running across different control systems are 1.95×10-5 and 1.65×10-5 times per hour when the triggering times equal 0.2 and 2.0 min respectively, and the stepping times equal 4 and 16 min respectively.Simulation result shows that the failure rates of train running across the boundary decrease when the reliabilities of a and b networks are improved, or the triggering time and stepping time of train are prolonged.The reliability requirements of subsystem components based on the required key attributes of system level are quantantatively identified by using the proposed approach.
-
Key words:
- traffic control /
- maglev train /
- running across boundary /
- Petri net /
- system theory /
- hierarchical model /
- reliability
-
图 2 发送系统与目标系统之间的连接方式
Figure 2. Connection framework of transmitting system and target system
-
[1] 杨光, 唐祯敏. 几种典型轨道交通运行控制系统的比较研究[J]. 铁道学报, 2009, 31 (1): 82-87. https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200901020.htmYANG Guang, TANG Zhen-min. Analysis of similarities and differences of operation control systems among several typical modes of rail traffic[J]. Journal of the China Railway Society, 2009, 31 (1): 82-87. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB200901020.htm [2] HOSSEINI M S, VAEZ-ZADEH S. Modeling and analysis of linear synchronous motors in high-speed maglev vehicles[J]. IEEE Transactions on Magnetics, 2010, 46 (7): 2656-2664. doi: 10.1109/TMAG.2009.2039999 [3] CHEN M Y, WANG M J, FU L C. Modeling and controller design of a maglev guiding system for application in precision positioning[J]. IEEE Transactions on Industrial Electronics, 2003, 50 (3): 493-506. doi: 10.1109/TIE.2003.812354 [4] 杨光, 唐祯敏. 高速磁悬浮列车运行控制系统体系结构研究[J]. 中国铁道科学, 2006, 27 (6): 68-72.YANG Guang, TANG Zhen-min. Study on the operation control system framework for high-speed maglev train[J]. China Railway Science, 2006, 27 (6): 68-72. (in Chinese). [5] 张明. 基于UML的高速磁悬浮列车分区运行控制系统建模[D]. 北京: 北京交通大学, 2007.ZHANG Ming. Modeling of high-speed maglev train decentralized control system with UML[D]. Beijing: Beijing Jiaotong University, 2007. (in Chinese). [6] 杨光, 唐祯敏. 高速磁悬浮列车的安全速度防护问题研究[J]. 北京交通大学学报, 2007, 31 (2): 38-42.YANG Guang, TANG Zhen-min. Study on safety speed pro-tection of the high-speed maglev train[J]. Journal of Beijing Jiaotong University, 2007, 31 (2): 38-42. (in Chinese). [7] VON BERTALANFFY L. The histroy and status of general systems theory[J]. The Academy of Management Journal, 1972, 15 (4): 407-426. [8] LEVESON N. A new accident model for engineering safer systems[J]. Safety Science, 2004, 42 (4): 237-270. doi: 10.1016/S0925-7535(03)00047-X [9] VERNEZ D, BUCHS D R, PIERREHUMBERT G E, et al. MORM—a Petri net based model for assessing OH & amp; amp; S risks in industrial processes: modelling qualitative aspects[J]. Risk Analysis, 2004, 24 (6): 1719-1735. doi: 10.1111/j.0272-4332.2004.00562.x [10] ZHENG Wei. Modeling and hazard analysis of railway station protection system based on stochastic Petri nets[C]//IEEE. The Proceeding of 2009 8th International Conference on Reliability, Maintainability and Safety. Chengdu: IEEE, 2009: 493-496. [11] ZHENG Wei, MUELLER J R, SLOVAK R, et al. Function modelling and risk analysis of automated level crossing based on national statistical data[C]//IEEE. The 2nd International Asia Conference on Informatics in Control, Automation and Robotics. Wuhan: IEEE, 2010: 281-284. [12] 林闯, 郑波. 含有非马尔可夫过程的排队Petri网模型和性能分析[J]. 电子学报, 2003, 31 (2): 166-170. https://www.cnki.com.cn/Article/CJFDTOTAL-DZXU200302001.htmLIN Chuang, ZHENG Bo. A model of queuing Petri net including non-Markovian process and performance analysis[J]. Acta Electronica Sinica, 2003, 31 (2): 166-170. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-DZXU200302001.htm [13] 林闯, 曲扬, 郑波, 等. 一种随机Petri网性能等价化简与分析方法[J]. 电子学报, 2002, 30 (11): 1620-1623. https://www.cnki.com.cn/Article/CJFDTOTAL-DZXU200211010.htmLIN Chuang, QU Yang, ZHENG Bo, et al. An approach to performance equivalent simplification and analysis of stochastic Petri nets[J]. Acta Electronica Sinica, 2002, 30 (11): 1620-1623. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-DZXU200211010.htm [14] 李志纯, 朱道立. 随机动态交通网络可靠度分析与评价[J]. 交通运输工程学报, 2008, 8 (1): 106-112. http://transport.chd.edu.cn/article/id/200801021LI Zhi-chun, ZHU Dao-li. Analysis and evaluation of reliabil-ity for stochastic and dynamic transport network[J]. Journal of Traffic and Transportation Engineering, 2008, 8 (1): 106-112. (in Chinese). http://transport.chd.edu.cn/article/id/200801021 -
下载:
点击查看大图
图(9)
计量
- 文章访问数: 642
- HTML全文浏览量: 93
- PDF下载量: 741
- 被引次数: 0
