基于改进Sarma法的岩质边坡稳定性分析

周志军; 牛涌; 张铁柱

doi:10.19818/j.cnki.1671-1637.2013.01.003

基于改进Sarma法的岩质边坡稳定性分析

doi: 10.19818/j.cnki.1671-1637.2013.01.003

1.
长安大学特殊地区公路工程教育部重点实验室, 陕西西安 710064
2.
云南省公路开发投资有限责任公司, 云南昆明 650200

基金项目:

国家自然科学基金项目 41172262

详细信息

作者简介:
周志军(1975-), 男, 江苏泰兴人, 长安大学副教授, 工学博士, 从事边坡稳定与防护工程研究

中图分类号: U416.14
计量
- 文章访问数: 1082
- HTML全文浏览量: 262
- PDF下载量: 679
- 被引次数: 0
出版历程
- 收稿日期: 2012-09-18
- 刊出日期: 2013-02-25

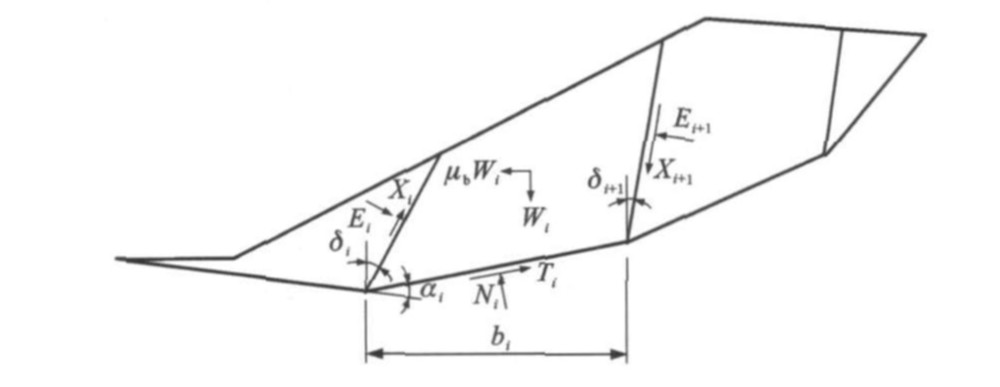
Stability analysis of rock slope based on improved Sarma method

1.
Key Laboratory for Special Area Highway Engineering of Ministry of Education, Chang'an University, Xi'an 710064, Shaanxi, China
2.
Yunnan Highway Development and Investment Co., Ltd., Kunming 650200, Yunnan, China

More Information

Author Bio:
ZHOU Zhi-jun(1975-), male, associate professor, PhD, +86-29-85355787, 5974100@qq.com

摘要

摘要: 以简单非均质岩质边坡的滑动为研究对象, 建立了Sarma法力学模型。根据岩质边坡块体静力平衡条件, 得出侧向正压力与临界加速度系数之间的关系, 推导了临界加速度系数公式, 得到边坡处于地震烈度条件下的安全稳定系数。分析了边坡的安全稳定系数与临界加速度系数之间的函数关系, 并利用此函数关系将传统Sarma法进行了改进。计算结果表明: 岩质边坡安全稳定系数与临界加速度系数之间呈单调递减的函数关系; 与传统Sarma法相比, 改进Sarma法无需迭代, 不存在收敛性问题, 算例分析中仅需6次计算就得到结果, 并能满足要求。
- 路基工程 /
- 岩质边坡 /
- 安全稳定系数 /
- 临界加速度系数 /
- Sarma法 /
- 力学模型
Abstract: The glide of simple heterogeneous rock slope was taken as research object, a mechanical model based on Sarma method was built. According to the static equilibrium condition of rock slope block, the relationship between critical acceleration coefficient and lateral normal pressure was got. The formula of critical acceleration coefficient was deduced, and the safety stability factor of slope under the condition of seismic intensity was got. The function relationship between safety stability factor and critical acceleration coefficient was analyzed, and the traditional Sarma method was improved by using the function relationship. Calculation result shows that the function relationship between safety stability factor and critical acceleration coefficient is monotone decreasing. Compared with the traditional Sarma method, the improved Sarma method does not need iteration, and does not exist convergence problem. The calculation number of example is only 6, and the calculation result meets requirement.
- subgrade engineering /
- rock slope /
- safety stability factor /
- critical acceleration coefficient /
- Sarma method /
- mechanical model

HTML全文

图 1 Sarma法力学模型

Figure 1. Mechanical model of Sarma method

下载: 全尺寸图片幻灯片

图 2 F与μ_b函数关系

Figure 2. Function relationship between F and μ_b

下载: 全尺寸图片幻灯片

图 3 计算模型

Figure 3. Calculation model

下载: 全尺寸图片幻灯片

表 1 计算结果

Table 1. Calculation result

F_i	i	μ_bi	b_i/m	c_i/kPa	φ_i/rad	c′_i/kPa	φ′_i/rad	W_i/kN	U_i/kPa	α_i/rad	e_i	S_i	R_i	δ_i/rad	P_i/kPa
1.0	1	0.038 7	3.56	34.1	28.4	48.5	0.0	967	42	46.0	6.2	151.8	175	0	42.4
	2		6.26	32.8	30.8	43.0	32.1	852	41	46.4	0.6	272.6	297	65	41.3
	3		4.26	38.5	32.7	46.8	18.7	591	39	42.1	0.8	195.3	221	49	39.6
	4		6.25	36.8	36.6	38.6	23.0	539	44	48.4	0.9	310.2	346	42	48.3
	5		5.27	37.3	38.5	36.3	25.9	863	50	49.5	0.8	265.5	302	50	46.3
	6		4.37	31.2	41.6	39.7	0.0	429	41	39.4	1.0	137.7	176	0	43.7
1.1	1	0.032 0	3.56	31.0	26.1	44.1	0.0	967	42	46.0	8.9	138.0	159	0	42.4
	2		6.26	29.8	28.4	39.1	29.7	852	41	46.4	0.6	247.8	270	65	41.3
	3		4.26	35.0	30.2	42.6	17.1	591	39	42.1	0.8	177.5	201	49	39.6
	4		6.25	33.4	34.0	35.1	21.1	539	44	48.4	1.0	282.0	315	42	48.3
	5		5.27	33.9	35.9	33.0	23.8	863	50	49.5	0.7	241.3	275	50	46.3
	6		4.37	28.4	38.9	36.1	0.0	429	41	39.4	1.0	125.2	160	0	43.7
1.2	1	0.024 4	3.56	28.4	24.2	40.4	0.0	967	42	46.0	14.0	126.5	146	0	42.4
	2		6.26	27.3	26.4	35.8	27.6	852	41	46.4	0.6	227.2	248	65	41.3
	3		4.26	32.1	28.1	39.0	15.7	591	39	42.1	0.8	162.8	184	49	39.6
	4		6.25	30.6	31.8	32.2	19.4	539	44	48.4	1.0	258.5	288	42	48.3
	5		5.27	31.1	33.5	30.2	22.0	863	50	49.5	0.7	221.2	252	50	46.3
	6		4.37	26.0	36.5	33.1	0.0	429	41	39.4	1.0	114.7	147	0	43.7
1.3	1	0.015 1	3.56	26.2	22.6	37.3	0.0	967	42	46.0	28.0	116.7	134	0	42.4
	2		6.26	25.2	24.6	33.0	25.8	852	41	46.4	0.6	209.7	229	65	41.3
	3		4.26	29.6	26.3	36.0	14.6	591	39	42.1	0.8	150.2	170	49	39.6
	4		6.25	28.3	29.8	29.7	18.0	539	44	48.4	1.0	238.6	266	42	48.3
	5		5.27	28.7	31.5	27.9	20.5	863	50	49.5	0.6	204.2	232	50	46.3
	6		4.37	24.0	34.3	30.5	0.0	429	41	39.4	1.0	105.9	136	0	43.7
1.4	1	0.002 5	3.56	24.3	21.1	34.6	0.0	967	42	46.0	226.0	108.4	125	0	42.4
	2		6.26	23.4	23.0	30.7	24.2	852	41	46.4	0.6	194.7	212	65	41.3
	3		4.26	27.5	24.6	33.4	13.6	591	39	42.1	0.8	139.5	158	49	39.6
	4		6.25	26.3	28.0	27.6	16.8	539	44	48.4	1.1	221.6	247	42	48.3
	5		5.27	26.6	29.6	25.9	19.1	863	50	49.5	0.6	189.6	216	50	46.3
	6		4.37	22.3	32.4	28.3	0.0	429	41	39.4	1.0	98.3	126	0	43.7
1.5	1	-0.018 0	3.56	22.7	19.8	32.3	0.0	967	42	46.0	-44.0	101.2	116	0	42.4
	2		6.26	21.8	21.6	28.6	22.7	852	41	46.4	0.6	181.7	198	65	41.3
	3		4.26	25.6	23.1	31.2	12.7	591	39	42.1	0.8	130.2	147	49	39.6
	4		6.25	24.5	26.4	25.8	15.8	539	44	48.4	1.1	206.8	231	42	48.3
	5		5.27	24.9	27.9	24.2	17.9	863	50	49.5	0.6	177.0	201	50	46.3
	6		4.37	20.8	30.6	26.5	0.0	429	41	39.4	1.0	91.8	118	0	43.7

下载: 导出CSV

参考文献(12)

[1]	SARMA S K. Stability analysis of embankments and slopes[J]. Geotechnique, 1973, 23(3): 423-433. doi: 10.1680/geot.1973.23.3.423
[2]	SARMA S K. Critical acceleration vs. static factor of safetyin stability analysis of earth dams and embankments[J]. Geotechnique, 1974, 24(4): 661-665. doi: 10.1680/geot.1974.24.4.661
[3]	MORGENSTERN N R, PRICE V E. The analysis of stability of general slip surface[J]. Geotechnique, 1965, 15(1): 79-93. doi: 10.1680/geot.1965.15.1.79
[4]	WONG F S. Slope reliability and response surface method[J]. Journal of Geotechnical Engineering, 1985, 111(1): 32-53. doi: 10.1061/(ASCE)0733-9410(1985)111:1(32)
[5]	朱大勇, 范鹏贤, 郭志昆. 关于Sarma法改进算法的补充[J]. 岩土力学与工程学报, 2006, 25(11): 2343-2345. https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX200611029.htm ZHU Da-yong, FAN Peng-xian, GUO Zhi-kun. Complementarities to modified computation procedure of Sarma method[J]. Chinese Journal of Rock Mechanics and Engineering, 2006, 25(11): 2343-2345. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX200611029.htm
[6]	王旭, 李显忠. 结合Morgenstern-Price法与Sarma法计算岩质-土质混合边坡稳定性[J]. 工程质量, 2010, 28(8): 72-75, 78. https://www.cnki.com.cn/Article/CJFDTOTAL-GCZL201008020.htm WANG Xu, LI Xian-zhong. The stability calculation of the soil-rock combined with Morgenstern-Price method and Sarma method[J]. Quality of Civil Engineering and Construction, 2010, 28(8): 72-75, 78. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GCZL201008020.htm
[7]	康海贵, 李炜. 基于安全系数解析式的边坡稳定评价体系研究[J]. 中国公路学报, 2008, 21(3): 1-5. doi: 10.3321/j.issn:1001-7372.2008.03.001 KANG Hai-gui, LI Wei. Research on evaluated system for slope reliability based on calculation formulae of safety factor[J]. China Journal of Highway and Transport, 2008, 21(3): 1-5. (in Chinese). doi: 10.3321/j.issn:1001-7372.2008.03.001
[8]	梧松, 郑荣跃. 改进局部安全系数法在土坡稳定性分析中的应用[J]. 岩土力学, 2004, 25(11): 1766-1769. doi: 10.3969/j.issn.1000-7598.2004.11.018 WU Song, ZHENG Rong-yue. Application of variable factor of safety method to slope stability analysis[J]. Rock and Soil Mechanics, 2004, 25(11): 1766-1769. (in Chinese). doi: 10.3969/j.issn.1000-7598.2004.11.018
[9]	CHEN W F. Limit Analysis and Soil Plasticity[M]. New York: Elsevier Scientific Publishing Co., 1975.
[10]	姚爱军, 苏永华. 复杂岩质边坡锚固工程地震敏感性分析[J]. 土木工程学报, 2003, 36(11): 34-38. doi: 10.3321/j.issn:1000-131X.2003.11.007 YAO Ai-jun, SU Yong-hua. A method for stability of slope engineering with complicated rock-mass[J]. China Civil Engineering Journal, 2003, 36(11): 34-38. (in Chinese). doi: 10.3321/j.issn:1000-131X.2003.11.007
[11]	苏永华, 赵明华, 邹志鹏, 等. 边坡稳定性分析的Sarma模式及可靠度计算方法[J]. 水利学报, 2006, 37(4): 457-463. doi: 10.3321/j.issn:0559-9350.2006.04.012 SU Yong-hua, ZHAO Ming-hua, ZOU Zhi-peng, et al. Sarma model for slope stability analysis and its reliability degree calculation method[J]. Journal of Hydraulic Engineering, 2006, 37(4): 457-463. (in Chinese). doi: 10.3321/j.issn:0559-9350.2006.04.012
[12]	汪时奇. 一类提高插值算法精度的数据变换法[J]. 上海机械学院学报, 1986, 8(2): 63-68. https://www.cnki.com.cn/Article/CJFDTOTAL-HDGY198603007.htm WANG Shi-qi. A kind of data-transformation methods increasing the accuracy of interpolations[J]. Journal of Shanghai Institute of Mechanical Engineering, 1986, 8(2): 63-68. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-HDGY198603007.htm