Influence of underwater towed system on ship maneuverability
-
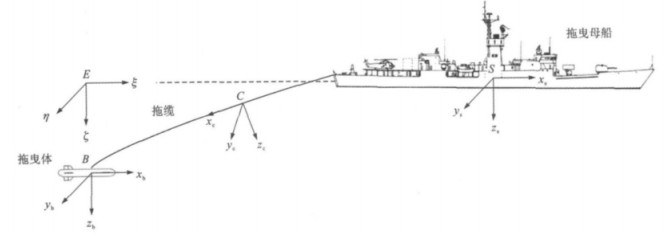
摘要: 将拖曳母船、拖缆和拖曳体视为一个相互作用的整体, 利用耦合边界条件, 将拖缆顶端和底端的张力与其产生的力矩, 分别计入船舶操纵性运动方程和拖曳体六自由度运动方程, 结合拖缆的有限差分方程, 建立了船/缆/体耦合运动模型, 采用数值计算方法, 对比分析了海洋拖曳系统对船舶操纵性产生的影响。计算结果表明: 当计入拖缆和拖曳体耦合影响后, 船舶稳态运动时的速度会降低, 改变量为3%~5%;船舶回转机动时, 速度、回转半径与横摇角会降低, 改变量分别为2%~3%、2%~4%和11%~21%。采用船/缆/体耦合运动模型计算得到的船舶操纵性能符合实际, 可为预报海洋拖曳系统的运动信息提供理论依据。Abstract: Towing ship, towed cable and towed vehicle were treated as an interactional whole system. Using the boundary conditions of the system, the tensions and moments of towed cable's upper end and bottom end were used into the MMG model of towing ship and the equation with six degrees of freedom for towed vehicle respectively. Coupled with the finite difference method of towed cable, a coupling motion model of the whole system was established. A numerical method was developed based on the mode[to predict the influence of underwater towed system on the maneuverability of towing ship. Numerical result indicates that the velocity of towing ship decreases by considering the coupling function of towed cable and towed vehicle in steady motion, and the variation is 3O//oo-5%. In the turning maneuver of towing ship, the velocity, turning radius and roll angle of towing ship reduce, and the variations are 2;-3;/0, 2;-4;//0 and 11%-21% respectively. The influence makes the maneuverability more normal. Moreover, it can be used as the basic theory to predict the behaviors of underwater towed system. 7 tabs, 6 figs, 15 refs.
-
表 1 拖曳母船参数
Table 1. Parameters of towing ship

表 2 拖缆参数
Table 2. Parameters of towed cable

表 3 拖曳体参数
Table 3. Parameters of towed vehicle

表 4 不同船舶工况时拖曳系统的稳态特性
Table 4. Steady-state characteristics of towed system under different engine conditions

表 5 不同舵角时拖曳母船的平均回转半径
Table 5. Turning radii of towing ship at different rudder angles

表 6 速度稳定值比较
Table 6. Comparison of steady velocities

表 7 横摇角稳定值比较
Table 7. Comparison of steady roll angles

-
[1] WU Jia-ming, YE Jia-wei, YANG Cheng, et al. Experimental study on a controllable underwater towed system[J]. Ocean Engineering, 2005, 32(14/15): 1803-1817. [2] CHOI J K, SHIRAISHI T, TANAKA T, et al. Safe operation of an autonomous underwater towed vehicle: towed force monitoring and control[J]. Automation in Construction, 2011, 20(8): 1012-1019. doi: 10.1016/j.autcon.2011.04.002 [3] 朱军, 庞永杰, 徐玉如. 规则波浪中舰船操纵运动计算[J]. 哈尔滨工程大学学报, 2004, 25(1): 1-5. doi: 10.3969/j.issn.1006-7043.2004.01.001ZHU Jun, PANG Yong-jie, XU Yu-ru. Maneuvering prediction of a ship in regualr waves[J]. Journal of Harbin Engin-eering University, 2004, 25(1): 1-5. (in Chinese) doi: 10.3969/j.issn.1006-7043.2004.01.001 [4] 张秀凤, 尹勇, 金一丞. 规则波浪中船舶运动六自由度数学模型[J]. 交通运输工程学报, 2007, 7(3): 40-43. doi: 10.3321/j.issn:1671-1637.2007.03.009ZHANG Xiu-feng, YIN Yong, JIN Yi-cheng. Ship motion mathematical model with six degrees of freedom in regular wave[J]. Journal of Traffic and Transportation Engineering, 2007, 7(3): 40-43. (in Chinese) doi: 10.3321/j.issn:1671-1637.2007.03.009 [5] BUCKHAM B, NAHON M, SETO M, at al. Dynamics and control of a towed underwater vehicle system part Ⅰ: model development[J]. Ocean Engineering, 2003, 30(4): 453-470. doi: 10.1016/S0029-8018(02)00029-X [6] ABLOW C M, SCHECHTER S. Numerical simulation of undersea cable dynamics[J]. Ocean Engineering, 1983, 10(6): 443-457. doi: 10.1016/0029-8018(83)90046-X [7] FENG Z, ALLEN R. Evaluation of the effects of the communication cable on the dynamics of an underwater flight vehicle[J]. Ocean Engineering, 2004, 31(8/9): 1019-1035. [8] PARK H I, JUNG D H, KOTERAYAMA W. A numerical and experimental study on dynamics of a towed low tension cable[J]. Applied Ocean Research, 2003, 25(5): 289-299. doi: 10.1016/j.apor.2004.02.003 [9] GOBAT J I, GROSENBAUGH M A. Time-domain numerical simulation of ocean cable structures[J]. Ocean Engineering, 2006, 33(10): 1373-1400. doi: 10.1016/j.oceaneng.2005.07.012 [10] TEIXEIRA F C, AGUIAR A P, PASCOAL A. Nonlinear adaptive control of an underwater towed vehicle[J]. Ocean Engineering, 2010, 37(13): 1193-1220. doi: 10.1016/j.oceaneng.2010.05.010 [11] NARASIMHAN M, SINGH S N. Adaptive optimal control of an autonomous underwater vehicle in the dive plane using dorsal fins[J]. Ocean Engineering, 2006, 33(3/4): 404-416. [12] GROSENBAUGH M A. Tansient behavior of towed cable systems during ship turning maneuvers[J]. Ocean Engineering, 2007, 34(11/12): 1532-1542. [13] 朱军, 黄若波, 胡忠平. 拖曳系统对舰船操纵性影响计算[J]. 船海工程, 2002(2): 5-10. doi: 10.3963/j.issn.1671-7953.2002.02.002ZHU Jun, HUANG Ruo-bo, HU Zhong-ping. Influence of towed system on ship maneuverability[J]. Ship and Ocean Engineering, 2002(2): 5-10. (in Chinese) doi: 10.3963/j.issn.1671-7953.2002.02.002 [14] 王飞, 黄国樑, 伍生春. 水下拖曳系统缆/船耦合运动模拟[J]. 上海交通大学学报, 2011, 45(4): 570-575. https://www.cnki.com.cn/Article/CJFDTOTAL-SHJT201104023.htmWANG Fei, HUANG Guo-liang, WU Sheng-chun. Dynamic research on the coupling response of cable-towing ship system[J]. Journal of Shanghai Jiaotong University, 2011, 45(4): 570-575. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SHJT201104023.htm [15] VAZ M A, PATEL M H. Three-dimensional behaviour of elastic marine cables in sheared currents[J]. Applied Ocean Research, 2000, 22(1): 45-53. doi: 10.1016/S0141-1187(99)00023-1 -





 下载:
下载: