出行成本对居民出行方式的影响

徐婷; 蓝瑧; 胡大伟; 孙小端; 王伟力

doi:10.19818/j.cnki.1671-1637.2013.01.014

出行成本对居民出行方式的影响

doi: 10.19818/j.cnki.1671-1637.2013.01.014

1.
长安大学汽车学院, 陕西西安 710064
2.
路易斯安那大学土木工程学院, 路易斯安那拉法耶特 70504
3.
西安公路研究院, 陕西西安 710054

基金项目:

国家自然科学基金项目 51108036

中央高校基本科研业务费专项资金项目 CHD2010JC085

中央高校基本科研业务费专项资金项目 CHD2011JC156

中央高校基本科研业务费专项资金项目 CHD2011TD015

详细信息

作者简介:
徐婷(1983-), 女, 江苏常州人, 长安大学讲师, 工学博士, 从事道路交通规划研究

中图分类号: U491.1
计量
- 文章访问数: 987
- HTML全文浏览量: 247
- PDF下载量: 1418
- 被引次数: 0
出版历程
- 收稿日期: 2012-09-15
- 刊出日期: 2013-02-25

Influence of trip cost on trip mode for resident

1.
School of Automobile, Chang'an University, Xi'an 710064, Shaanxi, China
2.
School of Civil Engineering, University of Louisiana, Lafayette 70504, Louisiana, USA
3.
Xi'an Highway Research Institute, Xi'an 710054, Shaanxi, China

More Information

Author Bio:
XU Ting(1983-), female, lecturer, PhD, +86-29-82334425, annabelxu@163.com

摘要

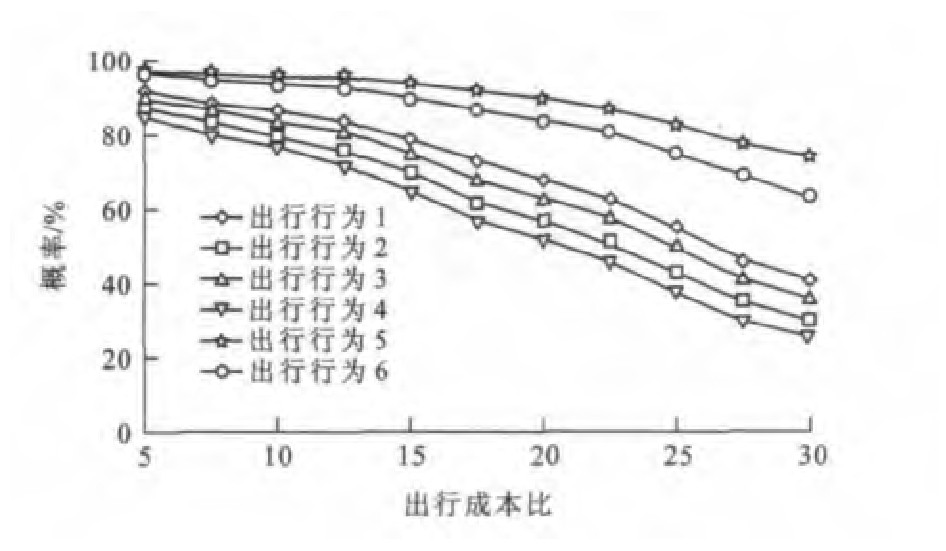
摘要: 以北京市居民出行行为为研究对象, 收集2010年部分区域居民出行基础数据, 使用相关性分析筛选与居民出行方式选择密切相关的影响因素。以小汽车出行方式的效用函数与公共交通出行方式的效用函数的差值构建新效用函数, 选择收入、出行目的、支付方式、出行时间比、出行成本比等作为影响因素, 将小汽车和公共交通出行时间比划分为1:5、1:3、1:1, 分析出行成本对居民出行方式的影响。分析结果表明: 当出行时间比为1:5时, 居民使用小汽车出行对应的弹性值均小于0.1, 出行成本调节缺乏弹性; 当出行时间比为1:3时, 对应的最大弹性值为0.39, 当出行成本比为25时, 40%~50%的出行者继续使用小汽车出行; 当出行时间比为1:1时, 对应的最大弹性值为0.89, 当出行成本比为22时, 40%~50%的出行者继续使用小汽车出行; 当出行时间在1:3和1:1之间, 要使得小汽车的出行分担率为30%, 则出行成本比为至少为5。
- 交通需求管理 /
- 出行方式 /
- 出行成本 /
- 非集计模型 /
- 效用函数 /
- 灵敏度分析
Abstract: The trip behaviors of residents in Beijing city were taken as study subject, the basic trip data of some regions in 2010 were collected, and correlation analysis was used to choose influence factors which were closely related to the mode choice of resident trip. New utility function was constructed by using the difference value of utility functions of public transit trip mode and car trip mode. The salary, trip purpose, payment mode, trip time ratio and trip cost ratio were taken as influence factors. The trip time ratio of car trip mode and public transit trip mode was divided into 3 conditions such as 1:5, 1:3 and 1:1, and the influence of trip cost on trip mode for resident was analyzed. Ayalysis result shows that when trip time ratio is 1:5, all the elastic values of car trip mode are less than 0.1, and trip cost adjustment is invalid. When trip time ratio is 1:3, the maximum elastic value of car trip mode is 0.39. When trip cost ratio is 25, 40%-50% residents will still use cars. When trip time ratio is 1:1, the maximum elastic value of car trip mode is 0.89. When trip cost ratio is 22, 40%-50% residents will still use cars. If trip time ratio is between 1:1 and 1:3, car trip sharing rate is forced down to 30%, trip cost ratio must be 5 at least.
- traffic demand management /
- trip mode /
- trip cost /
- disaggregate model /
- utility function /
- sensitivity analysis

HTML全文

图 1 工况1计算结果

Figure 1. Calculation results of condition 1

下载: 全尺寸图片幻灯片

图 2 工况2计算结果

Figure 2. Calculation results of condition 2

下载: 全尺寸图片幻灯片

图 3 工况3计算结果

Figure 3. Calculation results of condition 3

下载: 全尺寸图片幻灯片

表 1 伴随概率

Table 1. Adjoint probabilities

下载: 导出CSV

表 2 影响因素

Table 2. Influence factors

下载: 导出CSV

表 3 影响因素标定结果

Table 3. Calibration results of influence factors

下载: 导出CSV

表 4 出行时间比为1∶5时的弹性值

Table 4. Elastic values when trip time ratio is 1∶5

下载: 导出CSV

表 5 出行时间比为1∶3时的弹性值

Table 5. Elastic values when trip time ratio is 1∶3

下载: 导出CSV

表 6 出行时间比为1∶1时的弹性值

Table 6. Elastic values when trip time ratio is 1∶1

下载: 导出CSV

参考文献(21)

[1]	刘炳恩, 隽志才, 李艳玲, 等. 居民出行方式选择非集计模型的建立[J]. 公路交通科技, 2008, 25(5): 117-122. https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK200805021.htm LIU Bing-en, JUN Zhi-cai, LI Yan-ling, et al. Development of a multinomial logit model for travel mode choice of resident[J]. Journal of Highway and Transportation Research and Development, 2008, 25(5): 117-122. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK200805021.htm
[2]	北京市交通发展研究中心. 北京市交通发展年度报告(2010)[R]. 北京: 北京市交通发展研究中心, 2011. Beijing Transportation Research Center. Report for transpor-tation development in Beijing(2010)[R]. Beijing: Beijing Transportation Research Center, 2011. (in Chinese).
[3]	帅斌, 林渝钧, 种鹏云. 基于Logit模型的城市低碳交通分担率研究[J]. 武汉理工大学学报: 交通科学与工程版, 2012, 36(1): 74-78. https://www.cnki.com.cn/Article/CJFDTOTAL-JTKJ201201018.htm SHUAI Bin, LIN Yu-jun, CHONG Peng-yun. Urban lowcarbon transport share rate by logit model[J]. Journal of Wuhan Technology: Transportation Science and Engineering, 2012, 36(1): 74-78. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JTKJ201201018.htm
[4]	KAORI M, TAKAMASA A. A logit model for modal choice with a fuzzy logic utility function[J]. Traffic and Transportation Studies, 2000, 4(5): 311-318.
[5]	姚丽亚, 孙立山, 关宏志. 基于分层Logit模型的交通方式选择行为研究[J]. 武汉理工大学学报: 交通科学与工程版, 2010, 34(4): 738-741. https://www.cnki.com.cn/Article/CJFDTOTAL-JTKJ201004025.htm YAO Li-ya, SUN Li-shan, GUAN Hong-zhi. Study on modal split method based on nested logit model[J]. Jornal of Wuhan Technology: Transportation Science and Engineering, 2010, 34(4): 738-741. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JTKJ201004025.htm
[6]	杨励雅, 邵春福, HAGHANI A. 出行方式与出发时间联合选择的分层Logit模型[J]. 交通运输工程学报, 2012, 12(2): 77-83. http://transport.chd.edu.cn/article/id/201202011 YANG Li-ya, SHAO Chun-fu, HANGHANI A. Nested logit model of combined selection for travel mode and departure time[J]. Journal of Traffic and Transportation Engineering, 2012, 12(2): 77-83. (in Chinese). http://transport.chd.edu.cn/article/id/201202011
[7]	龚勃文. 交通方式划分的非集计模型及应用研究[D]. 长春: 吉林大学, 2007. GONG Bo-wen. Study on disaggregate model of traffic mode split and application[D]. Changchun: Jilin University, 2007. (in Chinese).
[8]	HENSHER D, GREME W. Specification and estimation of the nested logit model: alternative normalizations[J]. Transportation Research Part B: Methodological, 2002, 36(1): 1-17. doi: 10.1016/S0191-2615(00)00035-7
[9]	KWIGIZIE V, VALERIAN D, SANDO T. A cross-nested logit model for trip type-mode choice: an application[J]. Advances in Transportation Studies, 2011, 23(2): 29-40.
[10]	李霞, 邵春福, 曲天书, 等. 基于网络广义极值模型的居住地和通勤方式同时选择模型研究[J]. 北京大学学报: 自然科学版, 2010, 46(6): 926-933. https://www.cnki.com.cn/Article/CJFDTOTAL-BJDZ201006012.htm LI Xia, SHAO Chun-fu, QU Tian-shu, et al. Application of network generalized extreme value model to estimate residential location and commute mode choice[J]. Acta Scientiarum Naturalium Universitatis Pekinensis, 2010, 46(6): 926-933. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-BJDZ201006012.htm
[11]	李霞, 高利, 刘玉国. 基于交叉分层Logit的轨道站点周边居住地和出行方式联合选择模型[J]. 吉林大学学报: 工学版, 2011. 41(6):1614-1620. https://www.cnki.com.cn/Article/CJFDTOTAL-JLGY201106020.htm 41(6): 1614-1620. LI Xia, GAO Li, LIU Yu-guo. Simulationous residential location and travel mode choice estimation around rail transit station based on cross-nested logit model[J]. Journal of JilinUniversity: Engineering and Technology Edition, 2011, 41(6): 1614-1620. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JLGY201106020.htm
[12]	张秋萍, 陈义华. 基于非集计模型的交通方式选择研究[J]. 铁道运输与经济, 2010, 32(1): 51-55. https://www.cnki.com.cn/Article/CJFDTOTAL-TDYS201001028.htm ZHANG Qiu-ping, CHEN Yi-hua. Study on traffic mode choice based on disaggregate model[J]. Railway Transport and Economy, 2010, 32(1): 51-55. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-TDYS201001028.htm
[13]	冯忠祥, 袁华智, 刘静, 等. 驾驶人个人特征对行车速度的影响[J]. 交通运输工程学报, 2012, 12(6): 89-96. http://transport.chd.edu.cn/article/id/201206014 FENG Zhong-xiang, YUAN Hua-zhi, LIU Jing, et al. Influence of driver personal attributes on vehicle velocity[J]. Journal of Traffic and Transportation Engineering, 2012, 12(6): 89-96. (in Chinese). http://transport.chd.edu.cn/article/id/201206014
[14]	贾洪飞, 龚勃文, 宗芳. 交通方式选择的非集计模型及其应用[J]. 吉林大学学报: 工学版, 2007, 37(6): 1288-1293. https://www.cnki.com.cn/Article/CJFDTOTAL-JLGY200706013.htm JIA Hong-fei, GONG Bo-wen, ZONG Fang. Disaggregate modeling of traffic mode choice and its application[J]. Journal of Jilin University: Engineering and Technology Edition, 2007, 37(6): 1288-1293. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JLGY200706013.htm
[15]	TRANI A, BAIK H, SWINGLE H, et al. An integrated model for studying small transportation system[J]. Transportation Research Record, 2003(1850): 1-10.
[16]	王浩昌, 杜鹏飞, 赵冬泉, 等. 城市降雨径流模型参数全局灵敏度分析[J]. 中国环境科学, 2008, 28(8): 725-729. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGHJ200808017.htm WANG Hao-chang, DU Peng-fei, ZHAO Dong-quan, et al. Global sensitivity analysis for urban rainfall-runoff model[J]. China Environmental Science, 2008, 28(8): 725-729. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZGHJ200808017.htm
[17]	MULETA K, NICKLOW J. Sensitivity and uncertainty analysis coupled with automatic calibration for a distributed watershed model[J]. Journal of Hydrology, 2005, 306(14): 127-145.
[18]	赵建有, 袁华智, 蹇小平, 等. 城市低收入人群出行方式选择模型[J]. 长安大学学报: 自然科学版, 2012, 32(4): 65-69. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL201204015.htm ZHAO Jian-you, YUAN Hua-zhi, JIAN Xiao-ping, et al. Selection model of trip mode for urban low-income population[J]. Journal of Chang'an University: Natural Science Edition, 2012, 32(4): 65-69. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL201204015.htm
[19]	冯忠祥, 刘静, 李阳阳, 等. 攻击性驾驶行为选择模型及影响因素敏感度分析[J]. 中国公路学报, 2012, 25(2): 106-112. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201202015.htm FENG Zhong-xiang, LIU Jing, LI Yang-yang, et al. Selected model and sensitivity analysis of aggressive driving behavior[J]. China Journal of Highway and Transport, 2012, 25(2): 106-112. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201202015.htm
[20]	LEMP J D, KOCKELMEN K M, DAMIEN P. The continuous cross-nested logit model: formulation and application for departure time choice[J]. Transportation Research Part B: Methodological, 2010, 44(5): 646-661.
[21]	SALEH W, FARRELL S. Implications of congestion charging for departure time choice: work and non-work schedule flexibility[J]. Transportation Research Part A: Policy and Practice, 2005, 39(9): 773-791.