Calculation method for earth pressure behind integral abutment under horizontal reciprocating large displacement
-
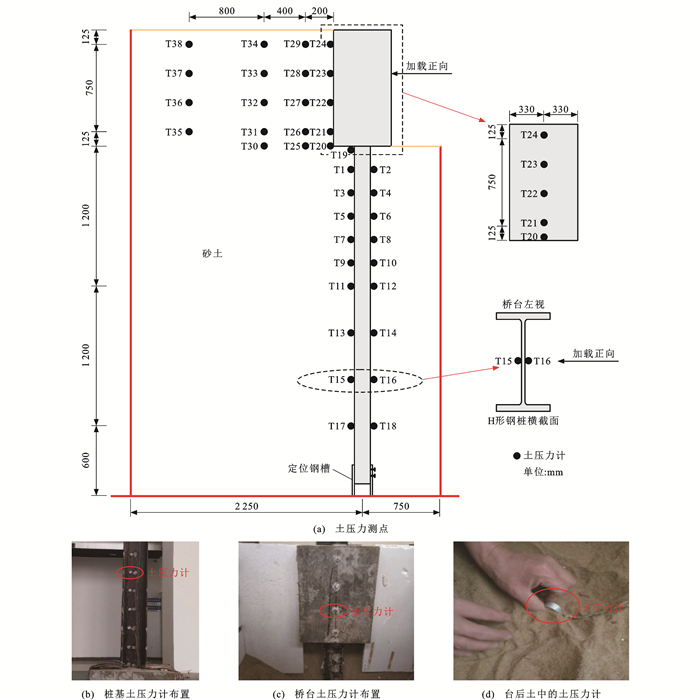
摘要: 为研究强震和温度作用下，整体桥台产生的水平往复大位移对桥台与台后填土相互作用的影响，进行了整体桥台-H形钢桩-土相互作用拟静力试验，并基于试验结果研究了大位移作用下整体桥台后土压力的分布规律；根据台后土压力分布，提出了台后土压力合力作用点位置与加载位移之间的关系式，并在现有研究的基础上给出了改进的整体桥台后土压力计算方法。研究结果表明：正向加载(桥台挤压台后土)时，台后各处土压力随加载位移的增加先增大后减小；台背处和台后20%桥台高度处土压力受桥台位移的影响更大，沿深度方向呈梯形分布；台背处土压力分布中，由于台底H形钢桩的约束，最大土压力位于入土深度0.875 m处，台底位置的土压力则略有减小；台后60%桥台高度和1.4倍桥台高度处土压力受桥台位移影响较小，沿深度方向呈三角形分布；负向加载(桥台背离台后土)时，台后土压力沿深度方向呈三角形分布，且台后各处土压力与加载位移不相关，其值相对于正向加载时可忽略；水平往复大位移作用下，整体桥台后土会产生脱空现象，脱空范围超过桥台高度的37.5%；台后土压力沿纵桥向呈指数型衰减，且相比小位移作用下衰减得更快；台后土压力合力作用点位置随加载位移的增大而逐渐降低，且台后土压力系数与加载位移具有明显的非线性关系，呈现先增大后减小的规律；现有土压力计算方法未考虑桥台位移的影响或认为台后土压力在桥台发生小位移时随桥台位移的增大而增大，发生大位移时则基本不变；提出的土压力拟合公式的判定系数为0.92，计算值与试验值的相对误差为6.2%，可作为现有土压力计算方法的有益补充。Abstract: To analyze the effect of horizontal reciprocating large displacement generated by the abutment on the interaction between the abutment and the backfill behind the abutment under the actions of strong earthquake and temperature, a quasi-static test for the interaction among the integral abutment, H-shaped steel pile, and soil was carried out. On the basis of the test results, the distribution law of the earth pressure behind the integral abutment under the action of large displacement was studied. According to the distribution of the earth pressure behind the abutment, the relational expression between the action point location of the resultant earth pressure behind the abutment and the loading displacement was proposed, and an improved calculation method for the earth pressure behind the integral abutment was given based on the existing research. Research results indicate that when the abutment is loaded in the positive direction (the abutment squeezes the soil behind the abutment), the earth pressure behind the abutment first increases and then decreases as the loading displacement rises. Earth pressures at the abutment back and 20% of the abutment height behind the abutment are highly affected by the abutment displacement and has a trapezoidal distribution along the depth direction. In the earth pressure distribution at the abutment back, due to the constraint of H-shaped steel pile at the bottom of the abutment, the maximum earth pressure is located at a depth of 0.875 m, and the earth pressure at the bottom of the abutment decreases slightly. Earth pressures at 60% of the abutment height and 1.4 times the abutment height behind the abutment are less affected by the abutment displacement and is triangularly distributed along the depth direction. When the abutment is loaded in the negative direction (the abutment deviates from the soil behind the abutment), the earth pressure behind the abutment is triangularly distributed along the depth direction, and the earth pressure behind the abutment has no connection with the loading displacement, and its value can be neglected relative to the positive loading. Under the action of a horizontal reciprocating large displacement, the soil behind the integral abutment will face a void phenomenon, and the void range will exceed 37.5% of the abutment height. The earth pressure behind the abutment reduces exponentially along the longitudinal direction, and it reduces faster than that under the action of a small displacement. The action point location of the resultant earth pressure behind the abutment decreases gradually as the loading displacement increases, and the earth pressure coefficient behind the abutment has an obvious nonlinear relationship with the loading displacement, which is reflected by the law of first increasing and then decreasing. Existing earth pressure calculation methods do not take into account the effect of the abutment displacement or consider that the earth pressure behind the abutment rises with the increase in the abutment displacement when small displacements occur and remains basically unchanged when large displacements occur. The determination coefficient of the proposed earth pressure fitting formula is 0.92, and the relative error between the calculated value and the test value is 6.2%, which can be a useful supplement to the existing earth pressure calculation methods.
-
图 3 正向加载作用下台后土压力沿桥台深度分布
Figure 3. Distributions of earth pressures behind abutment along abutment depth under positive loadings
图 4 负向加载作用下台后土压力沿桥台深度分布
Figure 4. Distributions of earth pressures behind abutment along abutment depth under negative loadings
图 5 台后土压力与加载位移的关系曲线
Figure 5. Relationship curves between earth pressure behind abutment and loading displacement
图 6 正向加载作用下台后土压力沿纵桥向分布
Figure 6. Distributions of earth pressures behind abutment along longitudinal direction under positive loadings
图 9 台后土压力合力作用点位置
Figure 9. Acting point locations of resultant earth pressure behind abutment
图 11 正向加载作用下台后土压力试验值与计算值对比
Figure 11. Comparison of earth pressures behind abutment between test and calculation values under positive loading
表 1 砂土的力学参数
Table 1. Mechanical parameters of sand
参数名称 相对密实度/% 含水率/% 密度/(g·cm-3) 孔隙率 内摩擦角/(°) 泊松比 参数值 53 1.3 1.5 0.8 35 0.3 
下载: 导出CSV
-
[1] 陈宝春, 付毳, 庄一舟, 等. 中国无伸缩缝桥梁应用现状与发展对策[J]. 中外公路, 2018, 38(1): 87-95. https://www.cnki.com.cn/Article/CJFDTOTAL-GWGL201801021.htmCHEN Bao-chun, FU Cui, ZHUANG Yi-zhou, et al. Application status and development countermeasures of jointless bridges in China[J]. Journal of China and Foreign Highway, 2018, 38(1): 87-95. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GWGL201801021.htm [2] SALMAN N N, ISSA M A. Displacement capacities of H-piles in integral abutment bridges[J]. Journal of Bridge Engineering, 2019, 24(12): 04019122. doi: 10.1061/(ASCE)BE.1943-5592.0001482 [3] SALMAN N N, ISSA M A. Calibration and parametric investigation of integral abutment bridges[J]. Engineering Structures, 2021, 227: 111381. doi: 10.1016/j.engstruct.2020.111381 [4] FROSCH R J, LOVELL M D. Long-term behavior of integral abutment bridges[R]. West Lafayette: Joint Transportation Research Program, 2011. [5] RAZMI J, MCCABE M. Analytical and computational modeling of integral abutment bridges foundation movement due to seasonal temperature variations[J]. International Journal of Geomechanics, 2020, 20(3): 04019189. doi: 10.1061/(ASCE)GM.1943-5622.0001622 [6] KIM W, LAMAN J A. Seven-year field monitoring of four integral abutment bridges[J]. Journal of Performance of Constructed Facilities, 2012, 26(1): 54-64. doi: 10.1061/(ASCE)CF.1943-5509.0000250 [7] CIVJAN S A, KALAYCI E, QUINN B H, et al. Observed integral abutment bridge substructure response[J]. Engineering Structures, 2013, 56: 1177-1191. doi: 10.1016/j.engstruct.2013.06.029 [8] HUNTLEY S A, VALSANGKAR A J. Behaviour of H-piles supporting an integral abutment bridge[J]. Canadian Geotechnical Journal, 2014, 51(7): 713-734. doi: 10.1139/cgj-2013-0254 [9] HUNTLEY S A, VALSANGKAR A J. Field monitoring of earth pressures on integral bridge abutments[J]. Canadian Geotechnical Journal, 2013, 50(8): 841-857. doi: 10.1139/cgj-2012-0440 [10] 赵云鹏, 于天来, 毕瑞锋. 整体式桥梁在温度荷载作用下的台后土压力研究[J]. 桥梁建设, 2016, 46(6): 56-60. https://www.cnki.com.cn/Article/CJFDTOTAL-QLJS201606012.htmZHAO Yun-peng, YU Tian-lai, BI Rui-feng. Study of earth pressure behind abutment of integral bridge under action of temperature load[J]. Bridge Construction, 2016, 46(6): 56-60. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-QLJS201606012.htm [11] 洪锦祥, 彭大文, 汪新惠. 整体式桥台桥梁的台后被动土压力研究[J]. 福州大学学报(自然科学版), 2003, 31(6): 721-725. https://www.cnki.com.cn/Article/CJFDTOTAL-FZDZ200306020.htmHONG Jin-xiang, PENG Da-wen, WANG Xin-hui. Passive earth pressure behind abutment of integral abutment bridges[J]. Journal of Fuzhou University (Natural Science Edition), 2003, 31(6): 721-725. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-FZDZ200306020.htm [12] 陈晓冬. 季节性温度荷载下整体式桥台桥梁的台后土压力研究[D]. 福州: 福州大学, 2002.CHEN Xiao-dong. Study on earth pressure of integral abutment bridges under seasonal temperature load[J]. Fuzhou: Fuzhou University, 2002. (in Chinese) [13] 王先前, 郭晓燕, 严国齐. 整体式桥梁力学性能的关键参数分析[J]. 铁道科学与工程学报, 2018, 15(9): 2276-2284. https://www.cnki.com.cn/Article/CJFDTOTAL-CSTD201809014.htmWANG Xian-qian, GUO Xiao-yan, YAN Guo-qi. Parametric study on the mechanical properties of integral bridges[J]. Journal of Railway Science and Engineering, 2018, 15(9): 2276-2284. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-CSTD201809014.htm [14] 彭大文, 陈晓冬, 袁燕. 整体式桥台桥梁台后土压力的季节性变化研究[J]. 岩土工程学报, 2003, 25(2): 135-139.PENG Da-wen, CHEN Xiao-dong, YUAN Yan. Study on seasonal fluctuation of earth pressure behind the abutment[J]. Chinese Journal of Geotechnical Engineering, 2003, 25(2): 135-139. (in Chinese) [15] FIORENTINO G, CENGIZ C, DE LUCA F, et al. Shaking table tests on an integral abutment bridge model: preliminary results[C]//PAPADRAKAKIS M, FRAGIADAKIS M. Proceedings of the 7th International Conference on Computational Methods in Structural Dynamics and Earthquake Engineering. Crete: COMPDYN, 2019: 10.7712/120119.7176.19103. [16] DAVIES L, BULL J, KUCKI T. Lightweight backfill materials in integral bridge construction[J]. Proceedings of the Institution of Civil Engineers—Bridge Engineering, 2014, 167(1): 3-16. [17] 彭大文, 洪锦祥, 郭爱民, 等. 整体式桥台桥梁的动力试验研究[J]. 中国公路学报, 2004, 17(4): 59-63. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200404013.htmPENG Da-wen, HONG Jin-xiang, GUO Ai-min, et al. Dynamic field-test of integral abutment bridge[J]. China Journal of Highway and Transport, 2004, 17(4): 59-63. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL200404013.htm [18] 赵云鹏. 升温荷载作用下整体式桥梁台后土压力计算方法研究[J]. 北方交通, 2017(1): 9-12. https://www.cnki.com.cn/Article/CJFDTOTAL-LNJT201701003.htmZHAO Yun-peng. Research on calculation method for pressure of earth at the back of integral type bridge abutment under the influence of temperature rise load[J]. Northern Communications, 2017(1): 9-12. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-LNJT201701003.htm [19] 王佳佳. 整体式桥台桥梁台后土压力的研究[D]. 哈尔滨: 东北林业大学, 2012.WANG Jia-jia. The research of earth pressure behind the abutment of integral abutment bridge[D]. Harbin: Northeast Forestry University, 2012. (in Chinese) [20] 王明昃, 袁明, 颜东煌. 全无缝桥梁整体式桥台台后主动土压力计算方法研究[J]. 中外公路, 2010, 30(2): 140-143. https://www.cnki.com.cn/Article/CJFDTOTAL-GWGL201002037.htmWANG Ming-ze, YUAN Ming, YAN Dong-huang. Study on calculation method of active earth pressure behind integral abutment of seamless bridge[J]. Journal of China and Foreign Highway, 2010, 30(2): 140-143. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GWGL201002037.htm [21] 黄福云, 林友炜, 程俊峰, 等. 整体式桥台-H形钢桩-土相互作用低周往复拟静力试验[J]. 中国公路学报, 2019, 32(5): 100-114. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201905011.htmHUANG Fu-yun, LIN You-wei, CHENG Jun-feng, et al. Interaction of integral abutment-H-shaped steel pile-soil under reciprocating low-cycle pseudo-static test[J]. China Journal of Highway and Transport, 2019, 32(5): 100-114. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201905011.htm [22] 林上顺, 林友炜, 黄福云, 等. 往复位移作用下整体桥台后土压力计算方法[J]. 中国公路学报, 2019, 32(2): 116-125. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201902013.htmLIN Shang-shun, LIN You-wei, HUANG Fu-yun, et al. Method to calculate earth pressure of backfill of integral abutment bridges under the action of reciprocating longitudinal displacement[J]. China Journal of Highway and Transport, 2019, 32(2): 116-125. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201902013.htm [23] FARAJI S, TING J M, CROVO D S, et al. Nonlinear analysis of integral bridges: finite-element model[J]. Journal of Geotechnical and Geoenvironmental Engineering, 2001, 127(5): 454-461. [24] 石丽峰, 徐明. 整体式桥台地震反应机理分析[J]. 岩土力学, 2014, 35(11): 3289-3297. https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201411038.htmSHI Li-feng, XU Ming. Analysis of seismic response of integral bridge abutments[J]. Rock and Soil Mechanics, 2014, 35(11): 3289-3297. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201411038.htm [25] 黄福云, 陈伟, 徐普, 等. 整体式桥台-H形钢桩-土体系抗震性能试验[J]. 中国公路学报, 2020, 33(9): 180-192. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL202009019.htmHUANG Fu-yun, CHEN Wei, XU Pu, et al. Experimental study on seismic performance of integral abutment-steel H-pile-soil system[J]. China Journal of Highway and Transport, 2020, 33(9): 180-192. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL202009019.htm [26] 刘昌杞. 桩与承台(基础)板连接的设计与施工[J]. 建筑技术, 1993, 24(3): 164-168. https://www.cnki.com.cn/Article/CJFDTOTAL-JZJI199303013.htmLIU Chang-qi. Design and construction of the connection between pile and pile cap (foundation) plate[J]. Architectural Technology, 1993, 24(3): 164-168. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JZJI199303013.htm [27] 钱海敏. 整体式桥台PHC管桩基础的抗震性能试验研究[D]. 福州: 福州大学, 2017.QIAN Hai-min. Experimental investigation on seismic performance of PHC Pile in integral abutment bridge[D]. Fuzhou: Fuzhou University, 2017. (in Chinese) [28] BURKE M P. Design of integral concrete bridges[J]. Concrete International, 1993, 15(6): 37-42. [29] CHEN Y F. Important considerations guidelines and practical details of integral bridges[J]. Journal of Engineering Technology, 1997, 14(1): 16-19. [30] ALIZADEH M H, RASHID A R K, CHIK Z, et al. Investigation of abutment displacement of a full height integral bridges in dense granule backfill[J]. American Journal of Engineering and Applied Sciences, 2010, 3(4): 749-756. [31] DICLELI M. Simplified model for computer-aided analysis of integral bridges[J]. Journal of Bridge Engineering, 2000, 5(3): 240-248. -
点击查看大图
图(11) / 表(1)
计量
- 文章访问数: 338
- HTML全文浏览量: 114
- PDF下载量: 47
- 被引次数: 0
