Effective prestress computation of existing PC simply-supported beam
-
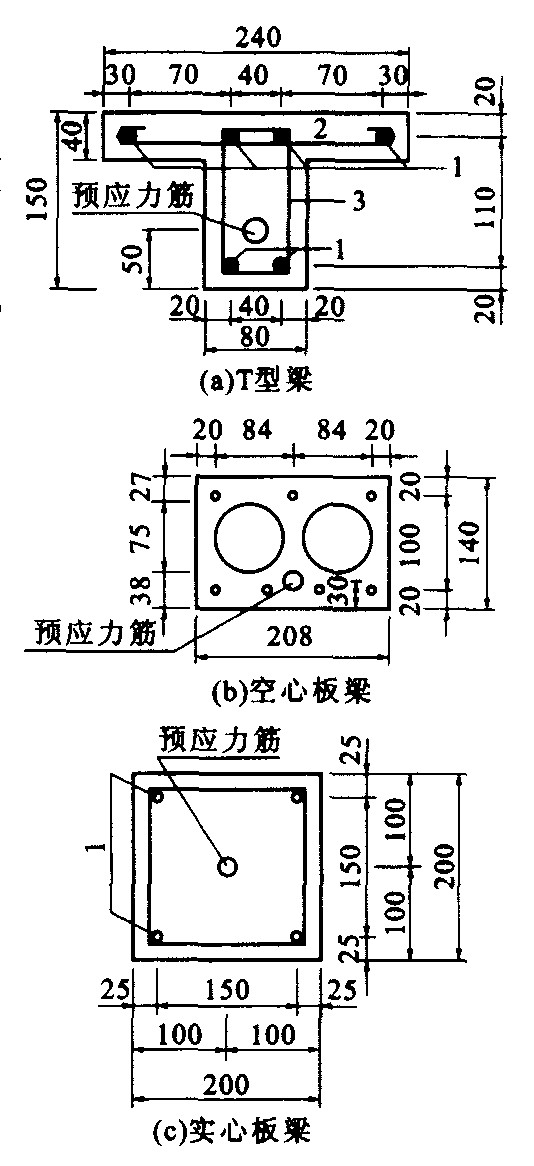
摘要: 为了对在役混凝土桥梁结构永存预应力和实际承载能力进行评估, 用后张法测试混凝土简支梁在不同预应力值作用下的自振频率和在相同预应力值、不同竖向力值作用下的挠度, 以混凝土简支梁的振动基频随预应力作用值的增大而有规律地增大为基础, 回归出简支梁的有效刚度与预应力函数表达式, 计算出PC简支梁的挠度计算值, 并与试验测试挠度值进行了比较。结果表明, 在混凝土受拉区开裂前, T型梁和实心板梁的计算结果误差均不超过10%, 其中实心板梁有80%的测试数据误差在±5%以内; 空心板梁的计算结果误差不超过15.71%, 有73.3%的误差不超过10%;用实测简支梁基频和挠度可推算出简支梁的有效预应力, 可进行预应力混凝土结构的安全评估。Abstract: In order to evaluate the permanent prestress and structure loading capability of existing prestressed concrete bridge, the longitudinal pretensioning force was enforced on the simply-supported concrete beam to gain its natural frequency and deflection by post-tensioning structure method.Based on the relation of its fundamental frequency increasing with its pretension force's increasing, a regression analysis of the data was conducted to develop an equation of its effective stiffness, the computation value and the measure value of its deflection were compared.The analysis results show that the deflection errors of T and rectangular beams are within 10%, the errors of hollow slab beam are within 15.71%, which indicates that using the tested fundamental frequency and deflection can calculate simply-supported beam's effective prestress.
-
Key words:
- bridge engineering /
- PC simply-supported beam /
- vibration frequency /
- deflection /
- effective prestress /
- experiment
-
表 1 T型梁计算值与试验实测值的比较
Table 1. Comparison of calculation values and test values for T beam
截面位置 预应力N/kN 竖向力P/kN 挠度/mm 误差/% 计算值 实测值 L/2 23.66 4.91 0.72 0.75 -4.17 7.11 1.04 0.96 7.69 7.71 1.13 1.16 -2.65 8.91 1.31 1.37 -4.58 9.65 1.41 1.52 -7.80 47.52 13.31 1.85 1.79 3.24 16.77 2.33 2.22 4.72 17.85 2.48 2.37 4.44 18.47 2.56 2.49 2.73 19.47 2.70 2.62 2.96 58.12 22.35 3.08 3.37 -9.42 23.27 3.21 3.43 -6.85 24.01 3.31 3.33 -0.60 24.95 3.44 3.72 -8.14 26.45 3.64 3.99 -9.62 表 2 空心板梁计算值与试验实测值的比较
Table 2. Comparison of calculation values and test values for hollow slab beam
截面位置 预应力N/kN 竖向力P/kN 挠度/mm 误差/% 计算值 实测值 L/2 19.44 1.00 0.45 0.48 -6.67 2.00 0.76 0.84 -10.53 3.33 1.18 1.28 -8.47 4.50 1.54 1.73 -12.34 6.17 2.06 2.27 -10.19 25.32 1.83 0.70 0.59 15.71 3.17 1.11 1.00 9.91 4.50 1.52 1.43 5.92 6.17 2.03 1.92 5.42 7.17 2.34 2.26 3.42 31.26 2.00 0.77 0.78 -1.30 3.33 1.18 1.23 -4.24 4.67 1.60 1.62 -1.25 6.00 2.01 2.12 -5.47 7.33 2.43 2.55 -4.94 表 3 实心板梁计算值与试验实测值的比较
Table 3. Comparison of calculation values and test values for rectangular beam
截面位置 预应力N/kN 竖向力P/kN 挠度/mm 误差/% 计算值 实测值 L/2 60 1.8 0.25 0.23 8.00 2.6 0.35 0.37 -5.71 3.6 0.49 0.50 -2.04 4.8 0.65 0.69 -6.15 5.9 0.81 0.84 -3.70 100 2.8 0.37 0.36 2.70 3.6 0.48 0.47 2.08 4.6 0.61 0.62 -1.64 5.6 0.74 0.75 -1.35 6.6 0.87 0.88 -1.15 140 2.4 0.31 0.31 0.00 3.9 0.50 0.51 -2.00 4.6 0.59 0.61 -3.39 5.6 0.72 0.74 -2.78 6.6 0.85 0.87 -2.35 -
[1] Liu Zi-ming, Wang Bang-mei. Some substances of existing bridge detection and evaluation[J]. Bridge Construction, 2002, 32(3): 1-6. (in Chinese) doi: 10.3969/j.issn.1003-4722.2002.03.001 [2] Liu Yang, Zhang Jian-ren. Reliability assessment of RC bridges throughout service lives[J]. China Journal of Highway and Transport, 2001, 14(2): 61-65. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL202102002.htm [3] Zou Jin-he. Experiments design of modal analysis for vibration system[J]. Physics experimentation, 2004, 24(11): 46-48. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-WLSL202006005.htm [4] Wen Xiang-rong, Zhi Hao, Miao Long-xiu. Research on structural dynamic load identification based on modal analysis method[J]. Journal of Northern Jiaotong University, 2000, 24 (4): 11-14. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GLSJ202103015.htm [5] Deng Rui-qiang, Huang Xiao-qing, Liu Yi-ping, et al. Measurement and modal analysis of the vibration of a bridge in multiple directions[J]. Journal of South China University of Technology(Natural Science Edition), 2003, 31(Sup): 52-55. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-DOUB202106002.htm [6] He Shuan-hai, Guo Qi, Song Yi-fan, et al. Health condition and load carrying capacity evaluation of RC beam based on dynamic testing[J]. Journal of Chang'an University(Natural Science Edition), 2003, 23(6): 36-39. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-FLKX202101020.htm [7] 谢功元. 在役混凝土梁永存预应力试验研究[D]. 西安: 长安大学, 2004. [8] Saiidi M, Douglas B, Feng S. Prestress force effect on vibration frequency of concrete bridges[J]. Journal of Structure Engineering, 1994, 120(7): 2 233-2 241. https://www.cnki.com.cn/Article/CJFDTOTAL-JYGC202001006.htm [9] Zhou Mi, He Shuan-hai, Song Yi-fan. Structural evaluation for beams based on deflection testing[J]. Journal of Chang'an University(Natural Science Edition), 2004, 24(5): 40-42. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SLTJ201904011.htm [10] Yan Dong-huang, Tian Zhong-chu, Li Xue-wen, et al. Finite element method and application for the shrinkage and creep of concrete bridges[J]. China Journal of Highway and Transport, 2004, 17(2): 55-58. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL202102002.htm [11] Guo Quan-quan, Li Zhu, Zhang Shan-yuan. Study on digital tensioning technique in prestressed concrete structure[J]. China Civil Engineering Journal, 2004, 37(7): 13-17. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC202101001.htm -





 下载:
下载: