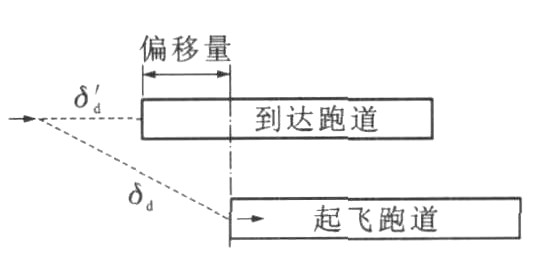
-
摘要: 为了精确评估近距平行跑道容量及其延误, 建立了符合实际运行特点的近距平行跑道运行时空图, 借鉴经典单跑道混合运行模式下的容量和延误水平计算模型, 对离港航班采取插缝放行, 利用双排队系统理论与逻辑推理方法, 分别建立了近距平行跑道容量与平均延误水平计算模型, 并运用仿真方法对模型进行了验证, 将模型计算结果和仿真结果进行了比较。对比结果表明: 跑道容量差异率为0.58%, 最大平均延误和队列差异率分别为1.7%和1.4%, 因此, 建立的模型客观反映了近距平行跑道系统实际运行状况, 是可行的。Abstract: In order to accurately assess the capacity and delay of closely spaced parallel runway, a time-space diagram was established in accord with realistic operational features, the calculation models of the capacity and delay under the classic mixed operation mode of single runway were referred, departure flights were allowed in the intervals of arrival flights, double queue system theory and logical discursion method were utilized, the calculation models of the capacity and average delay for closely spaced parallel runway were built separately, simulation method was used to check the models, and the calculation result and the simulation result were compared.Comparison result shows that the differentia rate of runway capacity is 0.58%, the maximum differentia rates of the average delay and queue are 1.7% and 1.4% respectively, so the new models reflect the realistic operational situation of closely spaced parallel runway, and are feasible.
-
Key words:
- traffic planning /
- closely spaced parallel runway /
- capacity /
- delay /
- calculation model /
- simulation
-
表 1 机型配对概率
Table 1. Matching probabilities of aircraft types
% P1=PhPh P2=PhPm P3=PmPh P4=PmPm 21.62 24.88 24.88 28.62 
下载: 导出CSV
表 2 容量仿真结果对比
Table 2. Comparison of capacity simulation results
容量/架·h-1 原航班计划 增加20%航班后 增加30%航班后 增加35%航班后 增加40%航班后 进港高峰架次 27 31 34 35 35 离港高峰架次 28 29 30 30 30 高峰航班起降架次 55 60 64 65 65 进港航班总架次 346 415 450 467 484 离港航班总架次 351 421 456 474 491
下载: 导出CSV
表 3 平均延误水平对比
Table 3. Comparison of average delays
对比参数 模型计算结果 仿真结果 进港航班 平均延误/s 78.02 79.34 等待队列/架 0.70 0.71 离港航班 平均延误/s 114.21 115.47 等待队列/架 0.81 0.82
下载: 导出CSV
-
[1] HARRIS R M. Models for runway capacity analysis[R]. Washington DC: Mitre Corporation, 1969. [2] NEWWELL G F. Airport capacity and delays[J]. Transportation Science, 1979, 13 (3): 201-241. doi: 10.1287/trsc.13.3.201 [3] HORONJEFF R M, MCKELVEY F X. Planning and Designof Airports[M]. San Trancisco: McGraw-Hill Professional, 1993. [4] MUDD L E. Airport capacity and delay[R]. Washington DC: Department of Transportation, Federal Aviation Administration, 1983. [5] LEE D A, NELSON C, SHAPIRO G. The aviation system analysis capability airport capacity and delay models[R]. Wash-ington DC: NASA, 1998. [6] 胡明华, 刘松, 苏兰根. 基于统计分析的单跑道容量估计模型研究[J]. 数据采集与处理, 2000, 15 (1): 74-77. https://www.cnki.com.cn/Article/CJFDTOTAL-SJCJ200001020.htmHU Ming-hua, LIU Song, SU Lan-gen. Research of airport capacity estimation model based on statistic analysis[J]. Journal of Data Acquisition & Processing, 2000, 15 (1): 74-77. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SJCJ200001020.htm [7] 蒋兵, 胡明华, 田勇, 等. 机场跑道容量评估模型和估计方法的进一步研究[J]. 交通运输工程学报, 2003, 3 (2): 80-83. http://transport.chd.edu.cn/article/id/200302015JIANG Bing, HU Ming-hua, TIAN Yong, et al. Further research of airport runway capacity evaluation[J]. Journal of Traffic and Transportation Engineering, 2003, 3 (2): 80-83. (in Chinese) http://transport.chd.edu.cn/article/id/200302015 [8] 余江, 王大海, 蒲云. 跑道容量的概率模型及容量曲线[J]. 交通运输工程学报, 2002, 2 (4): 99-102. http://transport.chd.edu.cn/article/id/200204021YUJiang, WANG Da-hai, PU Yun. Runway capacity probility model and capacity curve[J]. Journal of Traffic and Transportation Engineering, 2002, 2 (4): 99-102. (in Chinese) http://transport.chd.edu.cn/article/id/200204021 [9] 理查德德纽弗威尔, 阿米第R欧都尼. 机场系统: 规划、设计和管理[M]. 北京: 中国民航出版社, 2006. [10] 《运筹学》教材编写组. 运筹学[M]. 北京: 清华大学出版社, 2005. [11] 中国民用航空总局. 中国民用航空空中交通管理规则[R]. 北京: 中国民用航空总局, 1999. -
点击查看大图
图(4) / 表(3)
计量
- 文章访问数: 289
- HTML全文浏览量: 93
- PDF下载量: 577
- 被引次数: 0
