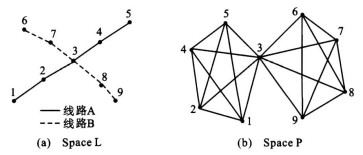
-
摘要: 从局部角度与整体角度对城市轨道交通网络性能进行了综合分析。从局部角度, 分析了轨道交通网络二线、三线和四线的基本组成功能单元, 并提出采用换乘次数、路网吸引区覆盖强度和换乘站的负荷强度等指标对各功能单元进行评价。从整体角度, 运用复杂网络理论, 在SpaceP拓扑空间中, 采用节点的度、群集系数和平均路径长度等参数来研究轨道交通网络的性能, 并从网络拓扑本身来认识网络特性。研究结果表明: 轨道交通网络最基本的形式为放射、交叉、环形, 三角与环形、三角与三角及多三角都是较为理想的复合形式; 在北京市轨道交通网络中, 随节点的度增大, 其概率分布呈先增加后减小趋势, 群集系数等于1的站点较多, 网络中直达站点和换乘1次就可完成出行站点之和超过了总数的80%, 可认为该网络出行较便捷。Abstract: The characteristics of urban rail network were comprehensively studied from part and overall perspectives.From part perspective, the 2-line, 3-line and 4-line basic units of urban rail transit network were analyzed.Indicators such as transfer times, coverage intensity of attraction zone and load of transfer station were proposed to evaluate each basic unit.From overall perspective, complex network theory was used, and in topological Space P, indicators such as the degree, clustering coefficient of node and average path length were proposed to characterize overall network, and network characteristics were understood from topological network.Analysis result shows that the basic units of urban rail transit network are radiation, cross and ring.Triangle with ring, triangle with triangle and multiple triangles are relatively ideal complex forms.In the urban rail network of Beijing, with the increase of node degree, its probability distribution follows the trend of first increasing and then decreasing.The clustering coefficients of many stations are 1, and travels with no more than one time transfer are more than 80%, so the current rail network of Beijing is comparatively convenient.
-
图 8 节点间最短路径长度累计分布概率
Figure 8. Cumulative probability distribution of shortest path lengths between nodes
表 1 两线基本功能单元图示及指标计算
Table 1. Graphs and indicator calculations of 2-line basic units
表 2 三线基本功能单元图示及指标计算
Table 2. Graphs and indicator calculations of 3-line basic units
表 3 四线基本功能单元圉示及指标计算
Table 3. Graphs and indicator calculations of 4-line basic units
-
[1] 沈景炎. 城市轨道交通线网规划的结构形态基本线形和交点计算[J]. 城市轨道交通研究, 2008, 11(6): 5-10. doi: 10.3969/j.issn.1007-869X.2008.06.002SHEN Jing-yan. Basic liner structure and intersection's calculation of URT network structure[J]. Urban Mass Transit, 2008, 11(6): 5-10. (in Chinese) doi: 10.3969/j.issn.1007-869X.2008.06.002 [2] 李海峰, 王炜. 轨道交通网络形态研究[J]. 规划师, 2006, 22(5): 85-88. doi: 10.3969/j.issn.1006-0022.2006.05.022LI Hai-feng, WANG Wei. Study on the rail transit network configuration[J]. Planners, 2006, 22(5): 85-88. (in Chinese) doi: 10.3969/j.issn.1006-0022.2006.05.022 [3] 王忠强, 黎青松, 陈旭梅. 轨道交通路网基本图式研究[J]. 西南交通大学学报, 2000, 35(3): 288-292. doi: 10.3969/j.issn.0258-2724.2000.03.016WANG Zhong-qiang, LI Qing-song, CHEN Xu-mei. Research on fundamental pattern of urban rail transit network[J]. Journal of Southwest Jiaotong University, 2000, 35(3): 288-292. (in Chinese) doi: 10.3969/j.issn.0258-2724.2000.03.016 [4] 王忠强. 城市轨道交通系统路网规划[D]. 成都: 西南交通大学, 1999.WANG Zhong-qiang. System network planning of urban rail transit[D]. Chengdu: Southwest Jiaotong University, 1999. (in Chinese) [5] 孙壮志. 城市交通网络形态特征分形计量研究[J]. 交通运输系统工程与信息, 2007, 7(1): 29-38. doi: 10.3969/j.issn.1009-6744.2007.01.005SUN Zhuang-zhi. The study of fractal approach to measure urban rail transit network morphology[J]. Journal of Transportation Systems Engineering and Information Technology, 2007, 7(1): 29-38. (in Chinese) doi: 10.3969/j.issn.1009-6744.2007.01.005 [6] 李进, 马军海. 城市地铁网络复杂性研究[J]. 西安电子科技大学学报: 社会科学版, 2009, 19(2): 51-55. doi: 10.3969/j.issn.1008-472X.2009.02.009LI Jin, MA Jun-hai. Research of complexity of urban subway network[J]. Journal of Xidian University: Social Science Edition, 2009, 19(2): 51-55. (in Chinese) doi: 10.3969/j.issn.1008-472X.2009.02.009 [7] 刘锐, 严宝杰, 黄志鹏. 城市公共交通网络的复杂性分析[J]. 交通运输系统工程与信息, 2009, 9(3): 17-22. doi: 10.3969/j.issn.1009-6744.2009.03.003LIU Rui, YAN Bao-jie, HUANG Zhi-peng. Complexity analysis of urban public transit network[J]. Journal of Transportation Systems Engineering and Information Technology, 2009, 9(3): 17-22. (in Chinese) doi: 10.3969/j.issn.1009-6744.2009.03.003 [8] 何胜学, 范炳全. 从公交线网的生成机理看复杂网络的多样性[J]. 系统工程学报, 2007, 22(6): 599-606. doi: 10.3969/j.issn.1000-5781.2007.06.007HE Sheng-xue, FAN Bing-quan. From unban transit networks to various complex networks[J]. Journal of Systems Engineering, 2007, 22(6): 599-606. (in Chinese) doi: 10.3969/j.issn.1000-5781.2007.06.007 [9] 赵金山, 狄增如, 王大辉. 北京市公共汽车交通网络几何性质的实证研究[J]. 复杂系统与复杂性科学, 2005, 2(2): 45-48. https://www.cnki.com.cn/Article/CJFDTOTAL-FZXT200502008.htmZHAO Jin-shan, DI Zeng-ru, WANG Da-hui. Empirical research on public transport network of Beijing[J]. Complex Systems and Complexity Science, 2005, 2(2): 45-48. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-FZXT200502008.htm [10] 管志忠, 刘永明. 图论中最短路问题的MATLAB程序实现[J]. 安庆师范学院学报: 自然科学版, 2007, 13(1): 26-29. https://www.cnki.com.cn/Article/CJFDTOTAL-AQSX200701008.htmGUAN Zhi-zhong, LIU Yong-ming. MATLAB program of the shortest path problem of graph theory[J]. Journal of Anqing Teachers College: Natural Science Edition, 2007, 13(1): 26-29. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-AQSX200701008.htm -
下载:
点击查看大图
图(8) / 表(3)
计量
- 文章访问数: 732
- HTML全文浏览量: 78
- PDF下载量: 585
- 被引次数: 0
