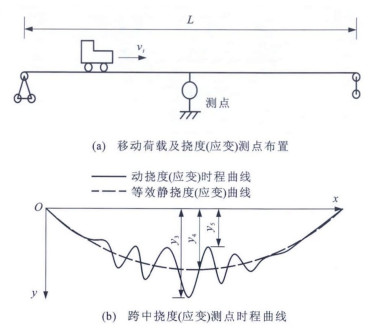
-
摘要: 以公路简支梁桥为研究对象, 采用四自由度的二分之一车辆模型, 建立了车桥耦合振动方程, 计算了不同车速下桥梁跨中截面的动挠度和应变时程曲线。对比了传统定义法、试验测试法、现行规范法的冲击系数计算值, 对前2种方法进行了修正, 获得了桥梁结构的最大动效应值, 并根据主梁的最大活载内力计算原理, 引入数值加权的概念对前2种方法进行了加权计算。分析结果表明: 传统定义法和试验测试法计算的冲击系数值比《公路桥涵设计通用规范》 (JTG D60—2004) 计算值小; 由时程曲线上最大动效应处得到的冲击系数平均值约是前2种方法计算值的2倍, 且其最大值比规范法计算值小37%;基于传统定义法的挠度冲击系数值大于应变冲击系数值, 而试验测试法得到的挠度冲击系数值普遍小于应变冲击系数值; 基于传统定义法和加权法的挠度冲击系数计算值比规范值大16%;试验测试法和加权法相结合的冲击系数计算方法考虑了移动荷载对整个桥梁冲击的历程效应, 计算比较稳定。Abstract: A simply supported beam highway bridge was taken as an example, the half vehicle model with four-degree-of-freedom was employed to set up vehicle-bridge coupled vibration function, and the time-history curves of dynamic deflection and strain at mid-span under different vehicle velocities were calculated.The impact factors (IM) calculated by traditional definition method, experiment method and current code provisions were compared, the first two methods were revised to reflect the maximum dynamic response of bridge.According to the calculation principle of the maximum internal force for girder under moving load, the weighted method was utilized to replenish the first two methods.Analysis result shows that the IMs calculated by traditional definition and experiment methods are less than those in General Code for Design of Highway Bridges and Culverts (JTG D60—2004).The average value of IMs obtained at the maximum dynamic response of time-history curves doubles the first two methods, and its maximum IM is 37%less than code provision.Deflection IMs calculated by traditional definition method are greater than strain IMs, but deflection IMs calculated by experiment method are mostly less than strain IMs.The IM calculated by traditional definition method and weighted method is 16% greater than code provision.The weighted method combining with experiment method reflects the whole impact course of bridge caused by moving load, and it is stable when calculating IM.
-
Key words:
- bridge engineering /
- impact factor /
- vehicle-bridge coupled vibration /
- weighted method /
- deflection /
- bending moment
-
图 1 移动荷载下简支梁跨中挠度(应变) 曲线
Figure 1. Deflection (strain) curves at mid-span of simply supported beam under moving load
图 2 四自由度二分之一车辆分析模型
Figure 2. Analytical model of half vehicle with four-degree-of-freedom
图 3 简支梁跨中挠度和应变曲线
Figure 3. Deflection and strain curves at mid-span of simply supported beam
-
[1] 许华东. 车辆作用下桥梁冲击系数分析[J]. 重庆交通大学学报: 自然科学版, 2013, 32 (1): 5-8. doi: 10.3969/j.issn.1674-0696.2013.01.02XU Hua-dong. Analysis of bridge impact coefficient with vehicles[J]. Journal of Chongqing Jiaotong University: Natural Science, 2013, 32 (1): 5-8. (in Chinese). doi: 10.3969/j.issn.1674-0696.2013.01.02 [2] 蒋培文, 贺拴海, 宋一凡, 等. 简支梁车桥耦合振动及其影响因素[J]. 长安大学学报: 自然科学版, 2013, 33 (1): 59-66. doi: 10.3969/j.issn.1671-8879.2013.01.011JIANG Pei-wen, HE Shuan-hai, SONG Yi-fan, et al. Vehiclebridge coupled vibration and its influencing factors of simple beam[J]. Journal of Chang'an University: Natural Science Edition, 2013, 33 (1): 59-66. (in Chinese). doi: 10.3969/j.issn.1671-8879.2013.01.011 [3] 韩万水, 王涛, 李永庆, 等. 基于模型修正梁格法的车桥耦合振动分析系统[J]. 中国公路学报, 2011, 24 (5): 47-55. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201105010.htmHAN Wan-shui, WANG Tao, LI Yong-qing, et al. Analysis system of vehicle-bridge coupling vibration with grillage method based on model updating[J]. China Journal of Highway and Transport, 2011, 24 (5): 47-55. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201105010.htm [4] 丁勇, 谢旭, 苟昌焕, 等. 钢桥交通振动计算方法与动力特性研究[J]. 浙江大学学报: 工学版, 2012, 46 (6): 1107-1114. doi: 10.3785/j.issn.1008-973X.2012.06.022DING Yong, XIE Xu, GOU Chang-huan, et al. Research on computational method and dynamic characteristics of traffic vibration of steel bridge[J]. Journal of Zhejiang University: Engineering Science, 2012, 46 (6): 1107-1114. (in Chinese). doi: 10.3785/j.issn.1008-973X.2012.06.022 [5] 刘永健, 刘世忠, 米静, 等. 双层公路钢桁梁桥车桥耦合振动[J]. 交通运输工程学报, 2012, 12 (6): 20-28. doi: 10.3969/j.issn.1671-1637.2012.06.004LIU Yong-jian, LIU Shi-zhong, MI Jing, et al. Vehiclebridge coupled vibration of highway double-deck steel truss bridge[J]. Journal of Traffic and Transportation Engineering, 2012, 12 (6): 20-28. (in Chinese). doi: 10.3969/j.issn.1671-1637.2012.06.004 [6] 殷新锋, 方志. 车辆制动作用下的车辆-路面-桥梁系统随机振动分析[J]. 计算力学学报, 2010, 27 (5): 936-941. https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG201005033.htmYIN Xin-feng, FANG Zhi. Random vibration analysis of vehicle-pavement-bridge system under vehicle braking[J]. Chinese Journal of Computational Mechanics, 2010, 27 (5): 936-941. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG201005033.htm [7] YANG Y B, LIAO S S, LIN B H. Impact formulas for vehicles moving over simple and continuous beams[J]. Journal of Structure Engineering, 1995, 121 (11): 1644-1650. doi: 10.1061/(ASCE)0733-9445(1995)121:11(1644) [8] 施尚伟, 杜松, 李莹雪. 桥梁冲击系数随机性分析[J]. 重庆交通大学学报: 自然科学版, 2012, 31 (3): 377-379, 393. https://www.cnki.com.cn/Article/CJFDTOTAL-CQJT201203007.htmSHI Shang-wei, DU Song, LI Ying-xue. Randoms analysis of bridge impact coefficient[J]. Journal of Chongqing Jiaotong University: Natural Science, 2012, 31 (3): 377-379, 393. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-CQJT201203007.htm [9] 龙建旭, 石东艳. 基于移动最小二乘拟合大跨结构动载实验冲击系数分析[J]. 四川建筑, 2011, 31 (2): 134-135, 137. doi: 10.3969/j.issn.1007-8983.2011.02.055LONG Jian-xu, SHI Dong-yan. Impact factor analysis of dynamic test for long-span bridge using least-square method[J]. Sichuan Architecture, 2011, 31 (2): 134-135, 137. (in Chinese). doi: 10.3969/j.issn.1007-8983.2011.02.055 [10] KIM Y J, TANOVIC R, WIGHT R G. Recent advances in performance evaluation and flexural response of existing bridges[J]. Journal of Performance of Constructed Facilities, 2009, 23 (3): 190-200. doi: 10.1061/(ASCE)CF.1943-5509.0000007 [11] 李伟钊, 张巍, 王宗林, 等. 一种基于低通滤波的公路简支梁桥实测冲击系数计算方法[J]. 振动与冲击, 2012, 31 (20): 46-50. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201220010.htmLI Wei-zhao, ZHANG Wei, WANG Zong-lin, et al. A method for calculation of tested impact factor of a simply supported girder bridge based on low-pass filtering[J]. Journal of Vibration and Shock, 2012, 31 (20): 46-50. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201220010.htm [12] 李炜明, 朱宏平, 张俊兵, 等. 车-桥系统在路面随机谱激励下放大效应的归类分析[J]. 中国公路学报, 2010, 23 (5): 58-63, 83. doi: 10.3969/j.issn.1001-7372.2010.05.009LI Wei-ming, ZHU Hong-ping, ZHANG Jun-bing, et al. Amplification effect classification analysis on vehicle-bridge system under road surface random excitation[J]. China Journal of Highway and Transport, 2010, 23 (5): 58-63, 83. (in Chinese). doi: 10.3969/j.issn.1001-7372.2010.05.009 [13] 周新平, 宋一凡, 贺拴海. 公路曲线梁桥车桥耦合振动数值分析[J]. 长安大学学报: 自然科学版, 2009, 29 (6): 41-46. doi: 10.3321/j.issn:1671-8879.2009.06.010ZHOU Xin-ping, SONG Yi-fan, HE Shuan-hai. Numerical analysis for coupled vibration of vehicle-bridge on highway curved-bridge[J]. Journal of Chang'an University: Natural Science Edition, 2009, 29 (6): 41-46. (in Chinese). doi: 10.3321/j.issn:1671-8879.2009.06.010 [14] 施颖, 宣纪明, 宋一凡. 不平整度桥面下连续梁桥车桥耦合振动分析[J]. 桥梁建设, 2009 (6): 15-18, 22. https://www.cnki.com.cn/Article/CJFDTOTAL-QLJS200906003.htmSHI Ying, XUAN Ji-ming, SONG Yi-fan. Analysis of vehiclebridge coupling vibration of continuous girder bridge under uneven deck[J]. Bridge Construction, 2009 (6): 15-18, 22. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-QLJS200906003.htm [15] DENG L, CAI C S. Development of dynamic impact factor for performance evaluation of existing multi-girder concrete bridges[J]. Engineering Structures, 2010, 32 (1): 21-31. doi: 10.1016/j.engstruct.2009.08.013 [16] MA Z, CHAUDHURY S, MILLAM J L, et al. Field test and 3DFE modeling of decked bulb-tee bridges[J]. Journal of Bridge Engineering, 2007, 12 (3): 306-314. doi: 10.1061/(ASCE)1084-0702(2007)12:3(306) [17] MOGHIMI H, RONAGH H R. Development of a numerical model for bridge-vehicle interaction and human response to traffic-induced vibration[J]. Engineering Structures, 2008, 30 (12): . -
下载:
点击查看大图
图(4) / 表(3)
计量
- 文章访问数: 904
- HTML全文浏览量: 128
- PDF下载量: 1071
- 被引次数: 0
