Bi-level programming model of timing optimization for multiple bus priority intersection
-
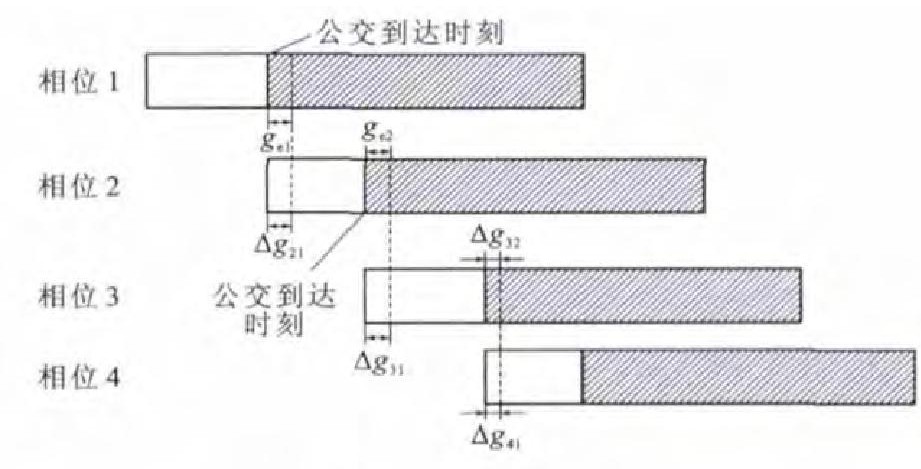
摘要: 公交优先感应信号控制会对非优先相位车辆产生不利影响, 为了均衡非优先相位的损失, 降低交叉口及下游公交站台的乘客延误, 建立了多路公交优先交叉口配时优化的双层规划模型。上层模型描述了基于交叉口延误以及下游公交站台乘客排队延误的优先策略时长优化, 下层模型在计算交叉口停驶-加速延误的基础上描述了各相位绿时损失的均衡过程, 以优先策略时长为决策变量, 将优先策略的影响引入到下层模型。利用Gauss-Seidel迭代法设计了求解算法, 并进行了实例分析。分析结果表明: 配时优化后, 周期内交叉口和下游站台乘客的总延误减少了23 576.12s, 同时交叉口车辆停驶-加速延误减少了62.87s, 双层规划模型在公交优先的前提下保障了交叉口的整体效益。Abstract: Induction signal control based on bus priority had negative effects on vehicles in nonpriority phases. In order to equilibrate the loss of non-priority phases, as well as to reduce the passenger delay at the intersection and the downstream stations, a bi-level programming model of signal timing optimization for multiple bus priority intersection was proposed. In the upper model, priority strategies were optimized based on the intersection delay and the passenger queuing delay at downstream stations. In the lower model, the equilibrium process of green loss was described based on the calculation of acceleration-deceleration delay at the intersection. With priority strategies as decision-making variables, the influence of priority strategies was introduced to the lower model. The solution algorithm was designed by using the Gauss-Seidel iterative method. An application example was analyzed. Analysis result indicates that after signal timing optimization, total passenger delays at the intersection and downstream stations in the period reduces by 23 576.12 s. Besides, the vehicle acceleration-deceleration delay at the intersection reduces by 62.87s. Bi-level programming model ensures the overall efficiency at the intersection under consideration of bus priority.
-
0. 引言
公交信号优先可以减少公交车在交叉口的延误, 提高公交车运行效率, 对增加公交吸引力, 改善公交服务水平有很大的潜力, 但公交信号优先也对非优先相位造成不利影响。Wu等针对不同的公交信号需求设置了3种不同运行特征的预处理信号, 并探讨了3种预处理信号的配时方法和优先车辆与非优先车辆的延误计算方法[1-2]; Wahlstedt利用微观仿真模型分析了公交优先对公交车和社会车辆的影响, 仿真结果表明公交优先缩短了公交出行时间, 但同时也增加了对向穿越车流的出行时间[3]; Liu等利用解析法分析了绿灯启亮提前和延长绿灯时间2种公交优先策略, 图解积分表明在考虑延误影响时, 可以利用D/D/1排队模型来描述2种优先策略对公交车和社会车辆的影响[4]; Zhou等同时考虑了公交车和社会车辆的效益, 利用并行遗传算法对单一公交信号优先交叉口的相位设置、周期时长、绿信比进行优化, 提出了社会车辆影响最小的公交信号优先配时方案[5]; 李劲夫以车辆出行者的人均延误、行人过街平均延误和停车率最小为目标, 建立了信号周期的多目标优化模型, 并设计了模型的粒子群算法[6]; 李淑庆等考虑人流密度对步行速度的影响以及出行费用与时间的换算关系, 将公交出行的路段阻抗、节点阻抗与费用阻抗统一换算为时间, 建立了一体化公交网络的均衡配流模型[7]; Kim等提出了多路公交优先的信号控制方法, 并利用仿真模型分析了不同优先策略在各种网络条件下的效益[8]; 柏海舰等针对公交调度问题, 以最大同时到站次数和最大同时到站车辆数量为优化目标, 以末车定时、站点允许排队长度、服务质量、线路服务时间跨度为约束条件, 建立了公交时刻优化方法[9]; Ma等将多路公交优先请求队列的服务描述为一个多阶段决策过程, 针对不同公交占有率水平、公交时刻表偏差和交通需求提出了多路优先请求的动态规划模型[10-11]; 孙煦等利用遗传算法定量求解公交优先交叉口配时优化的双层模型, 在保障交叉口交通顺畅的前提下实现了公交优先[12]; He等针对多路公交优先的孤立交叉口, 将优先信号控制转换为一个多项式可解的网络切割问题, 并设计了多路优先请求下的启发式算法[13]。上述研究主要分析了公交信号优先对交叉口内的公交车和社会车辆的影响, 但对公交优先控制下的绿时损失均衡考虑较少, 尤其对于多路公交优先控制, 较大的绿时损失容易造成拥堵和相位紊乱。另外, 研究也缺乏对交叉口与下游站台的协调考虑。
本文分析了基于公交相位延长绿灯时间(Green Extension, GE)、缩短红灯时间(Red Truncation, RT) 和插入优先相位(Phase Insertion, PI) 的多路公交优先请求控制策略绿时损失的均衡方法, 以车辆线性到达为基础, 对各种优先控制策略在4相位的非饱和交叉口进行延误分析。以交叉口与下游优先公交站台乘客排队延误最小为上层目标, 以交叉口车辆停驶-加速延误最小为下层目标, 以优先策略时长为决策变量, 建立了配时优化的双层规划模型, 以较小的上层损失保障了交叉口的整体效益。
1. 多路公交优先的感应控制策略
公交优先策略势必会对相邻相位造成绿时损失, 为了保障相邻相位车辆正常通行, 须将公交优先产生的不利影响分摊到整个周期, 以保持周期稳定。在考虑绿时损失均衡过程中, 需要压缩非优先相位的绿时[14], 但仅压缩非优先相位的绿时不利于提高绿时利用效率, 且与实际相位之间承接不相吻合, 因而在压缩非优先相位绿时的同时, 需向相邻相位拓展绿时。图 1~9为各种优先控制策略下的绿时损失均衡分析, 图中阴影区表示红灯, 空白区表示绿灯。t为公交到达时刻; gi为相位i的有效绿灯时间; gei为相位i延长绿灯时间; gmini为相位i的最小绿灯时间; ri为相位i的有效红灯时间; rsi为相位i缩短红灯时间; gpi为相位i插入相位时长; Δgi1为相位i左端绿时变化量; Δgi2为相位i右端绿时变化量。
图 7 同一时刻GE-PI策略的绿时损失均衡Figure 7. Green loss equilibrium of GE-PI strategy at same time
图 8 同一时刻PI-RT策略的绿时损失均衡Figure 8. Green loss equilibrium of PI-RT strategy at same time
图 9 同一时刻PI-PI策略的绿时损失均衡Figure 9. Green loss equilibrium of PI-PI stratgey at same time1.1 不同时刻不同相位多路公交优先请求
检测公交到达时, 分别在相位1与相位2绿时执行的末端有公交优先请求, 而剩余绿时不足以让公交通过, 在保障其余相位最小绿时的前提下, 对相位1、2采用如图 1所示相继延长绿时的控制策略(GE-GE), 表示为
检测公交车到达时, 分别在相位1绿时执行末端与相位4红时执行末端有公交优先请求, 在保障其余相位最小绿时的前提下, 对相位1与相位4分别采用如图 2所示延长绿时与缩短红时的控制策略(GE-RT), 表示为
检测公交车到达时, 分别在相位3红时执行末端与相位4红时执行末端有公交优先请求, 在保障其余相位最小绿时的前提下, 对相位3与相位4采用如图 3所示相继缩短红时的控制策略(RT-RT), 表示为
检测公车交到达时, 分别在相位4红时执行末端和相位1绿时执行中端有公交优先请求, 在保障非优先相位最小绿时的前提下, 对相位4和相位1分别采用如图 4所示缩短红灯时间和插入优先相位的控制策略(RT-PI), 表示为
检测公交车到达时, 分别在相位2绿时执行中端和相位1绿时执行末端有公交优先请求, 在保障其余相位最小绿时的前提下, 对相位2和相位1分别采用如图 5所示插入优先相位和延长绿灯时间的控制策略(PI-GE), 表示为
检测公交到达时, 分别在相位1红时执行中端和相位2红时执行中端有公交优先请求, 在保障各相位最小绿时的前提下, 对相位1和相位2采用如图 6所示相继插入优先相位的控制策略(PI-PI), 表示为
1.2 同一时刻不同相位多路公交优先请求
当交叉口同一时刻收到多路公交优先请求时, 无法通过相位变换同时满足不同相位的优先请求, 但利用优先控制策略可将优先请求公交的等待时间最小化。
检测在相位1绿时执行末端, 同时在相位1和相位3有公交优先请求, 为减少相位3优先公交的等待时间, 在保障其余相位最小绿时的前提下, 如图 7所示, 在对相位1延长绿灯时间结束后同时在相位3插入优先相位(GE-PI), 表示为
检测在相位3绿时执行中后期, 同时在相位2和相位4有公交优先请求, 为减少相位4优先公交的等待时间, 同时考虑相位顺承, 在保障其余相位最小绿时的前提下, 如图 8所示, 对相位3插入优先相位后同时缩短相位4红灯时间(PI-RT), 表示为
检测在相位2绿时执行中期, 同时在相位1和相位4有公交优先请求, 为减少相位4优先公交的等待时间, 同时考虑相位顺承, 在保障其余相位最小绿时的前提下, 如图 9所示, 对相位1插入优先相位后同时在相位4插入优先相位(PI-PI), 表示为
在图 1~9的优先策略中, 各相位分别在绿时左端、绿时右端、红时中端包含了绿时补偿或损失的过程。设相位i左端绿时增加量
优先策略不仅要保障优先公交顺利通过交叉口, 还须满足各相位的最短绿时。设Tpi为相位i的优先策略时长, 在GE策略下, Tpi=gei, 在RT策略下, Tpi=rsi; 在PI策略下, Tpi=gpi。Li为相位i公交通过交叉口轨迹长度, vi为相位i公交通过交叉口平均速度, 存在以下关系
2. 交叉口配时优化的双层规划模型
对多路公交优先交叉口进行配时优化, 旨在减少交叉口和交叉口下游公交站台的乘客延误, 同时尽量减少交叉口内车辆的停驶-加速延误, 提高交叉口运行效率。该问题可以刻画为一个Stackelberg博弈, 在最优方案下, 高层决策者的目标达到最优, 同时可使作为上级决策约束的下层决策者的目标在从属位置上也相应达到最优, 即下层决策以上层决策变量为参数的主从递阶决策问题[15]。对此采用组合优化的方法, 以交叉口与下游站台乘客排队延误最小为上层目标, 以交叉口停驶-加速延误最小为下层目标, 建立多路公交优先配时优化双层规划模型。
2.1 交叉口配时优化的上层模型
2.1.1 交叉口排队延误分析
在非饱和交叉口, 假设车流为线性到达, 在延误计算中不考虑车辆长度与交叉口非优先相位社会车辆排队对公交车的阻挡。图 10为采用优先策略前交叉口进口道车辆在周期内的延误分析。图 10中: Q为累计标准车辆数; qi为相位i车辆到达率(pcu·s-1); si为相位i进口道上车辆离开的饱和流率(pcu·s-1); c为交叉口的信号周期时长; 三角形面积S为优先控制前进口道车辆的延误。
图 11为GE策略下优先相位车辆在周期内的延误分析, 三角形面积S1为采用优先控制策略后进口道车辆的延误, Δd1为GE策略下优先相位进口道车辆的延误减少量。在相位i绿时执行末端延长绿灯时间Δgi2, 三角形面积之差S-S1为周期内相位i延长绿时后减少的延误时间Δd1, 表示为
图 12为RT策略下优先相位车辆在周期内的延误分析, Δd2为RT策略下优先相位进口道车辆的延误减少量。在相位i红时执行末端缩短红灯时间Δgi1, 三角形面积之差S-S1为周期内相位i缩短红时后减少的延误时间Δd2, 表示为
插入相位的延误计算与插入相位的位置有关, 在PI策略中, 插入时刻选择在相位红时执行中端, 以便将其不利影响均衡到2个相邻相位中。图 13为PI策略下优先相位车辆在周期内的延误分析, Δd3为PI策略下优先相位进口道车辆的延误减少量, 四边形面积S11、S12为采用PI策略后进口道的车辆延误。
设相位m与相位m+1为直接承担插入相位绿时损失的2个相邻相位, 则插入时刻t为
面积之差S-S11-S12为周期内相位i插入优先相位后减少的延误时间Δd3, 表示为
由于相邻相位弥补绿时的影响, 当前相位也会受到一部分绿时损失, 两端的绿时损失可理解为前一相位红时延长和当前相位绿时缩短。因而计算相位i延误变化量可以分解为计算
假设qsi为相位i的社会车辆到达率; qbi为相位i的公交车到达率; pb为每辆公交车的平均载客数; ps为每辆社会车辆的平均载客数。周期内交叉口相位i减少的乘客总延误ΔDRi为
2.1.2 下游优先公交站台乘客延误分析
假设站台乘客的到达率为λ, 同一线路相邻公交车准点到达的时间间隔为Tb, 公交车可以一次载完站台上该线路的所有等待乘客。图 14为公交车提前到达时站台乘客的延误分析, t1、t2、t3分别为相邻3辆同线路公交车的到达时刻。公交车提前Δg进站, 对于(t1, t1+Tb-Δg) 时段乘客将减少等待时间, 但同时也增加了(t1+Tb-Δg, t2) 时段乘客的等待时间。由图 14可知公交提前到达时, 站台乘客延误增加量为λΔg2。同理, 公交车延后Δg进站时, (t1, t2) 时段的乘客将增加等待时间, 但同时也减少了(t2, t2+Δg) 时段乘客的等待时间, 站台乘客延误增加量为λΔg2。
由以上分析可知, 公交车早晚点均会增加站台乘客的延误。假设优先公交车辆准点运行通过交叉口时恰好为该相位的绿时执行中端, 公交车早晚点的时间均不超过一个周期。由图 1~9中的优先策略分析可知, GE、PI策略减少了站台乘客的晚点延误, 而RT策略却增加了站台乘客的早点延误。在GE策略下相位i站台乘客延误减少量ΔDpi为
在RT策略下相位i站台乘客延误减少量ΔDpi为
在PI策略下相位i站台乘客延误减少量ΔDpi为
在非饱和交叉口中, 各个相位均有部分绿时富余, 通过相位传递实现非优先相位的绿时损失均衡。但周期内各相位的调节能力是有限的, 过长的优先相位时长会导致非优先相位二次排队, 从而造成拥堵。优先策略在保障公交车顺利通过交叉口的同时, 还需协调周期内各相位的调节能力。δGi、δRi均为0-1变量, 对应相位i有GE、RT策略时取值为1, 否则为0, 则优先策略时长满足下式
由交叉口与下游优先公交站台乘客的排队延误分析, 以交叉口乘客总延误ΔDRi与下游站台乘客延误减少量ΔDpi之和最大为目标函数, 分别以相位i优先策略gei、rsi、gpi的时长为决策变量, 得到如下上层模型为
式中: f(gei, rsi, gpi) 为以gei、rsi、gpi为变量的目标函数。
2.2 交叉口配时优化的下层模型
当车辆到达交叉口时, 当前相位为红灯, 车辆需要减速停车, 而后则需要加速至正常速度。车辆的停驶-加速过程势必造成车辆的延误。为了简化停驶过程, 不考虑交叉口排队队列对车辆停驶的影响, 将之简化为一个加减速的过程。假设车辆在上下游路段正常行驶的速度为v, 社会车辆制动加速度为as1, 起动加速度为as2, 公交车制动加速度为ab1, 起动加速度为ab2, 则每辆社会车辆的停驶-加速延误增加量Δds为
每辆公交车的停驶-加速延误增加量Δds为
由图 10~13, 交叉口相位i停驶-加速延误减少量ΔDsi为
以交叉口内车辆停驶-加速延误减少量最大为目标函数, 以保障各相位最小绿时为约束条件, 得到下层模型为
式中: f(Δgi1, Δgi2) 为以Δgi1、Δgi2为变量的目标函数。
式(21)、(25) 组成了多路公交优先配时优化的双层规划模型, 按以上模型优化后得到各相位的绿时
3. 算法设计
在上述双层模型中, 下层模型在上层模型的决策变量之下根据自身的约束条件和目标函数进行优化, 而后将优化解反馈到上层模型, 以此往复, 在可行域中求得整体最优解。基于上层决策对下层模型进行优化时, 需暂时固定gei、rsi、gpi。由于目标函数为多项式, 故借鉴Gauss-Seidel迭代法[16], 采用对角化方法进行优化, 算法步骤如下。
Step 1:固定上层决策变量gei、rsi、gpi, 视下层目标函数为f(t1, t2, …, t8), t1、t2、…、t8分别表示Δg11、Δg12、…、Δg42。设第k次外循环迭代后的下层变量Tk= (t1k, t2k, …, tjk, …, t7k, t8k), 初始化Tk中的元素序号j为1, 外循环迭代次数k为0, 预设目标函数初值为0。根据下层变量的约束条件, 随机产生初始解T0= (t10, t20, …, t80)。
Step 2:定义
Step 3:如果
Step 4:计算满足
Step 5:如果
Step 6:小循环终止准则为如果j > 8, k=k+1, 执行Step 7, 否则返回Step 3。
Step 7:优化决策变量, 暂时固定Tk中gei、rsi、gpi以外的变量, 对变量gei、rsi、gpi进行对角化迭代, 更新gei、rsi、gpi, 得到Tk。
Step 8:大循环收敛准则为如果|f(Tk+1) - f(Tk)|满足收敛精度, 则停止迭代; 否则j=1, 转至Step 2。
4. 算例分析
以1个4相位的交叉口为例, 相位组成与车辆到达情况见表 1。假设交叉口内每辆公交车平均载客数为35人, 每辆社会车辆平均载客数为3人。分别在相位1红时运行中端以及相位4红时运行末端检测到公交优先请求, 在相位1和相位4分别采用RT与PI策略, 插入相位的时长为gp1, 缩短红灯时长为rs4, 由图 3, 有gp1=Δg22+Δg31, rs4=Δg41。2个优先公交车下游站台乘客到达率分别为0.06、0.07per·s-1。社会车辆平均制动加速度为5.6m·s-2, 平均起动加速度为2.7m·s-2, 公交车平均制动加速度为5.3m·s-2, 平均起动加速度为2.2 m·s-2, 交叉口上下游路段平均车速为30m·s-1。将以上数据带入式(21)、(25) 中的模型求解, 计算结果见表 2。
表 1 交叉口交通流数据与控制参数Table 1. Traffic flow data and control parameters of intersection
表 2 优化模型计算结果Table 2. Calculation result of optimal model
由表 2可知, 配时优化后, 交叉口在周期内乘客的总延误减少了22 806.16s, 下游公交站乘客延误减少了769.96s, 交叉口车辆停驶-加速延误减少了62.87s, 双层规划模型在降低交叉口与站台乘客延误的同时也降低了交叉口的车辆停驶-加速延误。优化后各相位绿时均大于各相位的最小绿时, 保障了各相位车辆的正常通行。公交信号优先将交叉口各相位富余绿时资源通过相位承接转移到优先相位。公交车载客量明显大于社会车辆, 因而对绿时利用率较高, 绿时资源向优先相位转移有利于交叉口效率的提高。
图 15~16为双层规划模型的上层与下层目标函数值随迭代次数的变化情况, 图 17为上层单目标函数的优化迭代结果。由图 14~16可知, 与上层单目标优化相比, 双层规划模型以上层目标值1 350s的损失考虑了交叉口内车辆的停驶-加速延误。在实现公交优先的前提下, 最大限度减小公交优先对交叉口其余相位车辆的不利影响, 尤其是在绿时损失较大的多路公交优先中, 对下层目标的考虑是非常必要的。双层规划模型同时对交叉口和下游优先公交站台的乘客总延误以及交叉口的停驶-加速延误进行了优化, 提高了交叉口的整体效益。
5. 结语
本文分析了基于延长绿灯时间、缩短红灯时间、插入优先相位3种公交优先控制策略下的多路公交优先控制策略, 在交叉口延误计算的基础上建立了配时优化双层规划模型。实例分析结果表明, 模型优化了交叉口与下游优先公交站台乘客的排队延误, 同时也保障了交叉口内车辆通行的效率。相较单一上层目标优化结果, 双层规划模型以较小的上层目标损失, 保障了交叉口的整体效益, 在尽量减少非优先相位车辆通行效益损失的前提下, 实现了公交优先和损失均衡。模型在多路公交优先前提下从延误的角度考虑了交叉口优先相位与非优先相位间绿时资源的协调, 以交叉口整体效益最优进行配时优化, 在保持周期稳定的情况下进行绿时协调, 避免了相位紊乱, 便于实施对交叉口的协调控制。双层规划模型还可以从资源、环境等多角度对交叉口进行优化, 具有很好的拓展和利用空间, 在多路优先请求的群交叉口协调控制中也具有较大的应用和发展空间。
-
图 7 同一时刻GE-PI策略的绿时损失均衡
Figure 7. Green loss equilibrium of GE-PI strategy at same time
图 8 同一时刻PI-RT策略的绿时损失均衡
Figure 8. Green loss equilibrium of PI-RT strategy at same time
图 9 同一时刻PI-PI策略的绿时损失均衡
Figure 9. Green loss equilibrium of PI-PI stratgey at same time
-
[1] WU Jian-ping, HOUNSELL N. Bus priority using presignals[J]. Transportation Research Part A: Policy and Practice, 1998, 32 (8): 563-583. doi: 10.1016/S0965-8564(98)00008-1 [2] 马万经, 杨晓光. 单点公交优先感应控制策略效益分析与仿真验证[J]. 系统仿真学报, 2008, 20 (12): 3309-3313. https://www.cnki.com.cn/Article/CJFDTOTAL-XTFZ200812062.htmMA Wan-jing, YANG Xiao-guang. Efficiency analysis of transit signal priority strategies on isolated intersection[J]. Journal of System Simulation, 2008, 20 (12): 3309-3313. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-XTFZ200812062.htm [3] WAHLSTEDT J. Impacts of bus priority in coordinated traffic signals[J]. Procedia-Social and Behavioral Sciences, 2011, 16: 578-587. doi: 10.1016/j.sbspro.2011.04.478 [4] LIU Hong-chao, ZHANG Jie, CHENG Ding-xin. Analytical approach to evaluating transit signal priority[J]. Journal of Transportation Systems Engineering and Information Technology, 2008, 8 (2): 48-57. doi: 10.1016/S1570-6672(08)60017-3 [5] ZHOU Guang-wei, GAN A, SHEN L D. Optimization of adaptive transit signal priority using parallel genetic algorithm[J]. Tsinghua Science and Technology, 2007, 12 (2): 131-140. doi: 10.1016/S1007-0214(07)70020-2 [6] 李劲夫. 公交优先交叉口信号控制参数的多目标优化方法[J]. 长沙大学学报, 2012, 26 (2): 64-68. https://www.cnki.com.cn/Article/CJFDTOTAL-CSDX201202025.htmLI Jin-fu. Multi-objective optimization method of intersection signal control parameter based on bus priority[J]. Journal of Changsha University, 2012, 26 (2): 64-68. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-CSDX201202025.htm [7] 李淑庆, 李哲, 朱文英. 一体化公交网络均衡配流模型[J]. 交通运输工程学报, 2013, 13 (1): 62-69. doi: 10.3969/j.issn.1671-1637.2013.01.010LI Shu-qing, LI Zhe, ZHU Wen-ying. Equilibrium assignment model of integrated transit network[J]. Journal of Traffic and Transportation Engineering, 2013, 13 (1): 62-69. (in Chinese). doi: 10.3969/j.issn.1671-1637.2013.01.010 [8] KIM S, PARK M, CHON K S. Bus signal priority strategies for multi-directional bus routes[J]. KSCE Journal of Civil Engineering, 2012, 16 (5): 855-861. doi: 10.1007/s12205-012-1507-7 [9] 柏海舰, 董瑞娟, 张敏, 等. 基于同步多样性的公交时刻优化方法[J]. 交通运输工程学报, 2013, 13 (3): 79-85. http://transport.chd.edu.cn/article/id/201303011BAI Hai-jian, DONG Rui-juan, ZHANG Min, et al. Optimization method of bus time based on synchronization diversity[J]. Journal of Traffic and Transportation Engineering, 2013, 13 (3): 79-85. (in Chinese). http://transport.chd.edu.cn/article/id/201303011 [10] MA Wan-jing, LIU Yue, YANG Xiao-guang. A dynamic programming approach for optimal signal priority control upon multiple high-frequency bus requests[J]. Journal of Intelligent Transportation Systems: Technology, Planning, and Operations, 2013, 17 (4): 282-293. doi: 10.1080/15472450.2012.729380 [11] MA Wan-jing, LIU Yue, YANG Xiao-guang. A dynamic programming model for bus signal priority with multiple requests[C]∥TRB. Transportation Research Board 90th Annual Meeting. Washington DC: TRB, 2011: 2851-2866. [12] 孙煦, 陆化普. 公交优先下交叉口配时优化的双层模型与遗传算法[J]. 北京工业大学学报, 2012, 38 (6): 859-864. https://www.cnki.com.cn/Article/CJFDTOTAL-BJGD201206011.htmSUN Xu, LU Hua-pu. Bi-level optimization model of intersection timing about bus priority condition based on genetic algorithm[J]. Journal of Beijing University of Technology, 2012, 38 (6): 859-864. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-BJGD201206011.htm [13] HE Qing, HEAD K L, DING Jun. Heuristic algorithm for priority traffic signal control[J]. Transportation Research Record, 2011 (2259): 1-7. [14] 张卫华, 石琴, 刘强. 公交优先信号交叉口延误计算与配时优化方法[J]. 华中科技大学学报: 城市科学版, 2004, 21 (4): 30-33. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCJ200404009.htmZHANG Wei-hua, SHI Qin, LIU Qiang. Study of vehicle delay calculation and optimal signal-planning method for intersections with induced signal based on bus priority[J]. Journal of Huazhong University of Science and Technology: Urban Science Edition, 2004, 21 (4): 30-33. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-WHCJ200404009.htm [15] YANG Hai, ZHANG Xiao-ning, MENG Qiang. Stackelberg games and multiple equilibrium behaviors on networks[J]. Transportation Research Part B: Methodological, 2007, 41 (8): 841-861. [16] ALLEVI E, GNUDI A, KONNOV I V, et al. Gauss-Seidel method for multi-valued inclusions with Z mappings[J]. Journal of Global Optimization, 2012, 53 (1): 97-105. -
下载:
下载:
点击查看大图
计量
- 文章访问数: 734
- HTML全文浏览量: 159
- PDF下载量: 751
- 被引次数: 0
