采用刚度矩阵法的弹性层状体系数值解法

阳恩慧; 艾长发; 邱延峻

采用刚度矩阵法的弹性层状体系数值解法

阳恩慧^{1, 2,},
艾长发^{1, 2},
邱延峻^{1, 2}

1.
西南交通大学土木工程学院, 四川成都 610031
2.
西南交通大学道路工程四川省重点实验室, 四川成都 610031

基金项目:

国家自然科学基金项目 51308477

中央高校基本科研业务费专项资金项目 2682013BR030

详细信息

作者简介:
阳恩慧(1982-), 男, 湖南洞口人, 西南交通大学讲师, 工学博士, 从事道路工程计算力学及新材料研究

中图分类号: U213.1
计量
- 文章访问数: 570
- HTML全文浏览量: 100
- PDF下载量: 941
- 被引次数: 0
出版历程
- 收稿日期: 2014-03-16
- 刊出日期: 2014-08-25

Numerical method of multi-layer elastic system by using stiffness matrix method

YANG En-hui^{1, 2
,},
AI Zhang-fa^{1, 2},
QIU Yan-jun^{1, 2}

1.
School of Civil Engineering, Southwest Jiaotong University, Chengdu 610031, Sichuan, China
2.
Highway Engineering Key Laboratory of Sichuan Province, Southwest Jiaotong University, Chengdu 610031, Sichuan, China

More Information

Author Bio:
YANG En-hui(1982-), male, lecturer, PhD, +86-28-87634347, yeh1982@163.com

摘要

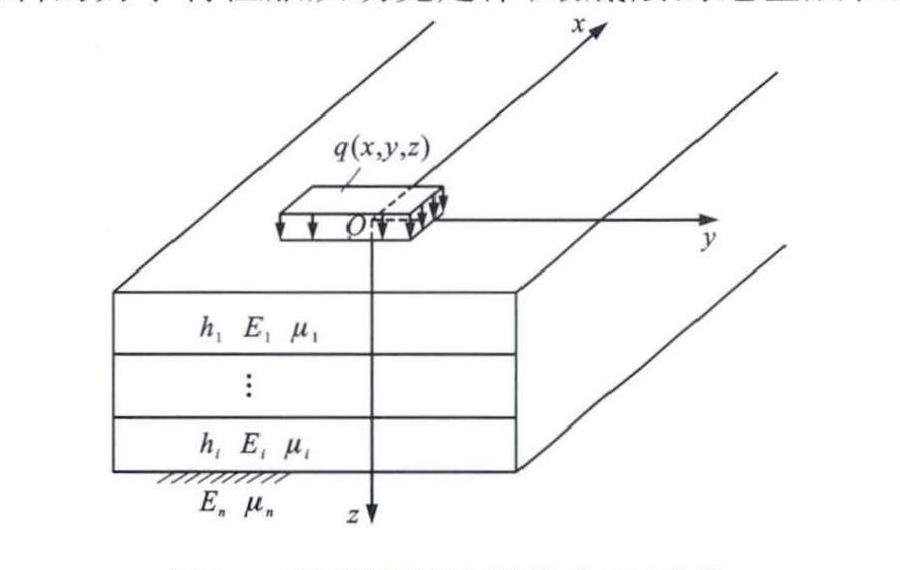
摘要: 从弹性力学基础理论出发, 采用刚度矩阵法, 推导了应用于直角坐标系下的三维多层弹性层状体系静力学数值解法。引入二维傅里叶变换及高斯积分求解法, 基于MATLAB数学软件平台编制计算程序, 实现三维多层弹性层状体系理论计算方法的数值求解。针对典型有砟轨道轨下基础结构, 采用提出的计算方法和编制的相应计算程序对其进行静力学分析, 并将所获得的计算结果与采用通用有限元程序ABAQUS的计算结果进行对比。分析结果表明: 采用提出的计算方法和通用有限元计算方法获得有砟轨道轨下基础最大竖向位移分别为1.50、1.95 mm, 最大竖向应力分别为0.34、0.21 MPa, 计算结果较为接近, 计算反映出来的各状态分量变换规律基本一致, 提出的计算方法及其相应计算程序可应用于多层弹性层状体系的静力学计算。
- 路基工程 /
- 弹性层状体系 /
- 刚度矩阵法 /
- 数值解法
Abstract: By using the theory of elastic mechanics and stiffness matrix method, a numerical method of static mechanics for calculating 3D multi-layer elastic system under rectangular coordinate system was developed.2D Fourier transform and Gauss integral method were used, a calculation program was given based on MATLAB mathematical software platform, and the numerical solution of 3D multi-layer elastic system was got.The static mechanics analysis of ballast track foundation structure was carried out by using the numerical method and program, and the calculation results were compared with the results obtained by using general finite element program ABAQUS.Analysis result shows that the maximum vertical displacements of ballast track foundation are 1.50, 1.95 mm respectively by using the numerical method and general finite element calculation method, and the maximum vertical stresses of the foundation are 0.34, 0.21 MPa respectively by using the two methods.The calculation results are close to each other, and the conversion rules of state components are basically the same according to the calculation, so the proposed numerical method and program can be applied to the static mechanics calculation of multi-layer elastic system.
- subgrade engineering /
- multi-layer elastic system /
- stiffness matrix method /
- numerical method

HTML全文

图 1 三维多层弹性层状体系理论计算

Figure 1. Theoretical calculation of 3D multi-layer elastic system

下载: 全尺寸图片幻灯片

图 2 单一层应力及位移

Figure 2. Stress and displacement of each layer

下载: 全尺寸图片幻灯片

图 3 有砟轨道系统整体结构计算模型

Figure 3. Calculation model of ballast track system overall structure

下载: 全尺寸图片幻灯片

图 4 轨枕节点力链杆等效转换

Figure 4. Equivalent conversion of sleeper nodal force

下载: 全尺寸图片幻灯片

图 5 有砟轨道结构单元划分及节点编号

Figure 5. Structure units and node numbers of ballast track

下载: 全尺寸图片幻灯片

图 6 有砟轨道轨下基础顶面竖向位移

Figure 6. Vertical displacement above ballast track foundation

下载: 全尺寸图片幻灯片

图 7 有限元计算模型

Figure 7. Finite element calculating model

下载: 全尺寸图片幻灯片

图 8 竖向位移计算结果对比

Figure 8. Comparison of vertical displacement calculating results

下载: 全尺寸图片幻灯片

图 9 竖向应力计算结果对比

Figure 9. Comparison of vertical stress calculating results

下载: 全尺寸图片幻灯片

表 1 有砟轨道计算参数

Table 1. Calculating parameters of ballast track

下载: 导出CSV

表 2 最大竖向位移对比

Table 2. Comparison of maximum vertical displacements

下载: 导出CSV

参考文献(24)

[1]	BURMISTER D M. The general theory of stresses and displacements in layered systems. Ⅰ[J]. Journal of Applied Physics, 1945, 16(2): 89-94. doi: 10.1063/1.1707558
[2]	BURMISTER D M. The general theory of stresses and displacements in layered soil system. Ⅱ[J]. Journal of Applied Physics, 1945, 16(3): 126-127. doi: 10.1063/1.1707562
[3]	BURMISTER D M. The general theory of stresses and displacements in layered soil system. Ⅲ[J]. Journal of Applied Physics, 1945, 16(5): 296-302. doi: 10.1063/1.1707590
[4]	王凯. N层弹性连续体系在圆形均布荷载作用下的应力与位移[J]. 土木工程学报, 1982, 15(2): 65-76. WANG Kai. Stress and displacements analysis of an N-layered elastic-continuous system under vertical load uniformly distributed on a circular area[J]. China Civil Engineering Journal, 1982, 15(2): 65-76. (in Chinese).
[5]	王凯. N层弹性连续体系在双圆均布复合荷载作用下的力学计算[J]. 固体力学学报, 1983(1): 136-153. https://www.cnki.com.cn/Article/CJFDTOTAL-GTLX198301017.htm WANG Kai. Calculation of stress, strains and displacements in an N-layered elastic system under the multiple inward horizontal loads on circular areas[J]. Acta Mechanica Solida Sinica, 1983(1): 136-153. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GTLX198301017.htm
[6]	王凯. N层弹性体系在多圆向心水平荷载作用下的力学计算[J]. 重庆交通学院学报, 1984(2): 50-64. https://www.cnki.com.cn/Article/CJFDTOTAL-CQJT198402005.htm WANG Kai. Calculation of stress, strains and displacements in an N-layered elastic system under the multiple inward horizontal loads on circular areas[J]. Journal of Chongqing Jiaotong University, 1984(2): 50-64. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-CQJT198402005.htm
[7]	王凯, 姚炳卿. N层弹性体系在多圆旋转水平荷载作用下的力学计算[J]. 西安公路学院学报, 1986, 4(3): 15-30. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL198603001.htm WANG Kai, YAO Bing-qing. Calculation of an N-layered elastic system under the action of multiple rotating-horizontal loads distributed on the circular areas[J]. Journal of Xi'an Institute of Highway, 1986, 4(3): 15-30. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL198603001.htm
[8]	张起森. 弹性层状体系理论的实验验证及应用[J]. 土木工程学报, 1985, 18(4);63-76. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC198504007.htm ZHANG Qi-sen. Experimental verification of the elastic layer system theory and its application[J]. China Civil Engineering Journal, 1985, 18(4): 63-76. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC198504007.htm
[9]	张起森, 郑健龙. 弹性层状体系考虑层间接触非线性的有限单元分析法[J]. 土木工程学报, 1989, 22(3): 63-75. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC198903009.htm ZHANG Qi-sen, ZHENG Jian-long. Finite element analysis of elastic layer system considering nonlinear contact condition between layers[J]. China Civil Engineering Journal, 1989, 22(3): 63-75. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC198903009.htm
[10]	黄卫. 弹性层状体系弯拉应力近似计算[J]. 岩土工程学报, 1995, 17(6): 52-54. https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC506.005.htm HUANG Wei. Regression formulas of tensile stress in the elastic multilayer[J]. Chinese Journal of Geotechnical Engineering, 1995, 17(6): 52-54. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC506.005.htm
[11]	任瑞波. 沥青路面结构计算方法与FWD应用技术的研究[D]. 哈尔滨: 啥尔滨建筑大学, 2000. REN Rui-bo. The study of asphalt pavement structure calculation and FWD application technique[D]. Harbin: Harbin University of Civil Engineering and Architecture, 2000. (in Chinese).
[12]	任瑞渡, 钟岱辉, 孔军, 等. 沥青路面层状粘弹性半空间轴对称问题的求解[J]. 山东建筑工程学院学报, 2002, 17(4): 1-7. doi: 10.3969/j.issn.1673-7644.2002.04.001 REN Rui-bo, ZHONG Dai-hui, KONG Jun, et al. The theoretical method for solving axisymmetrical problems in multilayered viscoelastic asphalt pavement half space[J]. Journal of Shandong Institute of Architecture and Engineering, 2002, 17(4): 1-7. (in Chinese). doi: 10.3969/j.issn.1673-7644.2002.04.001
[13]	钟阳, 王哲人, 郭大智. 求解多层弹性半空间轴对称问题的传递矩阵法[J]. 土木工程学报, 1992, 25(6): 37-43. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC199206004.htm ZHONG Yang, WANG Zhe-ren, GUO Da-zhi. The transfer matrix method for solving axisymmetrical problems in multilayered elastic half space[J]. China Civil Engineering Journal, 1992, 25(6): 37-43. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC199206004.htm
[14]	钟阳, 王哲人, 郭大智, 等. 求解多层弹性半空间非轴对称问题的传递矩阵法[J]. 土木工程学报, 1995, 28(1): 66-72. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC199501007.htm ZHONG Yang, WANG Zhe-ren, GUO Da-zhi, et al. Transfer matrix method for solving non-axisymmetrical problems in multilayered elastic half space[J]. China Civil Engineering Journal, 1995, 28(1): 66-72. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC199501007.htm
[15]	钟阳, 张永山. 求解多层弹性半空间轴对称动态问题的精确刚度矩阵法[J]. 力学季刊, 2003, 24(3): 395-400. doi: 10.3969/j.issn.0254-0053.2003.03.018 ZHONG Yang, ZHANG Yong-shan. Explicit solution for axisymmetrical multilayered elastic half space problems by exact stiffness matrix method[J]. Chinese Quarterly of Mechanics, 2003, 24(3): 395-400. (in Chinese). doi: 10.3969/j.issn.0254-0053.2003.03.018
[16]	钟阳, 郭大智, 张肖宁. 轴对称弹性半空间问题一般解的新方法[J]. 哈尔滨建筑大学学报, 1995, 28(2): 23-27. https://www.cnki.com.cn/Article/CJFDTOTAL-HEBJ502.003.htm ZHONG Yang, GUO Da-zhi, ZHANG Xiao-ning. New way of the general solution of axial symmetry elastic half space problem[J]. Journal of Harbin University of Architecture and Engineering, 1995, 28(2): 23-27. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-HEBJ502.003.htm
[17]	钟阳, 陈静云, 王龙, 等. 求解动荷载作用下多层粘弹性半空间轴对称问题的精确刚度矩阵法[J]. 计算力学学报, 2003, 20(6): 749-755. https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG200306017.htm ZHONG Yang, CHEN Jing-yun, WANG Long, et al. Explicit solution for dynamic response of axisymmetrical problems in multilayered viscoelastic half space by exact stiffness matrix method[J]. Chinese Journal of Computational Mechanics, 2003, 20(6): 749-755. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG200306017.htm
[18]	HUANG Y H, LIN C, DENG X J, et al. Kentrack: a Computer Program for Hot-mix Asphalt and Conventional Ballast Railway Trackbeds[M]. Lexington and Lanham: Asphalt Institute and NAPA Publication, 1984.
[19]	ROSE J G, SU B, LONG W B. Kentrack: a railway trackbed structural design and analysis program[C]//AREMA. Proceedings of the AREMA 2003 Annual Conference and Exposition. Chicago: AREMA, 2003: 1-25.
[20]	ROSE J G, KONDURI K C. Kentrack—a railway trackbed structural design program[C]/7 AREMA. Proceedings of the AREMA 2006 Annual Conference and Exposition. Lanham: AREMA, 2006: 1-31.
[21]	ABRAMOWITZ M, STEGUN I A. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables[M]. New Youk: Dover Publications, 1965.
[22]	刘尧军, 郭增强, 赵玉成. 秦沈客运专线不同填料路基动力特性的试验研究[J]. 石家庄铁道学院学报, 2004. https://www.cnki.com.cn/Article/CJFDTOTAL-SJZT200402005.htm 17(2): 19-22. LIU Yao-jun, GUO Zeng-qiang, ZHAO Yu-cheng. Research on dynamic characteristics of Qinshen high-speed railway roadbed with different filling materials[J]. Journal of Shijiazhuang Railway Institute, 2004, 17(2): 19-22. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-SJZT200402005.htm
[23]	WANG Kai-yun, HUANG Chao, ZHAI Wan-ming, et al. Progress on wheel-rail dynamic performance of railway curve negotiation[J]. Journal of Traffic and Transportation Engineering; English Edition, 2014, 1(3): 209-220.
[24]	杨尧. 客运专线铁路基床填料动静三轴试验研究[D]. 成都: 西南交通大学, 2009. YANG Yao. Experimental study on typical filler for passenger dedicated railway subgrade roadbed by static and dynamic triaxial test[D]. Chengdu: Southwest Jiaotong University, 2009. (in Chinese).