Probabilistic mechanical properties of LZ50 axle steel for railway vehicles
Article Text (Baidu Translation)
-
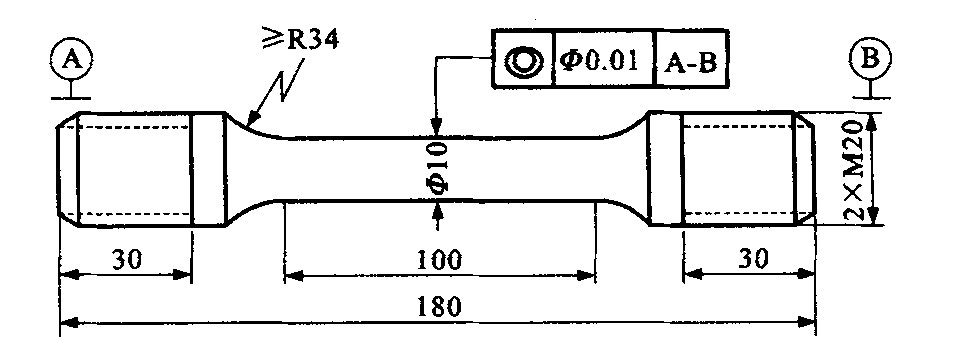
摘要: 完成了铁道车辆LZ5 0车轴钢的概率机械性能试验研究, 拓宽了确定有限疲劳可靠性数据良好假设分布的统一方法, 比较了6种常用分布(即三参数Weibull、两参数Weibull、正态、对数正态、极大值和极小值分布)对试验数据的描述效果, 综合分析了他们的拟合优度、失效机理的一致性和尾部预测的安全性。从预测的安全性角度, 选择了极小值分布为最佳统计模型, 提出了给定可靠度和置信度下基于极小值分布的机械性能参数估计方法, 并有效地估计了材料的概率机械性能参数。Abstract: Experimental study on the probabilistic mechanical properties was performed on the LZ50 axle steel for railway vehicles.The unified approach for determining appropriate statistical models of the limited fatigue reliability data was developed to analyze the present test data.The six commonly used distributions, namely three parameter Weibull, two parameter Weibull, normal, lognormal, extreme maximum value, and extreme minimum value, were compared in fits of the data from the three considerations of goodness-of-fit, consistency of failure mechanism and safety in tail region predictions.From the viewpoint of safety, the extreme minimum value distribution was selected as an optimal model of the data.A method based on this distribution was proposed for the estimation of mechanical properties under given reliability and confidence levels.The method has been well used for the estimation of probabilistic parameters of the mechanical properties of the present material.
-
Key words:
- railway vehicle /
- axle /
- LZ50 steel /
- mechanical properties /
- reliability /
- lognormal distribution
-
图 2 6种分布对名义屈服强度的描述效果
Figure 2. Effects of the six distributions fitting into the nominal yielding strength data
表 1 LZ50车轴钢的化学成分
Table 1. Chemical composition of the LZ50 axle steel
/ %C Si Mn Cr Ni Cu Al P S 0.470 0.260 0.780 0.020 0.028 0.150 0.021 < 0.014 < 0.007 
下载: 导出CSV
表 2 LZ50车轴钢的机械性能试验结果
Table 2. Test results of the mechanical properties of LZ50 axle steel
试样序号 弹性模量E/GPa 名义屈服强度Ss/MPa 名义屈服应变es/% 名义强度极限Sb/MPa 名义应变极限eb/% 真屈服强度σs/MPa 真屈服应变εs/% 真强度极限σb/MPa 真应变极限εb/MPa 延伸率δ/% 断面收缩率ψ/% 1 209.82 319.58 0.364 627.71 54.688 320.74 0.3633 970.99 43.624 23.80 42.84 2 195.88 328.49 0.376 635.35 50.778 329.73 0.3753 957.97 41.064 23.60 41.81 3 216.65 332.37 0.368 632.79 52.714 333.59 0.3673 966.36 42.340 23.90 42.16 4 191.85 336.21 0.376 636.62 53.288 337.47 0.3753 975.86 42.715 23.62 40.78 5 221.16 331.09 0.352 625.12 54.204 332.26 0.3514 963.96 43.311 25.68 41.13 6 224.11 333.65 0.360 628.95 53.598 334.85 0.3594 966.05 42.917 24.54 43.19 7 222.16 325.98 0.352 623.84 54.186 327.13 0.3514 961.87 43.299 25.38 40.78 8 198.92 333.65 0.384 634.06 53.960 334.93 0.3833 976.20 43.152 24.62 42.50 9 194.75 329.81 0.392 621.28 53.772 331.10 0.3912 955.35 43.030 24.04 40.81 10 222.16 328.54 0.360 623.84 52.912 329.72 0.3594 953.93 42.469 24.92 43.19
下载: 导出CSV
表 3 LZ50车轴钢的机械性能的均值Xm、均方差Sx和变异系数Vx
Table 3. Average values Xm, standard deviations Sxand variations coefficient Vxof the mechanical properties of LZ50 axle steel
参数 弹性模量E/GPa 名义屈服强度Ss/MPa 名义屈服应变es/% 名义强度极限Sb/MPa 名义应变极限eb/% 真屈服强度σs/MPa 真屈服应变εs/% 真强度极限σb/MPa 真应变极限εb/MPa 延伸率δ/% 断面收缩率ψ/% Xm 209.75 329.94 0.3684 628.96 53.41 331.15 0.3677 964.85 42.79 24.41 41.92 Sx 13.1111 4.7277 0.0134 5.4559 1.1060 4.7574 0.0133 7.8738 0.7243 0.7397 0.9963 Vx 0.0625 0.0143 0.0364 0.0087 0.0207 0.0144 0.0363 0.0082 0.0169 0.0303 0.0238
下载: 导出CSV
表 4 6种统计分布拟合试验数据的参量点估计值
Table 4. Point estimations for the statistical parameters of the six commonly used distributions fitting into the test data
机械性能 三参数Weibull 两参数Weibull 正态 对数正态 极大值 极小值 PL PS m PS m PL PS PL PS PL PS PL PS 弹性模量E/GPa 190.15 22.7898 1.0174 216.08 16.5808 209.75 15.2991 2.3209 0.0320 216.32 12.5608 202.93 13.0381 名义屈服强度Ss/MPa 0 332.18 76.0653 332.18 76.0654 329.94 5.3935 2.5184 0.0072 332.19 4.3059 327.50 4.6523 名义屈服应变es/% 0.3450 0.0270 1.6395 0.3749 29.1408 0.3684 1.4958 -0.4339 0.0175 0.3751 0.0128 0.3620 0.0122 名义强度极限Sb/MPa 618.94 11.5880 1.6592 631.62 122.80 628.96 6.1155 2.7986 0.0042 631.64 5.1265 626.30 5.0793 名义应变极限eb/% 0 53.9484 51.1501 53.9484 51.1501 53.4100 1.3105 1.7275 0.0108 53.9472 1.0269 52.8078 1.1512 真屈服强度σs/MPa 0 333.41 75.8973 333.41 75.8973 331.15 5.4246 2.5200 0.0072 333.42 4.3313 328.71 4.6784 真屈服应变εs/% 0.3450 0.0262 1.5771 0.3742 29.2170 0.3677 1.4892 -0.4347 0.0175 0.3744 0.0127 0.3614 0.0121 真强度极限σb/MPa 949.51 17.7105 1.7932 968.67 131.69 964.85 8.7551 2.9845 0.0039 968.69 7.3350 961.06 7.2603 真应变极限εb/MPa 0 43.1449 62.7053 43.1449 62.7053 42.7921 0.8596 1.6313 0.0089 43.1442 0.6732 42.3968 0.7556 延伸率δ/% 23.5450 0.9491 0.8855 24.7765 34.1600 24.4100 0.8366 1.3874 0.0148 24.7874 0.7214 24.0568 0.6751 断面收缩率ψ/% 40.2600 1.9369 1.4248 42.4140 43.6200 41.9190 1.1416 1.6223 0.0118 42.4221 0.9613 41.4230 0.9481
下载: 导出CSV
表 5 6种统计分布拟合试验数据相关系数r与线性相关系数rXY
Table 5. Relationship coefficient r and linear relationship coefficient rXYof the six distributions fitting into the test data
机械性能 三参数Weibull 两参数Weibull 正态 对数正态 极大值 极小值 r rXY r rXY r rXY r rXY r rXY r rXY 弹性模量E/GPa 0.9660 0.9619 0.9550 0.9374 0.9509 0.9368 0.9498 0.9360 0.9403 0.9019 0.9556 0.9361 名义屈服强度Ss/MPa 0.9881 0.9837 0.9881 0.9837 0.9735 0.9581 0.9721 0.9560 0.9429 0.9114 0.9887 0.9847 名义屈服应变es/% 0.9893 0.9739 0.9692 0.9448 0.9842 0.9787 0.9852 0.9802 0.9883 0.9875 0.9670 0.9411 名义强度极限Sb/MPa 0.9841 0.9836 0.9690 0.9550 0.9795 0.9752 0.9796 0.9753 0.9797 0.9633 0.9687 0.9545 名义应变极限eb/% 0.9739 0.9630 0.9739 0.9630 0.9484 0.9226 0.9447 0.9180 0.9055 0.8617 0.9763 0.9659 真屈服强度σs/MPa 0.9885 0.9841 0.9885 0.9841 0.9738 0.9586 0.9723 0.9564 0.9429 0.9120 0.9890 0.9851 真屈服应变εs/% 0.9891 0.9736 0.9690 0.9447 0.9840 0.9786 0.9851 0.9801 0.9882 0.9874 0.9668 0.9411 真强度极限σb/MPa 0.9899 0.9875 0.9765 0.9634 0.9903 0.9830 0.9904 0.9832 0.9866 0.9726 0.9760 0.9627 真应变极限εb/MPa 0.9736 0.9625 0.9736 0.9625 0.9471 0.9210 0.9441 0.9173 0.9037 0.8597 0.9755 0.9649 延伸率δ/% 0.9874 0.9809 0.9586 0.9224 0.9755 0.9664 0.9765 0.9678 0.9827 0.9826 0.9567 0.9196 断面收缩率ψ/% 0.9675 0.9363 0.9720 0.9297 0.9726 0.9540 0.9723 0.9537 0.9631 0.9424 0.9717 0.9291
下载: 导出CSV
表 6 6种统计分布拟合两个尾部数据时的概率预测值与试验值误差参量dF1和dF2
Table 6. Errors dF1and dF2between the experimental values and the predictions of six commonly used distributions to the minimum and sub-minimum data
机械性能 三参数Weibull 两参数Weibull 正态 对数正态 极大值 极小值 dF1 dF2 dF1 dF2 dF1 dF2 dF1 dF2 dF1 dF2 dF1 dF2 弹性模量E/GPa -0.00150 -0.01477 -0.06258 0.00005 -0.0537 -0.00004 -0.05027 0.00076 -0.0292 0.06698 -0.06557 0.03058 名义屈服强度Ss/MPa 0.01592 -0.04865 0.01592 -0.04865 0.03990 -0.06812 0.04041 -0.07044 0.06318 -0.15934 0.01523 0.11139 名义屈服应变es/% -0.03624 0.05991 -0.08050 0.01565 -0.06914 0.02701 -0.06515 0.03100 -0.03475 0.06141 -0.08392 0.01223 名义强度极限Sb/MPa -0.00085 -0.05004 -0.05613 -0.03265 -0.03740 -0.03796 -0.03653 -0.03787 -0.00084 0.09532 -0.05688 0.03928 名义应变极限eb/% 0.02317 -0.10019 0.02317 -0.10019 0.04500 -0.13421 0.04529 -0.13916 0.06427 0.16053 0.02266 0.11881 真屈服强度σs/MPa -0.03505 0.06111 0.01585 -0.04685 0.03984 -0.06575 -0.06512 0.03104 0.06317 0.15932 0.01516 0.11131 真屈服应变εs/% 0.01585 -0.04685 -0.08048 0.01568 -0.06910 0.02705 0.04036 -0.06806 -0.03470 0.06146 0.08389 0.01227 真强度极限σb/MPa -0.01233 0.03563 -0.05708 0.01426 -0.03876 0.02462 -0.03792 0.02523 -0.00205 0.09410 -0.05781 0.03834 真应变极限εb/MPa 0.02325 -0.10089 0.02325 -0.10089 0.04511 -0.13560 0.04533 -0.14005 0.06439 0.16054 0.02284 0.11899 延伸率δ/% -0.02159 0.05211 -0.10555 -0.01399 -0.09917 -0.00910 -0.09617 -0.00623 -0.07250 0.02360 -0.10807 -0.01192 断面收缩率ψ/% -0.07505 0.02110 -0.09760 -0.00140 -0.09190 0.00426 -0.09090 0.00529 -0.07210 0.02406 -0.09853 -0.00238
下载: 导出CSV
表 7 LZ50钢机械性能试验数据的t1-C(n-2)函数值
Table 7. t1-C(n-2) function values for the test data of LZ50 axle steel at given confidence level C
n-2 C/% 90.0 95.0 97.5 99.0 99.5 8 1.3968 1.8595 2.3060 2.8965 3.3554
下载: 导出CSV
表 8 极小值分布拟合LZ50钢机械性能数据的位置参量PL、尺度参量Ps和线性拟合残差s
Table 8. Location parameter PL, scale parameter Psand residual standard deviation s of the extreme minimum value distribution fitting into the test data of mechanical properties of LZ50 axle steel
参数 弹性模量E/GPa 名义屈服强度Ss/MPa 名度屈服应变es/% 名义强度极限Sb/MPa 名度应变极限eb/% 真屈服强度σs/MPa 真屈服应变εs/% 真强度极限σb/MPa 真应变极限εb/MPa 延伸率δ/% 断面收缩率ψ/% PL 202.93 327.50 0.3620 626.30 52.8078 328.71 0.3614 961.06 42.3968 24.0568 41.4230 Ps 13.0381 4.6523 0.0122 5.0793 1.1512 4.6784 0.0121 7.2603 0.7556 0.6751 0.9481 s 0.4159 0.2061 0.3998 0.3528 0.3062 0.2036 0.4000 0.3200 0.3105 0.4647 0.4374
下载: 导出CSV
表 9 可靠度R和置信度C水平下铁道车辆LZ50车轴钢的概率机械性能参量
Table 9. Probabilistic mechanical properties of the LZ50 axle steel for railway vehicles at given reliabilites and confidences
R 0.500 0.900 0.990 0.999 C/% 90 95 99 90 95 99 90 95 99 90 95 99 弹性模量E/GPa 190.207 187.576 181.678 165.646 163.014 157.116 135.009 132.377 126.480 104.928 102.297 96.3993 名义屈服强度Ss/MPa 324.390 323.925 322.882 315.626 315.161 314.1128 304.694 304.229 303.186 293.961 293.495 292.452 名义屈服应变es/% 0.35038 0.34802 0.34271 0.32740 0.32503 0.31973 0.29873 0.29637 0.29106 0.27059 0.26822 0.26291 名义强度极限Sb/MPa 621.813 620.944 618.995 612.244 611.375 609.426 600.309 599.440 597.491 588.591 587.721 585.772 名义应变极限eb/% 51.8695 51.6984 51.3150 49.7008 49.5297 49.1463 46.9957 46.8246 46.4413 44.3398 44.1687 43.7853 真屈服强度σs/MPa 325.600 325.138 324.102 316.786 316.324 315.288 305.793 305.331 304.295 295.000 294.537 293.501 真屈服应变εs/% 0.34988 0.34753 0.34226 0.32708 0.32473 0.31947 0.29865 0.29630 0.29104 0.27073 0.26838 0.26312 真强度极限σb/MPa 954.995 953.868 951.341 941.318 940.191 937.664 924.258 923.130 920.604 907.508 906.380 903.853 真应变极限εb/MPa 41.7762 41.6623 41.4071 40.3527 40.2389 39.9837 38.5772 38.4634 38.2082 36.8340 36.7201 36.4649 延伸率δ/% 23.3498 23.1975 22.8563 22.0780 21.9257 21.5845 20.4916 20.3394 19.0082 18.9341 18.7819 18.4407 断面收缩率ψ/% 40.4680 40.2667 39.8157 38.6819 38.4807 38.0296 36.4541 36.2528 35.8081 34.2667 34.0654 33.6144
下载: 导出CSV
-
[1] FreudenthalA M. Safety of structure[J]. Trans. ASCE, 1947, 112: 125—128. [2] FreudenthalA M. Physical and statistical aspects of fatigue[J]. Adv. Appl. Mech., 1956, 4: 116—156. [3] KecociogluD. Reliability analysis of mechanical componentsand systems[J]. Nucl. Eng. Des., 1972, 19: 259—290. [4] HaugenE B. Probabilistic Mechanical Design[M]. NewYork: JohnWiley andSons, 1980. [5] Proven J W. Probability Fracture Mechanics and Reliability [M]. Dordrecht: Martnus Nijhoff Publishers, 1987. [6] TB/T 1335-1996, 铁道车辆强度设计及试验鉴定规范[S]. [7] 肖纪美. 铁路车轴钢40与50的比较[J]. 材料导报, 2000, 14(6): 7—8.XIAO Ji-mei. Som e comparison between steels50 and40 usedfor railroad axles[J]. Transactions onMaterials, 2000, 14(6): 7—8. (inChinese). [8] 钟群鹏. 对40、50轴钢的几点看法[J]. 材料导报, 2000, 14(6): 9—10. https://www.cnki.com.cn/Article/CJFDTOTAL-CLDB200006005.htmZHONG Qun-peng. The views on carbon steel40 and50 forthe vehicle shaft[J]. Transactions onMaterials, 2000, 14(6): 9—10. (inChinese). https://www.cnki.com.cn/Article/CJFDTOTAL-CLDB200006005.htm [9] MencikJ. Reliability assessment with small amount of experi-mental data[R]. InStructuralSafety andReliability: ICOSSAR'01.187—194. [10] 赵永翔. 低周疲劳短裂纹行为和可靠性分析[D]. 成都: 西南交通大学, 1998.ZHAO Yong-xiang. Short fatigue crack behavior and reliability analysis in low cycle fatigue[D]. Chengdu: Southwest Jiaotong University, 1998. (in Chinese) [11] Tanaka T, Sakai T, Iwaya T. Distribution characteristics of fatigue lives and fatigue strengths of ferrous metals by the analysis of P-N data in the JSMEdata base on fatigue strength of metallic materials[A]. In Statistical Research on Fatigue and Fracture[C]. London: Elsevier Applied Science Publishers, 1987.125-157. [12] Baldwin JD, Thacker JG. Astrain -based fatigue reliability analysis method[J]. ASME Journal of Mechanical Design, 1995, 117: 229-234. [13] The Committee on Fatigue and Fracture Reliability of the Co-mmittee on Structural Safety and Reliability of the Structural Division. Fatigue reliability: introduction[J]. J. Struct. Div. Proc. ASCE, 1982, 108(ST1): 3-23. [14] 赵永翔, 孙亚芳, 高庆. 分析常用7种统计分布的统一线性回归方法[J]. 机械强度, 2001, 23(1): 102—106. doi: 10.3321/j.issn:1001-9669.2001.01.028ZHAO Yong-xiang, SUN Ya-fang, GAO Qing. Unified linear regression method for the analysis of seven commonly used statistical distributions[J]. J. Mech. Strength, 2001, 23(1): 102-106. (in Chinese). doi: 10.3321/j.issn:1001-9669.2001.01.028 [15] Zhao Y X, Gao Q, Wang J N. An approach for determining an appropriate assumed distribution of fatigue life under limited data[J]. Reliab. Eng. Sys. Saf., 2000, 67(1): 1-7. doi: 10.1016/S0951-8320(99)00039-3 [16] Zhao Y X, Gao Q, Sun X F. A statistical investigation of the fatigue lives of Q235 steel-welded joints[J]. Fatigue Fract. Eng. Mater. Struct., 1998, 21: 781-790. [17] Zhao YX, Wang JN, Gao Q. Random cyclic stress-strain resp- onses of a stainless steel pipe-weld metal I-a statistical investigation[J]. Nucl. Eng. Des., 2000, 199: 303-314. [18] Zhao YX, Wang JN, Gao Q. Statistical evolution of small fatigue crack in 1Cr18Ni9Ti weld metal[J]. Theor. Appl. Fract. Mech., 1999, 32: 55-64. [19] Zhao Y X. Size evolution of the surface short fatigue cracks of 1Cr18Ni9Ti pipe-weld metal with a local viewpoint[J]. Mater. Sci. Eng. A, 2003, 344: 229-239. [20] 赵永翔, 王金诺, 高庆. 确定有限疲劳可靠性数据良好假设分布的一种统一方法[J]. 中国机械工程, 2001, 12(12): 1343—1347. doi: 10.3321/j.issn:1004-132X.2001.12.005ZHAO Yongxiang, WANG Jinnuo, GAO Qing. Unified approach for determining an appropriate assumed distribution of limited fatigue reliability data[J]. Journal of China Mech. Eng., 2001, 12(12): 1 343-1 347. (in Chinese) doi: 10.3321/j.issn:1004-132X.2001.12.005 -
点击查看大图
图(2) / 表(9)
计量
- 文章访问数: 477
- HTML全文浏览量: 192
- PDF下载量: 284
- 被引次数: 0
