Conflict resolution in free flight with linear programming
Article Text (Baidu Translation)
-
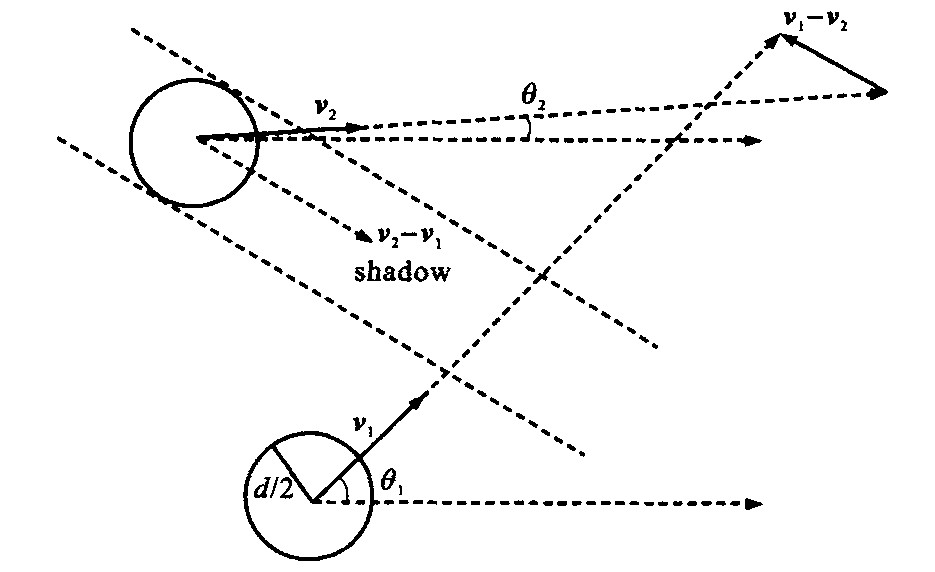
摘要: 为了避免可能的冲突, 任何两架飞行器之间的距离不能小于给定的安全距离, 采用线性规划的方法研究了同一空间中自由飞行的几架飞机之间的冲突解脱问题。提出了两种不同的解脱方案: 第一, 只允许速度变化; 第二, 只允许航向角变化。考虑到旅客在旅途中的舒适程度, 选取的目标函数要使上述改变量最小。针对两种方案分别列出了线性限制公式, 从而找出最优的解脱办法, 实例验证了该方法的可行性。Abstract: Aircrafts can not get closer to each other than a given safety distance in order to avoid their conflicts. Two different schemes were designed: the one only change flying velocity; the other only change flying angle. For passengers' comfort, the changes should be minimized. The linear formula respectly for the two kinds of schemes were deduced to find out the optimum resolution methods, an example shows that this method is feasible.
-
Key words:
- air traffic control /
- free flight /
- conflict resolution /
- linear programming
-
[1] Reich P G. Analysis of long-range air traffic system: separat-ion standards Ⅰ~Ⅲ[J]. Journal of the Institute ofNavigation, 1966, 19(1~3): 181-192. [2] Menon P K, Sweriduk G D, Sridhar B. Optimal strategies forfree-flight air traffic conflict resolution[J]. Journal ofGuidance, Control and Dynamics, 1998, 22(2): 202-211. [3] Oh J-H, Feron E. Fast detection and resolution of multipleconflicts for 3-dimensional free flight[A]. In Proceedings ofthe IEEE Conference on Decision and Control[C]. San Diego, CA, 1997.66-74. [4] MAO Zhi-hong, Eric Feron, Karl Bilimoria. Stability and per-formance of intersecting aircraft flows under decentralizedconflict avoidance rules[J]. IEEE Transactions on IntelligentTransportation Systems, 2001, 2(2): 101-109. [5] 甘应爱. 运筹学[M]. 北京: 清华大学出版社, 1990. [6] 骆慈孟. 空中交通管制学[M]. 北京: 中国民航出版社, 1993. [7] 孙台兴. 民航概论[M]. 北京: 中国民航出版社, 2000. [8] Hu J, Prandini M, Sastry S. Optimal maneuver for multipleaircraft resolution: a braid point of view[A]. In IEEEConference on Decision and Control[C]. Sidney, Australia, 2000. -





 下载:
下载: