Local buckling characteristics of stiffened rectangular plate on elastic foundation subjected to non-uniform loads
-
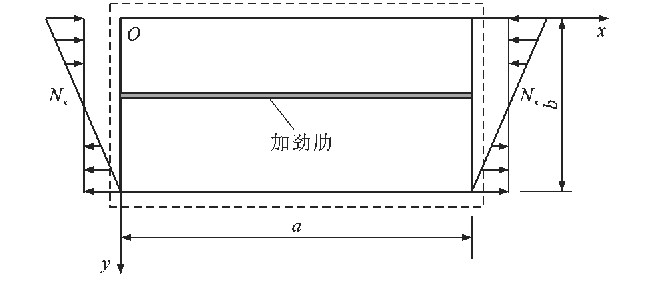
摘要: 针对弹性基底上板的局部稳定问题, 应用能量法推导了非均匀荷载作用下矩形加劲板的局部屈曲非线性特征方程, 建立了考虑弹性基底接触和纵向加劲肋作用的屈曲板迦辽金表达式; 基于牛顿迭代法, 建立了局部屈曲的非线性特征方程的增量迭代格式与屈曲荷载特征值的附加迭代方程。分析结果表明: 屈曲系数计算结果与有限元分析结果误差小于2%, 并且避免了有限元模拟的接触分析过程, 计算效率较高; 当荷载梯度为1时, 设置加劲肋的偏心构件的局部稳定性明显增强, 临界屈曲系数增加到51.1, 是普通板件的2.5倍; 加劲板件的纵向鼓曲波的长宽比约为0.6, 鼓曲波纵向排列相对密集, 而普通板件每个鼓曲波的长宽比约为1.0;在不增加加劲肋材料用量的前提下, 设置纵向加劲肋的最优位置为距离板件受压侧边缘的2/5板宽处, 临界屈曲系数增加为78.9, 是普通板件的4倍; 加劲肋的设置可将矩形钢管混凝土壁板的宽厚比增加到172, 将界限值提高2倍以上。可见, 在矩形钢管混凝土管壁设置纵向加劲肋能够有效提高偏压作用下管壁的局部稳定性, 改善矩形钢管混凝土的截面尺寸。Abstract: Based on the local stability of plate on elastic foundation, the energy method was adopted to deduce the nonlinear characteristic equation of local buckling for stiffened rectangular plate subjected to non-uniform loads.The effects of elastic foundation contact and longitudinal stiffening ribs were considered for plate's buckling analysis by using Galerkin method.The incremental iterative format of nonlinear characteristic equations of local buckling was given.The additional iterative equation of buckling characteristic value was proposed.Analysis result shows that the computational error of buckling coefficient is less than 2%compared with FEM, and the computational efficiency is higher because of avoiding the contact analysis process based on finite element simulation.When the load gradient is 1, the local stability of decentered component withstiffening ribs is greater because its buckling coefficient increases to 51.1that is 2.5times as large as the coefficient of the plate without stiffening ribs.The aspect ratio of buckling wave of stiffening plate is about 0.6, which shows relatively intensive arrangement.While the aspect ratio of buckling wave of non-stiffening plate is about 1.0.On the premise of unconverted sizes of stiffening rib, the optimal location of setting longitudinal stiffening rib is two fifths of plate's width distancing from the edge of plate's pressured side, and the critical buckling coefficient of stiffening plate increases to 78.9that is 4times as large as the value of non-stiffening plate.Because of the application of stiffening rib, the critical breadth-to-thickness ratio of rectangular concrete-filled steel tube increases to 172 that is 2times higher than the standard value.Obviously, when the longitudinal stiffening ribs are set on the wall of rectangular concrete-filled steel tube, the local stability of the wall effectively increases under the action of eccentric load, and the section sizes of rectangular concrete-filled steel tube are improved.
-
图 1 非均匀受压的矩形加劲板
Figure 1. Rectangular stiffened plate subjected to non-uniform compression
图 3 屈曲特征方程的迭代求解过程
Figure 3. Iterative solution process of buckling characteristic equation
图 6 屈曲系数与荷载梯度的关系曲线
Figure 6. Relationship curves between buckling coefficient and stress gradient
-
[1] UY B. Local and postlocal buckling of fabricated steel and composite cross sections[J]. Journal of Structural Engineering, 2001, 127 (6): 666-677. doi: 10.1061/(ASCE)0733-9445(2001)127:6(666) [2] SHAHWAN K W, WAAS A M. A mechanical model for the buckling of unilaterally constrained rectangular plates[J]. International Journal of Solids and Structures, 1994, 31 (1): 75-87. doi: 10.1016/0020-7683(94)90176-7 [3] BRADFORD M A, WRIGHT H D, UY B. Local buckling of the steel skin in lightweight composites induced by creep and shrinkage[J]. Advances in Structural Engineering, 1998, 2 (1): 25-34. doi: 10.1177/136943329800200104 [4] WRIGHT H D. Buckling of plates in contact with a rigid medium[J]. Structural Engineering, 1993, 71 (12): 209-215. [5] WRIGHT H D. Local stability of filled and encased steel sections[J]. Journal of Structural Engineering, 1995, 121 (10): 1382-1388. doi: 10.1061/(ASCE)0733-9445(1995)121:10(1382) [6] 何保康, 杨晓冰, 周天华. 矩形钢管混凝土轴压柱局部屈曲性能的解析分析[J]. 西安建筑科技大学学报: 自然科学版, 2002, 34 (3): 210-213. doi: 10.3969/j.issn.1006-7930.2002.03.002HE Bao-kang, YANG Xiao-bing, ZHOU Tian-hua. Theoretical analysis on local buckling behavior of concrete filled rectangular steel tube column subjected to axis force[J]. Journal of Xi'an University of Architecture and Technology: Natural Science Edition, 2002, 34 (3): 210-213. (in Chinese). doi: 10.3969/j.issn.1006-7930.2002.03.002 [7] CAI Jian, LONG Yue-ling. Local buckling of steel plates in rectangular CFT columns with binding bars[J]. Journal of Constructional Steel Research, 2009, 65 (4): 965-972. doi: 10.1016/j.jcsr.2008.07.025 [8] UY B, BRADFORD M A. Elastic local buckling of steel plates in composite steel-concrete members[J]. Engineering Structures, 1996, 18 (3): 193-200. doi: 10.1016/0141-0296(95)00143-3 [9] LIANG Q Q, UY B. Theoretical study on the post-local buckling of steel plates in concrete-filled box columns[J]. Computer and Structures, 2000, 75 (5): 479-490. doi: 10.1016/S0045-7949(99)00104-2 [10] CHAI H. Contact buckling and postbuckling of thin rectangular plates[J]. Journal of Mechanics and Physics of Solids, 2001, 49 (2): 209-230. doi: 10.1016/S0022-5096(00)00038-7 [11] BRADFORD M A, SMITH S T, OEHLERS D J. Semi-compact steel plates with unilateral restraint subjected to bending, compression and shear[J]. Journal of Constructional Steel Research, 2000, 56 (1): 47-67. doi: 10.1016/S0143-974X(99)00073-5 [12] SHAHWAN K W, WAAS A M. Buckling of unilaterally constrained plates: applications to the study of delaminations in layered structures[J]. Journal of the Franklin InstituteEngineering and Applied Mathematics, 1998, 335B (6): 1009-1039. [13] SHAHWAN K W, WAAS A M. Buckling of unilaterally constrained infinite plates[J]. Journal of Engineering Mechanics, 1998, 124 (2): 127-136. doi: 10.1061/(ASCE)0733-9399(1998)124:2(127) [14] MA X, BUTTERWORTH J W, CLIFTON C. Compressive buckling analysis of plates in unilateral contact[J]. International Journal of Solids and Structures, 2007, 44 (9): 2852-2862. [15] MA Xing, BUTTERWORTH J W, CLIFTON C. Initial compressive buckling of clamped plates resting on tensionless elastic or rigid foundations[J]. Journal of Engineering Mechanics, 2008, 134 (6): 514-518. [16] 刘永健, 李慧, 张宁. 非均匀受压矩形钢管混凝土局部弹性屈曲分析[J]. 建筑科学与工程学报, 2015, 32 (4): 1-8. doi: 10.3969/j.issn.1673-2049.2015.04.001LIU Yong-jian, LI Hui, ZHANG Ning. Local elastic buckling analysis of rectangular concrete-filled steel tube under non-uniform compression[J]. Journal of Architecture and Civil Engineering, 2015, 32 (4): 1-8. (in Chinese). doi: 10.3969/j.issn.1673-2049.2015.04.001 [17] HU H T, HUANG C S, WU M H, et al. Nonlinear analysis of axially loaded concrete-filled tube columns with confinement effect[J]. Journal of Structural Engineering, 2003, 129 (10): 1322-1329. [18] 刘永健, 张宁, 张俊光. PBL加劲型矩形钢管混凝土的力学性能[J]. 建筑科学与工程学报, 2012, 29 (4): 13-17. doi: 10.3969/j.issn.1673-2049.2012.04.003LIU Yong-jian, ZHANG Ning, ZHANG Jun-guang. Mechanical behavior of concrete-filled square steel tube stiffened with PBL[J]. Journal of Architecture and Civil Engineering, 2012, 29 (4): 13-17. doi: 10.3969/j.issn.1673-2049.2012.04.003 [19] 张耀春, 陈勇. 设直肋方形薄壁钢管混凝土短柱的试验研究与有限元分析[J]. 建筑结构学报, 2006, 27 (5): 16-22. doi: 10.3321/j.issn:1000-6869.2006.05.003ZHANG Yao-chun, CHEN Yong. Experimental study and finite element analysis of square stub columns with straight ribs of concrete-filled thin-walled steel tube[J]. Journal of Building Structures, 2006, 27 (5): 16-22. (in Chinese). doi: 10.3321/j.issn:1000-6869.2006.05.003 [20] 黄宏, 张安哥, 李毅, 等. 带肋方钢管混凝土轴压短柱试验研究及有限元分析[J]. 建筑结构学报, 2011, 32 (2): 75-82. https://www.cnki.com.cn/Article/CJFDTOTAL-JZJB201102012.htmHUANG Hong, ZHANG An-ge, LI Yi, et al. Experimental research and finite element analysis on mechanical performance of concrete-filled stiffened square steel tubular stub columns subjected to axial compression[J]. Journal of Building Structures, 2011, 32 (2): 75-82. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JZJB201102012.htm [21] LOPATIN A V, MOROZOV E V. Approximate buckling analysis of the CCFF orthotropic plates subjected to in-plane bending[J]. International Journal of Mechanical Sciences, 2014, 85 (1): 38-44. [22] 莫时旭, 钟新谷, 赵人达. 刚性基底上弹性约束矩形板的屈曲行为分析[J]. 工程力学, 2005, 22 (2): 174-178. https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX20050200U.htmMO Shi-xu, ZHONG Xin-gu, ZHAO Ren-da. Buckling behavior of elastically constrained rectangular plate on rigid base[J]. Engineering Mechanics, 2005, 22 (2): 174-178. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX20050200U.htm [23] PANDA S K, RAMACHANDRA L S. Buckling of rectangular plates with various boundary conditions loaded by nonuniform inplane loads[J]. International Journal of Mechanical Sciences, 2010, 52 (6): 819-828. [24] 秦凤江, 狄谨, 周绪红, 等. 钢箱梁U型肋加劲板受压稳定极限承载力影响因素分析[J]. 长安大学学报: 自然科学版, 2017, 37 (1): 58-66. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL201701008.htmQIN Feng-jiang, DI Jin, ZHOU Xu-hong, et al. Analysis on influence factors of stability ultimate bearing capacity of U-rib stiffened plate of steel box girder[J]. Journal of Chang'an University: Natural Science Edition, 2017, 37 (1): 58-66. (in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-XAGL201701008.htm [25] BORST R D, CRISFIED M A, REMMERS J J C, et al. Nonlinear Finite Element Analysis of Solids and Structures[M]. Chichester: John Wiley & amp; amp; Sons, Ltd., 2012. [26] LEISSA A W, KANG J H. Exact solutions for vibration and buckling of an SS-C-SS-C rectangular plate loaded by linearly varying in-plane stresses[J]. International Journal of Mechanical Sciences, 2002, 44 (9): 1925-1945. -
下载:
点击查看大图
图(13) / 表(1)
计量
- 文章访问数: 833
- HTML全文浏览量: 194
- PDF下载量: 486
- 被引次数: 0
