Suppression effect of rail vibration absorber on self-excited vibration of wheel-rail friction
-
摘要: 基于摩擦自激振动理论和现场调研,建立了DTVI2和科隆蛋扣件结构支撑下的小半径曲线区段轮对-钢轨-吸振器系统有限元模型,采用复特征值分析和瞬时动态分析研究了2种扣件区段钢轨吸振器对钢轨波磨的抑制效果;通过最小二乘法和粒子群算法研究了钢轨吸振器的不同参数对钢轨波磨的影响规律,确定2种扣件下钢轨吸振器的最优参数组合。研究结果表明:安装钢轨吸振器前后,轮对-钢轨-吸振器系统发生摩擦自激振动的主要频率为480 Hz;DTVI2扣件的最大垂向振动加速度由270.01 m·s-2下降到206.07 m·s-2,降低了23.71 %,功率谱密度(PSD)峰值由98.98 dB下降到94.92 dB;科隆蛋扣件的最大垂向振动加速度由300.97 m·s-2下降到211.44 m·s-2,降低了29.74%,PSD峰值由101.58 dB下降到95.14 dB;在DTVI2扣件区段中,钢轨吸振器质量为14.0 kg,连接刚度为9.0×106 N·m-1,连接阻尼为1.0×105 N·s·m-1时对钢轨波磨的抑制效果最好;在科隆蛋扣件区段中,钢轨吸振器质量为7.5 kg,连接刚度为1.07×107 N·m-1,连接阻尼为1.0×105 N·s·m-1时钢轨波磨的抑制效果最好。可见,安装钢轨吸振器能够有效抑制轮轨系统的摩擦自激振动,从而抑制钢轨波磨的产生和发展;钢轨吸振器对科隆蛋扣件区段钢轨波磨的抑制效果优于DTVI2扣件区段;合理选取钢轨吸振器的连接参数会进一步抑制钢轨波磨的产生和发展。Abstract: Based on the frictional self-excited vibration theory and field investigation, the finite element model of the wheelset-rail-vibration absorber system in the small-radius curve section supported by DTVI2 fasteners and Cologne egg fasteners were established. The suppression effect of the two kinds of rail vibration absorbers on the rail corrugation was studied by two methods of complex eigenvalue analysis and instantaneous dynamic analysis. The effect laws of different parameters of rail vibration absorber on rail corrugation were analyzed by the least square method and particle swarm algorithm, and the optimal parameter combinations of rail vibration absorber under two kinds of fasteners were determined. Research results show that the main frequency of the frictional self-excited vibration of the wheelset-rail-vibration absorber system is 480 Hz before and after installing the rail vibration absorber. The maximum vertical vibration acceleration of the DTVI2 fastener decreases from 270.01 m·s-2 to 206.07 m·s-2, representing a decline of 23.71%. The peak value of power spectral density (PSD) reduces from 98.98 dB to 94.92 dB. The maximum vertical vibration acceleration of the Cologne-egg fastener decreases from 300.97 m·s-2 to 211.44 m·s-2, representing a decline of 29.74%. The peak value of PSD reduces from 101.58 dB to 95.14 dB. In the DTVI2 fastener section, the suppression effect on rail corrugation is the best when the mass of the rail vibration absorber is 14.0 kg, the connection stiffness is 9.0×106 N·m-1 and the connection damping is 1.0×105 N·s·m-1. In the Cologne-egg fastener section, the suppression effect on rail corrugation is the best when the mass of the rail vibration absorber is 7.5 kg, the connection stiffness is 1.07×107 N·m-1 and the connection damping is 1.0×105 N·s·m-1. It can be seen that the installation of rail vibration absorbers can effectively suppress the frictional self-excited vibration of the wheel-rail system, thereby suppressing the formation and development of rail corrugation. The suppression effect of the rail vibration absorber on rail corrugation in the Cologne-egg fastener section is better than that in the DTVI2 fastener section. Appropriate selection of the connection parameters of the rail vibration absorber can suppress the formation and development of rail corrugation.
-
0. 引言
随着轨道交通的迅速发展,越来越多的轨道损伤问题开始凸显,其中钢轨波磨是轨道损伤的代表性问题。钢轨波磨的存在会加剧车辆行驶时的振动,引起轨道基础设施的损坏,严重影响乘客乘车体验和行车安全[1-4]。为更好地从根源上抑制钢轨波磨的产生和发展,国内外较多学者对钢轨波磨的形成机理进行探究:陈光雄等[5]发现轮轨间的饱和蠕滑力导致摩擦自激振动,从而导致钢轨波磨的产生;尧辉明等[6]提出了一种钢轨波浪形磨耗的微观波长锁定拓展机制,发现钢轨表面凸起处磨损比凹陷处磨损小;Jin等[7]发现轨道振动在垂直和水平方向被激发出的固有频率对钢轨波磨的形成和发展有很大影响。目前抑制钢轨波磨的主要方法是人工打磨和安装吸振器,人工打磨可以改善钢轨表面损伤但不能抑制钢轨波磨的再生,而安装钢轨吸振器可从根源上对钢轨波磨进行一定程度的抑制[8],但在一些轨道结构区段安装钢轨吸振器后仍会有钢轨波磨的出现。国内外较多学者从不同角度探究了钢轨吸振器对钢轨波磨的抑制效果:钱韦吉等[9]基于轮轨摩擦自激振动理论,建立了轮对-钢轨-吸振器系统的有限元模型,研究发现轮轨摩擦自激振动的频率范围与钢轨波磨的发生频率范围相重合,进而得出钢轨吸振器对摩擦自激振动有明显的抑制作用,从而实现钢轨波磨的有效抑制;邹钰等[10]通过安装复合吸振器,研究了钢轨的Pinned-Pinned振动,发现复合吸振器比单自由度吸振器更加优越,在此基础上利用遗传算法得到吸振器的最佳设计频率;Wu[11]建立了带调频轨道减振器的复合轨道模型,发现减振器采用较大质量有利于提高钢轨的衰减率,离散式减振器的有效位置在轨枕跨中间;孙晓静等[12]通过安装调频式钢轨阻尼器,钢轨振动衰减率提高了4~8倍,抑制竖向振动沿钢轨纵向传播;Thompson等[13]设计出一种调谐阻尼系统,研究发现钢轨阻尼器改变钢轨的动态响应,可减少轮轨系统的动态响应;Tao等[14]研究了动力吸振器的参数对减振效果的影响,确定了动力吸振器参数的最优值,从而提高吸振器的减振能力;Zhao等[15]建立了轮轨振动的动力学模型来分析轮轨振动特性,发现钢轨阻尼器的应用是控制地铁线路短距钢轨波磨的一种长期有效的方法;Bellette等[16]修正了磨损型钢轨波纹的反馈模型,研究发现车辆的速度变化越大,钢轨波磨的生长速率越低。
综上所述,前期研究都在注重钢轨吸振器本身的结构和特性对钢轨波磨的抑制效果,忽略了不同轨道结构下钢轨吸振器对钢轨波磨抑制效果的对比,并且不同轨道结构下钢轨吸振器的设计参数也存在差异,因此,本文基于轮轨系统的摩擦自激振动理论,分别建立了DTVI2扣件和科隆蛋扣件支撑下的轮对-钢轨-吸振器系统有限元模型,采用复特征值和瞬时动态两种分析方法,探究了钢轨吸振器对钢轨波磨的抑制机理,对比在2种扣件结构支撑下钢轨吸振器对钢轨波磨的抑制效果。通过对DTVI2和科隆蛋扣件的钢轨吸振器设计参数进行拟合,并采用粒子群算法确定钢轨吸振器的最优参数组合,进而针对不同轨道结构提出钢轨吸振器的最优抑振参数。
1. 钢轨波磨区段现场调研
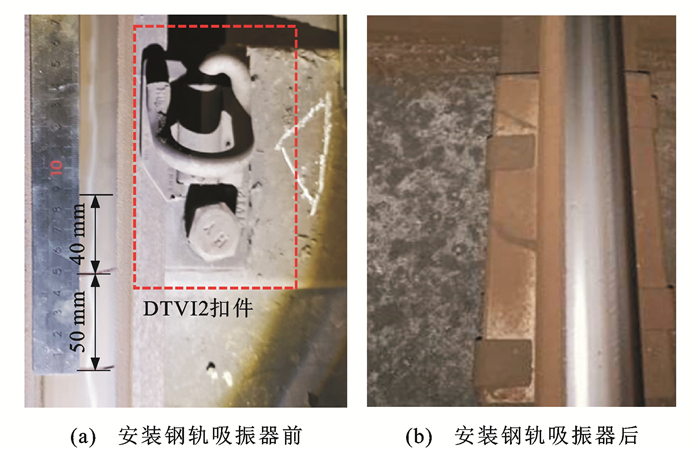
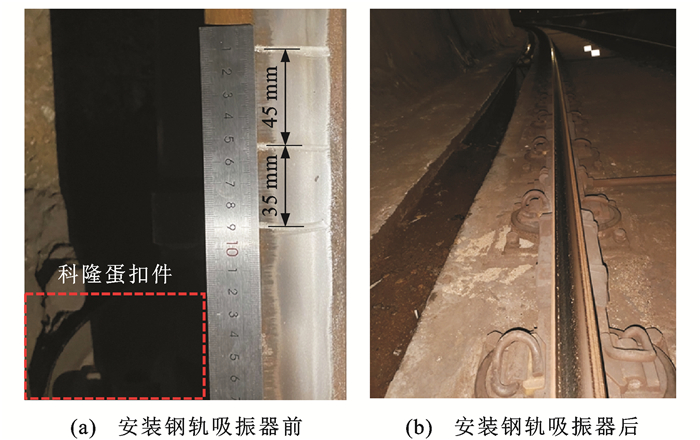
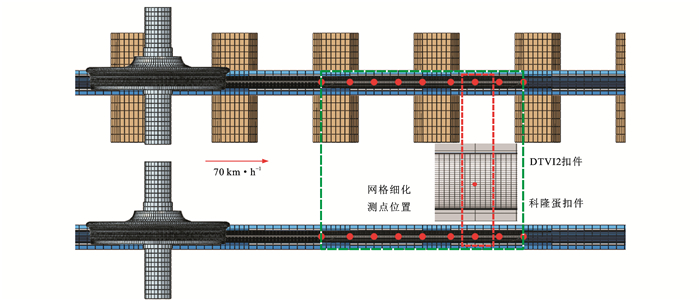
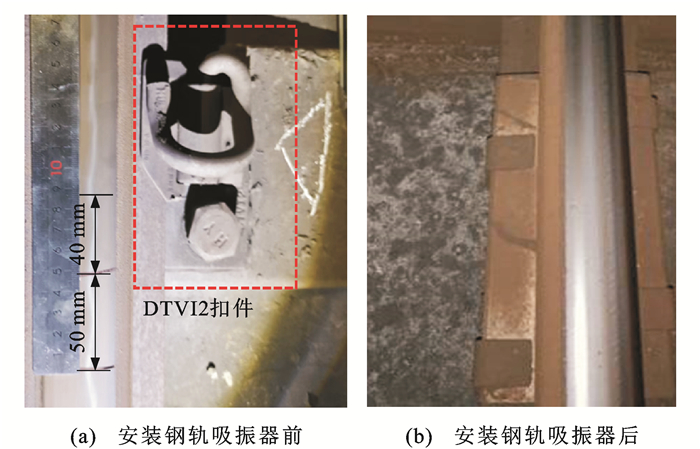
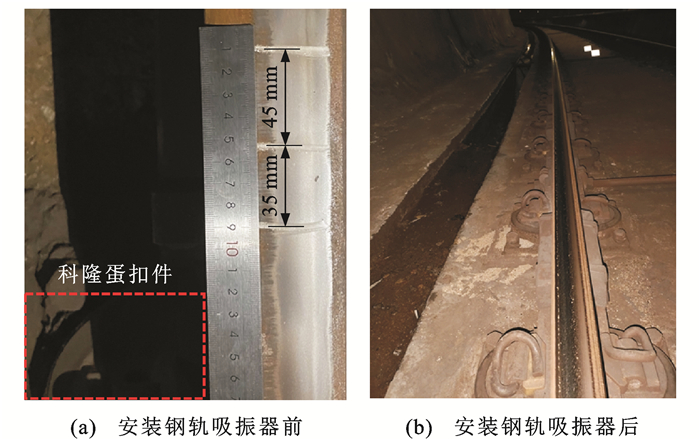
小半径曲线区段是钢轨波磨的高发区段[17],在不同扣件支撑的地铁线路中均有不同程度的钢轨波磨发生。地铁线路的扣件主要包括非减振型和减振型,非减振扣件主要有DTVI2扣件、弹条Ⅲ型扣件,减振扣件主要有科隆蛋扣件、先锋扣件。本文主要选取了重庆轨道1号线双碑-石井坡DTVI2扣件和较场口-小什字科隆蛋扣件支撑的小半径曲线处进行了现场调研。2个调研区段的线路条件基本一致:线路的钢轨型号选用60 kg·m-1,轨底坡为1/40,扣件间距为625 mm,曲线半径为350 m。2种扣件结构支撑区段均有钢轨波磨出现,为抑制钢轨波磨的发展,在扣件之间的钢轨轨腰处安装了钢轨吸振器[18]。双碑-石井坡区段为短轨枕支撑的小半径曲线区段,如图 1所示,测量发现此路段的低轨上出现了波长为40~50 mm的钢轨波磨。经过计算,诱发钢轨波磨的振动频率为390~490 Hz,安装钢轨吸振器后钢轨波磨得到有效抑制,经打磨后2~3月的钢轨表面无明显波磨现象。较场口-小什字区段为科隆蛋扣件支撑的小半径曲线区段,由图 2所示,测量发现此路段的低轨上出现了波长约为35~45 mm的钢轨波磨。经过计算,诱发钢轨波磨的振动频率约为430~560 Hz,安装吸振器后钢轨波磨得到有效抑制,经打磨后5~6个月钢轨表面仍无明显波磨。综上所述,安装吸振器前,科隆蛋扣件区段与DTVI2扣件区段的钢轨波磨特征基本一致,科隆蛋扣件区段钢轨波磨的形成周期比DTVI2扣件区段短;安装吸振器后,2种扣件区段的钢轨波磨现象均得到有效抑制,科隆蛋扣件区段的钢轨波磨形成周期比DTVI2扣件区段更长。
2. 数值仿真模型及理论方法
2.1 轮对-钢轨-吸振器系统的有限元模型
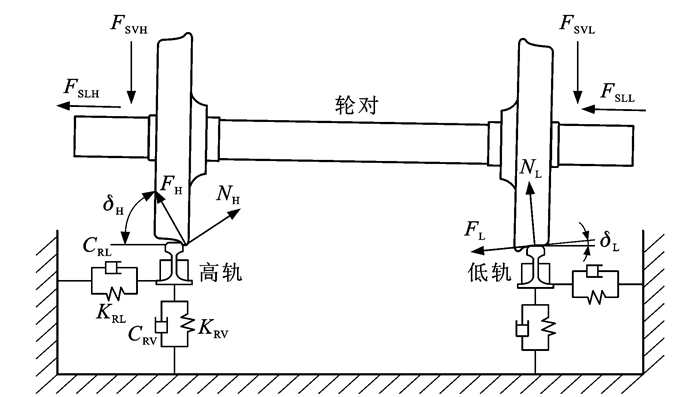
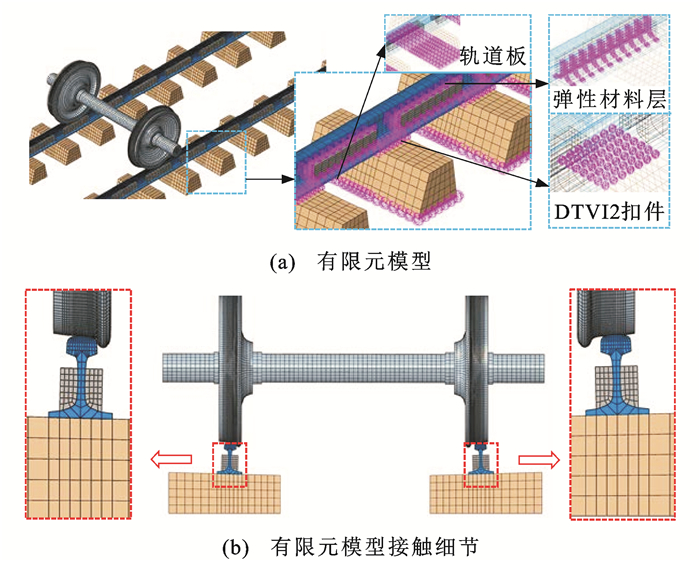
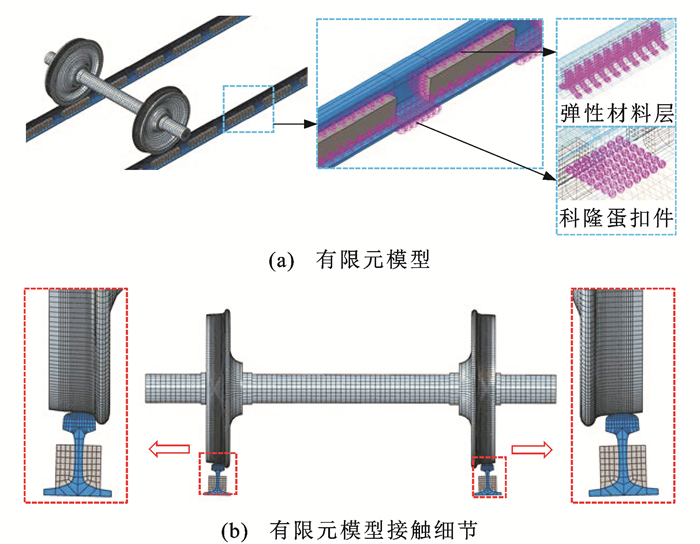
本节以重庆地铁1号线双碑-石井坡DTVI2扣件和较场口-小什字科隆蛋扣件支撑的小半径曲线区段作为对比研究对象,将2种扣件下的轮轨系统简化成如下的接触模型,如图 3所示,其中:δH和δL分别为导向轮对左侧和右侧与钢轨的接触角;FSVH、FSVL分别为轮对两端受到垂向悬挂力;FSLH、FSLL分别为轮对两端受到横向悬挂力;NH和NL分别为左、右轮轨间的法向接触力;FH和FL分别为左、右轮轨间的蠕滑力;KRV和CRV分别为扣件的垂向刚度和垂向阻尼;KRL和CRL分别为扣件的横向刚度和横向阻尼。
由于DTVI2扣件和科隆蛋扣件区段的轨下结构存在差异, 本文基于小半径曲线区段导向轮对-钢轨-吸振器系统的接触模型,分别创建了2种轮对-钢轨-吸振器系统的有限元模型。DTVI2扣件的轮对-钢轨-吸振器系统有限元模型如图 4所示。科隆蛋扣件的轮对-钢轨-吸振器系统有限元模型如图 5所示。由图 4、5可知:轮对-钢轨-吸振器系统的有限元模型主要包括轮对、钢轨、扣件和钢轨吸振器;其中轮对中车轮滚动圆直径为840 mm,车轮踏面为磨耗型踏面。为避免钢轨长度较短产生端面效应对有限元分析结果产生影响,模型中的钢轨长度为20 m,并对钢轨两端进行固定约束[19]。DTVI2扣件支撑的轮对-钢轨-吸振器系统有限元模型通过在短轨枕底部添加点对面弹簧阻尼单元模拟轨道板的支撑作用,在钢轨底部与短轨枕之间采取点对点弹簧-阻尼系统连接模拟扣件。而科隆蛋扣件支撑的轮对-钢轨-吸振器系统有限元模型在钢轨底部添加点对面弹簧单阻尼元模拟轨道板的支撑和扣件的减振作用。在钢轨吸振器的模型中均采用点对点弹簧-阻尼系统模拟钢轨吸振器弹性材料层连接质量块和钢轨[12, 20]。根据重庆轨道1号线的现场测试数据,并综合其他文献的相关数据[21-22]。轮对-钢轨-吸振器系统的有限元模型中材料参数如表 1所示,DTVI2扣件系统的有限元模型连接参数如表 2所示,科隆蛋扣件系统的有限元模型连接参数如表 3所示。
表 1 轮对-钢轨-吸振器系统有限元模型材料参数Table 1. Material parameters of finite element model of wheelset- rail-vibration absorber system部件 密度/(kg·m-3) 弹性模量/MPa 泊松比 轮对 7.8×103 2.06×105 0.3 钢轨 7.8×103 2.06×105 0.3 轨枕 2.8×103 1.90×105 0.3 钢轨吸振器 7.9×103 1.90×105 0.3 表 2 DTVI2扣件轮对-钢轨-吸振器系统的有限元模型连接参数Table 2. Finite element model connection parameters of wheelset- rail-vibration absorber system with DTVI2 fastener连接参数 横向 垂向 纵向 钢轨扣件刚度/(N·m-1) 8.8×106 4.1×107 8.8×106 钢轨扣件阻尼/(N·s·m-1) 1.9×103 9.9×103 1.9×103 轨道支撑刚度/(N·m-1) 5.0×107 8.9×107 5.0×107 轨道支撑阻尼/(N·s·m-1) 4.0×104 8.9×104 4.0×104 钢轨吸振器刚度/(N·m-1) 1.5×107 3.0×107 1.5×107 钢轨吸振器阻尼/(N·s·m-1) 2.0×104 4.0×104 2.0×104 表 3 科隆蛋扣件轮对-钢轨-吸振器系统的有限元模型连接参数Table 3. Finite element model connection parameters of wheelset- rail-vibration absorber system with Cologne-egg fastener连接参数 横向 垂向 纵向 钢轨扣件刚度/(N·m-1) 7.6×106 1.2×107 7.6×106 钢轨扣件阻尼/(N·s·m-1) 1.0×103 1.4×103 1.0×103 钢轨吸振器刚度/(N·m-1) 1.5×107 3.0×107 1.5×107 钢轨吸振器阻尼/(N·s·m-1) 2.0×104 4.0×104 2.0×104 2.2 轮对-钢轨-吸振器系统的摩擦自激振动理论
基于摩擦自激振动理论,本文采用复特征值分析法和瞬时动态分析法分别从频域和时域角度研究2种扣件下钢轨吸振器对轮轨系统摩擦自激振动特性的影响[23-24]。首先在复特征值分析过程中,考虑轮轨间存在摩擦滑动作用,轮轨子系统的运动方程为
\boldsymbol{M}_1 \ddot{x}_1+\boldsymbol{C}_1 \dot{x}_1+\boldsymbol{K}_1 x_1=\boldsymbol{q}_1 (1) 式中:M1、C1、K1分别为轮轨子系统的质量矩阵、阻尼矩阵和刚度矩阵;x1为轮轨子系统的节点位移;q1为轮轨子系统中轮轨接触面的摩擦力。
吸振器子系统的动力学公式为
\boldsymbol{M}_2 \ddot{x}_2+\boldsymbol{C}_2 \dot{x}_1+\boldsymbol{K}_2 x_2=\boldsymbol{q}_2 (2) 式中:M2、C2、K2分别为吸振器子系统的质量矩阵、阻尼矩阵和刚度矩阵;x2为吸振器系统的节点位移;q2为吸振器子系统中轮轨接触面的摩擦力。
将轮轨子系统和吸振器子系统进行耦合得到轮对-钢轨-吸振器系统的动力学公式,即
\boldsymbol{M}_3 \ddot{x}_3+\boldsymbol{C}_3 \dot{x}_3+\boldsymbol{K}_3 x_3=\boldsymbol{q}_3 (3) 式中:M3、C3、K3分别为轮对-钢轨-吸振器系统的质量矩阵、阻尼矩阵和刚度矩阵;x3为轮对-钢轨-吸振器系统的节点位移;q3为轮对-钢轨-吸振器系统中轮轨接触面的摩擦力[25]。
q3由刚度和位移的函数进行表示
\boldsymbol{q}_3=\mu \boldsymbol{K}_4 x_4 (4) 式中:μ为接触面的摩擦因数;x4为轮轨系统局部摩擦接触的节点位移;K4为轮轨系统局部接触的刚度矩阵。
将式(3)和(4)合并后进行整理可得
\boldsymbol{M}_3 \ddot{x}_3+\boldsymbol{C}_3 \dot{x}_3+\left(\boldsymbol{K}_3-\mu \boldsymbol{K}_4\right) x_3=0 (5) \boldsymbol{M}_3 \ddot{x}_3+\boldsymbol{C}_3 \dot{x}_3+\boldsymbol{K}_5 x_3=0 (6) 式中:K5为非对称矩阵。
相应的特征值方程为
\left(\lambda^2 \boldsymbol{M}_3+\lambda \boldsymbol{C}_3+\boldsymbol{K}_5\right) \boldsymbol{\varphi}=0 (7) 式中:λ为系统的特征值;φ为系统的特征向量。
通过子空间投影法对式(7)进行简化得到投影,空间中的运动方程为
\left(\lambda^2 \boldsymbol{M}_4+\lambda \boldsymbol{C}_4+\boldsymbol{K}_6\right) \boldsymbol{\varphi}_k=0 (8) 式中:M4、C4、K6分别为投影后系统的质量矩阵、阻尼矩阵和刚度矩阵;φk为该系统对应的第k阶特征向量。
对式(8)进行全部广义特征值求解,得
x(t)=\sum\limits_{k=1}^n \boldsymbol{\varphi}_k \exp \left(\alpha_k+\mathrm{j} \omega_k\right) t (9) 式中:x(t)为轮对-钢轨-吸振器系统t时刻的位移;αk为特征值的第k阶实部;ωk为特征值的第k阶虚部。
当复特征值实部α出现正值时,随着时间继续增加,该系统的不稳定振动越容易发生。通常情况下,当复特征值实部出现正值时,复特征值实部越大则系统越容易发生摩擦自激振动[26]。故本文通过复特征值实部大小来判断系统发生摩擦自激振动的可能性,从而对比钢轨吸振器在小半径曲线区段对钢轨波磨的抑制效果进行判断。
瞬时动态分析法主要有显式和隐式时间积分2种求解方法。本文选用显式时间积分法进行计算,在本文的分析中,整个系统的平衡方程是通过每一个时间增量步的方式来建立(时刻t),其中
\ddot{x}_{(t)}=\left(\boldsymbol{F}_{\mathrm{w} t}-\boldsymbol{F}_{\mathrm{n} t}\right) \boldsymbol{N}^{-1} (10) \left\{\begin{array}{l} \dot{x}_{(t+0.5 \Delta t)}=\dot{x}_{(t-0.5 \Delta t)}+\frac{t_{(t+\Delta t)}+t_{(t)}}{2} \ddot{x}_{(t)} \\ x_{(t+\Delta t)}=x_{(t)}+t_{(t+\Delta t)} \dot{x}_{(t+0.5 \Delta t)} \end{array}\right. (11) 式中:Fnt为t时刻的内力向量;Fwt为t时刻的外力向量;N为对角集中质量矩阵;x(t)为t时刻的位移;Δt为t时刻的时间增量。
由于系统的初始状态为稳定状态,即当t=0的时刻,系统的速度和加速度为0。依据式(11), 对系统的加速度进行时域积分,通过瞬时动态分析法计算得到轮轨系统的瞬时动态响应。轮轨系统的垂向振动越剧烈则钢轨波磨产生的可能性越大[9],因此,本文通过对比安装吸振器前后轮轨系统的垂向振动加速度幅值,从而对比钢轨吸振器在小半径曲线区段对钢轨波磨的抑制效果。
3. 结果分析
3.1 轮对-钢轨-吸振器系统的复特征值
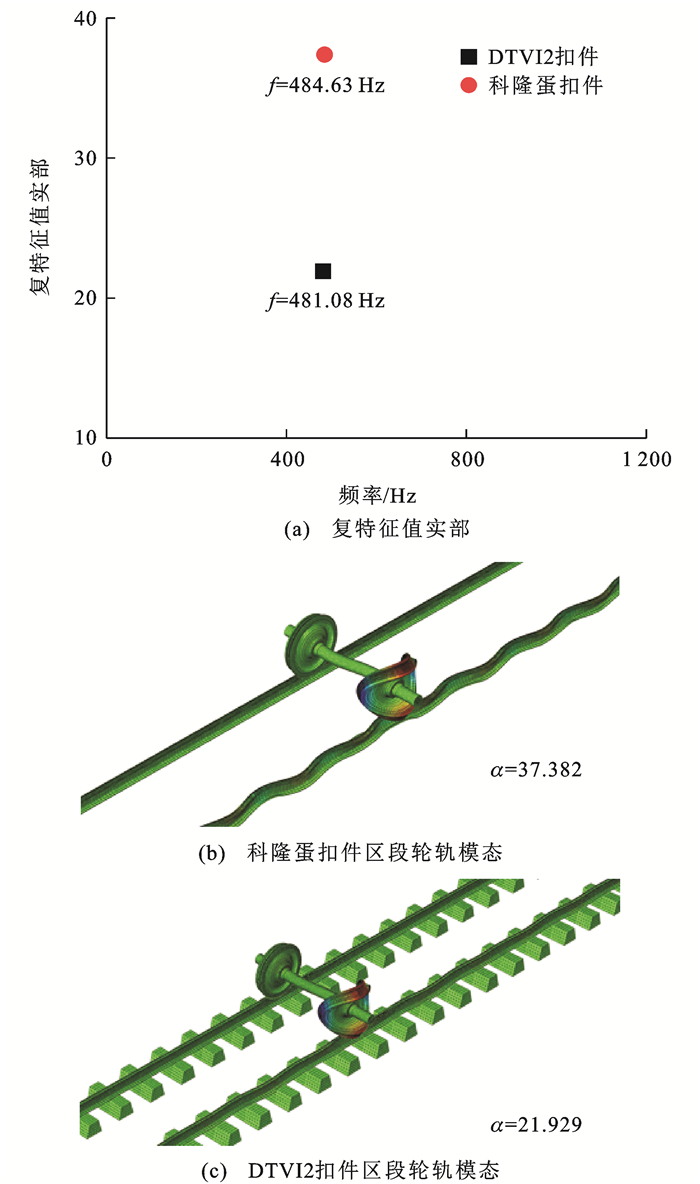
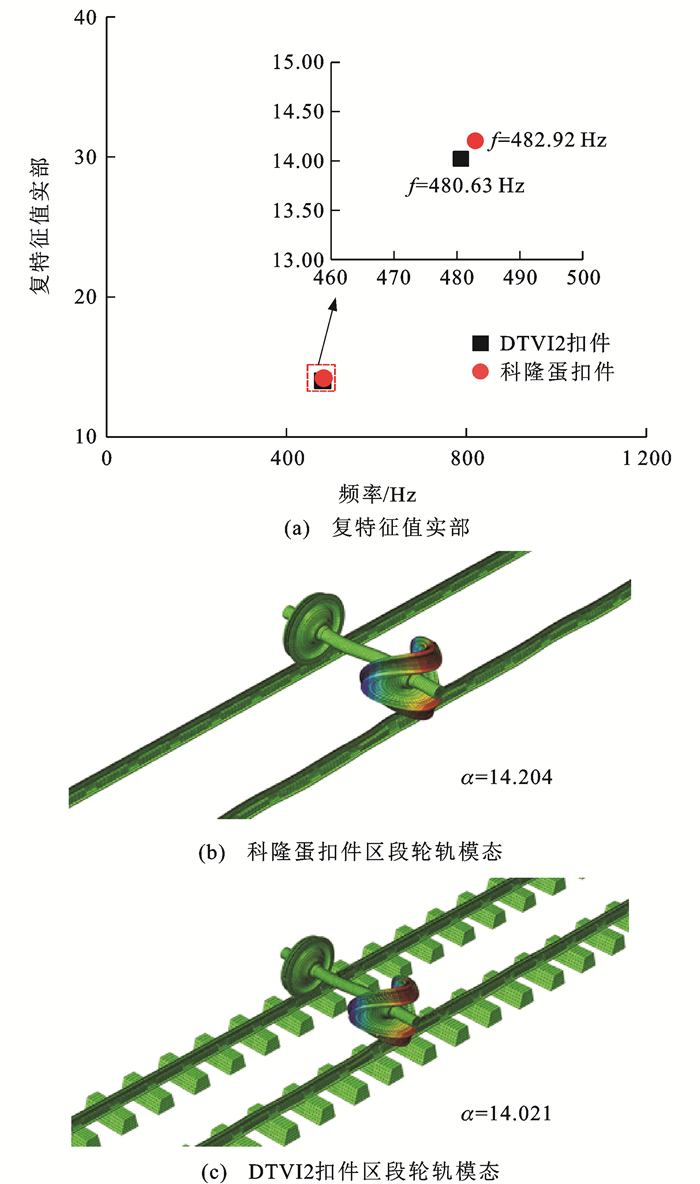
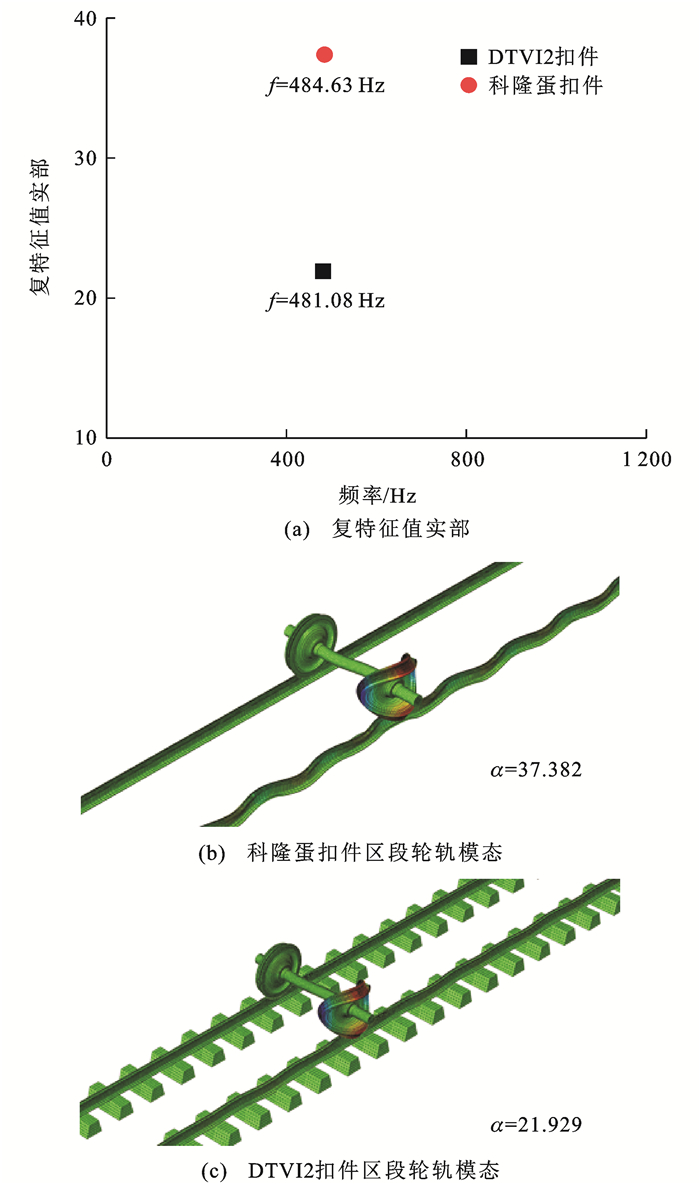
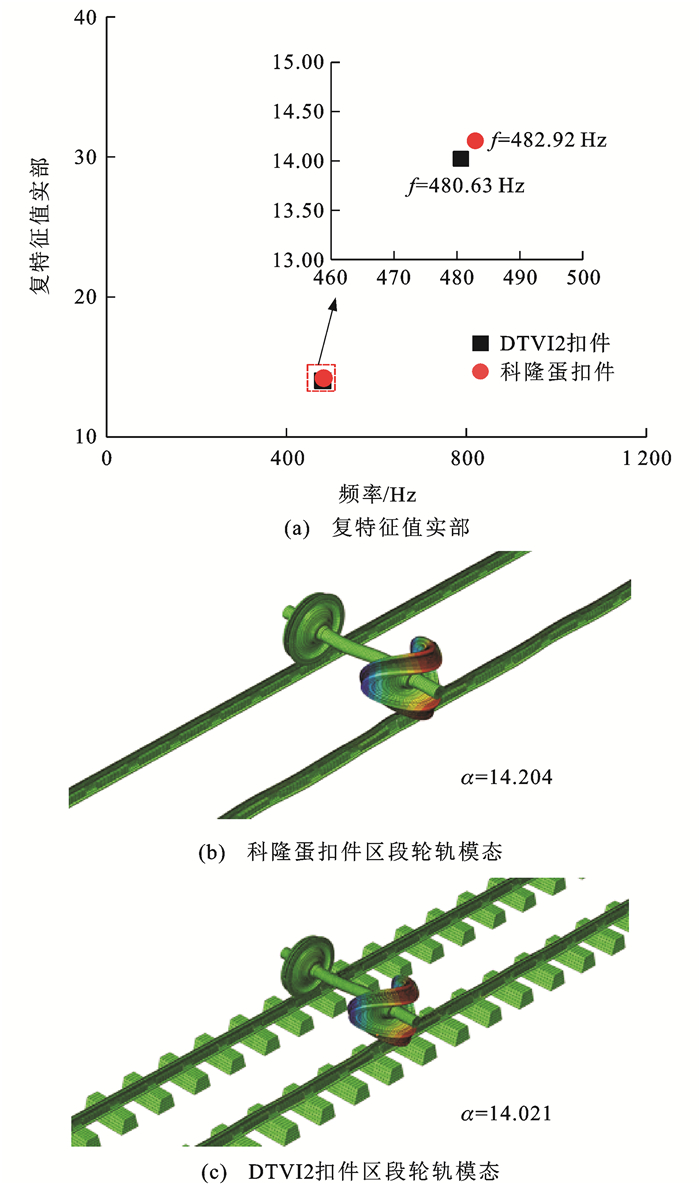
本节采用复特征分析法从频域角度分析DTVI2扣件和科隆蛋扣件区段安装吸振器前后轮对-钢轨-吸振器系统的摩擦自激振动特性,如图 6、7所示。由图 6可知:安装吸振器前,科隆蛋扣件支撑的轮轨系统中复特征值实部37.382对应频率约为484.63 Hz,DTVI2扣件支撑的轮轨系统中复特征值实部21.929对应频率约为481.08 Hz。由图 7可知:安装吸振器后,科隆蛋扣件的轮对-钢轨-吸振器系统中复特征值实部14.204对应频率约为482.92 Hz,DTVI2扣件的轮对-钢轨-吸振器系统中复特征值实部14.021对应频率约为480.63 Hz。安装吸振器前后,轮轨系统发生不稳定振动的主要频率几乎保持不变,但安装吸振器后的复特征值实部明显减小。证明安装钢轨吸振器后轮对-钢轨-吸振器系统发生摩擦自激振动的振动强度降低。并且科隆蛋扣件区段上的复特征值实部数值降低比DTVI2扣件区段大。
综上所述:(1)安装吸振器前,车辆通过小半曲线区段时轮轨系统的不稳定振动主要在低轨处发生;(2)科隆蛋扣件区段比DTVI2扣件区段的摩擦自激振动更剧烈;(3)通过安装钢轨吸振器可以有效抑制钢轨波磨的产生和发展,在科隆蛋扣件区段安装钢轨吸振器对抑制轮轨系统摩擦自激振动的效果更加明显。
3.2 轮对-钢轨-吸振器系统的瞬时动态分析
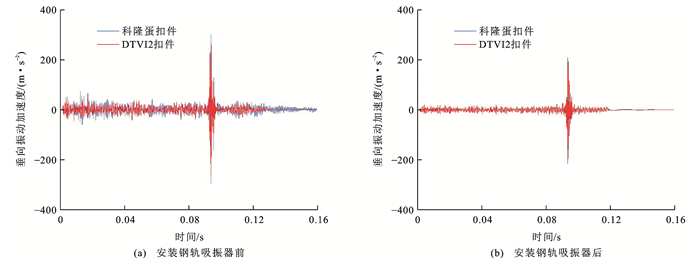
本节通过瞬时动态分析法得到轮对-钢轨-吸振器系统的瞬时动态响应,将钢轨表面的垂向振动加速度变化情况作为关注点。为保证结果的准确性,将测点所在的计算区域进行网格细化,2条钢轨的振动加速度测点依次均匀地分布在轮轨接触面上,提取计算结果的测点要远离车轮的初始位置来避免轮轨初始运动的干扰[27],2种扣件支撑下的钢轨表面测点位置见图 8。通过瞬时动态分析法得到轮对-钢轨-吸振器系统的垂向振动加速度,如图 9所示,可知:安装吸振器前,2种扣件支撑的轮轨系统均发生了剧烈的垂向振动,DTVI2扣件区段的振动强度低于科隆蛋扣件区段;安装吸振器后,2种扣件支撑的轮轨系统垂向振动强度均降低,科隆蛋扣件的最大垂向振动加速度由300.97 m·s-2下降到211.44 m·s-2;DTVI2扣件的最大垂向振动加速度由270.01 m·s-2下降到206.07 m·s-2。综上所述,科隆蛋扣件区段的垂向振动幅值比DTVI2扣件区段下降多,进而钢轨吸振器对科隆蛋扣件区段的抑振效果优于DTVI2扣件区段。
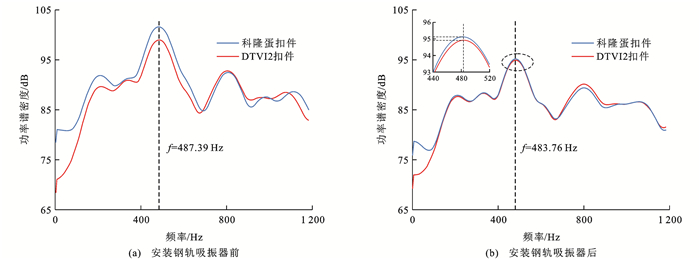
结合对轮对-钢轨-吸振器系统的瞬时动态分析结果,对图 8中测点处的垂向加速度进行了功率谱密度(Power Spectral Density, PSD)分析[28],如图 10所示,可知:在轮对-钢轨-吸振器系统未安装吸振器时,车辆通过小半径曲线区段时,轮对-钢轨-吸振器系统发生不稳定振动的趋势主要是487.39 Hz,DTVI2扣件和科隆蛋扣件区间的PSD峰值约为98.98和101.58 dB;安装钢轨吸振器后,轮对-钢轨-吸振器系统发生摩擦自激振动的主要频率为483.76 Hz,DTVI2扣件和科隆蛋扣件区间的PSD峰值约为94.92和95.14 dB。综上所述,安装吸振器后轮对-钢轨-吸振器系统的振动能量明显减轻,钢轨吸振器对科隆蛋扣件区段振动能量的抑制效果优于DTVI2扣件区段。
基于轮轨系统的摩擦自激振动理论,车辆通过未安装钢轨吸振器的小半曲线区段时,轮轨系统的不稳定振动主要在低轨处发生,与现场钢轨波磨的发生频率和位置吻合,从而轮轨系统发生的摩擦自激振动会诱导该区段产生钢轨波磨[29]。安装钢轨吸振器后,从轮轨系统的稳定性、振动幅值、振动能量等方面证明安装钢轨吸振器可以有效降低轮轨系统的摩擦自激振动,进而能一定程度有效抑制钢轨波磨的产生和发展,且在科隆蛋扣件区段的钢轨吸振器对钢轨波磨的抑制效果更优。
3.3 轮对-钢轨-吸振器系统的钢轨吸振器参数影响
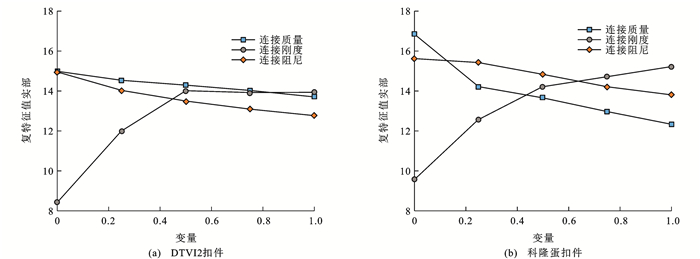
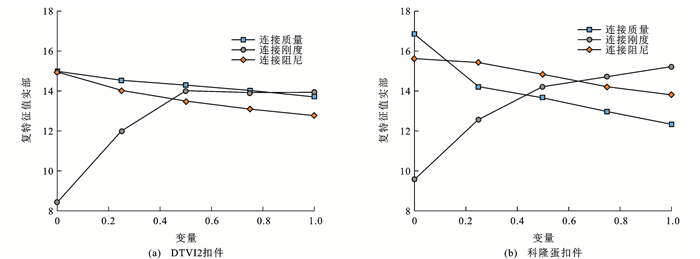
钢轨吸振器作为轨道交通中减振降噪的重要部件,其相关参数对轮轨系统的摩擦自激振动存在重要影响。结合2.1节中轮对-钢轨-吸振器系统的有限元模型,采用控制变量法探究吸振器参数对轮轨系统摩擦自激振动的影响规律。选取钢轨吸振器质量变化范围为4~14 kg[30-31],刚度范围为9.0×106~ 2.1×107 N·m-1,连接阻尼范围为2.0×104~1.0× 105 N·s·m-1[32]。对各参数进行归一化处理,参数之间的变量间隔设置为0.25。DTVI2扣件和科隆蛋扣件的初始参数作为控制变量,通过改变单一变量找到对轮对-钢轨-吸振器系统摩擦自激振动特性影响较大的参数。不同扣件下参数变化对轮对-钢轨-吸振器系统的摩擦自激振动影响规律如图 11所示,可知:以钢轨吸振器质量作为变量,复特征值实部随质量增加而减小;以吸振器连接刚度为变量,复特征值实部随吸振器连接刚度增加而增加;以吸振器连接阻尼作为变量,复特征值实部随连接阻尼增加而减小;对比钢轨吸振器3种参数影响下的结果变化,发现吸振器质量和吸振器连接刚度的参数对轮对系统摩擦自激振动特性影响较大。可见,适当地减小吸振器的连接刚度,增加吸振器的质量和连接阻尼可以一定程度上抑制钢轨波磨的产生。钢轨吸振器的相关参数对钢轨吸振效果的影响很大,在半径曲线区段,安装钢轨吸振器对由饱和蠕滑力引发的轮轨系统摩擦自激振动具有了良好的抑制效果。进而能预防和抑制短波波磨的产生和发展。但安装钢轨吸振器的缺点是只能抑制钢轨波磨的产生,延长钢轨波磨的生成时间,不能完全消除钢轨波磨。
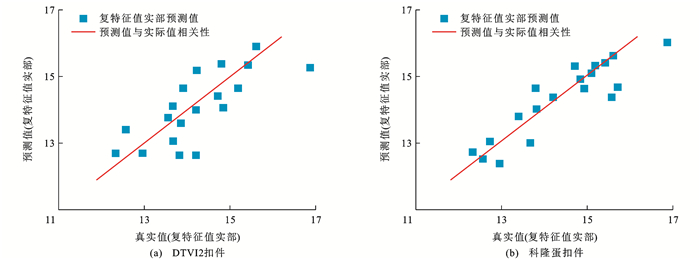
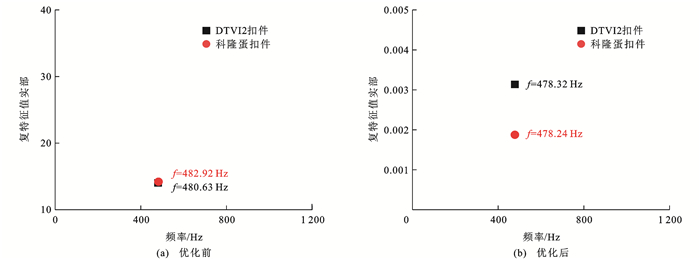
通过控制变量法得到单个参数对轮对-钢轨-吸振器系统摩擦自激振动特性的影响规律,如图 12所示,可知:拟合方程对复特征实部的预测值与真实值拟合效果较好。然后采用粒子群算法进行验证求解,选择位置的最小值和最大值分别为0和1,粒子初始速度最大值和最小值分别为-1和1。DTVI2扣件通过粒子群算法所得最优解为(1.00, 0.00, 1.00),即钢轨吸振器质量为14 kg,连接刚度为9.0× 106 N·m-1,连接阻尼为1.0×105 N·s·m-1。科隆蛋扣件通过粒子群算法所得最优解为(0.70, 0.89, 1.00),即钢轨吸振器质量为7.5 kg,连接刚度为1.1× 107 N·m-1,连接阻尼为1.0×105 N·s·m-1。采用复特征分析法从频域角度分析钢轨吸振器参数优化后轮对-钢轨-吸振器系统的摩擦自激振动特性,如图 13所示,可知:DTVI2扣件的复特征值实部为3.13×10-3;科隆蛋扣件的复特征值实部为1.87×10-3。综上所述,对比参数优化前后,采用优化后的参数的系统复特征值实部变小,因此,参数优化后能够有效抑制钢轨波磨的产生。
4. 结语
(1) 安装钢轨吸振器前,轮轨系统的摩擦自激振动诱导钢轨波磨产生。安装钢轨吸振器后,2种扣件区段轮轨系统的摩擦自激振动都得到有效的缓解,进而抑制了钢轨波磨的产生。
(2) 钢轨吸振器对科隆蛋扣件区段钢轨波磨的抑制效果优于DTVI2扣件区段。其中吸振器质量和吸振器连接阻尼的参数对轮对系统摩擦自激振动特性影响较大。
(3) 在DTVI2扣件的轮对-钢轨-吸振器系统中,钢轨吸振器质量为14 kg,连接刚度为9.0×106 N·m-1,连接阻尼为1.0×105 N·s·m-1对钢轨波磨的抑制效果最好。
(4) 在科隆蛋扣件的轮对-钢轨-吸振器系统中,钢轨吸振器质量为7.5 kg,连接刚度为1.1×107 N·m-1,连接阻尼为1.0×105 N·s·m-1对钢轨波磨的抑制效果最好。
(5) 研究成果为小半径曲线区段钢轨吸振器的参数选取提供了一定的参考。后续可以考虑从钢轨吸振器的结构设计方面来优化摩擦自激振动的抑制效果。
-
表 1 轮对-钢轨-吸振器系统有限元模型材料参数
Table 1. Material parameters of finite element model of wheelset- rail-vibration absorber system
部件 密度/(kg·m-3) 弹性模量/MPa 泊松比 轮对 7.8×103 2.06×105 0.3 钢轨 7.8×103 2.06×105 0.3 轨枕 2.8×103 1.90×105 0.3 钢轨吸振器 7.9×103 1.90×105 0.3 表 2 DTVI2扣件轮对-钢轨-吸振器系统的有限元模型连接参数
Table 2. Finite element model connection parameters of wheelset- rail-vibration absorber system with DTVI2 fastener
连接参数 横向 垂向 纵向 钢轨扣件刚度/(N·m-1) 8.8×106 4.1×107 8.8×106 钢轨扣件阻尼/(N·s·m-1) 1.9×103 9.9×103 1.9×103 轨道支撑刚度/(N·m-1) 5.0×107 8.9×107 5.0×107 轨道支撑阻尼/(N·s·m-1) 4.0×104 8.9×104 4.0×104 钢轨吸振器刚度/(N·m-1) 1.5×107 3.0×107 1.5×107 钢轨吸振器阻尼/(N·s·m-1) 2.0×104 4.0×104 2.0×104 表 3 科隆蛋扣件轮对-钢轨-吸振器系统的有限元模型连接参数
Table 3. Finite element model connection parameters of wheelset- rail-vibration absorber system with Cologne-egg fastener
连接参数 横向 垂向 纵向 钢轨扣件刚度/(N·m-1) 7.6×106 1.2×107 7.6×106 钢轨扣件阻尼/(N·s·m-1) 1.0×103 1.4×103 1.0×103 钢轨吸振器刚度/(N·m-1) 1.5×107 3.0×107 1.5×107 钢轨吸振器阻尼/(N·s·m-1) 2.0×104 4.0×104 2.0×104 -
[1] 李伟, 曾全君, 朱士友, 等. 地铁钢轨波磨对车辆和轨道动态行为的影响[J]. 交通运输工程学报, 2015, 15(1): 34-42. doi: 10.19818/j.cnki.1671-1637.2015.01.005LI Wei, ZENG Quan-jun, ZHU Shi-you, et al. Effect of metro rail corrugation on dynamic behaviors of vehicle and track[J]. Journal of Traffic and Transportation Engineering, 2015, 15(1): 34-42. (in Chinese) doi: 10.19818/j.cnki.1671-1637.2015.01.005 [2] 金学松, 李霞, 李伟, 等. 铁路钢轨波浪形磨损研究进展[J]. 西南交通大学学报, 2016, 51(2): 264-273. https://www.cnki.com.cn/Article/CJFDTOTAL-XNJT201602007.htmJIN Xue-song, LI Xia, LI Wei, et al. Review of rail corrugation progress[J]. Journal of Southwest Jiaotong University, 2016, 51(2): 264-273. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XNJT201602007.htm [3] CHEN G X, ZHOU Z R, QUYANG H, et al. A finite element study on rail corrugation based on saturated creep force-induced self-excited vibration of a wheelset-track system[J]. Journal of Sound and Vibration, 2010, 329(22): 4643-4655. doi: 10.1016/j.jsv.2010.05.011 [4] WU Bo-wen, CHEN Guang-xiong, LYU Jin-zhou, et al. Generation mechanism and remedy method of rail corrugation at a sharp curved metro track with Vanguard fasteners[J]. Journal of Low Frequency Noise, Vibration, and Active Control, 2020, 39(2): 368-381. doi: 10.1177/1461348419845992 [5] 陈光雄, 钱韦吉, 莫继良, 等. 轮轨摩擦自激振动引起小半径曲线钢轨波磨的瞬态动力学[J]. 机械工程学报, 2014, 50(9): 71-76. https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201409010.htmCHEN Guang-xiong, QIAN Wei-ji, MO Ji-liang, et al. A transient dynamics study on wear-type rail corrugation on a tight curve due to the friction-induced self-excited vibration of a wheelset-track system[J]. Journal of Mechanical Engineering, 2014, 50(9): 71-76. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201409010.htm [6] 尧辉明, 沈钢, 高利君. 基于试验验证的磨耗型钢轨波磨形成机理[J]. 同济大学学报(自然科学版), 2018, 46(10): 1427-1432. https://www.cnki.com.cn/Article/CJFDTOTAL-TJDZ201810016.htmYAO Hui-ming, SHEN Gang, GAO Li-jun. Formation mechanism of worn profile rail corrugation based on experimental verification[J]. Journal of Tongji University (Natural Science), 2018, 46(10): 1427-1432. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TJDZ201810016.htm [7] JIN Xue-song, WEN Ze-feng, ZHANG Wei-hua, et al. Numerical simulation of rail corrugation on a curved track[J]. Computers and Structures, 2005, 83(25/26): 2052-2065. [8] 尚文军. 钢轨吸振器对地铁钢轨波磨抑制作用的研究[J]. 现代城市轨道交通, 2015(3): 57-61. https://www.cnki.com.cn/Article/CJFDTOTAL-XDGD201503019.htmSHANG Wen-jun. Study of metro rail vibration absorber to reduce rail corrugation[J]. Modern Urban Transit, 2015(3): 57-61. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XDGD201503019.htm [9] 钱韦吉, 黄志强. 蠕滑力饱和条件下钢轨吸振器抑制短波波磨的理论研究[J]. 振动与冲击, 2019, 38(14): 68-73, 111. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201914010.htmQIAN Wei-ji, HUANG Zhi-qiang. Theoretical study on the suppression of short pitch rail corrugation induced vibration by rail vibration absorbers under saturated creep forces condition[J]. Journal of Vibration and Shock, 2019, 38(14): 68-73, 111. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201914010.htm [10] 邹钰, 文永蓬, 纪忠辉, 等. 车轨耦合下钢轨复合吸振器的减振方法[J]. 振动、测试与诊断, 2021, 41(5): 888-896, 1031. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCS202105009.htmZOU Yu, WEN Yong-peng, JI Zhong-hui, et al. Vibration reduction method of rail composite shock absorber with vehicle-track coupling[J]. Journal of Vibration, Measurement and Diagnosis, 2021, 41(5): 888-896, 1031. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCS202105009.htm [11] WU Tian-xing. On the railway track dynamics with rail vibration absorber for noise reduction[J]. Journal of Sound and Vibration, 2008, 309(3/4/5): 739-755. [12] 孙晓静, 张厚贵, 刘维宁, 等. 调频式钢轨阻尼器对剪切型减振器轨道动力特性的影响[J]. 振动与冲击, 2016, 35(14): 209-214. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201614034.htmSUN Xiao-jing, ZHANG Hou-gui, LIU Wei-ning, et al. Effect of tuning rail damper on dynamic properties of the track structure using Egg fastening system[J]. Journal of Vibration and Shock, 2016, 35(14): 209-214. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201614034.htm [13] THOMPSON D J, JONES C J C, WATERS T P, et al. A tuned damping device for reducing noise from railway track[J]. Applied Acoustics, 2007, 68(1): 43-57. doi: 10.1016/j.apacoust.2006.05.001 [14] TAO Tian-you, WANG Hao, YAO Cheng-yuan, et al. Parametric sensitivity analysis on the buffeting control of a long-span triple-tower suspension bridge with MTMD[J]. Applied Sciences, 2017, 7(4): 395. doi: 10.3390/app7040395 [15] ZHAO Cai-you, WANG Ping, SHENG Xi, et al. Theoretical simulation and experimental investigation of a rail damper to minimize short-pitch rail corrugation[J]. Mathematical Problems in Engineering, 2017, 2017: 2359404. [16] BELLETTE P A, MEEHAN P A, DANIEL W J T. Effects of variable pass speed on wear-type corrugation growth[J]. Journal of Sound and Vibration, 2008, 314(3/4/5): 616-634. [17] 关庆华, 张斌, 熊嘉阳, 等. 地铁钢轨波磨的基本特征、形成机理和治理措施综述[J]. 交通运输工程学报, 2021, 21(1): 316-337. doi: 10.19818/j.cnki.1671-1637.2021.01.015 GUAN Qing-hua, ZHANG Bin, XIONG Jia-yang, et al. Review on basic characteristics, formation mechanisms, and treatment measures of rail corrugation in metro systems[J]. Journal of Traffic and Transportation Engineering, 2021, 21(1): 316-337. (in Chinese) doi: 10.19818/j.cnki.1671-1637.2021.01.015 [18] CETTOUR-JANET R, BARBARULO A, LETOURNEAUX F, et al. An Arnoldi reduction strategy applied to the semi-analytical finite element method to model railway track vibrations[J]. Mechanical Systems and Signal Processing, 2019, 116: 997-1016. doi: 10.1016/j.ymssp.2018.07.013 [19] 田彩. 一种共振原理钢轨降噪结构的减振降噪特性分析[D]. 成都: 西南交通大学, 2019.TIAN Cai. Analysis of vibration and noise reduction characteristics of rail noise reduction structure based on resonance principle[D]. Chengdu: Southwest Jiaotong University, 2019. (in Chinese) [20] 杨新文, 赵治钧, 钱鼎玮, 等. 钢轨底部动力吸振器对钢轨振动与噪声的影响[J]. 哈尔滨工业大学学报, 2021, 53(3): 42-50. https://www.cnki.com.cn/Article/CJFDTOTAL-HEBX202103006.htmYANG Xin-wen, ZHAO Zhi-jun, QIAN Ding-wei, et al. Influence of dynamic vibration absorber beneath rail base on rail vibration and noise[J]. Journal of Harbin Institute of Technology, 2021, 53(3): 42-50. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-HEBX202103006.htm [21] 崔晓璐, 陈光雄, 杨宏光. 轮对结构和扣件刚度对钢轨波磨的影响[J]. 西南交通大学学报, 2017, 52(1): 112-117. https://www.cnki.com.cn/Article/CJFDTOTAL-XNJT201701016.htmCUI Xiao-lu, CHEN Guang-xiong, YANG Hong-guang. Influence of wheelset structure and fastener stiffness on rail corrugation[J]. Journal of Southwest Jiaotong University, 2017, 52(1): 112-117. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XNJT201701016.htm [22] 崔晓璐, 钱韦吉, 张青, 等. 直线线路科隆蛋扣件地段钢轨波磨成因的理论研究[J]. 振动与冲击, 2016, 35(13): 114-118, 152. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201613019.htmCUI Xiao-lu, QIAN Wei-ji, ZHANG Qing, et al. Forming mechanism of rail corrugation of a straight track section supported by Cologne-egg fasteners[J]. Journal of Vibration and Shock, 2016, 35(13): 114-118, 152. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201613019.htm [23] ABUBAKAR A R, OUYANG H J. Complex eigenvalue analysis and dynamic transient analysis in predicting disc brake squeal[J]. International Journal of Vehicle Noise and Vibration, 2006, 2(2): 143. doi: 10.1504/IJVNV.2006.011051 [24] OUYANG H J, NACK W, YUAN Y B, et al. Numerical analysis of automotive disc brake squeal: a review[J]. International Journal of Vehicle Noise and Vibration, 2005, 1(3/4): 207. doi: 10.1504/IJVNV.2005.007524 [25] EL BESHBICHI O, WAN C, BRUNI S, et al. Complex eigenvalue analysis and parameters analysis to investigate the formation of railhead corrugation in sharp curves[J]. Wear, 2020, 450/451: 203150. doi: 10.1016/j.wear.2019.203150 [26] 许洋, 赵新利, 徐涆文, 等. 钢轨动力吸振器减振降噪特性分析[J]. 噪声与振动控制, 2021, 41(2): 219-224. https://www.cnki.com.cn/Article/CJFDTOTAL-ZSZK202102038.htmXU Yang, ZHAO Xin-li, XU Han-wen, et al. Analysis of vibration and noise reduction characteristics of rail vibration absorbers[J]. Noise and Vibration Control, 2021, 41(2): 219-224. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZSZK202102038.htm [27] 崔晓璐, 吕东, 李童, 等. 山地地铁浮置板轨道支撑结构钢轨波磨机制研究[J]. 润滑与密封, 2022, 47(6): 52-58. https://www.cnki.com.cn/Article/CJFDTOTAL-RHMF202206007.htmCUI Xiao-lu, LYU Dong, LI Tong, et al. Study on rail corrugation mechanism of floating slab track support structure in mountain metro[J]. Lubrication Engineering, 2022, 47(6): 52-58. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-RHMF202206007.htm [28] CARRIGAN T D, TALBOT J P. A new method to derive rail roughness from axle-box vibration accounting for track stiffness variations and wheel-to-wheel coupling[J]. Mechanical Systems and Signal Processing, 2023, 192: 110232. doi: 10.1016/j.ymssp.2023.110232 [29] XU J, CUI X L, DING H H, et al. Optimization of vibration absorbers for the suppression of rail corrugation in the sharp curved section with Cologne-egg fasteners[J]. Vehicle System Dynamics, 2024, 62(2): 395-410. doi: 10.1080/00423114.2023.2170255 [30] 文永蓬, 纪忠辉, 翁琳, 等. 双重钢轨吸振器对轨道系统的振动抑制研究[J]. 机械工程学报, 2020, 56(12): 184-195. https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB202012023.htmWEN Yong-peng, JI Zhong-hui, WENG Lin, et al. Study on vibration suppression of track system via double rail vibration absorber[J]. Journal of Mechanical Engineering, 2020, 56(12): 184-195. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB202012023.htm [31] 陈彦恒, 谢小山. 钢轨动力吸振器对地铁车轨振动的影响分析[J]. 铁道标准设计, 2017, 61(8): 37-41. https://www.cnki.com.cn/Article/CJFDTOTAL-TDBS201708008.htmCHEN Yan-heng, XIE Xiao-shan. Influence of dynamic vibration absorber for rail on vehicle-track vibrations[J]. Railway Standard Design, 2017, 61(8): 37-41. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDBS201708008.htm [32] 赵悦, 肖新标, 韩健, 等. 高速有砟轨道钢轨动力吸振器垂向吸振特性及其参数影响[J]. 机械工程学报, 2013, 49(16): 17-25. https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201316004.htmZHAO Yue, XIAO Xin-biao, HAN Jian, et al. Vertical characteristic and its parameter effect of rail vibration absorber used in high-speed ballasted track[J]. Journal of Mechanical Engineering, 2013, 49(16): 17-25. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201316004.htm 期刊类型引用(1)
1. 雷震宇,王志强. 基于缩尺试验的第三介质对波磨演化影响研究. 铁道工程学报. 2025(01): 26-30 .  百度学术
百度学术其他类型引用(0)
-






 下载:
下载:












 下载:
下载:




 百度学术
百度学术