Vertical vibration control of curved track structure based on inertial enhancement effect
Article Text (Baidu Translation)
-
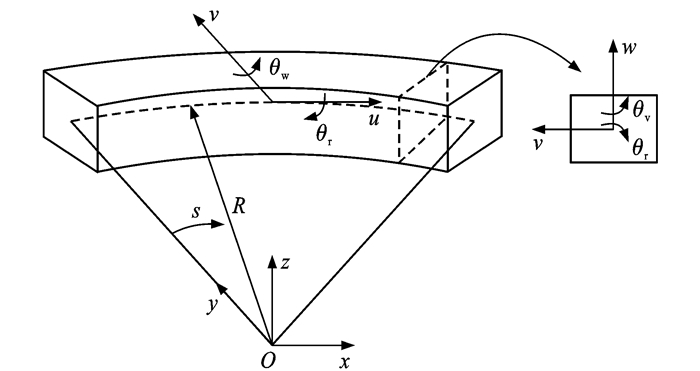
摘要: 针对曲线轨道的垂向振动控制，基于惯性增强效应，引入了调谐质量阻尼惯容器(TMDI)和振幅放大型调谐质量阻尼器(AM-TMD)，以实现更佳的振动控制效果；将曲线轨道考虑为离散支承的曲线Timoshenko梁结构，采用能量泛函变分法建立了有限长曲线轨道分析模型；在曲线钢轨两端引入完美匹配层作为低反射边界条件，用于更好地模拟无限长轨道结构；通过与已有无限长离散支承曲线轨道动力响应计算结果的对比，验证了分析模型的准确性以及完美匹配层的有效性；分析了垂向固定谐荷载作用下，调谐质量阻尼器(TMD)、TMDI和AM-TMD对曲线轨道动力响应的影响，评估了TMD、TMDI和AM-TMD的减振性能；分析了振幅放大系数与TMD工作能力之间的关系，揭示了AM-TMD的工作机理。研究结果表明：TMDI的引入有效地弥补了传统TMD在实现宽频控制时的质量缺陷，与TMD相比，同参数的TMDI工作带宽拓宽约1.5倍，最大振动衰减提升了约5.5 dB；AM-TMD的实质在于利用振幅放大机构来同步增大TMD的有效质量、刚度和阻尼，进而全面提升TMD的工作能力，与TMD相比，同参数的AM-TMD工作带宽拓宽约2.0倍，最大振动衰减提升了约6.1 dB。可见，从宽频控制、高衰减率的角度考虑，TMDI、AM-TMD比TMD更具优势。Abstract: In view of vertical vibration control of curved tracks, tuned mass damper inerter (TMDI) and amplitude magnification tuned mass damper (AM-TMD) were introduced based on inertial enhancement effect to realize better vibration control effects. Curved track was considered as a curved Timoshenko beam structure with discrete supports, and the energy functional variational method was used to establish a finite length curved track analysis model. Perfectly matched layer was introduced at both ends of curved track as a low reflection boundary condition for better simulating the infinite length track structure. The accuracy of analysis model and the effectiveness of perfectly matched layer were verified through the comparison with the dynamic response calculation results of existing infinite length discrete supported curved tracks. The effects of tuned mass damper (TMD), TMDI, and AM-TMD on the dynamic response of curved tracks under the action of fixed vertical harmonic load were systematically analyzed, and the vibration damping performances of TMD, TMDI, and AM-TMD were compared and evaluated. The relationship between the amplitude magnification factor and the operational capability of TMD was analyzed, and the working mechanism of AM-TMD was revealed. Research results show that the introduction of TMDI can efficiently make up for the quality defects of traditional TMD in implementing broadband control. Compared with TMD, the operating bandwidth of TMDI with the same parameters is broadened by about 1.5 times, and the maximum vibration attenuation rate increases by about 5.5 dB. The essence of AM-TMD lies in applying an amplitude magnification mechanism to synchronously enhance the effective mass, stiffness, and damping of TMD, thereby comprehensively improving the operational capability of TMD. Compared with TMD, the operating bandwidth of AM-TMD with the same parameters is expanded by about 2.0 times, and the maximum vibration attenuation rate increases by about 6.1 dB. Therefore, TMDI and AM-TMD are more effective than TMD from the perspectives of broadband control and high attenuation rate.
-
Key words:
- urban rail transit /
- curved track /
- vibration control /
- inertial enhancement effect /
- inerter /
- amplitude magnification
-
图 6 曲线钢轨跨中垂向位移频响函数
Figure 6. Frequency response function of vertical displacement at mid-span of curved rail
图 8 TMD对曲线钢轨振动衰减率的影响
Figure 8. Influence of TMD on vibration decay rate of curved rail
图 9 TMD参数对曲线钢轨振动衰减率的影响
Figure 9. Influences of TMD parameters on vibration decay rate of curved rail
图 11 TMDI对曲线钢轨振动衰减率的影响
Figure 11. Influence of TMDI on vibration decay rate of curved rail
图 13 AM-TMD对曲线钢轨动力响应的影响
Figure 13. Influence of AM-TMD on dynamic response of curved rail
图 14 AM-TMD对曲线钢轨振动衰减率的影响
Figure 14. Influence of AM-TMD on vibration decay rate of curved rail
图 15 TMD、TMDI与AM-TMD减振性能综合对比分析
Figure 15. Comprehensive comparative analysis of vibration reduction performance between TMD, TMDI and AM-TMD
表 1 T60钢轨与DTVI2型扣件参数
Table 1. Parameters of T60 rail and DTVI2 fastener
参数 数值 A/m2 7.745×10-3 E/Pa 2.059×1011 G/Pa 7.919×1011 Iy/m4 3.217×10-5 I0/m4 3.714×10-5 J/m4 2.150×10-6 ηr 0.01 ρ/(kg·m-3) 7 830 R/m 300 κ 0.532 9 kw/(MN·m-1) 40 cw/(kN·s·m-1) 30 kr/(kN·m·rad-1) 225 cr/(N·m·s·rad-1) 160 ls/m 0.6 
下载: 导出CSV
-
[1] ZHANG Hou-gui, LIU Wei-ning, LIU Wei-feng, et al. Study on the cause and treatment of rail corrugation for Beijing metro[J]. Wear, 2014, 317(1/2): 120-128. [2] 袁扬, 刘维宁, 刘卫丰. 基于现场测试的曲线段地铁地面振动传播规律[J]. 中国铁道科学, 2012, 33(4): 133-138. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK201204025.htmYUAN Yang, LIU Wei-ning, LIU Wei-feng. Propagation law of ground vibration in the curve section of metro based on in-situ measurement[J]. China Railway Science, 2012, 33(4): 133-138. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK201204025.htm [3] 黄强, 姚湘静, 黄宏伟, 等. 地铁运行时轨道-隧道-地层振动实测与分析[J]. 振动、测试与诊断, 2018, 38(2): 260-265, 416. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCS201802008.htmHUANG Qiang, YAO Xiang-jing, HUANG Hong-wei, et al. Field measurement and analysis of train-induced vibration in the whole track-tunnel-ground system[J]. Journal of Vibration, Measurement and Diagnosis, 2018, 38(2): 260-265, 416. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCS201802008.htm [4] ZHOU Li, SHEN Zhi-yun. Dynamic analysis of a high-speed train operating on a curved track with failed fasteners[J]. Journal of Zhejiang University—Science A, 2013, 14(6): 447-458. doi: 10.1631/jzus.A1200321 [5] MARTÍNEZ-CASAS J, DI GIALLEONARDO E, BRUNI S, et al. A comprehensive model of the railway wheelset-track interaction in curves[J]. Journal of Sound and Vibration, 2014, 333(18): 4152-4169. doi: 10.1016/j.jsv.2014.03.032 [6] 王开云, 翟婉明. 提速和高速铁路曲线轨道轮轨动态相互作用性能匹配研究[J]. 中国铁道科学, 2014, 35(1): 142-144. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK201401024.htmWANG Kai-yun, ZHAI Wan-ming. Study on performance matching of wheel-rail dynamic interaction on curved track of speed-raised and high-speed railways[J]. China Railway Science, 2014, 35(1): 142-144. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK201401024.htm [7] DAI Jian, ANG K K. Steady-state response of a curved beam on a viscously damped foundation subjected to a sequence of moving loads[J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2015, 229(4): 375-394. doi: 10.1177/0954409714563366 [8] YANG Y B, WU C M, YAU J D. Dynamic response of a horizontally curved beam subjected to vertical and horizontal moving loads[J]. Journal of Sound and Vibration, 2001, 242(3): 519-537. doi: 10.1006/jsvi.2000.3355 [9] LI Wei, XIAO Guang-wen, WEN Ze-feng, et al. Plastic deformation of curved rail at rail weld caused by train-track dynamic interaction[J]. Wear, 2011, 271(1/2): 311-318. [10] 李克飞, 刘维宁. 基于变速及曲线车轨耦合频域解析模型的地铁减振轨道动力特性研究[J]. 中国铁道科学, 2013, 34(6): 131-133. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK201306023.htmLI Ke-fei, LIU Wei-ning. Study on the dynamic characteristics of metro vibration mitigation track based on frequency-domain analytical model of coupled vehicle & track in variable-speed and curved sections[J]. China Railway Science, 2013, 34(6): 131-133. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTK201306023.htm [11] 杜林林, 刘维宁, 刘卫丰, 等. 曲线轨道钢轨横向振动频域响应特性研究[J]. 铁道学报, 2021, 43(6): 95-103. https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB202106013.htmDU Lin-lin, LIU Wei-ning, LIU Wei-feng, et al. Study on lateral dynamic response of curved track in frequency domain[J]. Journal of the China Railway Society, 2021, 43(6): 95-103. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB202106013.htm [12] 刘卫丰, 杜林林, 刘维宁. 横向固定谐振荷载下曲线轨道参数对钢轨位移响应的影响研究[J]. 振动工程学报, 2019, 32(5): 837-844. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDGC201905012.htmLIU Wei-feng, DU Lin-lin, LIU Wei-ning. Study on influence of parameters on displacement of curved track subjected to lateral non-moving harmonic load[J]. Journal of Vibration Engineering, 2019, 32(5): 837-844. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDGC201905012.htm [13] 刘卫丰, 杜林林, 刘维宁. 曲线轨道参数对钢轨振动衰减率的影响研究[J]. 振动与冲击, 2019, 38(3): 244-251. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201903035.htmLIU Wei-feng, DU Lin-lin, LIU Wei-ning. Effects of curved track parameters on rail vibration decay rate[J]. Journal of Vibration and Shock, 2019, 38(3): 244-251. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201903035.htm [14] 杜林林, 刘维宁, 刘卫丰, 等. 曲线轨道空间振动响应特性研究[J]. 土木工程学报, 2018, 51(9): 110-120. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC201809013.htmDU Lin-lin, LIU Wei-ning, LIU Wei-feng, et al. Study on dynamic characteristics of a curved track in 3 dimensions[J]. China Civil Engineering Journal, 2018, 51(9): 110-120. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC201809013.htm [15] THOMPSON D J. A continuous damped vibration absorber to reduce broad-band wave propagation in beams[J]. Journal of Sound and Vibration, 2008, 311(3/4/5): 824-842. [16] LIU H P, WU T X, LI Z G. Theoretical modelling and effectiveness study of rail vibration absorber for noise control[J]. Journal of Sound and Vibration, 2009, 323(3/4/5): 594-608. [17] 刘卫丰, 张厚贵, 陈嘉梁, 等. 北京地铁采用调频式钢轨减振器治理钢轨波磨的试验研究[J]. 振动工程学报, 2019, 32(4): 695-700. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDGC201904017.htmLIU Wei-feng, ZHANG Hou-gui, CHEN Jia-liang, et al. A test of treating rail corrugation by tuned rail damper for Beijing metro[J]. Journal of Vibration Engineering, 2019, 32(4): 695-700. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDGC201904017.htm [18] 陈嘉梁, 刘维宁, 刘卫丰, 等. 北京地铁DTVI2扣件钢轨波磨整治措施的试验研究[J]. 机械工程学报, 2018, 54(4): 64-69. https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201804011.htmCHEN Jia-liang, LIU Wei-ning, LIU Wei-feng, et al. Demonstration test on treatment solution against rail corrugation occurs at DTVI2 fastener track sections in Beijing metro[J]. Journal of Mechanical Engineering, 2018, 54(4): 64-69. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201804011.htm [19] 盛曦, 曾会柯, 石灿, 等. 声子晶体型浮置板轨道低频减振性能研究[J]. 振动与冲击, 2022, 41(12): 36-42, 107. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ202212006.htmSHENG Xi, ZENG Hui-ke, SHI Can, et al. A study on low-frequency vibration-mitigation performance of a phononic crystal floating slab track[J]. Journal of Vibration and Shock, 2022, 41(12): 36-42, 107. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ202212006.htm [20] ASAI T, ARAKI Y, IKAGO K. Energy harvesting potential of tuned inertial mass electromagnetic transducers[J]. Mechanical Systems and Signal Processing, 2017, 84: 659-672. doi: 10.1016/j.ymssp.2016.07.048 [21] SUN Li-min, HONG Dong-xiao, CHEN Lin. Cables interconnected with tuned inerter damper for vibration mitigation[J]. Engineering Structures, 2017, 151: 57-67. doi: 10.1016/j.engstruct.2017.08.009 [22] LAURENTIU M, AGATHOKLIS G. The tuned mass- damper-inerter for harmonic vibrations suppression, attached mass reduction, and energy harvesting[J]. Smart Structures and Systems, 2017, 19(6): 665-678. [23] YILMAZ C, KIKUCHI N. Analysis and design of passive band-stop filter-type vibration isolators for low-frequency applications[J]. Journal of Sound and Vibration, 2006, 291(3/4/5): 1004-1028. [24] LI Jing-ru, LI Sheng. Generating ultra wide low-frequency gap for transverse wave isolation via inertial amplification effects[J]. Physics Letters A, 2018, 382(5): 241-247. doi: 10.1016/j.physleta.2017.11.023 [25] XI Cheng-yang, DOU Ling-ling, MI Yong-zhen, et al. Inertial amplification induced band gaps in corrugated-core sandwich panels[J]. Composite Structures, 2021, 267: 113918. doi: 10.1016/j.compstruct.2021.113918 [26] FENG Qing-song, YANG Zhou, GUO Wen-jie, et al. Enhanced suppression of vibrational wave transmission in structures with periodically attached absorbers exploiting amplitude magnification mechanisms[J]. European Journal of Mechanics—A, 2022, 95: 104666. doi: 10.1016/j.euromechsol.2022.104666 [27] NIE Rui, LI Tian-yun, ZHU Xiang, et al. A general Fourier formulation for in-plane and out-of-plane vibration analysis of curved beams[J]. Shock and Vibration, 2021: 5511884. [28] 冯青松, 杨舟, 郭文杰, 等. 基于人工弹簧模型的周期结构带隙计算方法研究[J]. 力学学报, 2021, 53(6): 1684-1697. https://www.cnki.com.cn/Article/CJFDTOTAL-LXXB202106015.htmFENG Qing-song, YANG Zhou, GUO Wen-jie, et al. Research on band gap calculation method of periodic structure based on artificial spring model[J]. Chinese Journal of Theoretical and Applied Mechanics, 2021, 53(6): 1684-1697. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-LXXB202106015.htm [29] DENG Jie, GUASCH O, ZHENG Ling. Reconstructed Gaussian basis to characterize flexural wave collimation in plates with periodic arrays of annular acoustic black holes[J]. International Journal of Mechanical Sciences, 2020, 194: 106179. [30] 杜林林. 地铁列车曲线运行车轨耦合解析模型及振源特性研究[D]. 北京: 北京交通大学, 2018.DU Lin-lin. Study on the theoretical model of coupled vehicle and track and the characteristics of vibration sources in a curved track[D]. Beijing: Beijing Jiaotong University, 2018. (in Chinese) [31] XIAO Xin-biao, LI Ya-guang, ZHONG Ting-sheng, et al. Theoretical investigation into the effect of rail vibration dampers on the dynamical behaviour of a high-speed railway track[J]. Journal of Zhejiang University—Science A, 2017, 18(8): 631-647. doi: 10.1631/jzus.A1600697 [32] 文永蓬, 纪忠辉, 翁琳, 等. 双重钢轨吸振器对轨道系统的振动抑制研究[J]. 机械工程学报, 2020, 56(12): 184-195. https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB202012023.htmWEN Yong-peng, JI Zhong-hui, WENG Lin, et al. Study on vibration suppression of track system via double rail vibration absorber[J]. Journal of Mechanical Engineering, 2020, 56(12): 184-195. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JXXB202012023.htm [33] LU Zheng, HUANG Biao, ZHANG Qi, et al. Experimental and analytical study on vibration control effects of eddy-current tuned mass dampers under seismic excitations[J]. Journal of Sound and Vibration, 2018, 421: 153-165. doi: 10.1016/j.jsv.2017.10.035 -
点击查看大图
图(15) / 表(1)
计量
- 文章访问数: 232
- HTML全文浏览量: 73
- PDF下载量: 33
- 被引次数: 0
